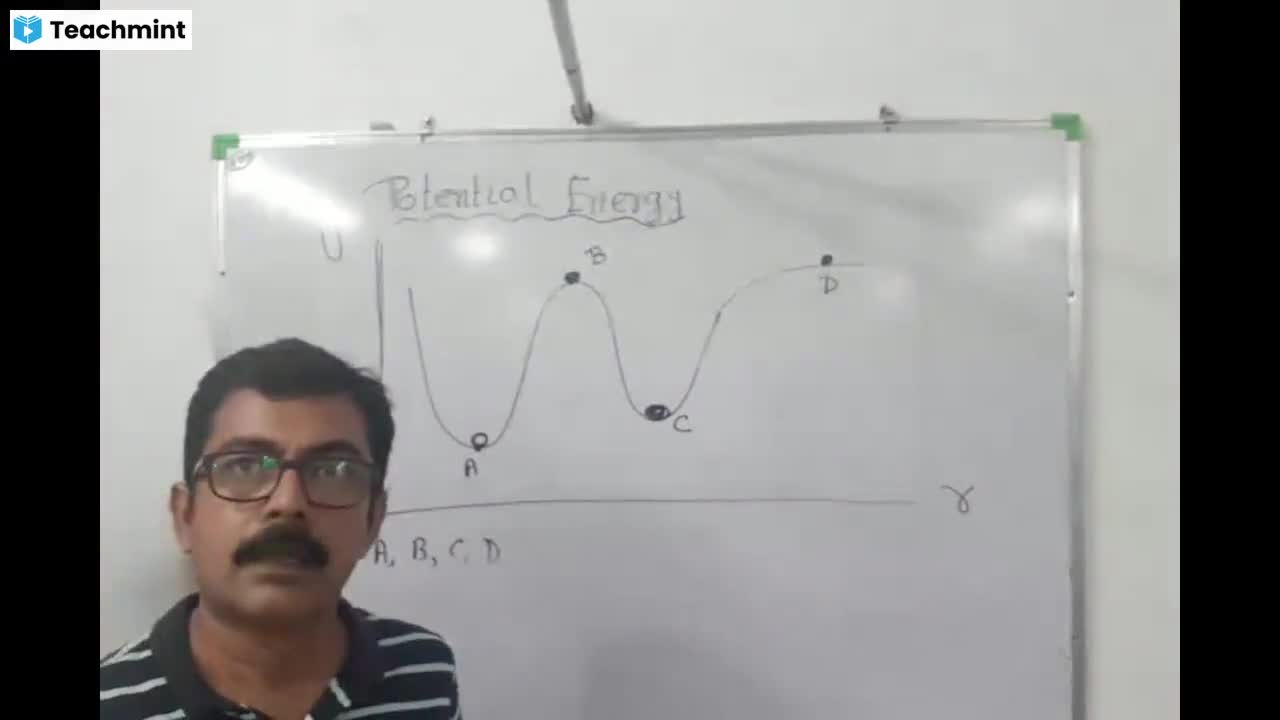
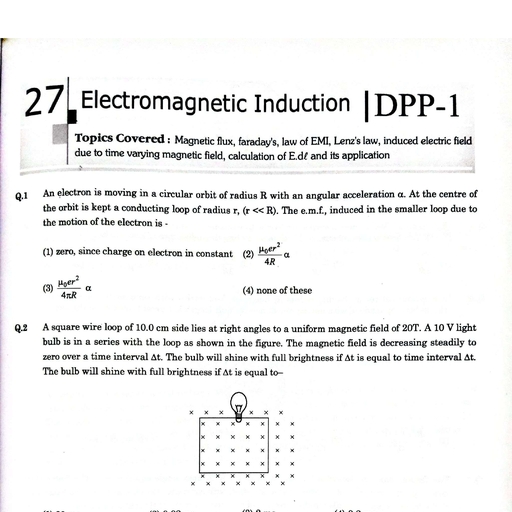
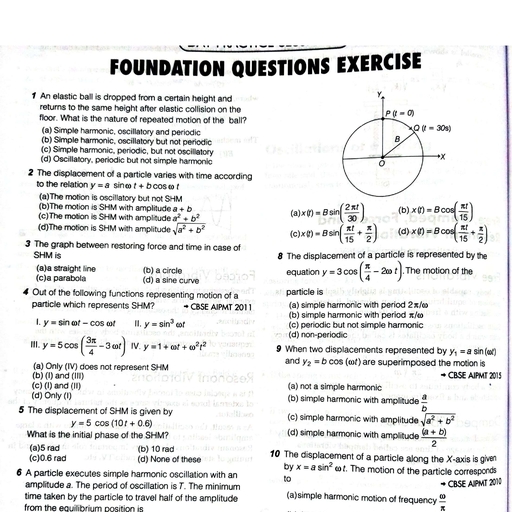
Page 1 :
Q2, , Q3, , Q4, , Q5, , Q6, , Q7, , Q8, , Q9, , , , 8 Circular Motion |DPP-1, , |, , Topics Covered : Circular Motion Kinematics + Relative Motion, , A particle is moving in a circular path. The acceleration and momentum of the particle at a certain, moment are a = (41 +3}) m/s? and p = (8i - 6j) kg-m/s. The motion of the particle is, , (1) Uniform cireular motion (2) accelerated circular motion, (8) decelerated circular motion (4) We cannot say anything with 4 and ponly, , If a body is accelerating, , (1) it may speed up (2) it may speed down, , (8) it may move with same speed . (4) it may move with same velocity, (a) a,b @)b,c (8) a,b, (4)b, ed, , A particle moves along a circle with a constant speed. If a is acceleration and E is kinetic energy of, the particle, then , (1) ais constant (2) E is constant (8) a is variable (4) E is variable, () a,b (2) b,c (8) a,d 4ocd, , A motorcyclist of mass m is to negotiate a curve of radius r with a speed v. The minimum value of the, coefficient of friction so that this negotiation may take place safely, is, , 2, (1) verg @ @ = @) &, er v vr, , A body is just being revolved in a vertical circle of radius R with a uniform speed. The string breaks, when the body is at the highest point. The horizontal distance covered by the body after the string, breaks is, , (1) 2R (QR (3) RY2 (4) 4R, , Two cars are going round curves, one car traveling at 60 km/hr and the other at 30 km/hr. Each car, experiences the same centripetal acceleration. The radii of the two curves are in the ratio :, (1) 4:1 (2) 2:1 (3) 1:2 (41:4, , If a cycle wheel of radius 4m completes one revolution in two seconds, the acceleration of the cycle in, ms~ (motion of cycle in uniform), (1) 4 (2) 4n2 (3) 2n? (4) 72, , A spaceman in training is rotated in a seat at the end of a horizontal rotating arm of length 5m. If he, can withstand accelerations upto 9g, then what is the maximum number of revolutions per second, permissible ? Take g = 10 m/s?, , (1) 18.5 rps (2) 1.35 rps (3) 0.675 rps (4) 6.75 rps, , A particie of mass m is moving in a circular path of constant radius r such that its centripetal, acceleration a, is varying with time as a, = k*rt‘, where k is a constant. The power delivered to the, particle by the forces acting on it is , (0 (2) mk?r?t? (3) ; mk?r2t2 (4) 2mk?r?t3

Page 2 :
Q.10 A bucket full of water is revolved in vertical circle of radius 2m. What should be the maximum tims., period of revolution so that the water doesn't fall of bucket (approximately)(1) 1 sec (2) 2 sec (3) 8 sec (4) 4 sec, , Q.11 A particle moves in x-y plane. The position vector of particle at any time t is F = {(2t)i + (2t?)}} m. The rate, of change of @ at time t = 2s. (where 0 is the angle which its velocity vector makes with positive x-axis) ig, , , , (a) = rad/s (2) a wndlls (3) “rad/s (4) srad/s, Answer & Solutions, 1.2) 2.(3) 3.(2) 4.(2) 5.(1) 6.(1) 7.(2) 8.(3) 9.(4) 10.(3), , 11.(1)

Page 3 :
Circular Motion | DPP-2, , Topics Covered: Circular Motion on Banked Road, , Q.1, , Q2, , Q.3, , Q.4, , Q.5, , Q6, , Q7, , Q.8, , Q.9, , A car is just on the point of slipping when traveling on level ground at a speed v around a bend of, radius r. Under the same road surface conditions, the car is just on the point of slipping when, traveling on level ground at a speed 2v around a bend of radius R. Then, , ()r=R (2)r=2R (3) R= 2r (4) R= 4r, , A car of mass m moves in a horizontal circular path of radius r metre. At an instant its speed is, V m/s and is increasing at a rate of a m/sec?. Then the acceleration of the car is :, , v2 2, @ a Q)a (3) ,Ja? (4) (4) yar, r r, , A motor car of mass m travels with a uniform speed v on a convex bridge of radius r. When the car is, at the middle point of the bridge, then the force exerted by the car on the bridge is :, , 2 2 2, (1) mg 2) mg + (3) mg-S @) mg 7, r, A rail track is banked for speed v by making the height of the outer rail h higher than that of inner., The distance between the rails is d. The radius of curvature of the track is r then h_v? a og v? h_v h _v?, (1) ==— 2) tansin = — (3) tant — = — 4) — = —, d rg @) d gO d yg > dg, The safe velocity required for scooterist negotiating a curve of radius 200 m on a road with the angle of, , repose of tan-! (0.2) will be(1) 20 km/hr (2) 200 m/s (8) 72 km/hr (4) 72 m/s, , For a heavy vehicle moving on a circular curve of a highway the road bed is banked at an angle 0, corresponding to a particular speed. The correct angle of banking of the road for vehicles moving at, , 60 km/hr will be - (If radius of curve = 0.1 km), (1) tan (0,283) (2) tan- (2. 83) (3) tan (0.05) (4) tan (0.5), , A car moves at a constant speed on a road as shown in figure. The normal force by the road on the, car is N, and N, when it is at the points A and B , A B, (1) N,=N, (2)N,>Ny (3) N,<N, (4) insufficient information, Acyclist bends while taking turn to, (1) reduce friction (2) generate required centripetal force, (3) reduce apparent weight (4) reduce speed, , A car moving on a horizontal road may be thrown out of the road in taking a turn :, (1) by the gravitational force, , (2) due to the lack of proper centripetal force, , (3) due to the rolling frictional force between the tyre and road, , (4) due to the reaction of the ground

Page 4 :
Q.10 A cyclist riding the bicycle at a speed of 14./3 m/s takes a turn around a circular road of radius 20, V3 m without skidding. Given, g = 9.8 m/s?, what is his inclination to the vertical ?, (1) 30° (2) 90° (3) 45° (4) 60°, , Q.11 A small ball describes a horizontal circle on the smooth inner surface of a conical funnel. If the, height of the plane of the circle above the vertex be 10 cm. What is the speed of the particle (1) 2 m/s (2) 4 m/s (3) 16 m/s (4) 1 m/s, , Answer & Solutions, , 1.(4) 2.(3) 3.03 4.(1 : : : : 7 ;, 11.(4) a a 5@) 64) 70) 8@) 92) 10

Page 5 :
circular Motion | DPP-3, , Topics, , Qi, , Q2, , Q3, , Q4, , Covered : Circular Motion is Vertical Plane, , shown in the figure. The body of mass m, of angular amplitude 0 while the other, the mass 4m and the horizontal surface, , Two bodies of mass m and 4m are attached with string as, hanging from a string of length / is executing oscillations, body is at rest. The minimum coefficient of friction between, , , , , , , , , , should be 4m, a ~, 2-cos8, 8 7 A, @ A= ") (2) 2 cos? (* 8) (cae oes *s) @ = 2eos0e, , = 1.2 m), as shown. At an, , An object attached to the end of a string swings in a vertical circle (R, the string has a magnitude, , instant when @= 30°, the speed of the object is 5.1 m/s and the tension in, of 20 N. What is the mass of the object ?, , , , (1) 2.0 kg (2) 1.5 ke 7()1.8 kg (4) L2 kg, , One end of a string of length 1.5 m is tied to the stone of mass 0.4 kg and the other end to a small pivot on, a smooth vertical board, What is the minimum speed of the stone required at its lowermost point so that, the string does not slack at any point in its motion along the vertical circle ?, , , , (1) 3.2 ms (2) 4.2 ms+ (3) 6.8 ms (4) 8.6 ms?, A small block is placed hh f radi, siding placed over a sphere of radius R. It leaves the sphere at, from top if it starts, ZA, R, qa & 2R P, ) Q) — (3) R (4) None