Page 1 :
Senior Secondary Course, , CHEMISTRY (313), , 1, , Course Coordinator, Dr. Rajeev Prasad, , NATIONAL INSTITUTE OF OPEN SCHOOLING, (An autonomous organisation under MHRD, Govt. of India), A-24-25, Institutional Area, Sector-62, NOIDA-201309 (U.P.), Website: www.nios.ac.in, Toll Free No. 18001809393
Page 4 :
Chairman’s Message, Dear learner,, As the needs of the society in general, and some groups in particular, keep on changing, with time, the methods and techniques required for fulfilling those aspirations also, have to be modified accordingly. Education is an instrument of change. The right type, of education at right time can bring about positivity in the outlook of society, attitudinal, changes to face the new/fresh challenges and the courage to face difficult situations., This can be very effectively achieved by regular periodic curriculum renewal. A static, curriculum does not serve any purpose, as it does not cater to the current needs and, aspirations of the individual and society., For this purpose only, educationists from all over the country come together at regular, intervals to deliberate on the issues of changes needed and required. As an outcome, of such deliberations, the National Curriculum Framework (NCF 2005) came out,, which spells out in detail the type of education desirable/needed at various levels of, education - primary, elementary, secondary or senior secondary., Keeping this framework and other national and societal concerns in mind, we have, currently revised the curriculum of Chemistry course at Senior Secondary Level, as, per the Common Core Curriculum developed by COBSE (Council of Boards of School, Education) and NCERT (National Council for Educational Research and Training), making it current and need based. Textual material production is an integral and, essential part of all NIOS programmes offered through open and distance learning, system. Therefore, we have taken special care to make the learning material user, friendly, interesting and attractive for you., I would like to thank all the eminent persons involved in making this material, interesting and relevant to your needs. I hope you will find it appealing and absorbing., On behalf of National Institute of Open Schooling, I wish you all a bright and, successful future., (Dr. S. S. Jena), Chairman, NIOS
Page 5 :
A Note From the Director, Dear Learner,, The Academic Department at the National Institute of Open Schooling tries to bring, new programmes every now and then in accordance with your needs and, requirements., The Chemistry course at Senior secondary level has now been revised as per the, Common Core Curriculum developed by COBSE (Council of Boards of School, Education) and NCERT (National Council for Educational Research and Training), making it current and need based., The National Curriculum Framework developed by the National Council for, Educational Research and Training was kept as a reference point. Leading experts, in the subject of the country were involved and with their active involvement, study, materials based on the new curriculum have been updated. Old, outdated information, has been removed and new, relevant things have been added., I am happy to place this new revised study material in Chemistry in your hands. I, hope you will find the new material that is now in your hands interesting and exciting., Any suggestions for further improvement are welcome., Let me wish you all a happy and successful future., , (Dr. Kuldeep Agarwal), Director (Academic), National Institute of Open Schooling
Page 6 :
A Word With You, Dear Learner,, Welcome to the Revised Chemistry Course of National Institute of Open Schooling based on, the National Common Core Curriculum. This Course has three volumes I and II consist of, theory portion and volume III is a practical manual., The first book is in your hands. This book comprises one lesson of module 1 on Some Basic, Concepts of Chemistry, three lessons of Module 2 on Atomic Structure and Chemical Bonding,, four lessons of Module 3 on States of Matter, two lessons of Module 4 on Chemical Energetics, and five lessons of module five on Chemical Dynamics., In Module 1 you will learn about the various units used to calculate the number of atoms,, molecules and ions involved in chemical reactions. Module 2 will enlighten you about what, lies inside the atoms and the different types of chemical bonds through which atoms combine., Module 3 deals with the various aspects of three states of matter viz, solid, liquid and gas. You, will also learn about the properties of solutions and colloids. Module 4 deals with chemical, reactions accompanied with energy changes and basic concepts like internal energy, enthalpy,, entropy and spontaneity. Module 5 highlights on the Characteristics of Chemical Dynamics,, catalysis interconversion of Chemical energy into electrical energy in electrochemistry,, electrochemical cells and fuel cell ., As a policy decision to reduce content load at the term end examination(public examination),, it has been decided that the lessons Periodic table and atomic properties, The Solid State,, Spontaneity of Chemical Reactions, Chemical Equilibrium and Adsorption and Catalysis, which are fundamental chapters in Senior Secondary Chemistry are for assessment through, Tutor Marked Assignments(TMA) and the rest lessons upto module 5 are for assessment, through Public Examination(PE) because these chapters are very critical for competitive, examinations., We hope you will enjoy the course and find it interesting., Wishing you happy learning., , Yours, (Rajeev Prasad), Course Coordinator, Chemistry, Email: aochem@nios.ac.in
Page 7 :
How to use the Study Material, Your learning material has been developed by a team of Chemistry experts in open and distance learning. A, consistent format has been developed for self-study. The following points will give you an idea on how to make, the best use of the print material., Title is an advance organisor and conveys an idea about the contents of the lesson. Reflect on it., Introduction highlights the contents of the lesson and correlates it with your prior knowledge as, well as the natural phenomena in operation in our immediate environment. Read it thoroughly., Objectives relate the contents to your desired achievements after you have learnt the lesson., Remember these., Content of the lesson has been divided into sections and sub-sections depending on thematic unity, of concepts. Read the text carefully and make notes on the side margin of the page. After completing, each section, answer intext questions and solve numerical problems yourself. This will give you an, opportunity to check your understanding. You should continue reading a section till such time that, you gain mastery over it., At some places you will find some text in italics and bold. This indicates that it is important. You, must learn them., Solved Examples will help you to understand the concepts and fix your ideas. In fact, problem, solving is an integral part of training in Chemistry. Do them yourself and note the main concept, being taught through a particular example., Activities are simple experiments which you can perform at your home or work place using readily, available (low cost) materials. These will help you to understand physics by doing. Do them, yourself and correlate your findings with your observations., Intext questions are based on the concepts discussed in every section. Answer these questions, yourself in the space given below the question and then check your answers with the model answers, given at the end of the lesson. This will help you to judge your progress. If you are not satisfied with, the quality and authenticity of your answers, turn the pages back and study the section again., What have you learnt is essentially summary of the learning points for quick recapitulation. You, may like to add more points in this list., Terminal exercises in the form of short, long and numerical questions will help you to develop a, perspective of the subject, if you answer these meticulously. Discuss your responses with your peers, or counsellors., Answers to intext questions : These will help you to know how correctly you have answered the, intext questions., Audio: For understanding difficult or abstract concepts, audio programmes are available on certain, content areas. You may listen to these on FM Gyanvani,Mukt Vidya Vani or may buy the CDs from, Priced Publication Unit, NIOS, Video: Video programmes on certain elements related to your subject have been made to clarify, certain concepts. You may watch these at your study center or may purchase these CDs from Priced, Publication Unit, NIOS., These are few selected websites that you can access for extended learning., Studying at a distance requires self-motivation, self-discipline and self-regulation. Therefore you must develop, regular study habit. Drawing a daily schedule will help you in this endeavour. You should earmark a wellventilated and well-lighted space in your home for your study.
Page 8 :
Course Overview, Module I, Module II, , Module III, , R, PA, , T, , Module IV, , Module V, , Module VI, , PA, , RT, , Some Basic Concepts of Chemistry, 1. Atoms, Molecules and Chemical Arithmetic, Atomic Structure and Chemical Bonding, 2. Atomic Structure, 3. Periodic Table and Periodicity in Properties, 4. Chemical Bonding, States of Matter, 5. The Gaseous State and Liquid State, 6. The Solid State, 7. Solutions, 8. Colloids, Chemical Energetics, 09. Chemical Thermodynamics, 10. Spontaneity of Chemical Reactions, Chemical Dynamics, 11. Chemical Equilibrium, 12. Ionic Equilibrium, 13. Electrochemistry, 14. Chemical Kinetics, 15. Adsorption and Catalysis, , Chemistry of Elements, 16. Occurrence and Extraction of Metals, 17. Hydrogen and s-Block Elements, 18. General Characteristics of the p-block Elements, 19. p-block Elements and their Compounds – I, 20. p-block Elements and their Compounds - II, 21. d-Block and f-Block Elements, 22. Coordination Compounds, Module VII Chemistry of Organic Compounds, 23. Nomenclature and General Principles, 24. Hydrocarbons, 25. Compounds of Carbon Containing Halogens, (Haloalkanes and Haloarenes), 26. Alcohols, Phenols and Ethers, 27. Aldehydes, Ketones and Carboxylic Acids, 28. Compounds of Carbon Containing Nitrogen, 29. Biomolecules, Module VIII Chemistry in Everyday Life, 30. Drugs and Medicines, 31. Soaps, Detergents and Polymers, 32. Environmental Chemistry
Page 9 :
Contents, Chemistry in the Service of Humanity, From Vedic Period to Modern India, Module and Lessons, Module I: Some Basic Concepts of Chemistry, 1. Atoms, Molecules and Chemical Arithmetic, Module II: Atomic Structure and Chemical Bonding, 2. Atomic Structure, 3. Periodic Table and Periodicity in Properties, 4. Chemical Bonding, Module III: States of Matter, 5. The Gaseous and Liquid State, 6. The Solid State, 7. Solutions, 8. Colloids, Module IV: Chemical Energetics, 9. Chemical Thermodynamics, 10. Spontaneity of Chemical Reactions, Module V: Chemical Dynamics, 11. Chemical Equilibrium, 12. Ionic Equilibrium, 13. Electrochemistry, 14. Chemical Kinetics, 15. Adsorption and Catalysis, Curriculum, Feedback Form, TMA : Tutor Marked Assignments, PE : Public Examination, , (i)-(iv), , Page No., , Mode of Assessment, TMA/PE, , 1, , PE, , 45, 76, 96, , PE, TMA, PE, , 135, 175, 207, 231, , PE, TMA, PE, PE, , 251, 276, , PE, TMA, , 291, 320, 353, 394, 417, 433, 447, , TMA, PE, PE, PE, TMA
Page 10 :
CHEMISTRY IN THE SERVICE OF HUMANITY, FROM VEDIC PERIOD TO MODERN INDIA, There can be no progress in science without chemistry. Chemical research is changing in a big, way. Chemistry is no longer making a few compounds and studying their properties. We now design, new materials with the properties that are desired by us. Some of the important problems faced, by humanity can eventually be solved by the use of chemistry. There are many areas in chemistry, related to biology as well as to advanced materials that constitute major directors of chemical, research today., India in its history of chemical research has a number of chemists who have enriched the field, with their contributions. They are not only the inspiration for the younger generations, but also, the founders of the field of chemistry, and its research in India., During Vedic period, Yajurveda clearly states that Gold, silver, Copper, Tin, Lead and Iron were, known to the vedic people. These materials were of use in society. Agriculture, use of plant products, and their growth necessitated the use of these metals. Lot of natural principles that have now been, liked to the Ideas of chemistry were adapted by vedic people. This is very clearly reflected in, Mahabharata and Arthashatra of Kautilya., Kautilya’s Arthashatra is a treasure of knowledge discussing about mines, metals, precious articles, like gems, rubys, pearls etc. A detailed description of mines and factories that deal elaborately, with occurrence, nature and purification of substances have been discussed in detail. It is stated, that in all ores, heaviness of the is indication of the metal content. This modern chemistry that state, that the ‘greater the density of the ore, greater is the metal content’., Arthashastra also describes the qualities of Gold and silver. Gold which is of the colour of lotus, filament, soft, lustrous and not producing any type of sound is stated to be the best. The reddish, yellow coloured metal is of medium quality and the red coloured one is the lowest quality., This indicates that the colour is taken as a measure of the purity or quality. As the red colour, increases, the copper content increases. Similarly, Gold contaminated with lead gives brittleness, to the metal and this material was used to mould on wooden anvils., Intellectuals of the society tried to identify and use different plants and other materials as medicines, for curing diseases and for longevity, of the society which they called as Rasayana. The word, Rasayana has been used as a synonym for chemistry in Sanskrit and other Indian languages., Metal and medicinal plant combinations and preparations started with Nagarjuna, the great, alchemist of India and the founder of Rasasastra and wrote number of books. 8th century16th century was the period where Rasa sastra classics were written by many saints or alchemists., Among them Vagbhata, Nityanatha, Manthana Bhairava, SomaDeva, Yasodhara, Chakrapani ,, Basavaraju etc., were well known., , (i)
Page 11 :
Modern chemistry research can utilize the ancient principles of Yantras, Medicinal plants,, Metallic, their combinations, and their formulations mentioned in Rasendra Mangalam and, Rasarnava of Nagarjuna, Ashtanga Hridayam, Rasaratna Samucchayam of Vagbhata,, Chakradatta of Chakrapani, Ananda Kanda of Manthana Bhairava, Rasa Ratnakaram of, Nityanatha, Rasendra Chudamani of Soma Deva, Rasa Hridaya Tantra of Govinda Bikshu,, Rasa Prakasa Sudhakara of Yasodhara , Madhava Nidana of Madhavakar, Sarangadhara, Samhita of Sarangadhara Mishra BhavaPrakasa of Bhava Mishra and Basavarajeeyam of, Basavaraju ., These Yantras have contributed very much during alchemy period in metallic chemistry in, understanding the nature of impurities in metals, minerals and liquids used as perfumes; in, beverages. These yantras are also used to make ksharas, the alkalis of modern chemistry, and Dyes from Plant sources., Modern Chemistry is enriched by our Esteemed Chemists. Some of the prominent ones, are:, Prafulla Chandra Ray is another name that is etched indelibly in the history of chemistry. He, was a great visionary who set up the first chemical factory in India which later became the Bengal, Chemical and Pharmaceutical Works Ltd., Prafulla Chandra was born on 2 August, 1861 in RaraliKatripura, a village in the district of Khalna which is now in Bangladesh. His early education took, place in the village school. Later he studied in schools in Kolkata and the Presidency College., His publications on mercurous nitrite and its derivatives brought him recognition from all over the, world. He inspired a generation of young chemists in India thereby building up an Indian school, of chemistry. Famous Indian scientists Meghnad Saha and Shanti Swarup Bhatnagar were among, his students., Prafulla Chandra Ray believed that the progress of India could be achieved only by industrialization., He was a scientist as well as an entrepreneur and is considered as the father of Indian, pharmaceutical industry., Shanti Swarup Bhatnagar was born on 21St February 1894 in Bhera in the district of Shapur, in Punjab (now in Pakistan). He obtained a DSc degree from the University of London where, he studied the surface tension of oils., Bhatnagar was conferred with the Order of British Empire, or the Knighthood, by the British, Government in 1941. He was made Knight Bachelor in 1943 and he was elected as the Fellow, of the Royal Society. As India attained Independence, Bhatnagar strived to provide the necessary, scientific assistance to the then fledgling industries through the CSIR laboratories. He received, Padma Vibhushan in 1954 from the Government of India., Shanti Swarup Bhatnagar played a significant role in building the post-independence science and, technology infrastructure and in the formation of India’s science policies. Today he is remembered, by SS Bhatnagar Award, the prestigeous award named after him and given by the CSIR for, , (ii)
Page 12 :
excellent achievement in The award is different fields of science. often termed as the Indian Nobel, Prize., Dr. C. N. R. Rao. Prof. Chintamani Nagesha Ramachandra Rao has inspired a generation of, chemists in the country. In 1967 he was awarded the Marlow Medal by the Faraday Society of, Englnd for outstanding contribution in Physical chemistry by a young scientist. In 1968 he received, the SS Bhatnagar Prize in Chemical Sciences given by the Council of Scientific and Industrial, Research, New Delhi. Rao rejoined Indian Institute of Science in late 1976 and began a new, department on Solid State and Statistical Chemistry. His passion is nanotechnology, especially, nanomaterials consisting of carbon molecules., Har Govind Khorana. He was a chemist turned biochemist and is known as the first scientist, to synthesize oligonucleotides, the chain of nucleotide, outside an organism. Today oligonucleotides, are indispensable tools in biochemisitry, helping scientists to understand the mechanism of protein, synthesis, genetic information transfer and other mechanisms of life. Khorana has won many awards, and honours for his achievements among which are Padma Bhushan, Membership of National, Academy of Sciences, USA and the Fellowship of the American Association for the Advancement, of Science., Another chemist who has attained immortality by his contribution to chemistry is Yellapragada, Subbarow. Born in a remote village of Andhra Pradesh, Subbarow travelled to USA to continue, his studies While studying there, he devised a technique for the estimation of proteins in solutions., The procedure is still being adopted by chemists all over the world, though in a modified form,, for the detection and measuring of proteins. Called Friske-Subbarow method, it is one of the, longstanding chemical techniques that is followed world over., An India-born structural biologist Dr. Ramakrishnan whose quest for scientific excellence took, him from undergraduate schools in India to graduate and post-doc studies in US and research, in UK was jointly awarded the Nobel Prize in Chemistry for work on proteins that control life., The Swedish Nobel Committee awarded the Prize to Dr Ramakrishnan, who is currently affiliated, with the MRC Laboratory of Molecular Biology in Cambridge, UK, for his work on proteinproducing ribosomes, and its translation of DNA information into life., The Saga of Indian chemists is continuing. As the scene changes from individuals to team work,, Indian chemists have time and again proven their prowess by developing alternative routes of, synthesis of essential drugs to help the health services in the country. The effort has also spawned, a vibrant drugs and pharmaceutical industry in the country are other areas where Indian chemists, have contributed their efforts. There have been so many changes and the future is exciting. There, are number of scientists working in various Universities, Indian Institutes of Technologies, Indian, Institute of Science and CSIR., Yet, the challenges of Chemistry are unending and our journey continues., , (iii)
Page 13 :
CAREER OPPORTUNITIES AFTER STUDYING CHEMISTRY, Chemical sciences (Chemistry) offer access to a wide range of careers. Chemistry is the study, of substances, their makeup and applications. In fact it is the study of all materials and is vital, to every aspect of our lives. Organic Chemistry, Inorganic Chemistry, Analytical chemistry and, Biochemistry are the main branches of chemistry. The career options in chemistry are practically, endless! However, employment options depend on how far the education, training and experience, taken. A chemistry or biochemistry degree can lead to career paths in professions such as medicine,, pharmacology, agriculture, chemical engineering, forensic science, etc., Some of the Important career opportunities after studying chemistry at senior secondary level is, as, , Petrochemical and, Pharmaceutical industries, Analytical chemist, , Clinical biochemist, Chemical development, engineer, , Forensic scientist, Scientific journalist, , CHEMISTRY, , Toxicologist, Laboratory assistant, , Teacher/Professor, , Research associate/, Research assistant, , Quality controller, , Textile Industry, Plastics and Polymer, Industry, , Biotechnology, , Biochemistry, , (iv)
Page 14 :
MODULE - I, SOME BASIC CONCEPTS OF CHEMISTRY, 1. Atoms, Molecules and Chemical Arithmetic
Page 16 :
NATIONAL INSTITUTE OF OPEN SCHOOLING, ACADEMIC DEPARTMENT, , SENIOR SECONDARY CHEMISTRY, CURRICULUM, , RATIONALE, According to present scheme of school education at Senior Secondary stage, chemistry emerges out, as a separate discipline. It is this stage where major emphasis is to be laid on providing suitable conceptual, foundation. The present Senior secondary level Chemistry (313) course at NIOS has now been revised, as per the Common Core Curriculum developed by COBSE (Council of Boards of School Education), and NCERT (National Council for Educational Research and Training) making it current and need based., The present chemistry course has been developed basically around the themes: Why do chemical, reactions occur? What is the quantitative relationship among reacting constituents in a chemical reaction?, How far and how fast will a chemical reaction proceed under a given set of conditions? Can we predict, whether a chemical reaction will occur or not? What is the relation between the structure of a chemical, substance and its functions/properties? In what way is a chemical reaction relevant for getting new types, of substances and materials for daily life and chemical industries?, Some interdisciplinary aspects have also been provided to make the course more meaningful and, functional., , OBJECTIVES OF THE COURSE, It is expected that a learner will be able to:, , CHEMISTRY, , 433
Page 17 :
z, , understand the principles, theories and laws of chemistry responsible for various chemical processes/, reactions,, , z, , realise the role of chemistry in production of many elements (metals/non-metals) and compounds, useful in industries and daily life,, , z, , appreciate the chemical nature of inorganic and organic substances around him/her,, , z, , choose various vocational, professional and applied courses of his/her choice based on knowledge, of chemistry gained., , Apart from this, the present course also aims at developing the following capacity in the learner:, z, , perform chemical calculations to know about the chemical reactions and chemical compounds,, , z, , explain chemical reactions, concepts and phenomenon,, , z, , develop awareness about uses and abuses of chemical substances,, , z, , develop skills arranging/setting apparatus, handling apparatus and chemicals properly, and, , z, , analyse and synthesise simple compounds., , SPECIAL FEATURES OF THE COURSE, The academic standard of the present chemistry course in comparable with the chemistry course of any, State Education Board or Central Board of Secondary Education, New Delhi. The rationale behind the, course is that every lesson has a role to play in understanding the holistic view of chemistry., The present course provides eight modules and a laboratory manual. A student appearing for senior, secondary examination is expected to offer all the eight modules and practicals., , COURSE, , STRUCTURE, , The Revised Chemistry Course has three parts, I and II consist of theory portion and part III is, a practical manual. Part I and II of theory portion has eight modules. Part-I consists of five modules, namely as : Some basic concepts of chemistry, Atomic Structure and Chemical Bonding, States of, matter, Chemical Energetics, and Chemical Dynamics. Part-II consists of three modules namely:, Chemistry of Elements, Chemistry of Organic Compounds, and Chemistry in Everyday Life. Each module, is further divided into different lessons., The number of modules, marks allotted to each module and suggested study hours is as follows:, , 434, , CHEMISTRY
Page 18 :
Module, , Marks Allotted, , Study Hours, , 1. Atoms Molecules and Chemical Arithmetic, , 4, , 13, , 2. Atomic Structure and Chemical Bonding, , 10, , 30, , 3. States of Matter, , 8, , 28, , 4. Chemical Energetics, , 6, , 23, , 5. Chemical Dynamics, , 12, , 36, , 6. Chemistry of Elements, , 18, , 60, , 7. Chemistry of Organic Compounds, , 18, , 60, , 8. Chemistry in Everyday Life, , 04, , 20, , 80, , 270, , Total, , COURSE DESCRIPTION, , z, , MODULE 1: SOME BASIC CONCEPTS OF, CHEMISTRY, Time: 13 Hrs, Marks: 04, , Mole, mass, volume relationship in chemical, reactions, , z, , Stoichiometry and calculations based on, stoichiometry., , z, , Limiting reagent concept and percentage yield, , Lesson 1: Atoms, Molecules and Chemical, Arithmatics, (Time allocation: 13 Hrs), z, , Importance and scope of chemistry, , z, , Historical approach to particulate nature of, matter., , z, , A simple idea of basic SI units, , z, , Laws of chemical combination, , z, , Dalton’s atomic theory: Concept of elements,, atoms and molecules, , z, , Mole Concept, , z, , Counting and weighing of atoms and molecules, , z, , Avogadro constant, , z, , Molar quantities, , z, , Atomic and molecular masses., , z, z, , MODULE 2: ATOMIC STRUCTURE AND, CHEMICAL BONDING, Time : 30 Hrs, Marks:10, Lesson 2 Atomic Structure, (Time allocation: 12 Hrs), z, , Fundamental particles of an atom: electron,, proton and neutron, , z, , Rutherford’s nuclear model, , z, , Atomic number and mass number, , z, , Isotopes and isobars, , z, , Line spectrum of H atom, , z, , Bohr model (no derivation), , z, , Wave particle duality and de Broglie relationship, , z, , Heisenberg’s uncertainty principle., , Chemical formula and percentage composition, , z, , The wave mechanical model – orbitals, , Empirical and molecular formulae, , z, , Quantum numbers, , CHEMISTRY, , 435
Page 19 :
z, , Types of orbitals (s, p, d,f): Shapes of s, p and, d orbitals, , MODULE 3: STATES OF MATTER, (Time : 28 Hrs), Marks: 08, , z, , Aufbau principle – electron configuration of, atoms, , Lesson 5 Gaseous and Liquid States, (Time allocation: 10 Hrs), , z, , Pauli’s exclusion principle, , z, , Hund’s rule, , z, , Stability of completely filled and half- filled, orbitals, , z, , The three states of matter, , z, , Intermolecular interaction and its types, , z, , General behaviour of gases: the gas laws, , z, , Ideal gas equation, , z, , Dalton’s law of partial pressure, , z, , Kinetic molecular theory of gases (no, derivation), , z, , Kinetic energy and Urms, , z, , Graham’s law of diffusion, , z, , Real gases – deviation from ideal gas behaviour, , z, , Vander Waals’ equation, , z, , Liquefaction of gases, , z, , Critical constants, , z, , Properties of Liquids, , z, , Vapour pressure, , z, , Surface tension, , Types of bonds and their characteristics (ionic, and covalent, hydrogen bonding), Born Haber, Cycle., , z, , Viscosity (Only qualitative idea, no mathematical, derivation), , z, , Gay-Lussac’s law of combining volumes, , z, , Bond parameters- bond order, bond length,, bond angle, bond enthalpy, , Lesson 6: The Solid State, (Time allocation: 8 Hrs), , z, , Bond polarity, dipole moment, covalent, character of ionic bond., , Lesson 3: Periodic Table and Periodicity in, Properties, (Time allocation: 6 Hrs), z Early attempts at classification of elements, z, , Long form of periodic table., , z, , IUPAC Nomenclature of elements with atomic, numbers>100, , z, , Variation in atomic properties (size: atomic and, ionic radii, ionization enthalpy, electron gain, enthalpy, valency and electronegativity), , Lesson 4: Chemical Bonding, (Time allocation: 12 Hrs), z, , z, , Why do atoms combine? (potential energy, diagram), , z, , Resonance., , z, , Shapes of molecules: VSEPR theory (upto 6, electron pairs only), , z, , Valence Bond Theory(VBT), , z, , Hybridization of atomic orbitals, , z, , Multiple bonds (s and p bonds), , z, , M.O. Theory: Homonuclear diatomic, molecules(H2,O2, F2,C2 ), 436, , z, , Nature of solid state, , z, , Classification of solids: amorphous and, crystalline, , z, , Classification of crystalline solids, , z, , Two dimensional lattice and unit cells, , z, , Packing in crystals, , z, , Unit Cells and their types, , z, , Packing efficiency: Packing Efficiency of Body, Centred Cubic Lattice, Packing Efficiency of, ccp and hcp Lattices, CHEMISTRY
Page 20 :
z, , Calculation of density of unit cell, , z, , Types of system, , z, , Structure of simple ionic compounds, , z, , Types of processes, , z, , Electrical and magnetic properties of solids, , z, , Properties of a system, , z, , Band theory of metals ,conductors,, semiconductors(n-and p-type semiconductors), , z, , Standard state of a substance, , z, , Exothermic and endothermic reactions, , z, , Thermochemical equations, , z, , First law of thermodynamics, , z, , Concepts of internal energy and enthalpy, change., , z, , Imperfection in solids (Frenkel and Schottky, defects), , Lesson 7: Solutions, (Time allocation: 5 Hrs), z, , Components of a solution, , z, , Heat and work, , z, , Concentration of solution: different modes of, expression, , z, , Standard enthalpy of reactions, , z, , z, , Types of solutions, , z, , Solubility of gases in liquids, , Enthalpy changes during physical(fusion,, vaporization, sublimation, and solution) and, chemical processes(atomization, ionization), , z, , Raoult’s law, , z, , Hess’s law and its application, , z, , Ideal and non-ideal solutions, , z, , Bond enthalpy., , z, , Colligative properties of solutions, , z, , Abnormal molecular mass: van’t Hoff factor, , Lesson 8: Colloids (Time allocation: 5 Hrs), , Lesson 10: Spontaneity of Chemical Reactions, (Time allocation: 10 Hrs), z, , Spontaneous and non-spontaneous processes, , z, , Concept of entropy, entropy change and, spontaneity, , z, , The colloidal solution, , z, , True solution, colloidal solution and suspension, , z, , Classification of colloids, , z, , 2nd law of thermodynamics, , z, , Preparation and properties of colloidal solutions, , z, , z, , Coagulation: Hardy Schultz Rule, , Gibbs energy change and spontaneity of a, chemical reaction, , z, , Application of colloids, , z, , Gibb’s energy change and equilibrium constant, , z, , Emulsions and Gels, , z, , 3rd law of thermodynamics and absolute entropy, , z, , Elementary idea of Nano materials, , MODULE 4: CHEMICAL ENERGETICS, (Time : 23 Hrs), Marks: 06, Lesson 9 Chemical Thermodynamics, (Time allocation: 13 Hrs), z, , System and surroundings, , CHEMISTRY, , MODULE 5: CHEMICAL DYNAMICS, (Time : 36 Hrs), Marks: 12, Lesson 11 Chemical Equilibrium, (Time allocation: 8 Hrs), z, , Static and dynamic equilibrium, , z, , Reversible and irreversible reactions, , 437
Page 21 :
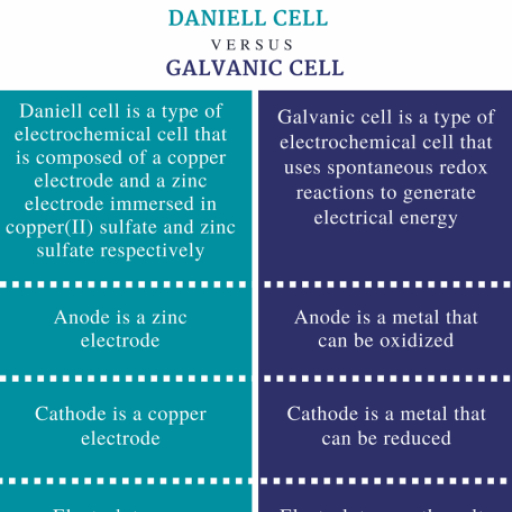
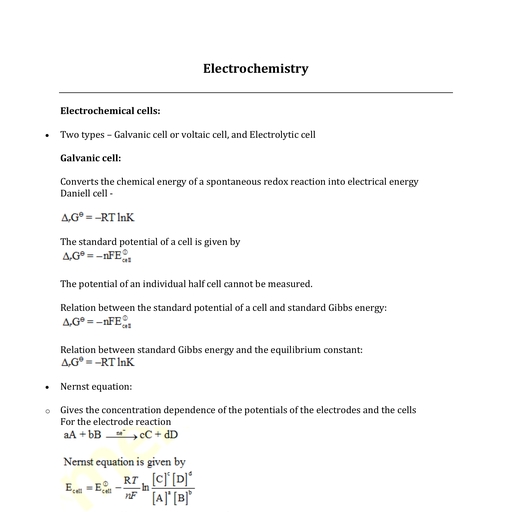
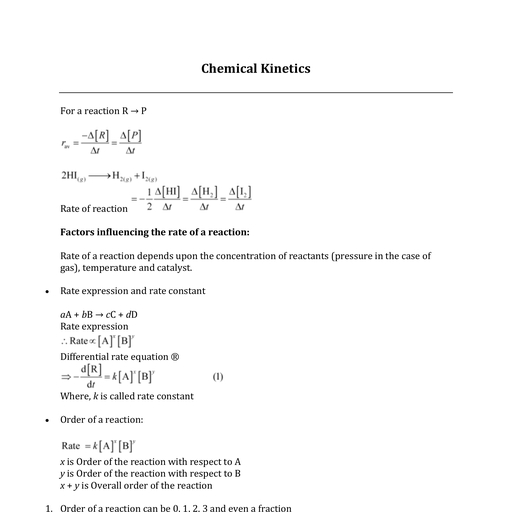
z, , Equilibrium in physical and chemical processes, , z, , Equilibrium in homogeneous and heterogeneous, systems, , z, , Law of mass action and equilibrium constants, , z, , Relationship between Kc and Kp, , z, , Characteristics of equilibrium constants, , z, , Factors affecting equilibrium state: Le, Chatelier’s principle, , Lesson 12: Ionic Equilibrium, (Time allocation: 10 Hrs), , z, , Dry cells, electrolytic cells and Galvanic cells;, lead accumulator, EMF of a cell, standard, electrode potential,, , z, , Electrochemical series and its application, , z, , Nernst equation and its application to chemical, cells, , z, , Relationship between E.M.F. and Gibbs free, energy change, , z, , Batteries and fuel cells, , z, , corrosion, , z, , General concepts of acids and bases, , Lesson 14: Chemical Kinetics, (Time allocation: 6 Hrs), , z, , Ionization constants of weak acids and bases, , z, , Rate of reaction: average and instantaneous, , z, , Strong and weak electrolytes, Ionization of, Weak Electrolytes., , z, , Factors affecting rate of reaction, , z, , Rate law and specific rate constant, , z, , Order and molecularity of a reaction, , z, , Zero order reaction, integrated rate equation, for a zero order reaction., , z, , Ionization of water, , z, , Concept of pH, , z, , Salt hydrolysis, , z, , Buffer solutions, , z, , First order reaction, , z, , Henderson’s equation, , z, , Half life period, , z, , Solubility product(Ksp) and its applications, , z, , Effect of temperature on reaction rate, , z, , Common ion effect, , z, , Collision theory, , Lesson 13: Electrochemistry, (Time allocation: 7 Hrs), z, , Oxidation and reduction (electron transfer, concept), , Lesson 15: Adsorption and Catalysis, (Time allocation: 5 Hrs), z, , Adsorption-Physical and Chemical, , z, , Adsorption Isotherms (Freundlich and, Langmuir), , z, , Oxidation number, , z, , Balancing of redox reaction by oxidation number, and ion electron methods, , z, , Catalysis-Homogenous and Heterogeneous, , z, , Activation energy, , z, , Electrochemical cells, , z, , Electrolytic conduction (conductance, conductivity, molar conductivity, effect of dilution,, Kohlrausch law), , MODULE 6: CHEMISTRY OF ELEMENTS, (Time : 60 Hrs), Marks: 18, , z, , Faraday’s Laws of Electrolysis, Products of, Electrolysis, 438, , Lesson 16: Occurrence And Extraction of, Metals, (Time allocation: 6 Hrs), z, , Occurrence of metals, , CHEMISTRY
Page 22 :
Important ores of some common elements :, , z, , Physical and chemical properties, , Na, Al, Sn, Pb, Ti, Fe, Cu, Ag and Zn, , z, , Inert pair effect, , z, , Mineral Wealth of India, , z, , Anomalous properties of first element, , z, , Principles of extraction of Aluminium, Copper,, Zinc and Iron from their ores., , z, , Lesson 19: p-block elements and their, Compounds-I, (Time allocation: 10 Hrs), , Lesson 17: Hydrogen and s-Block Elements, (Time allocation: 10 Hrs), , z, , General Introduction to p-block Elements, , z, , p-block elements of group 13 and 14., , History and Occurrence of Hydrogen, Position of Hydrogen in periodic table, Isotopes of Hydrogen, Hydrides, Uses of Hydrogen, Hydrogen Economy (Hydrogen as a fuel), Water; structure and aggregation of water;, Heavy water., Hydrogen Peroxide, Group I Alkali metals, Electronic configuration trends in atomic and, physical properties, Some general characteristics of group I, elements,, Occurrence and uses of the alkali metals,, Diagonal Relationship between Lithium and, Magnesium,, Preparation and Properties of Some Important, Compounds: Sodium carbonate, sodium, chloride, sodium hydroxide and sodium, hydrogencarbonate, Biological importance of sodium and potassium, Reactivity, electrode potential, Reaction with, oxygen, hydrogen, halogens and water;, Solubility and thermal stability of their oxo salts., , z, , Boron family-borax, boric acid, boron hydrides,, halides., , z, , Carbon family-allotropes (graphite, diamond,, elementary idea of fulleren), , z, , Oxides, carbides, halides, , z, , Nitrogen family-Ammonia, Nitric acid,, fertilizers( N & P), , z, , Fixation of nitrogen: industrial and biological, , z, , Nitrogenous and phosphatic fertilizers, , z, z, z, z, z, z, z, , z, z, z, , z, , z, z, , z, , z, z, , Lesson 18: General Characteristics of the, p-block Elements (Time allocation: 6 Hrs), z General Introduction to P- Block Elements, z, , Occurrence in nature and electronic, configuration, , CHEMISTRY, , Lesson 20: p-Block Elements and their, Compounds-II, (Time allocation: 8 Hrs), z, , Oxygen family-oxides, acidic, basic and, amphoteric, Ozone (Oxidizing Properties), , z, , Halogens- Hydrides, oxides and oxoacids of, chlorine., , z, , Bleaching powder-preparation and properties,, , z, , Interhalogen compounds., , z, , Noble gases-compounds of xenon: xenon, fluorides, oxides (preparation, structure), , Lesson 21: d-block and f-block Elements, (Time allocation: 12 Hrs), z, , Electronic configuration, , z, , General trends in the Chemistry of first row, transition elements, , z, , Properties-metallic character, oxidation state,, ionic radii, catalytic properties, coloured ions,, complex formation, magnetic properties,, interstitial compounds, alloy formation., , 439
Page 23 :
Preparation, properties and applications of, some important compounds: KMnO 4 ,, K2Cr2O7, , z, , Qualitative analysis of organic Compounds, , z, , Quantitative analysis of organic Compounds, , z, , f-Block elements- electronic configuration,, properties and lanthanide contraction, , z, , Occurrence, , z, , Lanthanoids :oxidation states, , z, , Actinoids : electronic configuration oxidation, states and comparison with lanthanides, , Lesson 24: Hydrocarbons, (Time allocation: 10 Hrs), z Definition and types of hydrocarbons (Alkane,, z Alkene, Alkyne, Arene), z IUPAC Nomenclature, z Preparation and properties of hydrocarbons, , z, , Lesson 22: Coordination Compounds, (Time allocation: 8 Hrs), , z, , Physical properties of hydrocarbons, , z, , Chemical properties of hydrocarbons (addition,, substitution, elimination, oxidation), , z, , Werner’s theory, , z, , Conformation (ethane only), , z, , Nomenclature, , z, , Stability of carbocations, , z, , Bonding-V.B. Approach, , z, , Carcinogenicity and Toxicity, , z, , CFT (Colour and magnetic properties), , z, , Structural and stereoisomerism, , z, , Importance of coordination compounds, , Lesson 25: Compounds of Carbon Containing, Halogens (Haloalkanes and Haloarenes), (Time allocation: 8 Hrs), , MODULE 7: CHEMISTRY OF ORGANIC, COMPOUNDS, (Time : 60 Hrs), Marks: 18, Lesson 23: Nomenclature and General, Principles, (Time allocation: 10 Hrs), z, , Rules of IUPAC Nomenclature, , z, , Types of bond fission, , z, , Types of reactions: substitution, addition,, elimination, oxidation/reduction (electrophilic, and nucleophillic), , z, , Electron displacement in a covalent bond:, inductive effect, electromeric effect, resonance, and hyperconjugation, and steric effect, , z, , Isomerism–structural and stereoisomerism, isomerism, , z, , Assigning absolute configuration (R-S and, D-L) to a chiral centre, , 440, , z, , IUPAC Nomenclature of halogen compounds, , z, , Preparation of haloalkanes and haloarenes, , z, , Physical and chemical properties and uses, , Lesson 26: Alcohol, Phenols and Ethers, (Time allocation: 7 Hrs), z, , Classification, , z, , IUPAC Nomenclature, , z, , Methods of preparation, , z, , Physical and Chemical properties, , Lesson 27: Aldehydes, Ketones and Carboxylic, acids., (Time allocation: 9 Hrs), z Carboxylic Acids and acid derivatives, z, , IUPAC Nomenclature, , z, , Preparation and Properties, , z, , Interconversion of acid derivatives, , CHEMISTRY
Page 24 :
Lesson 28: Compounds of Carbon Containing, Nitrogen, (Time allocation: 8 Hrs), z Nitro compounds - amines, z, , Classification of amines, , z, , IUPAC nomenclature, , z, , Preparation and properties, , Lesson 29: Biomolecules, (Time allocation: 8 Hrs), z, , Difference between DNA and RNA, , z, , Biomolecules in biological systems., , z, , Structures of proteins, lipids and carbohydrates, , z, , Vitamins and enzymes, , z, , Hormones (classification & functions), , z, , Classification of Polymers - Natural and, synthetic, , z, , Methods of Polymerization (addition and, condensation), copolymerization., , z, , Some important polymers: natural and synthetic, like polythene, nylon 66, polyesters, bakelite,, rubber., , z, , Biopolymers - Biodegradable and nonbiodegradable polymers., , Lesson 32: Environmental Chemistry, (Time allocation: 6 Hrs), z, , Environmental concerns, , z, , Pollutants, , z, , Air Pollution, , z, , Air pollutants, , z, , Photochemical Smog, , z, , Acid Rain, , z, , Green house effect, , z, , Global warming, , z, , Depletion of ozone layer, , z, , Control of Air Pollution, , z, , Water Pollution, , z, , Water pollutants, , z, , Biological oxygen demand, , z, , Prevention of water pollution, , z, , Soil Pollution, , Lesson 31: Soaps, Detergents and Polymers, (Time allocation: 10 Hrs), , z, , Green chemistry as an alternative tool for, reducing pollution,, , z, , Cleansing agents – soaps and detergents, , z, , Achievement of Green chemistry, , z, , Cleansing action of soap and detergents., , z, , Strategies to control environmental pollutions, , MODULE 8: CHEMISTRY IN EVERYDAY, LIFE, Marks: 04, (Time : 20 Hrs), Lesson 30: Drugs and Medicines, (Time allocation: 4 Hrs), z, , Distinction between drugs and medicines, , z, , Classification, , z, , Analgesics, tranquilizers, antiseptics,, disinfectants, antimicrobials, anti fertility drugs,, antibiotics, antacids, antihistamines, , z, , Elementary idea of antioxidants, Chemicals in, foods – Preservatives and Artificial sweetening, agents, Artificial Sweetening agents,, , CHEMISTRY, , 441
Page 25 :
CURRICULUM FOR PRACTICAL WORK, IN CHEMISTRY, , Objectives of the present course in practical, work are as follows:, 1. To develop and inculcate laboratory skills and, techniques, 2. To enable the student to understand the basic, chemical concepts., 3. To develop basic competence of analysing and, synthesising chemical compounds and mixtures., , method (Paraffin oil may be used as, bath)., 3. Preparation of dilute solutions, (i) Preparation of dilute solutions of known, concentration of sulphuric acid,, hydrochloric acid and nitric acid from, their stock solution., 4. Experiment related to pH change, , To meet these objectives three different types of, laboratory experiments are provided in the present, practical course., , (a) Determination of pH of following, substances by using a universal indicator, solution or pH papers., , 1. Experiment for developing laboratory skills/, techniques, , (i) Salt solution (ii) Acids and bases of, different dilutions (iii) Vegetable and fuit, juices, , 2. Concept based experiments, 3. Traditional experiments (for analysing and, synthesising chemicals), A. Introduction, B. General Safety Measures, C. List of Experiments, 1. Basic Laboratory Techniques, (i) Acquaintance with chemistry laboratory, and basic laboratory techniques (cutting,, bending and boring of glass tubes, sealing, of apparatus, filtration, distillation,, crystallisation, preparation calibration,, cleaning of glass apparatus and use of, burner etc.), (ii) Measurement of volume, length, mass, and density ., 2. Characterization of Chemical substances, (i) Determination of melting point of a solid, organic compound of low melting point, (below 100°C) by glass capillary tube, 442, , (b) Study of pH change by common-ion, effect in case of weak acids and weak, bases by above method (specific, examples of CH 3 COOH and, CH3COONa; and NH4OH and NH4Cl, may be taken)., 5. Surface Chemistry, (a) Preparation of lyophilic and lyophobic, sol. Lyophilic sol - starch Lyophobic sol, - aluminium hydroxide, ferric hydroxide., 6. Electrochemistry, Variation of cell potential in Zn/Zn2+|| Cu2+/Cu, with change in concentration of electrolytes, (CuSO4 or ZnSO4) at room temperature., 7. Thermochemistry, Any one of the following experiments, (i) To determine the enthalpy of dissolution, of copper sulpahte or potassium nitrate., , CHEMISTRY
Page 26 :
(ii) To determine the enthalpy of, neutralization of strong acid (HC1) with, strong base (NaOH), 8. Chemical Equilibrium, Study of the shift in equilibrium of the reaction, between ferric ions and thiocyanate ions by, increasing/decreasing the concentration of these, ions., 9. Chemical Kinetics, (a) Study of the effect of concentration on, the rate of reaction between sodium, thiosulphate and hydrochloric acid., (b) Study of the effect of temperature on the, rate of reaction between sodium, thiosulphate and hydrochloric acid., 10. Preparation of Inorganic Compounds, (i) Preparation of double salt of ferrous, ammonium sulphate or potash alum., (ii) Preparation of potassium ferric oxalate., 11. Preparation of Organic Compounds, Preparation of any one of the following, compounds, , Detection of nitrogen, sulphur, chlorine, bromine, and iodine in an organic compound, (combinations of halogens to be avoided). Not, more than two of the above elements should, be present in the given organic compound., 14. Characteristic Tests for cabohydrates; fats and, proteins and their detection, Study of simple reactions of cabohydrates; fats, and proteins. in pure form and detection of their, presence in given food stuffs., 15. Volumetric Analysis (Quantitative analysis), (a) preparation of solution of oxalic acid and, ferrous ammouium sulphate of known, molarity by weighing (non-evaluative)., Use of chemical balance to be, demonstrated., (b) A study of acid-base titration (single, titration only), (i) To find out the Molarity of given, NaOH solution by titrating against, standard solution of oxalic acid., Both the solutions to be provided, (c) A study of redox titrations (single titration, only), , (i) Acetanilide, (ii) Iodoform., 12. Chromatography, (a) Separation of coloured substances by, paper chromatography, and comparison, of their Rf values for a mixture of red and, blue ink or a black ink., OR, (b) Separation of coloured substances by, paper chromatography, and comparison, of their Rf values for juice of a flower or, grass., , CHEMISTRY, , 13. Detection of Elements, , (i) To find out the Molarity and, strength of given potassium, permanganate solution by titrating, against M/50 Mohr’s salt (Ferrous, ammonium sulphate ) solution. Both, the solutions to be provided., (ii) To find out the Molarity and, strength of given potassium, permanganate solution by titrating, against M/10 oxalic acid solution., Both the solutions to be provided., , 443
Page 27 :
Anions:, , 16. Qualitative Analysis, , CO3 2-,S2-, SO32-, SO4 2-, NO2-, NO3-,, C1-, Br-, I-, PO4 3-, C2O42-, CH3COO-, , Elementary qualitative analysis of a salt involving, detection of one cationic and one anionic, species from the following groups. (Salts, insoluble in hydrochloric acid excluded)., , 17. Tests for the functional groups present in organic, compounds, Test of functional groups present in given, unknown organic compounds, (i) Test of unsaturation, (ii) Test for Carboxylic, phenolic, aldehydic, and ketonic groups., , Cations:, Pb2+, Cu2+, As3+, A13+, Fe3+, Mn2+, Ni2+ ,, Zn2+ , Co2+, Ca2+, Sr2+, Ba2+, Mg2+, NH4+, , SCHEME OF PRACTICAL EXAMINATION, There will be a practical examination of 20 marks and 3 hours duration., The distribution of marks is as follows., Experiments, , Marks, , 1., , Salt Analysis, (one cation + one anion), , 4, , 2., , Volumetric Analysis, , 6, , (i), (ii), 3., , write-up in which student may be asked, to write brief method, indicator, equation, end point., , 2, , Set-up of experiment, , 2, , (iii) Results, , 2, , (i), , Detection of elements in an organic compound, , 2, , (ii), , Detection of functional group, , 2, OR, , Setting up of one experiment from experiments at serial, number 2 to 10, 12 and 13. 4, 4., , Viva-voce, , 3, , 5., , Record book, , 3, , Total, , 20, , 444, , CHEMISTRY
Page 28 :
SCHEME OF STUDY, The revised course in Chemistry provides you with package of learning opportunities which comprise, of:, z, , Printed Self Learning Material (SLM) is in three parts i.e. Part-1 , Part-2 and a laboratory manual., , z, , Supplementary Materials in the form of Audio and Video Programmes., , z, , Video tutorials in Chemistry available on the NIOS website (www.nios.ac.in) as well as YouTube., , z, , 30 Personal Contact Programme (PCP) sessions for theory part and 06 Personal Contact Programme, (PCP) sessions for practicals at your study centre. Please contact your study centre for the PCP, schedule or visit our NIOS website at www.nios.ac.in., , z, , Face-to-Face Personal Contact Programme (PCP) at your study centre, live Personal Contact, Programmes (PCPs) through audio streaming are webcast on Mukta Vidya Vani, which can be, accessed through NIOS website (www.nios.ac.in)., , SCHEME OF EVALUATION, The learner will be assessed through Continuous and Comprehensive Evaluation (CCE) in the form of, Tutor Marked Assignment (TMA) as well as Public Examination. The following table shows the details:, Mode of Evaluation, , Syllabus/Contents, , Duration, , Weightage, , Tutor Marked Assignment, , All Contents assigned, , Self Paced, , 20%, , (TMA), , for TMA, , Public/Final Examination, , All Contents assigned, for Public Examination, , 3 Hours, , 80%, , Practical Examination, , 3 Hours, , CHEMISTRY, , 445
Page 30 :
Atoms, Molecules and Chemical Arithmetic, , MODULE - 1, Some Basic Concepts of, Chemistry, , 1, Notes, , ATOMS, MOLECULES AND, CHEMICAL ARITHMETIC, , Chemistry is the study of matter and the changes it undergoes. Chemistry is, often called the central science, because a basic knowledge of chemistry is, essential for the study of biology, physics, geology, ecology, and many other, subjects., Although chemistry is an ancient science, its modern foundation was laid in the, nineteenth century, when intellectual and technological advances enabled, scientists to break down substances into ever smaller components and consequently, to explain many of their physical and chemical characteristics., Chemistry plays a pivotal role in many areas of science and technology e.g., in health, medicine, energy and environment, food, agriculture and new, materials., As you are aware, atoms and molecules are so small that we cannot see them, with our naked eyes or even with the help of a microscope. Any sample of matter, which can be studied consists of extremely large number of atoms or molecules., In chemical reactions, atoms or molecules combine with one another in a definite, number ratio. Therefore, it would be pertinent if we could specify the total, number of atoms or molecules in a given sample of a substance. We use many, number units in our daily life. For example, we express the number of bananas, or eggs in terms of ‘dozen’. In chemistry we use a number unit called mole, which is very large., With the help of mole concept it is possible to take a desired number of atoms/, molecules by weighing. Now, in order to study chemical compounds and reactions, in the laboratory, it is necessary to have adequate knowledge of the quantitative, relationship among the amounts of the reacting substances that take part and, CHEMISTRY, , 1
Page 31 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Notes, , Atoms, Molecules and Chemical Arithmetic, , products formed in the chemical reaction. This relationship is knows as, stoichiometry. Stoichiometry (derived from the Greek Stoicheion = element and, metron = measure) is the term we use to refer to all the quntatitative aspects of, chemical compounds and reactions. In the present lesson, you will see how, chemical formulae are determined and how chemical equations prove useful in, predicting the proper amounts of the reactants that must be mixed to carry out a, complete reaction. In other words we can take reactants for a reaction in such a, way that none of the reacting substances is in excess. This aspect is very vital in, chemistry and has wide application in industries., , OBJECTIVES, After reading this lesson you will be able to :, , 2, , z, , explain the scope of chemistry;, , z, , explain the atomic theory of matter;, , z, , state the laws of chemical combinaton;, , z, , explain Dalton’s atomic theory;, , z, , define the terms element, atoms and molecules., , z, , state the need of SI units;, , z, , list base SI units;, , z, , explain the relationship between mass and number of particles;, , z, , define Avogadro’s constant and state its significance;, , z, , calculate the molar mass of different elements and compounds;, , z, , define molar volume of gases at STP., , z, , define empirical and molecular formulae;, , z, , differentiate between empirical and molecular formulae;, , z, , calculate precentage by mass of an element in a compound and also work out, empirical formula from the percentage composition;, , z, , establish relationship between mole, mass and volume;, , z, , calculate the amount of substances consumed or formed in a chemical reaction, using a balanced equation and mole concept, and, , z, , explain the role of limiting reagent in limiting the amount of the products, formed., CHEMISTRY
Page 32 :
Atoms, Molecules and Chemical Arithmetic, , MODULE - 1, , 1.1 SCOPE OF CHEMISTRY, , Some Basic Concepts of, Chemistry, , Chemistry plays an important role in all aspects of our life. Let us discuss role, of chemistry in some such areas., 1.1.1 Health and Medicine, Three major advances in this century have enabled us to prevent and treat, diseases. Public health measures establishing sanitation systems to protect vast, numbers of people from infectious diseases; surgery with anesthesia, enabling, physicians to cure potentially fatal conditions, such as an inflamed appendix; and, the introduction of vaccines and antibiotics that made it possible to prevent, diseases spread by microbes. Gene therapy promises to be the fourth revolution, in medicine. (A gene is the basic unit of inheritance.) Several thousand known, conditions, including cystic fibrosis and hemophilia, are carried by inborn, damage to a single gene. Many other ailments, such as cancer, heart disease,, AIDS, and arthritis, result to an extent from impairment of one or more genes, involved in the body’s defenses. In gene therapy, a selected healthy gene is, delivered to a patient’s cell to cure or ease such disorders. To carry out such, a procedure, a doctor must have a sound knowledge of the chemical properties, of the molecular components involved., , Notes, , Chemists in the pharmaceutical industry are researching potent drugs with few, or no side effects to treat cancer, AIDS, and many other diseases as well as, drugs to increase the number of successful organ transplants. On a broader scale,, improved understanding of the mechanism of ageing will lead to a longer and, healthier lifespan for the world’s population., 11.2 Energy and the Environment, Energy is a by-product of many chemical processes, and as the demand for, energy continues to increase, both in technologically advanced countries like the, United States and in developing ones like India. Chemists are actively trying, to find new energy sources. Currently the major sources of energy are fossil, fuels (coal, petroleum, and natural gas). The estimated reserves of these fuels, will last us another 50-100 years at the present rate of consumption, so it is, urgent that we find alternatives., Solar energy promises to be a viable source of energy for the future. Every year, earth’s surface receives about 10 times as much energy from sunlight as is, contained in all of the known reserves of coal, oil, natural gas, and uranium, combined. But much of this energy is “wasted” because it is reflected back into, space. For the past thirty years, intense research efforts have shown that solar, energy can be harnessed effectively in two ways. One is the conversion of, CHEMISTRY, , 3
Page 33 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Notes, , Atoms, Molecules and Chemical Arithmetic, , sunlight directly to electricity using devices called photovoltaic cells. The other, is to use sunlight to obtain hydrogen from water. The hydrogen can then be, fed into a fuel cell to generate electricity. Although our understanding of the, scientific process of converting solar energy to electricity has advanced, the, technology has not yet improved to the point where we can produce electricity, on a large scale at an economically acceptable cost. By 2050, however, it has, been predicted that solar energy will supply over 50 percent of our power needs., Another potential source of energy is nuclear fission, but because of environmental, concerns about the radioactive wastes from fission processes, the future of the, nuclear industry is uncertain. Chemists can help to devise better ways to dispose, of nuclear waste. Nuclear fusion, the process that occurs in the sun and other, stars, generates huge amounts of energy without producing much dangerous, radioactive waste. In another 50 years, nuclear fusion will likely be a significant, source of energy., Energy production and energy utilization are closely tied to the quality of our, environment. A major disadvantage of burning fossil fuels is that they give off, carbon dioxide, which is a greenhouse gas (that is, it promotes the heating of, Earth’s atmosphere), along with sulfur dioxide and nitrogen oxides, which result, in acid rain and smog. Harnessing solar energy has no such detrimental effects, on the environment. By using fuel-efficient automobiles and more effective, catalytic converters, we should be able to drastically reduce harmful auto, emissions and improve the air quality in areas with heavy traffic. In addition,, electric cars, powered by durable, long-lasting batteries, should be more, prevalent in the next century, and their use will help to minimize air pollution., 1.1.3 Materials and Technology, Chemical research and development in the twentieth century have provided us, with new materials that have profoundly improved the quality of our lives and, helped to advance technology in countless ways. A few examples are polymers, (including rubber and nylon), ceramics (such as cookware), liquid crystals (like, those in electronic displays), adhesives, and coatings (for example, latex paint)., What is in store for the near future? One likely possibility is room-temperature, superconductors. Electricity is carried by copper cables, which are not perfect, conductors. Consequently, about 20 percent of electrical energy is lost in the, form of heat between the power station and our homes. This is a tremendous, waste. Superconductors are materials that have no electrical resistance and can, therefore conduct electricity with no energy loss., , 4, , CHEMISTRY
Page 34 :
Atoms, Molecules and Chemical Arithmetic, , 1.1.4 Food and Agriculture, How can the world’s rapidly increasing population be fed? In poor countries,, agricultural activities occupy about 80 percent of the workforce and half of an, average family budget is spent on foodstuffs. This is a tremendous drain on a, nation’s resources. The factors that affect agricultural production are the, richness of the soil, insects and diseases that damage crops, and weeds that, compete for nutrients. Besides irrigation, farmers rely on fertilizers and, pesticides to increase crop yield., , MODULE - 1, Some Basic Concepts of, Chemistry, , Notes, , 1.2 PARTICULATE NATURE OF MATTER, Chemistry deals with study of structure and composition of matter. Since ancient, time people have been wondering about nature of matter. Suppose we take a, piece of rock and start breaking it into smaller and smaller particles can this, process go on far ever resulting in smaller and smaller particles or would it come, to stop when such particles are formed which can no longer to broken into still, smaller particles? Many people including Greek philosophers Plato and Aristotle, believed that matter is continuous and the process of subdivision of matter can, go on., On the other hand, many people believed that the process of subdivision of mater, can be repeated only a limited nuimber of times till such particles are obtained, which cannot be further subdivided. They believed that mattr is composed of, large number of very tiny particles and thus has particle naturew. The smallest, indivisible particles of matter were given the name ‘atom’ from the Greek word, “atoms” meaning ‘indivisible’. It is generally agreed that the Greek philosopher, Leucippus and his student Democritus were the first to propose this idea, about, 440 B.C.. However, Maharshi Kanad had propounded the atomic concept of, matter earlier (500 BC) and had named the smallest particle of matter as, "PARMANU"., , 1.3 LAWS OF CHEMICAL COMBINATIONS, There was tremendous progress in Chemical Sciences after 18th century. It arose, out of an interest in the nature of heat and the way things burn. Major progress, was made through the careful use of chemical balance to determine the change, in mass that occurs in chemical reactions. The great French Chemist Antoine, Lavoisier used the balance to study chemical reactions. He heated mercury in, a sealed flask that contained air. After several days, a red substance mercury, (II) oxide was produced. The gas remaining in the flask was reduced in mass., The remaining gas was neither able to support combustion nor life. The, remaining gas in the flask was identified as nitrogen. The gas which combined, with mercury was oxygen. Further he carefully performed the experiment by, CHEMISTRY, , 5
Page 35 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Notes, , Atoms, Molecules and Chemical Arithmetic, , taking a weighed quantity of mercury (II) oxide. After strong heating, he found, that mercury (II) oxide, red in colour, was decomposed into mercury and, oxygen. He weighed both mercury and oxygen and found that their combined, mass was equal to that of the mercury (II) oxide taken. Lavoisier finally came, to the conclusion that in every chemical reaction, total masses of all the, reactants is equal to the masses of all the products. This law is known as the, law of conservation of mass., There was rapid progress in science after chemists began accurate determination, of masses of reactants and products. French chemist Claude Berthollet and, Joseph Proust worked on the ratio (by mass) of two elements which combine, to form a compound. Through a careful work, Proust demonstrated the, fundamental law of definite or constant proportions in 1808. In a given, chemical compound, the proportions by mass of the elements that compose, it are fixed, independent of the origin of the compound or its mode of, preparation., In pure water, for instance, the ratio of mass of hydrogen to the mass of oxygen, is always 1:8 irrespective of the source of water. In other words, pure water, contains 11.11% of hydrogen and 88.89% of oxygen by mass whether water, is obtained from well, river or from a pond. Thus, if 9.0 g of water are, decomposed, 1.0 g of hydrogen and 8.0 g of oxygen are always obtained., Furthermore, if 3.0 g of hydrogen are mixed with 8.0 g of oxygen and the mixture, is ignited, 9.0 g of water are formed and 2.0 g of hydrogen remains unreacted., Similarly sodium chloride contains 60.66% of chlorine and 39.34% of sodium, by mass whether we obtained it from salt mines or by crytallising it from water, of ocean or inland salt seas or synthesizing it from its elements sodium and, chlorine. Of course, the key word in this sentence is ‘pure’. Reproducible, experimental results are highlights of scientific thoughts. In fact modern science, is based on experimental findings. Reproducible results indirectly hint for a, truth which is hidden. Scientists always worked for findings this truth and in, this manner many theories and laws were discovered. This search for truth plays, an important role in the development of science., The Dalton’s atomic theory not only explained the laws of conservations of mass, and law of constant proportions but also predicted the new ones. He deduced, the law of multiple proportions on the basis of his theory. The law states that, when two elements form more than one compound, the masses of one, element in these compound for a fixed mass of the other element are in, the ratio of small whole numbers. For example, carbon and oxygen form two, compounds: carbon monoxide and carbon dioxide. Carbon monoxide contains, 1.3321 g of oxygen for each 1.0000 g of carbon, whereas carbon dioxide, contains 2.6642 g of oxygen for 1.0000 g of carbon. In other words, carbon, dioxide contains twice the mass of oxygen as is contained in carbon monoxide, , 6, , CHEMISTRY
Page 36 :
Atoms, Molecules and Chemical Arithmetic, , (2.6642 g = 2 × 1.3321 g) for a given mass of carbon. Atomic theory explains, this by saying that carbon dioxide contains twice as many oxygen atoms for a, given number of carbon atoms as does carbon monoxide. The deduction of law, of multiple proportions from atomic theory was important in convincing, chemists of the validity of the theory., , 1.4 DALTON’S ATOMIC THEORY, , MODULE - 1, Some Basic Concepts of, Chemistry, , Notes, , As we learnt earlier, Lavosier laid the experimental foundation of modern, chemistry. But the British chemist John Dalton (1766–1844) provided the basic, theory; all matter – whether element, compound, or mixture –is composed of, small particles called atoms. The postulates, or basic assumptions of Dalton's, theory are presented below in this section., 1.4.1 Postulates of Dalton's Atomic Theory, The English scientist John Dalton was by no means the first person to propose, the existence of atoms, as we have seen in the previous section, such ideas date, back to classical times. Dalton’s major contribution was to arrange those ideas, in proper order and give evidence for the existence of atoms. He showed that, the mass relationship expressed by Lavoisier and Proust (in the form of law of, conservation of mass and law of constant proportions) could be interpreted most, suitably by postulating the existence of atoms of the various elements., In 1803, Dalton published a new system of chemical philosophy in which the, following statements comprise the atomic theory of matter:, 1. Matter consists of indivisible atoms., 2. All the atoms of a given chemical element are identical in mass and in all, other properties., 3. Different chemical elements have different kinds of atoms and in particular, such atoms have different masses., 4. Atoms are indestructible and retain their identity in chemical reactions., 5. The formation of a compound from its elements occurs through the, combination of atoms of unlike elements in small whole number ratio., Dalton’s fourth postulate is clearly related to the law of conservation of mass., Every atom of an element has a definite mass. Also in a chemical reaction there, is rearrangement of atoms. Therefore after the reaction, mass of the product, should remain the same. The fifth postulate is an attempt to explain the law of, definite proportions. A compound is a type of matter containing the atoms of, , CHEMISTRY, , 7
Page 37 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Notes, , Atoms, Molecules and Chemical Arithmetic, , two or more elements in small whole number ratio. Because the atoms have, definite mass, the compound must have the elements in definite proportions by, mass., The Dalton’s atomic theory not only explained the laws of conservations of mass, and law of constant proportions but also predicted the new ones. He deduced, the law of multiple proportions on the basis of his theory. The law states that, when two elements form more than one compound, the masses of one, element in these compound for a fixed mass of the other element are in, the ratio of small whole numbers. For example, carbon and oxygen form two, compounds: Carbon monoxide and carbon dioxide. Carbon monoxide contains, 1.3321 g of oxygen for each 1.000g of carbon, whereas carbon dioxide contains, 2.6642 g of oxygen for 1.0000 g of carbon. In other words, carbon dioxide, contains twice the mass of oxygen as is contained in carbon monoxide (2.6642, g = 2 × 1.3321 g) for a given mass of carbon. Atomic theory explains this by, saying that carbon dioxide contains twice as many oxygen atoms for a given, number of carbon atoms as does carbon monoxide. The deduction of law of, multiple proportions from atomic theory was important in convincing chemists, of the validity of the theory., 1.4.2 What is an Atom?, As you have just seen in the previous section that an atom is the smallest particle, of an element that retains its (elements) chemical properties. An atom of one, element is different in size and mass from the atoms of the other elements. These, atoms were considered ‘indivisible’ by Indian and Greek ‘Philosophers’ in the, beginning and the name ‘atom’ was given as mentioned earlier. Today, we know, that atoms are not indivisible. They can be broken down into still smaller particles, although they lose their chemical identity in this process. But inspite of all these, developments atom still remains a building block of matter., 1.4.3 Molecules, A molecule is an aggregate of at least two atoms in a definite arrangement, held together by chemical forces (also called chemical bonds). It is smallest, particle of matter, an element or a compound, which can exist independently., A molecule may contain atoms of the same element or atoms of two or more, elements joined in a fixed ratio, in accordance with the law of definite, proportions stated. Thus, a molecule is not necessarily a compound, which, by, definition, is made up of two or more elements. Hydrogen gas, for example,, is a pure element, but it consists of molecules made up of two H atoms each., Water, on the other hand, is a molecular compound that contains hydrogen and, , 8, , CHEMISTRY
Page 38 :
MODULE - 1, , Atoms, Molecules and Chemical Arithmetic, , oxygen in a ratio of two H atoms and one O atom. Like atoms, molecules are, electrically neutral., The hydrogen molecule, symbolized as H2, is called a diatomic molecule, because it contains only two atoms. Other elements that normally exist as, diatomic molecules are nitrogen (N2) and oxygen (O2), as well as the Group, 17 elements-fluorine (F2), chlorine (Cl2), bromine (Br2), and iodine (I2). Of, course, a diatomic molecule can contain atoms of different elements. Examples, are hydrogen chloride (HCl) and carbon monoxide (CO)., , Some Basic Concepts of, Chemistry, , Notes, , The vast majority of molecules contain more than two atoms. They can be atoms, of the same element, as in ozone (O3), which is made up of three atoms of, oxygen, or they can be combinations of two or more different elements., Molecules containing more than two atoms are called polyatomic molecules., Like ozone, water (H2O) and ammonia (NH3) are polyatomic molecules., 1.4.4 Elements, Substances can be either elements or compounds. An element is a substance, that cannot be separated into simpler substances by chemical means. To date,, 118 elements have been positively identified. Eighty-three of them occur, naturally on Earth. The others have been created by scientists via nuclear, processes., For convenience, chemists use symbols of one or two, letters to represent the, elements. The first letter of a symbol is always capitalized, but the following, letter is not. For example, Co is the symbol for the element cobalt, whereas CO, is the formula for the carbon monoxide molecule. Table 1.l shows the names, and symbols of some of the more common elements; a complete list of the, elements and their symbols appears inside the front cover of this book. The, symbols of some elements are derived from their Latin names for example, Au, from auram (gold), Fe from ferrurn. (iron), and Na from natrium (sodium) while, most of them come from their English names., Table 1.1: Some Common Elements and Their Symbols, Name, , Symbol, , Name, , Symbol, , Name, , Aluminium, , Al, , Fluorine, , F, , Oxygen, , 0, , Arsenic, , As, , Gold, , Au, , Phosphorus, , P, , Barium, , Ba, , Hydrogen, , H, , Platinum, , Pt, , Bismuth, , Bi, , Iodine, , I, , Potassium, , K, , Bromine, , Br, , Iron, , Silicon, , Si, , CHEMISTRY, , Fe, , Symbol, , 9
Page 39 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Notes, , Atoms, Molecules and Chemical Arithmetic, , Calcium, , Ca, , Lead, , Pb, , Silver, , Ag, , Carbon, , C, , Magnesium, , Mg, , Sodium, , Na, , Chlorine, , Cl, , Manganese, , Mn, , Sulfur, , S, , Chromium, , Cr, , Mercury, , Hg, , Tin, , Sn, , Cobalt, , Co, , Nickel, , Ni, , Tungsten, , W, , Copper, , Cu, , Nitrogen, , N, , Zinc, , Zn, , Chemists use chemical formulas to express the composition of molecules and, ionic compounds in terms of chemical symbols. By composition we mean not, only the elements present but also the ratios in which the atoms are combined., , INTEXT QUESTIONS 1.1, 1. Chemistry plays a vital role in many areas of science and technology. What, are those areas?, 2. Who proposed the particulate nature of matter?, 3. What is law of conservation of mass?, 4. What is an atom?, 5. What is a molecule?, 6. Why is the symbol of sodium Na?, 7. How is an element different from a compound?, , 1.5 SI UNITS (REVISITED), Measurement is needed in every walk of life. As you know that for every, measurement a ‘unit’ or a ‘reference standard’ is required. In different countries,, different systems of units gradually developed. This created difficulties whenever, people of one country had to deal with those of another country. Since scientists, had to often use each other’s data, they faced a lot of difficulties. For a practical, use, data had to be first converted into local units and then only it could be used., In 1960, the ‘General Conference of Weights and Measures’, the international, authority on units proposed a new system which was based upon the metric system., This system is called the ‘International System of Units’ which is abbreviated as, SI units from its French name, Le Système Internationale d’Unitès. You have, learned about SI units in your earlier classes also and know that they are based, upon seven base units corresponding to seven base physical quantities. Units, needed for various other physical quantities can be derived from these base SI, units. The seven base SI units are listed in Table 1.2., 10, , CHEMISTRY
Page 40 :
MODULE - 1, , Atoms, Molecules and Chemical Arithmetic, , Some Basic Concepts of, Chemistry, , Table 1.2: SI Base Units, Physical Quantity, Length, Mass, Time, Electrical current, Temperature, Amount of substance, Luminous intensity, , Name of SI Unit, , Symbol for SI unit, , Metre, Kilogram, Second, Ampere, Kelvin, Mole, Candela, , m, kg, s, A, K, mol, cd, , Notes, , For measuring very large or very small quantities, multiples or sub-multiples of, these units are used. Each one of them is denoted by a symbol which is prefixed, to the symbol of the unit. For example, to measure long distances we use the unit, kilometre which is a multiple of metre, the base unit of length. Here kilo is the, prefix used for the multiple 103. Its symbol is k which is prefixed to the symbol of, metre, m . Thus the symbol of kilometre is km and, 1 km = 1.0 × 103 m = 1000 m, Similarly, for measuring small lengths we use centimetre (cm) and millimetre, (mm) where, 1 cm = 1.0 × 10–2 m = 0.01 m, 1 mm = 1.0 × 10–3 m = 0.001 m, Some prefixes used with SI units are listed in Table 1.3., Table 1.3: Some prefixes used with SI units, Prefix, , Symbol, , Meaning, , Example, , Tera, Giga, Mega, Kilo, Hecta, Deca, Deci, Centi, Milli, Micro, Nano, , T, G, M, k, h, da, d, c, m, µ, n, , 1012, 109, 106, 103, 102, 101, 10-1, 10–2, 10–3, 10–6, 10–9, , 1 terametre (Tm) = 1.0 ×1012 m, 1 gigametre (Gm) = 1.0 × 109 m, 1 megametre (Mm) = 1.0 × 106 m, 1 kilometre (km) = 1.0 × 103 m, 1 hectametre (hm) = 1.0 × 102 m, 1 decametre (dam) = 1.0 × 101 m, 1 decimetre (dm) = 1.0 × 10–1 m, 1 centimetre (cm) = 1.0 × 10–2 m, 1 millimetre (mm) = 1.0 × 10–3 m, 1 micrometre (µm) = 1.0 × 10–6 m, 1 nanometre (nm) = 1 × 10–9 m, , Pico, , p, , 10–12, , 1 picometre (pm) = 1 × 10–12m, , Before proceeding further try to answer the following questions:, , CHEMISTRY, , 11
Page 41 :
MODULE - 1, , Atoms, Molecules and Chemical Arithmetic, , Some Basic Concepts of, Chemistry, , INTEXT QUESTIONS 1.2, 1. Name the SI Unit of mass, 2. What symbol will represent 1.0 × 10–6 g ?, 3. Name the prefixes used for (i) 102 and (ii) 10–9, Notes, , (i) .................................................................................................................., (ii) ................................................................................................................., 4. What do the following symbols represent?, (i) Ms, , (ii) ms, , (i) .................................................................................................................., (ii) ................................................................................................................., , 1.6 RELATIONSHIP BETWEEN MASS AND NUMBER, OF PARTICLES, Suppose you want to purchase 500 screws. How, do you think, the shopkeeper, would give you the desired quantity? By counting the screws individually? No,, he would give the screws by weight because it will take a lot of time to count, them. If each screw weighs 0.8 g, he would weigh 400 g screws because it is the, mass of 500 screws (0.8 × 500 = 400 g). You will be surprised to note that the, Reserve Bank of India gives the desired number of coins by weight and not by, counting.This process of counting by weighing becomes more and more labour, saving as the number of items to be counted becomes large. We can carry out the, reverse process also. Suppose we take 5000 very tiny springs (used in watches), and weigh them. If the mass of these springs is found to be 1.5 g, we can conclude, that mass of each spring is 1.5 ÷ 5000 = 3 × 10–4 g., Thus, we see that mass and number of identical objects or particles are interrelated. Since atoms and molecules are extremely tiny particles it is impossible to, weigh or count them individually. Therefore we need a relationship between the, mass and number of atoms and molecules (particles). Such a relationship is, provided by ‘mole concept’., , 1.7 MOLE – A NUMBER UNIT, Mass of an atom or a molecule is an important property. However, while discussing, the quantitative aspects of a chemical reaction, the number of reacting atoms or, molecules is more significant than their masses.ACTIVITY 1.1, , 12, , CHEMISTRY
Page 42 :
Atoms, Molecules and Chemical Arithmetic, , It is observed experimently that iron and sulphur do not react with each other in, a simple mass ratio. When taken in 1:1 ratio by mass (Fe:S), some sulphur is left, unreacted and when taken in 2:1 ratio by mass (Fe:S) some iron is left unreacted., , MODULE - 1, Some Basic Concepts of, Chemistry, , Let us now write the chemical equation of this reaction, Fe + S → FeS, From the above chemical equation, it is clear that 1 atom of iron reacts with 1, atom of sulphur to form 1 molecule of iron (II) sulphide (FeS). It means that if, we had taken equal number of atoms of iron and sulphur, both of them would, have reacted completely. Thus we may conclude that substances react in a simple, ratio by number of atoms or molecules., , Notes, , From the above discussion it is clear that the number of atoms or molecules of a, substance is more relevant than their masses. In order to express their number we, need a number unit. One commonly used number unit is ‘dozen’, which, as you, know, means a collection of 12. Other number units that we use are ‘score’ (20), and ‘gross’(144 or 12 dozens). These units are useful in dealing with small numbers, only. The atoms and molecules are so small that even in the minute sample of any, substance, their number is extremely large. For example, a tiny dust particle, contains about 1016 molecules. In chemistry such large numbers are commonly, represented by a unit known as mole. Its symbol is ‘mol’ and it is defined as., A mole is the amount of a substance that contains as many elementary entities, (atoms, molecules or other particles) as there are atoms in exactly 0.012 kg, or 12 g of the carbon-12 isotope., The term mole has been derived from the Latin word ‘moles’ which means, a ‘heap’ or a ‘pile’. It was first used by the famous chemist Wilhelm Ostwald, more than a hundred years ago., Here you should remember that one mole always contains the same number of, entities, no matter what the substance is. Thus mole is a number unit for dealing, with elementary entities such as atoms, molecules, formula units, electrons etc.,, just as dozen is a number unit for dealing with bananas or oranges. In the next, section you will learn more about this number., , 1.8 AVOGADRO’S CONSTANT, In the previous section we have learned that a mole of a substance is that amount, which contains as many elementary entities as there are atoms in exactly 0.012, kilogram or 12 gram of the carbon-12 isotope. This definition gives us a method, by which we can find out the amount of a substance (in moles) if we know the, number of elementary entities present in it or vice versa. Now the question arises, CHEMISTRY, , 13
Page 43 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Atoms, Molecules and Chemical Arithmetic, , how many atoms are there in exactly 12 g of carbon-12. This number is determined, experimentally and its currently accepted value is 6.022045 × 1023. Thus 1 mol =, 6.022045 × 1023 entities or particles, or atoms or molecules., For all practical purposes this number is . rounded off to 6.022 × 1023., , Notes, , The basic idea of such a number was first conceived by an Italian scientist, Amedeo Avogadro. But, he never determined this number. It was, determinned later and is known as Avogadro’s constant in his honour., This number was earlier known as Avogadro’s number. This number alongwith, the unit, that is, 6.022 × 1023 mol–1 is known as Avogadro constant. It is, represented by the symbol NA. Here you should be clear that mathematically a, number does not have a unit. Avogadro’s number 6.022 × 1023 will not have any, unit but Avogradro’s constant will have unit of mol–1. Thus Avogradro’s constant,, NA = 6.022 × 1023 mol–1., Significance of Avogadro’s Constant, You know that 0.012 kg or 12 g of carbon –12 contains its one mole of carbon, atoms. A mole may be defined as the amount of a substance that contains 6.022 ×, 1023 elementary entities like atoms, molecules or other particles. When we say, one mole of carbon –12, we mean 6.022 × 1023 atoms of carbon –12 whose mass, is exactly 12 g. This mass is called the molar mass of carbon-12. The molar mass, is defined as the mass ( in grams) of 1 mole of a substance. Similarly, a mole of, any substance would contain 6.022 × 1023 particles or elementary entities. The, nature of elementary entity, however,depends upon the nature of the substance as, given below :, S.No., 1., , Type of Substance, Elements like Na, K, Cu which, , Elementary Entity, Atom, , exist in atomic form, 2., , Elements like O, N, H, which, , Molecule, , exist in molecular form (O2, N2, H2), 3., , Molecular compounds like NH3, H2O, CH4, , Molecule, , 4., , Ions like Na+, Cu2+, Ag+, Cl–, O2–, , Ion, , 5., , Ionic compounds like NaCl, NaNO3, K2SO4, , Formula unit, , Formula unit of a compound contains as many atoms or ions of different types, as is given by its chemical formula. The concept is applicable to all types of, compounds. The following examples would clarify the concept., , 14, , CHEMISTRY
Page 44 :
MODULE - 1, , Atoms, Molecules and Chemical Arithmetic, , Formula, , Atoms/ions present in one formula unit, , H2O, , Two atoms of H and one atom of O, , NH3, , One atom of N and three atoms of H, , NaCl, , One Na+ ion and one Cl– ion, , NaNO3, , One Na+ ion and one NO3– ion, , K2SO4, , Two K+ ions and one SO2—, ion, 4, , Ba3(PO4)2, , Three Ba2+ ions and two PO3—, ions, 4, , Some Basic Concepts of, Chemistry, , Notes, , Now, let us take the examples of different types of substances and correlate their, amounts and the number of elementary entities in them., 1 mole C, , = 6.022 × 1023, , C atoms, , 1 mole O2, , = 6.022 × 1023, , O2 molecules, , 1 mole H2O, , = 6.022 × 1023, , H2O molecules, , 1 mole NaCl, , = 6.022 × 1023, , formula units of NaCl, , 1 mole Ba2+ ions, , = 6.022 × 1023, , Ba2+ ions, , We may choose to take amounts other than one mole and correlate them with, number of particles present with the help of relation :, Number of elementary entities = number of moles × Avogadro’s constant, 1 mole O2, , = 1 × (6.022 × 1023), , = 6.022 × 1023 molecules of O2, , 0.5 mole O2, , = 0.5 × (6.022 × 1023) = 3.011 × 10, , 0.1 mole O2, , = 0.1 × (6.022 × 1023) = 6.022 ×10, , 23, , 22, , molecules of O2, molecules of O2, , INTEXT QUESTIONS 1.3, 1., , A sample of nitrogen gas consists of 4.22 × 1023 molecules of nitrogen., How many moles of nitrogen gas are there?, , 2., , In a metallic piece of magnesium, 8.46 × 1024 atoms are present. Calculate, the amount of magnesium in moles., , 3., , Calculate the number of Cl2 molecules and Cl atoms in 0.25 mol of Cl2 gas., , CHEMISTRY, , 15
Page 45 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Atoms, Molecules and Chemical Arithmetic, , 1.9 MOLE, MASS AND NUMBER RELATIONSHIPS, You know that, and, , 1 mol = 6.022 × 1023 elementary entities, , Molar mass = Mass of 1 mole of substance, = Mass of 6.022 × 1023 elementary entities., , Notes, , As discussed earlier the elementary entity can be an atom, a molecule, an ion or a, formula unit. As far as mole – number relationship is concerned it is clear that one, mole of any substance would contain 6.022 × 1023 particles (elementary entities)., For obtaining the molar mass, i.e., mole-mass relationship we have to use atomic, mass scale., 1.9.1 Atomic Mass Unit, By inernational agreement, a unit of mass to specify the atomic and molecular, masses has been defined. This unit is called atomic mass unit and its symbol is, ‘amu’. The mass of one C-12 atom, is taken as exactly 12 amu. Thus, C-12 atom, serves as the standard. The Atomic mass unit is defined as a mass exactly equal, to the 1/12th of the mass of one carbon-12 atom., 1 amu =, , Mass of one C -12 atom, 12, , Atomic mass unit is also called unified atomic mass unit whose symbol is ‘u’., Another name of atomic mass unit is dalton (symbol Da). The latter is mainly, used in biological sciences., 1.9.2 Relative Atomic and Molecular Masses, You are aware that atomic mass scale is a relative scale with C-12 atom (also, written as 12C) chosen as the standard. Its mass is taken as exactly 12. Relative, masses of atoms and molecules are the number of times each atom or molecules, is heavier than, , 1, th of the mass of one C-12 atom. Often, we deal with elements, 12, , and compounds containing isotopes of different elements. Therefore, we prefer, to use average masses of atoms and molecules. Thus, Relative atomic mass =, , Average mass of 1 atom of the element, 1, th of the mass of one C -12 atom, 12, , and Relative molecular mass =, , Average mass of 1 molecule of the substance, 1, th of the mass of one C -12 atom, 12, , Experiments show that one O-16 atom is 1.333 times as heavy as one C-12 atom., Thus, 16, , CHEMISTRY
Page 46 :
MODULE - 1, , Atoms, Molecules and Chemical Arithmetic, , Relative atomic mass of O-16 = 1.333 × 12 = 15.996 ~ 16.0, , Some Basic Concepts of, Chemistry, , The relative atomic masses of all elements have been determined in a similar, manner. Relative molecular masses can also be determined experimentally in a, similar manner . In case we know the molecular formula of a molecule, we can, calculate its relative molecular mass by adding the relative atomic masses of all, its constituent atoms. Let us calculate the relative molecular mass of water, H2O., Relative molecular mass of water, H2O = (2 × relative atomic mass of H) +, (relative atomic mass of O), , Notes, , = (2 × 1) + (16) = 2 + 16 = 18, The relative atomic and molecular masses are just numbers and dimensionless,, unit-less quantities., 1.9.3 Atomic, Molecular and Formula Masses, From the definition of atomic mass unit, we can calculate the atomic masses. Let, us again take the example of oxygen-16 whose relative atomic mass is 16. By, definition:, Relative atomic mass of O-16 = 16 =, , Since 1 amu =, ∴, , 16 =, , mass of one O -16 atom, 1, th the mass of one C -12 atom, 12, 1, th the mass of one C-12 atom, 12, , mass of one O -16 atom, 1 amu, , Mass of one O-16 atom = 16 amu, Or, , Atomic mass of O-16 = 16 amu., , From this example we can see that numerical value of the relative atomic mass, and atomic mass is the same. Only, the former has no unit while the latter has the, unit amu., Molecular and formula masses can be obtained by adding the atomic or ionic, masses of all the constituent atoms or ions of the molecule or formula unit, respectively. Let us understand these calculations with the help of following, examples., Example 1.1 : Calculate the molecular mass of ammonia, NH3., Solution : One molecule of NH3 consists of one N atom and three H atoms., Molecular mass of NH3 = (Atomic mass of N) + 3 (Atomic mass of H), CHEMISTRY, , 17
Page 47 :
MODULE - 1, , Atoms, Molecules and Chemical Arithmetic, , Some Basic Concepts of, Chemistry, , = [14 + (3 × 1)] amu, = 17 amu, Example 1.2 : Calculate the formula mass of sodium chloride (NaCl)., Solution : One formula unit of sodium chloride consists of one Na+ ion and one, Cl– ion., , Notes, , Formula mass of NaCl = (Ionic mass of Na+ ) + (Ionic mass of Cl–), = 23 amu + 35.5 amu, = 58.5 amu., You would have noticed in the above example that ionic mass of Na+ ion has, been taken as 23 amu which is the same as the atomic mass of Na atom. Since, loss or gain of few electrons does not change the mass significantly, therefore, atomic masses are used as ionic masses. Similarly we have taken ionic mass of, Cl– as 35.5 amu which is the same as the atomic mass of Cl–., 1.9.4 Molar Masses, We know that molar mass is the mass of 1 mol of the substance. Also, 1 mol of, any substance is the collection of its 6.022 × 1023 elementary entities. Thus, Molar mass = Mass of 6.022 × 1023 elementary entities., (i) Molar mass of an element, You know that the relative atomic mass of carbon–12 is 12. A 12g sample of it, would contain 6.022 × 1023 atoms. Hence the molar mass of C-12 is 12 g mol–, 1. For getting the molar masses of other elements we can use their relative atomic, masses., Since the relative atomic mass of oxygen -16 is 16, a 16 g sample of it would, contain 6.022 × 1023 oxygen atoms and would constitute its one mole. Thus, the, molar mass of O–16 is 16 g mol–1. Relative atomic masses of some common, elements have been listed in Table 1.4, Table 1.4 : Relative atomic masses of some elements, (upto 1st place of decimal), Element, , 18, , Relative, Atomic Mass, , Element, , Relative, Atomic Mass, , Hydrogen, H, , 1.0, , Phosphorus, P, , 31.0, , Carbon, C, , 12.0, , Sulphur, S, , 32.1, , Nitrogen, N, , 14.0, , Chlorine, Cl, , 35.5, , Oxygen, O, , 16.0, , Potassium, K, , 39.1, , Sodium, Na, , 23.0, , Iron, Fe, , 55.9, CHEMISTRY
Page 48 :
MODULE - 1, , Atoms, Molecules and Chemical Arithmetic, , Some Basic Concepts of, Chemistry, , (ii) Molar mass of a molecular substance, The elementary entity in case of a molecular substance is the molecule. Hence,, molar mass of such a substance would be the mass of its 6.022 × 1023 molecules,, which can be obtained from its relative molecular mass or by multiplying the, molar mass of each element by the number of its moles present in one mole of the, substance and then adding them., Let us take the example of water, H2O. Its relative molecular mass is 18. Therefore,, 18 g of it would contain 6.022 × 1023 molecules. Hence, its molar mass is 18 g, mol–1 . Alternately we can calculate it as :, , Notes, , Molar mass of water, H2O = (2 × molar mass of H) + (molar mass of O), = (2 × 1 g mol–1) + (16 g mol–1), = 18 g mol–1, Table 1.5 lists molecular masses and molar masses of some substances., Table 1.5 : Molecular masses and molar masses of some substances, Molar mass / (g mol–1), , Element or Compound, , Molecular mass / amu, , O2, , 32.0, , 32.0, , P4, , 124.0, , 124.0, , S8, , 256.8, , 256.8, , H2O, , 18.0, , 18.0, , NH3, , 17.0, , 17.0, , HCl, , 36.5, , 36.5, , CH2Cl2, , 85.0, , 85.0, , (iii) Molar masses of ionic compounds, Molar mass of an ionic compound is the mass of its 6.022 × 1023 formula units., It can be obtained by adding the molar masses of ions present in the formula unit, of the substance. In case of NaCl it is calculated as, Molar mass of NaCl = molar mass of Na+ + molar mass of Cl–, = (23 g mol–1) + (35.5 g mol–1), = 58.5 g mol–1, Let us take some more examples of ionic compounds and calculate their molar, masses., , CHEMISTRY, , 19
Page 49 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Atoms, Molecules and Chemical Arithmetic, , Example 1.3 : Calculate the molar mass of, (i) K2SO4, , (ii) Ba3(PO4)2, , Solution :, (i) Molar mass of K 2 SO 4 = (2 × molar mass of K+) + (molar mass of SO2–, ), 4, = (2 × molar mass of K+) +, (molar mass of S + 4 × molar mass of O), , Notes, , = [(2 × 39.1) + (32.1 + 4 × 16)] g mol–1], = (78.2 + 32.1 + 64) g mol–1 = 174.3 g mol–1, (ii) Molar mass of Ba3(PO4)2 = (3 × molar mass of Ba2+) +, 2 (molar mass of PO3–, ), 4, = (3 × molar mass of Ba2+) +, 2 (molar mass of P + 4 × molar mass of O), = [(3 × 137.3) + 2 (31.0 + 4 × 16.0)] g mol–1, = (411.9 + 190.0) g mol–1 = 601.9 g mol–1, Now you have learned about the mole, mass and number relationships for all, types of substances. The following examples would illustrate the usefulness of, these relationships., Example 1.4 : Find out the mass of carbon -12 that would contain 1.0 × 1019, carbon-12 atoms., Solution :, , Mass of 6.022 × 1023 carbon-12 atoms = 12 g, Mass of 1.0 × 1019 carbon-12 atoms =, , 12 × 1 × 1019, g, 6.022 × 1023, , = 1.99 × 10–4 g, Example 1.5 : How many molecules are present in 100 g sample of NH3?, Solution :, , Molar mass of NH3 = (14 + 3) g mol–1 = 17 g mol–1, , ∴ 17 g sample of NH3 contains 6.022 × 1023 molecules, 6.022 × 1023 molecule, × 100g, 17g, = 35.42 × 1023 molecules, , Therefore, 100 g sample of NH3 would contain, , = 3.542 × 1024 molecules, , 20, , CHEMISTRY
Page 50 :
MODULE - 1, , Atoms, Molecules and Chemical Arithmetic, , Example 1.6 : Molar mass of O is 16 g mol–1. What is the mass of one atom and, one molecule of oxygen?, , Some Basic Concepts of, Chemistry, , Solution : Mass of 1 mol or 6.022 × 1023 atoms of O = 16 g, ∴, , Mass of 1atom of O =, , 16g, 6.022 × 10 23, , = 2.66 × 10–23 g, , Notes, , Since a molecule of oxygen contains two atoms (O2),, its mass = 2 × 2.66 × 10–23 g = 5.32 × 10–23 g., , INTEXT QUESTIONS 1.4, 1., , Calculate the molar mass of hydrogen chloride, HCl., , 2., , Calculate the molar mass of argon atoms, given that the mass of single atom, is 6.634 × 10–26 kg., , 3., , Calculate the mass of 1.0 mol of potassium nitrate, KNO3 (atomic masses :, K = 39 amu; N = 14 amu, O = 16 amu)., , 4., , The formula of sodium phosphate is Na3PO4. What is the mass of 0.146, mol of Na3PO4? (atomic masses : Na = 23.0 amu, P = 31.0 amu; O = 16.0, amu)., , 1.10 MASS, MOLAR MASS AND NUMBER OF MOLES, Mass, molar mass and number of moles of a substance are inter-related quantities., We know that :, Molar mass (M) = Mass of one mole of the substance., Molar mass of water is 18 g mol–1. If we have 18 g of water, we have 1mol of it., Suppose we have 36 g water (18 × 2), we have 2 mol of it. In general in a sample, of water of mass (n × 18) g, the number of moles of water would be n. We may, generalize the relation as, mass of the substance, Number of moles (amount) of a substance = molar mass of the substance, m, M, m =n×M, , n =, , or, , These relations are useful in calculations involving moles of substances., , CHEMISTRY, , 21
Page 51 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Atoms, Molecules and Chemical Arithmetic, , Example 1.7 : In a reaction, 0.5 mol of aluminium is required. Calculate the, amount of aluminium required in grams? (atomic mass of Al = 27 amu), Solution :, , Molar mass of Al = 27 g mol–1, Required mass = no. of moles × molar mass, = (0.5 mol) × (27 g mol–1), , Notes, , = 13.5 g, , 1.11 MOLAR VOLUME, Vm, Molar volume is the volume of one mole of a substance. It depends upon, temperature and pressure. It is related to the density, by the relation., Molar volume =, , Molar mass, Density, , In case of gases, we use their volumes at standard temperature and pressure, (STP). For this purpose 0 0C or 273 K temperature is taken as the standard, temperature and 1bar pressure is taken as the standard pressure. At STP, the, molar volume of an ideal gas is 22.7 litre*. You will study that gases do not, behave ideally and therefore their molar volume is not exactly 22.7 L. However,, it is very close to 22.7 L and for all practical purposes we take the molar volume, of all gases at STP as 22.7 L mol–1., , INTEXT QUESTIONS 1.5, 1., , How many moles of Cu atoms are present in 3.05 g of copper (Relative, atomic mass of Cu = 63.5)., , 2., , A piece of gold has a mass of 12.6 g. How many moles of gold are present, in it? (Relative atomic mass of Au = 197), , 3., , In a combustion reaction of an organic compound, 2.5 mol of CO2 were, produced. What volume would it occupy at STP (273K, 1bar) ?, , 1.12 MOLCULAR AND EMPIRICAL FORMULAE, In your previous classes, you have studied how to write chemical formula of a, sustance. For example, water is represented by H2O, carbon dioxide is represented, * Earlier 1 atmosphere pressure was taken as the standard pressure and at STP (273K, 1atm) the molar, volume of an ideal gas was taken as 22.4 L mol–1. The difference in the value is due to the change in the, standard pressure (1bar) which is slightly less than 1atm., , 22, , CHEMISTRY
Page 52 :
MODULE - 1, , Atoms, Molecules and Chemical Arithmetic, , by CO2, methane is represented by CH4, dinitrogen penta oxide is represented by, N2O5, and so on. You are aware, formula for a molecule uses a symbol and subscript, number to indicate the number of each kind of atoms present in the molcule, (subscript 1 is always omitted). Such a formula is called molecular formula as it, represents a molecule of a substance. A molecule of water consists of two hydrogen, atoms and one oxygen atom. So its molecular formula is written as H2O. Thus a, molecular formula shows the actual number of atoms of different elements, in a molecule of a compound., , Some Basic Concepts of, Chemistry, , Notes, , There is another kind of formula, the empirical formul of a compound, which, gives only relative number of atoms of different elements. These numbers are, expressed as the simplest ratio. For example, empirical formula of glucose, which, consists of carbon, hydrogen and oxygen in the ratio of 1:2:1 is CH2O (empirical, formulae are also called simplest formulae). Molecular formula of a substance is, always an integral multiple of its empirical formula (i.e. molecular formula = Xn, where X is empirical formula and n is an integer). For example molecular formula, of glucose is C6H12O6 which is 6 × its empirical formula. Thus, while empirical, formula gives only a ratio of atoms, the molecular formula gives the actual number, of atoms of each element in an individual molecule. In some cases the ratio of, atoms shown in a molecular formula cannot be reduced to smaller integers. In, such cases molecular and empirical formulae are the same, for example, sucrose, C12H22O11 which is popularly known as cane-sugar. In case of certain elements, a, molecule consists of several atoms for example P4, S8, etc. In such cases, empirical, formula will be symbol of the element only., As you know, common salt, which is chemically called sodium chloride is, represented as NaCl. This salt is ionic in nature and does not exist in molecular, form. Therefore, NaCl is its empirical formula which shows that sodium and chlorine, atoms are present in NaCl in the ratio of 1:1. Similar is the case with all ionic substanes., KCl, NaNO3, MgO are examples of empirical formulae as these are all ionic, compounds. Table 1.6 provides a few more examples., Table 1.6: Molecular and Empirical Formulae, Substance, Ammonia, Carbon dioxide, Ethane, Fructose, Sulphur, Benzene, Sodium chloride, Calcium oxide, CHEMISTRY, , Molecular formula, , Empirical formula, , NH3, CO2, C2H6, C6H12O6, S8, C6H6, —, —, , NH3, CO2, CH3, CH2O, S, CH, NaCl, CaO, 23
Page 53 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Notes, , Atoms, Molecules and Chemical Arithmetic, , 1.13 CHEMICAL COMPOSITION AND FORMULAE, How much carbon is present in one kilogram of methane whose molecular formula, is CH4? How much nitrogen is present in one kilogram of ammonia, NH3? If we, have prepared a substance that is made of 58.8% carbon, 28.4% oxygen, 8.28%, nitrogen and 6.56% hydrogen, what is its empirical formula? You have studied, atomic masses, formulae, and the mole concept. Can we solve the problem, using these basic concepts? The answer is ‘yes’. Atomic masses, formulae and the, mole concept are the basic tools needed to solve such problems. What is percentage, composition? Let us take up this aspect in a little detail and try to understand., 1.13.1 Percentage Composition, If we know the formula of a compound, we can find out how much of each of the, elements is present in a given quantity of the compound. Aluminium is obtained, from its oxide. Al2O3 (which is found as the ore, bauxite). From the formula we, can calculate how much aluminium can be obtained, at least in prinicple, from a, given amount of aluminium oxide. Calculation is done by making use of the idea, of percentage composition, Percentage mass of an element in a compound, mass of element in one molecular formula or, in one empirical formula, × 100, =, molecular mass or empirical formula, mass of compound, , Mass of element in 1mol of compound, × 100, Molar mass of compound, Let us calculate percentage composition of aluminium oxide, Al2O3, =, , Pecentage of aluminium =, , Mass of aluminium in 1 mol Al 2 O3, × 100, Molar mass of Al2 O3, , Molar mass of Al2O3= (2 × 27.0) g + (3 × 16.0) g = 102.0 g, Since 1 mol of Al2O3 contains 2 mol of Al atoms, the mass of Al is 2 × 27.0 g =, 54.0 g Al, 54.0 g, Percentage of Aluminium = 102.0 g × 100 = 52.9 %, , We can calculate percentage of oxygen in the same way. One mole of Al2O3, contains 3 mole of O atoms, that is, 3 × 16.0 g oxygen therefore, 3 × 16.0 g, Percentage of oxygen = 102.0 g × 100 = 47.1%, , 24, , CHEMISTRY
Page 54 :
Atoms, Molecules and Chemical Arithmetic, , Example 1.8: Butanoic acid, has the formula C4H8O2. What is the elemental, analysis of butanoic acid?, , MODULE - 1, Some Basic Concepts of, Chemistry, , Solution : Molecular formula of the butanoic acid is C4H8O2., In one mole of butanoic acid there are 4 mol of carbon atoms, 8 mol of hydrogen, atoms and 2 mol of oxygen atoms. Thus, 1 molar mass of butanoic acid will be, equal to the sum of 4 × molar mass of carbon atoms, 8 × molar mass of hydrogen, atoms, and 2 × molar mass of oxygen atoms., , Notes, , Molar mass of butanoic acid = 4 × 12.0 g + 8 × 1.0 g + 2 × 16.0 g = 88.0 g, 48.0 g, Percentage of C by mass = 88.0 g × 100 = 54.5%, 8.0 g, Percentage of H by mass= 88.0 g × 100 = 9.1%, 32.0 g, Percentage of O by mass = 88.0 g × 100 = 36.4%, , The percentage of O in butanoic acid can also be calculated as follows :, Percentage of O by mass = 100 – (Percentage of C by mass +, Percentage of H by mass), = 100 – (54.5 + 9.1) = 36.4%, , 1.14 DETERMINATION OF EMPIRICAL FORMULAE –, FORMULA STOICHIOMETRY, We have just seen that if we know the formula of a compound we can calculate, the percentage composition. Now the question arises, can we determine the, formula of the compound if we know the percentage composition of a compound., The answer will be ‘yes’, but this formula will not be molecular formula; instead, it would be empirical formula as it would give simplest ratio of different atoms, present in a compound. Normally we determine the percentage composition of, different elements present in an unknown compound and determine its formula., Let us take a simple example of water. Water consists of 11.11% hydrogen and, 88.89% oxygen by mass. From the data, we can determine empirical formula of, water. Now if we assume that we have a 100.00 g sample of water, then the, percentage composition tells us that 100.0 g of water contains 11.11 g of hydrogen, atoms and 88.89 g of oxygen atoms., From the atomic mass table, we find that 1 mol of hydrogn atoms has a mass of, 1.0g, and 1 mol of oxygen atoms has a mass of 16.0 g. Now we can write unit, conversion factors so that the mass of hydrogen can be converted to moles of H, atoms and the mass of oxygen can be converted to moles of O atoms., CHEMISTRY, , 25
Page 55 :
MODULE - 1, , Atoms, Molecules and Chemical Arithmetic, , Some Basic Concepts of, Chemistry, , Moles of H =, , Mass of H, 11.11 g, =, Molar mass of H 1.0 g mol−1, , Similarly,, Mass of O, 88.89 g, =, = 5.55 mol, Molar mass of O 16.0 g mol−1, Thus in water, the ratio of moles of hydrogen atoms to moles of oxygen atoms is, 11.11 : 5.55., Moles of O =, , Notes, , Since a mole of one element contains the same number of atoms as a mole of, another element, the ratio of moles of atoms in a compound is also the ratio, of the number of atoms. Therefore, the ratio of hydrogen atoms to oxygen, atoms is 11.11:5.55. Now by dividing each by the smaller of the two numbers we, can convert both numbers to integers, 11.11, 5.55, = 2 and, =1, 5.55, 5.55, , Thus ratio hydrogen and oxygen atoms in water is 2 : 1 and empirical formula of, water is H2O., , INTEXT QUESTIONS 1.6, 1. For the compound Fe3O4, calculate percentage of Fe and O., 2. State percent composition for each of the following:, (a) C in SrCO3 (b) SO3 in H2SO4, 3. What are the empirical formulae of substances having the following, molecular formulae?, H2O2, C6H12, Li2CO3, C2H4O2, S8, H2O, B2H6, O3, S3O9, N2O3, 4. A compound is composed of atoms of only two elements, carbon and, oxygen. If the compound contain 53.1% carbon, what is its empirical, formula., , 1.15 CHEMICAL EQUATION AND REACTION, STOICHIOMETRY, You have studied that a reaction can be represented in the form of a chemical, equation. A balanced chemical equation carries a wealth of information, qualitative as well as quantitative. Let us consider the following equation and, learn what all information it carries., 4Fe(s), 26, , + 3O2(g) → 2Fe2O3(s), , ...(1.1), CHEMISTRY
Page 56 :
MODULE - 1, , Atoms, Molecules and Chemical Arithmetic, , Some Basic Concepts of, Chemistry, , 1. Qualitative Information, Qualitatively the equation (2.1) tells that iron reacts with oxygen to form iron, oxide., 2. Quantitative Information, Quantitatively a balanced chemical equation specifies numerical relationship, among the quantities of its reactants and products. These relationships can be, expressed in terms of :, , Notes, , (i) Microscopic quantities, namely, atoms, molecules and formula units., (ii) Macroscopic quantities, namely, moles, masses and volumes (in case of, gaseous substances) of reactants and products., Now let us again take the reaction (1.1) given earlier and get the quantitative, information out of it., 1.15.1 Microscopic Quantitative Information, The reaction (1.1), 4Fe(s) + 3O2(g) → 2Fe2O3(s), , ...(1.2), , tells that 4 atoms of iron react with 3 molecules of oxygen to form 2 formula, units of iron oxide. Often this information is written below each reactant and, product for ready reference as shown below:, 4Fe(s), 4 atoms of Fe, , +, , →, , 3O2(g), 3 molecules of O2, , 2Fe2O3(s), , ...(1.2a), , 2 formula units of Fe2O3, , 1.15.2 Macroscopic Quantitative Information, The microscopic quantitative information discussed in the previous section can, be converted into macroscopic information with the help of mole concept which, you have learnt in unit 1., (a) Mole Relationships, We know that Avogadro number of elementary entities like atoms, molecules,, ions or formula units of a substance constitute one mole of it. Let us multiply the, number of atoms, molecules and formula masses obtained in the previous section, (Eq.2.1a) by Avogadro’s constant, NA, 4 Fe(s), , +, , 3O2(g), , 4 atoms of Fe, , 3 molecules of O2, , 4 × NA atoms of Fe, 4 mol of Fe, , 3 × NA molecules of O2, 3 mol of O2, , CHEMISTRY, , →, , 2Fe2O3(s), , ..(1.3), , 2 formula units of Fe2O3, 2 × NA formula units of Fe2O3, 2 mol of Fe2O3, , 27
Page 57 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Atoms, Molecules and Chemical Arithmetic, , We may rewrite the above equation as, 4Fe(s), 4 mol of Fe, , +, , →, , 3O2(g), 3 mol of O2, , 2Fe2O3(s), , ...(1.3a), , 2 mol of Fe2O3, , The above equation (1.3a) gives us the mole relationship between reactants and, products. Here 4 mol of Fe react with 3 mol of O2 and produce 2 mol of Fe2O3., Notes, , (b) Mass Relationships, The mole relationships which you have learnt in the previous section, can be, converted into mass relationship by using the fact that mass of one mole of any, substance is equal to its molar mass which can be calculated from its formula, with the help of relative atomic masses of its constituent elements., In the reaction that we are discussing, the relative atomic masses of iron and, oxygen are 55.8 and 16.0 respectively. Therefore, (i) molar mass of Fe, , = 55.8 g mol–1, , (ii), , = 2 × 16.0 = 32 g mol–1, , molar mass of O2, , (iii) molar mass of Fe2 O3 = (2 × 55.8 + 3 × 16.0) g mol–1, = 159.6 g mol–1, Using these molar masses we can convert the mole relationship given by equation 2.1b into mass relationship as given below :, 4Fe(s), , +, , 3O2(g), , 4 mol Fe, , →, , 2Fe2O3(s), , 3 mol O2, , (4 × 55.8) g Fe, 223.2 g Fe, , 2 mol Fe2O3, , (3 × 32) g O2, 96 g O2, , (2 × 159.6) g Fe2O3, 319.2 g Fe2O3, , Thus 223.2 g iron would react with 96 g oxygen and produce 319.2 g iron oxide,, We may rewrite the above equation as, 4Fe(s), , +, , 223.2 g Fe, , 3O2(g) →, , 2Fe2O3(s), , 96 g O2, , 319.2 g Fe2O3, , (1.3b), , (c) Volume Relationships, We know that one mole of any gas occupies a volume of 22.7 L* at STP (standard temperature and pressure, 0oC and 1 bar pressure). We can use this information to arrive at volume relationships between gaseous substances. The reaction, that we are considering involves only one gaseous substance, O2. We may rewrite, the equation (2.1b) as, 4Fe(s), 4 mol, , +, , 3O2(g), 3 mol, , →, , 2Fe2O3(s), , (2.1b), , 2 mol., , (3 × 22.7) L at STP, 68.1 L at STP, * Earlier, the standard pressure was taken as 1 atmosphere and the volume of one mole of gas at STP, was taken as 22.4 L., , 28, , CHEMISTRY
Page 58 :
MODULE - 1, , Atoms, Molecules and Chemical Arithmetic, , Thus 4 mol of iron would react with 68.1 L of oxygen at STP to produce 2 mol, of iron oxide. (The volume relationship becomes more useful for reactions involving 2 or more gaseous substances)., , Some Basic Concepts of, Chemistry, , We can express microscopic as well macroscopic quantitative relationships involved in the above reaction as shown below:, 4Fe(s), , →, , + 3O2(s), , 2Fe2O3(s), , 4 atoms, , 3 molecules, , 2 formula units, , 4 mol, 223.2 g, –, , 3 mol, 96 g, 68.1 L at STP, , 2 mol, 319.2 g, –, , Notes, , We may use even mixed relations. For example, we may say 4 mol of iron would, react with 68.1 L (at STP) of oxygen to produce 319.2 g of iron oxide., Let us understand these relationships with two more examples., (a) Let us work out the mole, mass and volume relationships for the reaction, involved in manufacture of ammonia by Haber’s process., N2(g) +, , Microscopic relationship, Microscopic relationships, , 1 Molecule, , 3H2(g), 3 Molecules, , 2NH3(g) ...(2.2), 2 Molecules, , (i) Moles, , 1 mol, , 3 mol, , 2 mol, , (ii) Mass, , 28 g, , (3 × 2.0) = 6.0 g, , (2 × 17.0) = 34 g, , (iii) Volume, , 1 × 22.7 L, = 22.7 L, 1 vol, , (3 × 22.7), = 68.1 L, 3 vol, , (2 × 22.7), = 45.4 L, 2 vol, , or, , (b) Let us take one more reaction, the combustion reaction of butane and work, out the different types of relationships. The reaction is :, 2C4H10(g), 2 molecules, 2 mol, 2 × (4 × 12 +10 × 1) g, 116 g, 2 × 22.7 = 45.4 L, 2 vol, , +, , 13O2(g), , →, , 13 molecules, 13 mol, (13 × 32) g, 416 g, 13 × 22.7 = 295.1 L, 13 vol, , 8CO2(g), , +, , 8 molecules, 8 mol, 8 × (12 + 2 × 16) g, 352 g, 8 × 22.7 = 181.6 L, 8 vol, , 10H2O(g), 10 molecules, 10 mol, 10 × (2 ×1+16) g, 180 g, 10 × 22.7 = 227 L, 10 vol, , Now let us use the mole, mass and volume relationships to make some calculations., Example 1.9 : In the manufacture of ammonia by Haber process, nitrogen reacts, with hydrogen at high temprature and high pressure in the presence of a catalyst, and gives ammonia., N2(g) + 3H2(g), 2NH3(g), How much hydrogen would be needed to produce one metric ton of ammonia?, , CHEMISTRY, , 29
Page 59 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Atoms, Molecules and Chemical Arithmetic, , Solution : We should first find out the mass relationships for the reaction., N2(g), , +, , 3H2(g), , 1 mol, , 2NH3(g), , 3 mol, , 2 mol, , 1 × 28g = 28 g 3 × 2g = 6.0 g, , 2 × 17g = 34 g, , We know that :, 1 metric ton = 1000 kg = 103 kg = 106 g, , Notes, , From the mass relationship 34 g NH3 requires 6.0 g H2 for its manufacture., 6.0 × 106, g = 1.76 × 105g of H2., ∴ 10 g NH3 would require, 34, 6, , Thus 1 metric ton of ammonia will be obtained by using 1.176 × 105g of Hydrogen., Example 1.10 In a rocket motor fuelled by butane, C4H10, how many kg of O2, should be provided with each kg of butane to provide for complete combustion?, Solution :, , The combustion reaction of butane is, 2C4H10(g) + 13O2(g), 2 mol, 2 × 58 = 116 g, , 8CO2(g) + 10H2O(g), , 13 mol, 13 × 32 = 416 g, , Thus, to completely burn 116 g butane, oxygen required is 416g., Therefore, to completely burn 1 kg (1000 g) butane, oxygen required will be, =, , 416 × 1000, g O2, 116, , = 3586 g O2, = 3.586 kg O2 ≈ 3.59 kg O2, Example 1.11 When lead sulphide; PbS and lead oxide, PbO, are heated together, the products are lead metal and sulphur dioxide, SO2 ,, PbS(s) + 2PbO(s), , heat, , 3Pb (1) + SO2(g), , If 14.0 g of lead oxide reacts according to the above equation, how many (a), moles of lead (b) grams of lead, (c) atoms of lead and (d) grams of sulphur, dioxide are formed?, (Atomic mass : Pb = 207.0, S = 32.1 ; O =16.0), Solution : For each part of the question we will use the balanced equation, PbS(s) + 2PbO(s), 1mol, , 30, , 2mol, , heat, , 3Pb (1) + SO2(g), 3 mol, , 1mol, , CHEMISTRY
Page 60 :
Atoms, Molecules and Chemical Arithmetic, , Now formula mass of PbO = (207.0 + 16.0) = 223.0 amu, , MODULE - 1, Some Basic Concepts of, Chemistry, , Thus, one mole of lead oxide formula units have a mass of 223.0 g. Therefore,, 14.0 g PbO, , 14.0 g of PbO is 223.0 g mol –1 PbO = 6.28 × 10–2 mol PbO, (a), , The balanced equation shows that 2 mol of PbO form 3 mol of Pb. Therefore, 6.28 × 10-2 mol of PbO form, , Notes, , 3 mol Pb, 6.28 × 10-2 mol PbO × 2 mol PbO = 9.42 × 10-2 mol Pb, , (b), , The atomic mass of Pb is 207.0 ; this tells us that one mol of lead has a mass, 207.0 g. Thus, 9.42 × 10-2 mol of Pb has a mass of, 9.42 × 10-2 mol Pb ×, , (c), , 207.0 g Pb, = 19.5 g Pb, 1mol Pb, , 9.42 × 10-2 mol of Pb is, 9.42 × 10-2 mol of Pb × 6.022 × 1023 atoms mol–1 = 5.67 × 1022 Pb atoms, , (d), , The balanced equation shows that 2 mol of PbO form 1 mol of SO2., Therefore, 6.28 × 10-2 mol of PbO formula unit forms, 1mol SO 2, 6.28 × 10-2 mol PbO × 2 mol PbO, , = 3.14 × 10-2 mol SO2, Now the relative molecular mass of SO2 = 32.1 + 2(16.0) = 64.1, Molar mass of SO2 = 64.1 g mol–1, Therefore, 3.14 × 10-2 mol of SO2 molecules have a mass of 3.14 × 10–2 mol × 64.1, g mol–1 = 2.01 g, , INTEXT QUESTIONS 1.7, 1. How many grams of NH3 can be made according to the reaction, N2(g) + 3H2(g) ⎯⎯→ 2NH3 (g), from (a) 0.207 mol of N2 (b) 22.6 g of H2, , CHEMISTRY, , 31
Page 61 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Atoms, Molecules and Chemical Arithmetic, , 2. In reaction, C2H4(g) + 3O2(g) ⎯⎯→ 2CO2(g) + 2H2O( l ), How many (a) moles of O2 are consumed and (b) moles of H2O are formed, when 4.16 X 10-2 mol of C2H4 react?, , Notes, , 1.16 LIMITING REAGENT, We generally find that substances which react with each other are not present in, exactly the same proportionin a reaction mixture as stated by a balanced chemical equation. For example, if 2 mol each of hydrogen and oxygen are mixed and, a spark is passed through the mixture, water is formed, according to the equation, 2H2, , +, , 2 mol, , ⎯⎯→, , O2, 1mol, , 2H2O, 2 mol, , Here, 2 mol of hydrogen react with only 1 mol of oxygen, and 1 mol of oxygen, therefore remains unreacted. In this example hydrogen is said to be the limiting, reagent or reactant because its amount becomes zero and the reaction therefore, stops before the other reactant; that is, the oxygen is used up completly. The, amount of hydrogen present initially limits the amount of product that is formed., Example 1.12: 3 mol of sulphur dioxide SO2 is mixed with 2 mol of oxygen O2,, and after reaction is over sulphur trioxide, SO3 is obtained., (i) Which is the limiting reagent?, (ii) What is the maximum amount of SO3 that can be formed?, Solution :, , (i), , We must first write the balanced equation, 2SO2 + O2 → 2SO3, , According to the above equation, (a) 2 mol of SO3 can be formal from 2 mol of SO2., ∴ Amount of SO that can be formed from 3 mol of SO ., 3, , = (3 mol SO2) ×, , 2, , 2mol SO3, = 3 mol SO3, 2mol SO 2, , (b) 2 mol of SO3 can be formed from 1 mol of O2. Therefore, the amount of, SO3 that can be formed from 2 mol of O2., = (2 mol O2) ×, , 2mol SO3, = 4 mol SO3, 1mol O 2, , According to the definition, the limiting reactant is that reactant which gives the, smallest amount. In this case SO2 is the limiting reactant., 32, , CHEMISTRY
Page 62 :
MODULE - 1, , Atoms, Molecules and Chemical Arithmetic, , (ii), , The maximum amount of product that can be obtained is the amount, formed by the limiting reagent. Thus a the maximum amount of SO3 that, can be obtained is 3 mol., , Some Basic Concepts of, Chemistry, , Example 1.13: 2.3 g of sodium metal is introduced into a 2L flask filled with, chlorine gas at STP (273 K, 1bar). After the reaction is over, find :, (i), , What is the limiting reagent in this reaction?, , (ii), , How many moles of sodium chloride are formed?, , (iii), , Which substance is left unconsumed at the end of the reaction? Find out, its mass in grams., , (iv), , What percentage of the substance present in excess is converted into, sodium chloride?, , Notes, , (Given : Na = 23, Cl = 35.5), Solution :, 2 Na (s), , +, , 2mol, , or, , (i), , Cl2(g), , 2NaCl (s), , 1 mol, , 2 mol, , 22.7 L at STP, , Moles of sodium introduced =, , 2.3g, = 0.1 mol, 23g mol –1, , From the above equation, it is clear that 2 mol NaCl is formed from 2 mol Na, Therefore 0.1 mol Na can produce =, , 2 × 0.1, = 0.1 mol NaCl, 2, , Molar volume at STP = 22.7 L, Therefore moles of chlorine in 2 L volume at STP =, , 2L, 22.7 Lmol –1, , = 0.088 mol, , From equation : 1 mol Cl2 can produce 2 mol NaCl, Therefore 0.088 mol Cl2 can produce 2 × 0.088 = 0.176 mol NaCl., Since sodium produces less amount of NaCl, it is the limiting reagent., (ii) Sodium being the limiting reagent, as calculated in (i), the moles of NaCl, produced = 0.1 mol, (iii) From above equation, 2 mol NaCl is produced from 1 mol Cl2, Therefore 0.1 mol NaCl is produced from, , 1 × 0.1, = 0.05 mol Cl2, 2, , Initial moles of Cl2 = 0.088 mol, , CHEMISTRY, , 33
Page 63 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Atoms, Molecules and Chemical Arithmetic, , Moles of Cl2 left unconsumed = (0.088 – 0.05) mol = 0.038 mol, Therefore, mass of Cl2 left unconsumed = 0.038 g × 71.0 g mol–1 = 2.698 g, (because molar mass of Cl2 = 2 × 35.5 = 71.0 g mol–1), (iv) Moles of Cl2 consumed = 0.05 mol out of 0.088 mol, , Notes, , ∴ Percent of Cl2 consumed and converted into NaCl =, , 0.05, × 100 = 56.8 %, 0.088, , Example 1.14: 2.0 g mixture of MgCO3 and CaCO3 are heated till no further loss, of weight takes place. The residue weighs 1.04 g. Find the percentage composition of the mixture. (Mg = 24, Ca = 40, C = 12, O = 16), Solution :, , Mixture of MgCO3 and CaCO3 taken = 2.0 g, Let the mass of MgCO3 be = x g, Therefore the mass of CaCO3 = (2.0 – x) g, , The decomposition reactions are, MgCO3 (s), , → MgO(s) +, , (24+12+48) g, , (24+16) g, , 84 g, , CO2(g), , (i), , CaO(s) + CO2(g), , (ii), , 40 g (Residue), , CaCO3 (s), , →, , (40 + 12 + 48) g, 100 g, , (40 + 16) g, 56 g (Residue), , From the equation (i), 84 g MgCO3 leaves a residue = 40 g, x g MgCO3 will leave residue =, , 40 x, g, 84, , From the equation (ii), 100 g CaCO3 leaves a residue = 56 g, (2.0 – x) g CaCO3 will leave residue =, Total mass of the residue =, , 56 × (2.0 – x), g, 100, , 40 x, 56 × (2.0 – x ), +, = 1.04 g (given), 84, 100, , 40 × 100x + 84 × 56 × 2 – 84 × 56x = 84 × 100 × 1.04, 4000x + 9408 – 4704x = 8736, 9408 – 8736 = (4704 – 4000)x, 672 = 704x, 34, , CHEMISTRY
Page 64 :
Atoms, Molecules and Chemical Arithmetic, , 672, Therefore, mass of MgCO3 in the mixture = x =, = 0.96 g, 704, , Therefore, percentage of MgCO3 =, , MODULE - 1, Some Basic Concepts of, Chemistry, , 0.96, × 100 = 48 %, 2.0, , and percentage of CaCO3 = 100 – 48 = 52 %, , Notes, , WHAT YOU HAVE LEARNT, z, , Chemistry plays an important role in many aspects of our life like health and, medicine, energy and environment, materials and technology, food and, agriculture., , z, , Matter has particulate matter., , z, , According to the law of conservation of mass, in any chemical reaction, the, total mass of all the reactants is equal to the total mass of all the products., , z, , According to the law of definite proportion, in a chemical compound, the, proportions by mass of the elements that compose it are fixed and, independent of the origin of the compound or its mode of preparation., , z, , According to the law of multiple proportions when two elements form two, or more compounds, the masses of one element that combine with a fixed, mass of the other element are in the ratio of small whole numbers., , z, , John dalton gave the atomic theory in which he proposed that it is the, smallest indivisible particle of matter. Atoms of the same element are all, identicle while atoms of different elements differ. Atoms of different elements, combine in a simple whole number ratio to form a molecule., , z, , An atom is the smallest particle of an element that retains its chemical, properties., , z, , A molecule is the smallest particle of matter which can exist independently., , z, , An element is a substance that cannot be separated into simipler substnaces, by chemical means., , z, , Mole is the amount of a substance which contains as may elementary entities, as there are atoms present in 0.012 kg or 12 g of C-12. Thus mole denotes a, number., , z, , The number of elementary entities present in one mole of a substance is, 6.022 × 1023., , CHEMISTRY, , 35
Page 65 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Notes, , Atoms, Molecules and Chemical Arithmetic, z, , The mass of one mole of a substance is called its molar mass It is numerically, equal to relative atomic mass or relative molecular mass expressed in grams, per mole (g mol–1) or kilogram per mole (kg mol–1)., , z, , Molar volume is the volume occupied by one mole of a substance. One mole, of an ideal gas at standard pressure and temperature, STP (273 K and 1 bar), occupies 22.7 litres., , z, , In ionic substances, molar mass is numerically equal to the formula mass of, the compound expressed in grams., , z, , If the molar mass of a substance is known, then the amount of a substance, present in a sample having a definite mass can be calculated. If M is the molar, mass, then, the amount of substance n, present in a sample of mass m is, expressed as n =, , 36, , m, ., M, , z, , A chemical formula is used not only to represent the name of a compound, but also to indicate its composition in terms of (i) relative number of atoms, and (ii) relative number of moles of atoms., , z, , A molecular formula of a substance shows(i) the number of atoms of, different elements in one molecule.(ii) the number of moles of atoms of, different elements in one mole of molecule., , z, , An empirical formula shows only a ratio of (i) number of atoms, and (ii), moles of atoms in a compound., , z, , Molecular formula is always an integral multiple of the empirical formula., , z, , The empirical formula of a compound can be determined from its chemical, analysis., , z, , In order to determine a compound’s molecular formula, molecular mass also, must be known., , z, , Stoichiometry is the quantitative study of the composition of chemical compounds (compound or formula stoichiometry) and of the substances consumed and formed in chemical reactions (reaction or equation stoichiometry)., , z, , Chemical equations specify not only the identities of substances consumed, and formed in a reaction, but also the relative quantities of these substances, in terms of (a) atoms, molecules, and formula units and (b) moles of these, entities., , z, , A balanced chemical equation demonstrates that all the atoms present in the, reactants are accounted for in the product; atoms are neither created nor, destroyed in a reaction., CHEMISTRY
Page 66 :
Atoms, Molecules and Chemical Arithmetic, z, , The stoichiometric ratios among the moles of reactants shown in a balanced, equation are useful for determining which substance is entirely consumed, and which substance(s) is (are) left over., , MODULE - 1, Some Basic Concepts of, Chemistry, , TERMINAL EXERCISE, 1., , How many atoms are present in a piece of iron that has a mass of 65.0 g/, (atomic mass; Fe = 55.9 amu)., , 2., , A piece of phosphorus has a mass of 99.2 g. How many moles of phosphorus,, P4 are present in it? (atomic mass, P = 31.0 amu), , 3., , Mass of 8.46 × 1024 atoms of fluorine is 266.95 g. Calculate the atomic, mass of fluorine., , 4., , A sample of magnesium consists of 1.92 × 1022 Mg atoms. What is the mass, of the sample in grams? (atomic mass = 24.3 amu), , 5., , Calculate the molar mass in g mol–1 for each of the following:, , 6., , (i), , Sodium hydroxide, NaOH, , (ii), , Copper Sulphate CuSO4 .5H2O., , (iii), , Sodium Carbonate, Na2CO3 .10H2O, , Notes, , For 150 gram sample of phosphorus trichloride ( PCl3 ), calculate each of, the following:, (i), , Mass of one PCl3 molecule., , (ii) The number of moles of PCl3 and Cl in the sample., (iii)The number of grams of Cl atoms in the sample., (iv)The number of molecules of PCl3 in the sample., 7., , Find out the mass of carbon-12, that would contain 1 × 1019 atoms., , 8., , How many atoms are present in 100 g sample of C-12 atom?, , 9., , How many moles of CaCO3 would weigh 5 g?, , 10. If you require 1.0 × 1023 molecules of nitrogen for the reaction N2 + 3H2, → 2NH3., (i) What is the mass (in grams) of N2 required?, (ii) How many moles of NH3 would be formed in the above reaction from, 1.0 × 1023 molecules of N2?, (iii) What volume would NH3 gas formed in (ii) occupy at STP?, , CHEMISTRY, , 37
Page 67 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Atoms, Molecules and Chemical Arithmetic, , 11. Write empirical formulae of the following compounds:, CO, Na2SO3, C4H10, H2O2, KC1, 12. The empirical formula of glucose is CH2O which has a formula mass of 30, amu. If the molecular mass of glucose is 180 amu. Determine the molecular, formula of glucose, , Notes, , 13. What is ratio of masses of oxygen that are combined with 1.0 gram of nitrogen in the compound NO and N2O3?, 14. A compound containing sulphur and oxygen on analysis reveals that it contains 50.1% sulphur and 49.9% oxygen by mass. What is the simplest formula of the compound?, 15. Hydrocarbons are organic compound composed of hydrogen and carbon., A, 0.1647 g sample of a pure hydrocarbon on burning in a combustion tube, produced 0.5694 g of CO2 and 0.0845 g of H2O. Determine the percentage, of these elements in the hydrocarbon., 16. On combustion 2.4 g of a compound of carbon, hydrogen and oxygen gave, 3.52 g of CO2 and 1.44 g of H2O. The molecular mass of the compound was, found to be 60.0 amu., (a) What are the masses of carbon, hydrogen and oxygen in 2.4 g of the, compound?, (b) What are the empirical and molecular formulae of the compound?, 17. (i) What mass of oxygen is required to react completely with 24 g of CH4, in the following reaction?, CH4(g) + 2O2(g) → CO2(g) + 2H2O(1), (ii) How much mass of CH4 would react with 96 g of oxygen., 18. In the reaction H2 + C12 → 2HC1, How many grams of chlorine, C12 are needed to react completely with, 0.245 g of hydrogen, H2, to give hydrogen chloride, HC1? How much HC1, is formed?, 19. 3.65 g of H2 and 26.7 g of O2 are mixed and reacted. How many grams of, H2O are formed?, 20. Caustic soda NaOH can be commercially prepared by the reaction of Na2CO3, with slaked line, Ca(OH)2. How many grams of NaOH can be obtained by, treating 2.0 kg of Na2CO3 with Ca(OH)2?, 21. A portable hydrogen generator utilizes the reaction, CaH2 + H2O → Ca(OH)2 + 2H2, How many grams of H2 can be produced by a 100 g cartridge of CaH2?, , 38, , CHEMISTRY
Page 68 :
Atoms, Molecules and Chemical Arithmetic, , 22. The reaction 2Al + 3MnO → Al2O3 + 3Mn proceeds till the limiting, substance is consumed. A mixture of 220 g Al and 400 g MnO was heated to, initiate the reaction. Which initial substance remained in excess and by how, much ? ( Al = 27, Mn = 55)., , MODULE - 1, Some Basic Concepts of, Chemistry, , 23. KClO4 may be prepared by means of following series of reactions, Cl2 + 2KOH → KCl + KClO + H2O, 3KClO → 2KCl + KClO3, , Notes, , 4KClO3 → 3KClO4 + KCl, How much Cl2 is needed to prepare 400 g KClO4 by the above sequence?, (K = 39, Cl = 35.5, O = 16, H = 1), 24. 2.0 g of a mixture of Na2CO3 and NaHCO3 was heated when its weight, reduced to 1.876 g. Determine the percentage composition of the mixture., 25. Calculate the weight of 60 % sulphuric acid required to decompose 150 g of, chalk (calcium carbonate). Given Ca = 40, C = 12, O = 16, S = 32), , ANSWERS TO INTEXT QUESTIONS, 1.1, 1. Health, medicine, energy, food, agriculture etc., 2. Leucippus and his student Democritus, 3. In every chemical reaction total masses of all the reactants is equal to the, masses of all the products., 4. An atom is extremely small particles of matter that retains its identity during, chemical reaction., 5. Molecule is an aggregate of at least two atoms in a definite arragement held, togethrer its chemical forces., 6. It is derived from the Latin name of sodium i.e. Natrium, 7. An elements comprises of atoms of one type only while a compound, comprises atoms of two or more types combined in a simple but fixed ratio., , 1.2, 1., 2., 3., , Kilogram, μg, (i) h, (ii) n, , CHEMISTRY, , 39
Page 69 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Atoms, Molecules and Chemical Arithmetic, , 4., , (i) Megasecond, 106 s, (ii) millisecond, 10–3 s., , 1.3, 4.22 × 1023 molecules, , 1., , Moles of N2 gas = 6.022 × 1023 molecules mol –1 = 0.70 mol, , 2., , 8.46 × 1024 atoms, Amount of magnesium (moles) = 6.022 × 1023 atoms mol –1 = 14.05 mol, , 3., , No. of Cl2 molecules in 0.25 mol Cl2 = 0.25 × 6.022 × 1023 molecules, , Notes, , = 1.5055 × 1023 molecules, Since each Cl2 molecule has 2 Cl atoms, the number of Cl atoms = 2 × 1.5055, × 1023 = 3.011 × 1023 atoms., , 1.4, 1., , Molar mass of hydrogen chloride = molar mass of HCl, = 1 mol of H + 1 mol of Cl, = 1.0 g mol–1 + 35.5 g mol–1, = 36.5 g mol–1, , 2., , Molar mass of argon atoms, , = mass of 1 mol of argon, = mass of 6.022 × 1023 atoms of argon., = 6.634 × 10–26 kg × 6.022 × 1023 mol–1, = 39.95 × 10–3 kg mol–1, = 39.95 g mol–1, , 3., , Molar mass of KNO3 = mass of 1 mol of K + mass of 1 mol of N + mass of 3, mol of O., Since molar mass of an element is numerically equal to its atomic mass but, has the units of g mol–1 in place of amu = 39.1 g + 14.0 g + 3 × 16.0 g, , 4., , ∴ Molar mass of KNO3, , = 39.1 g + 14.0 g + 48.0 g = 101.1 g mol–1, , Mass of 1 mol of Na3PO4, , = 3 × (mass of 1mol of Na) + mass of 1 mol, of P + 4 × (mass of 1mol of oxygen), = 3 (23.0 g) + 31.0 g + 4(16.0) g, = 69.0 g + 31.0 g + 64.0 g = 164.0 g, , ∴ Mass of 0.146 mol of Na3PO4 = 0.146 × 164.0 g = 23.94 g, , 40, , CHEMISTRY
Page 70 :
MODULE - 1, , Atoms, Molecules and Chemical Arithmetic, , Some Basic Concepts of, Chemistry, , 1.5, 3.05 g, , 1., , Moles of Cu atoms in 3.05 g copper = 63.5 g mol –1 = 0.048 mol, , 2., , Moles of gold, Au = 197 g mol –1 = 0.064 mol, , 3., , Molar volume of any gas at STP (298 K, 1 bar) = 22.7 L, , 12.6 g, , Notes, , ∴ Volume occupied by 2.5 mol CO2 at STP = 2.5 × 22.7 L = 56.75 L, 1.6, 1., , Molar mass of Fe3O4 = 3 × 56.0 + 4 × 16.0, = (168.0 + 64.0) = 232.0 g mol–1, , 2., , Percentage of Fe, , =, , 168.0, × 100 = 72.41%, 232.0, , Percentage of O, , =, , 64.0, × 100 = 27.59%, 232.0, , (a) Molar mass of SrCO3 = 87.6 + 12.0 + 48.0 = 147.6 g mol–1, Percentage of carbon C in SrCO3 =, (b), , 12.0, × 100 = 8.13%, 147.6, , Molar mass of H2SO4 = 2.0 + 32.1 + 64.0 = 98.1 g mol–1, , Molar mass of SO3 = 32.1 + 48.0 = 80.1 g mol–1, Percentage of SO3 in H2SO4=, 3. Substance, H2O2, C6 H12, Li2CO3, C2H4O2, S8, H2O, B2 H 6, O3, S3O9, N2O3, CHEMISTRY, , 80.1× 100, = 81.65%, 98.1, , Empirical formula, HO, CH2, Li2CO3, CH2O, S, H2O, BH3, O3, SO3, N2O3, 41
Page 71 :
MODULE - 1, Some Basic Concepts of, Chemistry, , Atoms, Molecules and Chemical Arithmetic, , 4. Percentage of carbon, Percentage of Oxygen, , = 53.1%, = 46.9%, , Suppose we take 100 g of the substance then moles of carbon, =, , 53.1, g = 4.43 mol, 12.0, , Notes, mole of oxygen =, , molar ratio of C and O =, , 46.0, = 2.93 mol, 16.0, 4.43 2.93, :, 2.93 2.93, , = 1.50 : 1 or 3 : 2, Empirical formula of the compound is C3O2, 1.7, 1., , In equation, N2(g), , +, , 1 mol, , 3H2(g) →, , 2NH3(g), , 3 mol, , 2 mol, , 0.207 mol of N2 gives 0.414 mol of NH3, 0.414 mol of NH3 = 0.414 mol × 17.0 g mol–1 = 7.038 g of NH3, 22.6 g of hydrogen =, , 22.6, = 11.3 mol of hydrogen, 2.0, , 11.3 mol of hydrogen will give, , 2, 3, , × 11.3 mol of NH3 = 7.53 mol, , Therefore, mass of NH3 = 7.53 mol × 17.0 g mol–1 = 128.01 g, 2., , C2H4(g) + 3O2(g), 1 mol, , 3 mol, , →, , 2CO2(g), , +, , 2 mol, , 2H2O(g), 2 mol, , (a) 4.16 × 10-2 mol of C2H4 will consume 3 × 4.16 × 10-2 mol of oxygen, = 12.48 × 10-2 = 1.248 × 10-1 mol of O2, (b) moles of H2O formed = 2 × 4.16 × 10-2 mol, = 8.32 × 10-2 mol of H2O, , 42, , CHEMISTRY
Page 72 :
MODULE - II, ATOMIC STRUCTURE AND CHEMICAL BONDING, 2. Atomic Structure, 3. Periodic Table and Periodicity in Properties, 4. Chemical Bonding
Page 74 :
MODULE - 2, , Atomic Structure, , Atomic Structure and, Chemical Bonding, , 2, Notes, , ATOMIC STRUCTURE, , Chemistry has been defined as the study of matter in terms of its structure,, composition and the properties. As you are aware, matter is made up of atoms,, and therefore an understanding of the structure of atom is very important. You, have studied in your earlier classes that the earliest concept of atom ( smallest, indivisible part of matter ) was given by ancient Indian and Greek philosophers, (600-400 BC). At that time there were no experimental evidence. The origin of, the concept of atom was based on their thoughts on ‘What would happen if we, continuously keep dividing matter’. John Dalton revived the concept of atom in, the beginning of nineteenth century in terms of his atomic theory which, successfully explained the laws of chemical combination. Later experiments, showed that the atom is not indivisible but has an internal structure., In this lesson you will learn about the internal structure of an atom which will, help you to understand the correlations between its structure and properties. You, would learn about these in the later lessons., , OBJECTIVES, After reading this lesson you will be able to :, •, explain the atomic number, isotopes and isobars;, •, recognize the fundamental particles of atom;, •, describe Rutherford’s experiment and explain its results;, •, define electromagnetic radiation;, •, list and define the characteristic parameters of electromagnetic radiation;, •, discuss line spectrum of hydrogen;, •, explain Bohr’s postulates and discuss his model;, •, draw energy level diagram of hydrogen atom showing different series of, lines in its spectrum;, CHEMISTRY, , 45
Page 75 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Atomic Structure, , •, •, •, •, •, •, •, •, •, •, •, •, , to explain the stability of half filled and completely filled orbitals;, explain wave particle duality of matter and radiation;, formulate Heisenberg’s uncertainty principle;, explain the need for quantum mechanical model;, draw probability pictures of an electron in an atom;, list quantum numbers and discuss their significance;, draw the shapes of s, p and d orbitals;, recognize nodal plane;, explain Pauli’s exclusion principle;, define Aufbau principle;, explain Hund’s rule of maximum multiplicity; and, explain the stability of half filled and fully filled orbital., , 2.1 DISCOVERY OF FUNDAMENTAL PARTICLES OF, ATOM, In 1897 J.J. Thomson discovered electron as a constituent of atom. He determined, that an electron had a negative charge and had very little mass as compared to, that of the atom. Since an atom was found to be electrically neutral it was inferred, that some source of positive charge must be present in the atom. This soon led to, the experimental discovery of the proton, which is a positively charged subatomic, particle. Proton was found approximately 1840 times heavier than an electron., Further experiments revealed that the atomic masses were more than that expected, from the presence of just protons and electrons in the atom. For example, the, mass of helium atom was expected to be double that of hydrogen atom but was, actually found to be almost four times the mass of hydrogen atom. This suggested, the presence of neutral particles with mass comparable to that of protons in the, atom. Sir James Chadwick in 1932 discovered this neutral particle and called it, neutron subsequently. Thus we may conclude that atoms are not indivisible but, are made up of three fundamental particles whose characteristics are given in, Table 2.1., Table 2.1 Fundamental particles of atom and their characteristics, Particle, , Mass/ kg, , Actual Charge / C, , e, , 9.109 389 × 10–31, , – 1.602 177 × 10–19, , –1, , Proton, , p, , 1.672 623 × 10, , 1.602 177 × 10, , +1, , Neutron, , n, , 1.674 928 × 10–27, , 0, , 0, , Electron, , Symbol, , –27, , –19, , Relative charge, , Since atoms are made up of still smaller particles, they must have an internal, structure. In the next section we shall take up some of the earlier ideas about the, internal structure of atom., 46, , CHEMISTRY
Page 76 :
Atomic Structure, , MODULE - 2, Atomic Structure and, Chemical Bonding, , INTEXT QUESTION 2.1, 1. Compare the mass of an electron with that of the proton., 2. What is a fundamental particle?, 3. What is the name given to neutral particles in the atom?, Notes, , 2.2 ATOMIC NUMBER, MASS NUMBER, ISOTOPES AND, ISOBARS, All atoms can be identified by the number of protons and neutrons they contain., The atomic number (Z) is the number of protons in the nucleus of each atom., of an element. In, a neutral atom the number of protons is equal to the number, of electrons, so the atomic number also indicates the number of electrons present, in the atom. The chemical identity of an atom can be determined solely from, its atomic number. For example, the atomic number of nitrogen is 7. This means, that each neutral nitrogen atom has 7 protons and 7 electrons. Or, viewed, another way, every atom in the universe that contains 7 protons is correctly, named “nitrogen.”, The mass number (A) is the total number of neutrons and, protons present in, the nucleus of an atom of an element. Except for the most common form of, hydrogen, which has one proton and no neutrons, all atomic nuclei contains both, protons and neu-trons. In general the mass number is given by, mass number = number of protons + number of neutrons = atomic number, + number of neutrons, The number of neutrons in an atom is equal to the difference between the mass, mem-ber and the atomic number, or (A - Z). For example, the mass number, of fluorine is 19 and the atomic number is 9 (indicating 9 protons in the nucleus)., Thus the number of neutrons in an atom of fluorine is 19 - 9 = 10. Note that, the atomic number, num-ber of neutrons, and mass number all must be positive, integers (whole numbers)., Atoms of a given element do not all have the same mass. Most elements have, two or more isotopes, atoms that have the same atomic number but different, mass numbers. For example, there are three isotopes of hydrogen. One, simply, known as hydrogen, has one proton and no neutrons. The deturium isotope, contains one proton and one neutron, and tritium has one proton and two, neutrons. The accepted way to denote the atomic number and mass number of, an atom of an element (X) is as follows:, , CHEMISTRY, , 47
Page 77 :
MODULE - 2, , Atomic Structure, , Atomic Structure and, Chemical Bonding, , mass number, , A, ZX, atomic number, Thus, for the isotopes of hydrogen, we write, Notes, , 1H, 1, , 2, 1H, , 3, 1H, , hydrogen, , deuterium, , tritium, , As another example, consider two common isotopes of uranium with mass, numbers of 235 and 238, respectively:, 235U, 92, , 238U, 92, , The first isotope is used in nuclear reactors and atomic bombs, whereas the, second isotope lacks the properties necessary for these applications. With the, exception of hydrogen, which has different names for each of its isotopes,, isotopes of elements are identified by their mass numbers. Thus the above two, isotopes are called uranium-235 (pronounced “uranium two thirty-five”) and, uranium-238 (pronounced “uranium two thirty-eight”)., The chemical properties of an element are determined primarily by the protons, and electrons in its atoms; neutrons do not take part in chemical changes under, normal conditions. Therefore, isotopes of the same element have similar, chemistries, forming the same types of compounds and displaying similar, reactivities., The following example shows how to calculate the number of protons, neutrons,, and electrons using atomic numbers and mass numbers., Example 2.1: Give the number of protons, neutrons, and electrons in each of, the following species:, (a) 17 O, 8, , (b) 199 Hg, 80, , (c) 200 Hg, 80, , Solution:, (a) The atomic number is 8, so there are 8 protons. The mass number is 17,, SO the number of neutrons is 17 - 8 = 9. The number of electrons is the same, as the number of protons, that is, 8., (b) The atomic number is 80, so there are 80 protons. The mass number is 199,, so the number of neutrons is 199 - 80 = 119. The number of electrons is 80., , 48, , CHEMISTRY
Page 78 :
MODULE - 2, , Atomic Structure, , (c) Here the number of protons is the same as in (b), or 80. The number of, neutrons is 200 - 80 = 120. The number of electrons is also the same as in (b),, 80. The species in (b) and (c) are chemically similar isotopes of mercury., , Atomic Structure and, Chemical Bonding, , 2.3 EARLIER MODELS, Once it was established that the atom is not indivisible, the scientists made attempts, to understand the structure of the atom. A number of models have been proposed, for the internal structure of the atom. The first attempt to describe the structure, of atom in terms of a model was made by J.J Thomson., , Notes, , 2.3.1 Thomson’s Model, On the basis of his experiments on discharge tubes, Thomson proposed that atoms, could be considered as a large positively charged body with a number of small, negatively charged electrons scattered throughout it. This model (Fig. 2.1) was, called as plum pudding model of the atom., , Fig. 2.1 : A pictorial representation of Thomson’s, plum-pudding model, , The electrons represent the plums in the pudding made of positive charge. It is, sometimes also called as watermelon model. In this, the juicy pulp of the, watermelon represents the positive charge and the seeds represent the electrons., , J.J.Thomson, (1856-1940), Won Nobel prize in Physics in 1906, , Ernest Rutherford, (1871-1937), Won Nobel prize in Chemistry in 1908, , 2.3.2 Rutherford’s Experiment, Ernest Rutherford performed an experiment called ‘Gold Foil Experiment’ or ‘áray scattering experiment’ to test the structure of an atom as proposed by Thomson., In this experiment a beam of fast moving alpha particles (positively charged helium, ions) was passed through a very thin foil of gold. He expected that the alpha, CHEMISTRY, , 49
Page 79 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Atomic Structure, , particles would just pass straight through the gold foil and could be detected by, a photographic plate. But, the actual results of the experiment (Fig. 2.2) were, quite surprising. It was observed that most of the α-particles did pass straight, through the foil but a number of particles were deflected from their path. Some, of these deflected slightly while a few deflected through large angles and about, 1 in 10,000 á- particles suffered a rebound., , Notes, electron, nucleus, Source of alpha particles, , lead plate, , photographic plate gold foil, , Fig 2.2: Schematic representation of Rutherford’s, α-ray scattering experiment., , Fig 2.3 : Schematic representation, of Rutherford’s model, , These results led Rutherford to conclude that :, •, , the atom contained some dense and positively charged region located at the, center of the atom that he called as nucleus., , •, , all the positive charge of the atom and most of its mass was contained in the, nucleus., , •, , the rest of the atom would be empty space which contained the much, smaller and negatively charged electrons (Fig. 2.3)., , The model proposed by Rutherford explained the observation in the α-ray, scattering experiments as shown below in Fig 2.4., , undeflected, , a-rays, , Large angle, deflection, , Fig 2.4 : Explanation of the results of á- ray, scattering experiment., , Small angle, deflection, , Fig. 2.5 : Failure of Rutherford’s, model, , However, there was a problem with the Rutherford’s model. According to the, 50, , CHEMISTRY
Page 80 :
MODULE - 2, , Atomic Structure, , Maxwell’s theory of electromagnetic radiation, a charged particle undergoing, acceleration would continuously emit radiation and lose energy. Since the electron, in the atom is also a charged particle and is under acceleration, it is expected to, continuously lose energy. As a consequence, the electron moving around the, nucleus would approach the nucleus by a spiral path (Fig. 2.5) and the atom, would collapse. However, since it does not happen we can say that the Rutherford’s, model failed to explain the stability of the atom., The next attempt to suggest a model for atom was made by Neils Bohr, a student, of Rutherford. This model used the concept of quantisation of energy of electrons, in the atom. Since this fact was suggested by line spectrum of hydrogen atom it is, worthwhile to understand the meaning of a spectrum. For this we begin with the, understanding of the nature of an electromagnetic radiation., , Atomic Structure and, Chemical Bonding, , Notes, , INTEXT QUESTION 2.2, 1. List the three constituent particles of an atom., 2. What was the aim of Rutherford’s α-rays scattering experiment?, 3. Briefly describe Rutherford’s model of an atom., 4. On what basis was the Rutherford’s model rejected?, , 2.4 ELECTROMAGNETIC RADIATIONS, Electromagnetic radiation is a kind of energy, which is transmitted through space, in the form of electric and magnetic fields. These do not require any medium to, propagate. Visible light, radiant heat, radio waves, X-rays and gamma radiation, are some of the examples of electromagnetic radiations. According to the, Maxwell’s theory, an electromagnetic radiation can be visualised as oscillating electric, and magnetic fields. These travel as waves in the planes perpendicular to each other, and also to the direction of propagation (Fig. 2.6 (a) ). These radiations travel with, the velocity of light (3.0 × 108 m s–1)., ld, , fie, , ic, tr, , ec, , El, , Dire, , n, , gatio, , ropa, , of p, ction, , ld, , tic, , fie, , ne, , ag, , M, , (a), CHEMISTRY, , 51
Page 81 :
MODULE - 2, , Atomic Structure, , Atomic Structure and, Chemical Bonding, , Crest, , Crest, , l, , Amplitude, , l, , Notes, Trough, , Trough, , (b), Z, Electric field, component, l, , CREST, , CREST, , Direction of, propagation, , Y, Magnetic field, component, , X, , THROUGH, , l, , THROUGH, , Fig. 2.6 : (a) An electromagnetic wave showing electric and magnetic fields travelling in planes, perpendicular to each other and also to the direction of propagation (b) Characteristics of, , electromagnetic wave, , 2.4.1 Characteristic Parameters of Electromagnetic Radiations, The electromagnetic radiations are characterized by a number of parameters., These are, Amplitude: This refers to the maximum height to which the wave oscillates., It equals the height of the crests or depth of the troughs., Wavelength : It is the linear distance between two consecutive wave-crests or, wave- troughs as shown in Fig. 2.6(b). It is represented by a Greek letter, lambda (λ) and is expressed in terms of m, cm, nm or Angstrom (1Å = 10-10 m)., Frequency: It is defined as the number of wave crests or wave troughs that, pass through a given point per second. It is represented by a Greek letter nu, (ν) and is expressed in terms of s-1( second inverse or per second). It is also, called as Hz (Hertz)., Wave number: It equals the number of waves per unit length. It is denoted, as v (nu bar) and is equal to the reciprocal of the wavelength. The SI unit of v is, m–1 (meter inverse). However, sometimes it is also expressed as cm–1 (centimeter, inverse)., 52, , CHEMISTRY
Page 82 :
MODULE - 2, , Atomic Structure, , 1, v=, λ, , .... (2.1), , Atomic Structure and, Chemical Bonding, , Velocity: It is defined as the linear distance travelled by the wave in one second., The velocity in meters per second can be obtained by multiplying frequency in, Hertz ( s–1 ) with wavelength in meters., c, .... (2.2), λ, The velocity of a radiation depends on the medium. In vacuum the velocity is, equal to 3.00 x 108 m s-1., , c=νλ, , or, , v=, , Notes, , The electromagnetic radiations also show the characteristics of particles. These, are called as quanta. These quanta are actually bundles of energy. A quantum of, visible light is called a photon. The energy of the quantum ( or photon) is, proportional to the frequency of the radiation. The two are related as, ... (2.3), , E = hv, , The energy of the quantum can also be related to the wavelength or wave number, as, c, or E = hcv, ... (2.4), λ, the energy of photon can be readily calculated from these equations if we know, the frequency, wavelength or wave number., E=h, , Example 2.2 : A microwave radiation has a frequency of 12 gigahertz. Calculate, the energy of the photon corresponding to this radiation. ( h = 6.626 × 10–34 J s, and 1 gigahertz = 109 Hz.)., Solution:, , The energy is given by the expression, E = hv, Substituting the values we get,, 10, , E = 6.626 × 10–34 Js × 1.2 × 10 s–1 = 7.95 × 10–24 J, Example 2.3 : The green light has a wavelength of 535 nm. Calculate the energy, of a photon of green light., Solution: We know that, , E = hv =, , hc (6.626 × 10−34 Js) × (3.0 × 108 ms −1 ), =, = 3.71 × 10−19 J, −, 9, λ, 535 × 10 m, , 2.4.2 Electromagnetic Spectrum, Depending on their characteristics (wavelength , frequency and wave number), electromagnetic radiations are of many types and constitute what is called as an, electromagnetic spectrum (Fig. 2.7). The part of the spectrum that we can see is, called visible spectrum and is a very small part of the overall spectrum., CHEMISTRY, , 53
Page 83 :
MODULE - 2, , Atomic Structure, , Radio waves, , Visible, , Gamma rays, , Atomic Structure and, Chemical Bonding, , Notes, , Fig. 2.7: The electromagnetic spectrum, , INTEXT QUESTION 2.3, 1. What is an electromagnetic radiation?, 2. List any three characteristics of electromagnetic radiation., 3. What is wave number? How is it related to wave length?, 4. What is the difference between a ‘quantum’ and a ‘ photon’?, , 2.5 LINE SPECTRUM, You know that when we pass a beam of sunlight through a prism we get a range, of colours from violet to red (VIBGYOR) in the form of a spectrum (like rainbow)., This is called a continuous spectrum because the wavelengths of the light varies, continuously that is without any break. Let us take another example. You are, , Fig. 2.8: a) a Continuous spectrum, , 54, , b) a Line spectrum, , CHEMISTRY
Page 84 :
MODULE - 2, , Atomic Structure, , aware of the flame tests for identifying cations in the qualitative analysis., Compounds of sodium impart a bright yellow colour to the flame, copper gives a, green flame while strontium gives a crimson red coloured flame. If we pass such, a light through a prism it gets separated into a set of lines. This is called as a line, spectrum. Fig. 2.8 differentiates between a continuous and a line spectrum., , Atomic Structure and, Chemical Bonding, , 2.5.1 Line Spectrum of Hydrogen Atom, When an electric discharge is passed through a discharge tube containing, hydrogen gas at low pressure, it emits some light. When this light is passed, through a prism it splits up into a set of five lines. This spectrum is called the, line spectrum of hydrogen (Fig. 2.9)., , Fig. 2.9: A schematic diagram showing line spectrum, of hydrogen in the visible range, , Notes, , Johann Balmer, (1825-1898), , On careful analysis of the hydrogen spectrum it was found to consist of a few sets, of lines in the ultraviolet, visible and infrared regions. These sets of lines were, observed by different scientists. These spectral emission lines could be expressed, in the form of a general formula as:, v=, , 1, = RH, λ, , ⎛ 1, 1 ⎞, ⎜⎜ 2 − 2 ⎟⎟ cm-1 ; RH = 109677 cm-1, ⎝ n1 n2 ⎠, , …(2.5), , Where n1 and n2 are positive integers (n1 < n2) and RH is called Rydberg’s constant., The different sets of lines observed in the hydrogen atom spectrum named after, their discoverers and the values of n1 and n2 are given in the Table 2.2., Table 2.2 : Summary of the emission lines observed in hydrogen spectrum, Series, , n1, , n2, , Region of spectrum, , Lyman, , 1, , 2,3,4….., , Ultraviolet, , Balmer, , 2, , 3,4,5….., , Visible, , Paschen, , 3, , 4,5,6….., , Infrared, , Bracket, , 4, , 5,6,7….., , Infrared, , Pfund, , 5, , 6,7,8….., , Infrared, , CHEMISTRY, , 55
Page 85 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Atomic Structure, , The line spectrum of hydrogen atom was explained by Bohr’s model, which is, discussed in section 2.5., Example 2.4 : Calculate the wavelength of the Balmer line corresponding to, n2= 3., , Notes, , ⎛ 1, 1 ⎞, Solution: According to Balmer series v = RH ⎜⎜ 2 − 2 ⎟⎟, n2 ⎠, ⎝2, where RH = 109,677 cm-1, 1 ⎞, ⎛ 1, For n2 = 3 ; v = 109, 677 ⎜ 2 − 2 ⎟ = 109,677, 3 ⎠, ⎝2, , Since,, , ⎛ 5 ⎞, ⎜ ⎟, ⎝ 36 ⎠, , 1, 36, λ = v ; λ = 109, 677 × 5, , = 6.56 x 10-5 cm, = 656 nm, , 2.6 BOHR’S MODEL, In 1913, Niels Bohr (1885-1962) proposed another model of the atom where, electrons move around the nucleus in circular paths. Bohr’s atomic model is built, upon a set of postulates, which are as follows :, 1., , The electrons move in a definite circular paths around the nucleus ( Fig, 2.10). He called these circular paths as orbits and postulated that as long as, the electron is in a given orbit its energy does not change (or energy remains, fixed). These orbits were therefore referred to as stationary orbits or, stationary states or non radiating orbits., , Fig. 2.10: Bohr’s model, , 2., , The electron can change its orbit by absorbing or releasing energy. An, electron at a lower (initial) state of energy, Ei can go to a higher (final) state, of energy, Ef by absorbing a single photon of energy as given by, E = hv = Ef – Ei, , 56, , Bohr won the Nobel Prize in, Physics in 1922 for his work., , ...(2.6), , CHEMISTRY
Page 86 :
MODULE - 2, , Atomic Structure, , Similarly, when electron changes its orbit from a higher (initial) state of, energy Ei to a lower (final) state of energy Ef , a single photon of energy hí, is released (Fig. 2.11)., E1 E2, , h1, , E3, , Atomic Structure and, Chemical Bonding, , E 1 E 2 E3, , h1, , Notes, , Fig. 2.11 : Absorption and emission of photon causes the electron to change its energy level., , 3., , The angular momentum of an electron of mass me moving in a circular, orbit of radius r and velocity v is an integral multiple of h/2π., , nh, … (2.7), 2π, where n is a positive integer, known as the principal quantum number., me vr =, , Bohr obtained the following expressions for the energy of an electron in stationary, states of hydrogen atom by using his postulates :, ⎛ 1 ⎞, Energy of the orbit, En = − RH ⎜ 2 ⎟, ⎝n ⎠, , …(2.8), , mz 2e4, Bohr could correlate RH to other properties as RH =, where,, m = mass of the electron, z = nuclear charge, e = electronic charge, , 8h 2ε02, , ;, , …(2.9), , h = Planck’s constant, ε0 = permitivitty of the medium, , The negative sign in the energy expression means that there is an attractive, interaction between the nucleus and the electron. This means that certain amount, of energy (called ionisation energy) would be required to remove the electron, from the influence of the nucleus in the atom. You may note here that the energies, of the Bohr orbits are inversely proportional to the square of the quantum number, n. As n increases the value of the energy increases (becomes lesser negative or, more positive). It means that as we go farther from the nucleus the energy of the, orbit goes on increasing., 2.6.1 Explanation of Line Spectrum of Hydrogen Atom, As per the second postulate mentioned above, the energy emitted in the transition, of a single electron from an initial stationary state of energy Ei to a final stationary, state of energy Ef is given as hν = Ei − Ef . Substituting the expressions for energy, from eq. 2.8 we can get the formula given in eq.2.5. Thus Bohr’s model provides, an explanation for the observed line spectrum of hydrogen as summarized in, , CHEMISTRY, , 57
Page 87 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Atomic Structure, , Table 2.2. Fig. 2.12 shows the energy level diagram for hydrogen atom and the, transitions responsible for the observed line spectrum., , Notes, , Fig. 2.12: Energy level diagram for H-atom, showing various transitions responsible for the, observed line spectrum, , INTEXT QUESTION 2.4, 1. What is the difference between a line spectrum and a continuous spectrum?, 2. What are the main postulates of Bohr’s model?, 3. How does the energy of a Bohr orbit vary with the principle quantum number, ‘n’., , 2.7 WAVE – PARTICLE DUALITY, In section 2.3 you have learnt about the wave nature of light. As you are aware, that some of the properties of light e.g., diffraction and interference can be, explained on the basis of its wave nature. On the other hand some other properties, like photoelectric effect and scattering of light can be explained only on the basis, of particle nature of light. Thus light has a dual nature possessing the properties, of both a wave and a particle, i.e., light could under some conditions behave like, a particle and under other conditions behave as a wave., 58, , CHEMISTRY
Page 88 :
MODULE - 2, , Atomic Structure, , In 1923 a young French physicist, Louis de Broglie, argued that if light can show, wave as well as particle nature, why should particles of matter ( e.g., electron), not possess wave like characteristics? He proposed that matter particles should, indeed have a wave nature and said that a particle of mass m moving with a, velocity v has an associated wavelength, λ (some times called de Broglie, wavelength) given by the formula;, h, h, λ=, or, ...(2.10), p, mv, Where p ( = mv ) is the momentum of the particle. The de Broglie wavelength of, a body is inversely proportional to its momentum. Since the magnitude of h is, very small, the wavelength of the objects of our everyday world would be too, small to be observed. Let us make a calculation to see this., λ=, , Atomic Structure and, Chemical Bonding, , Notes, , Example 2.5 : Calculate the de Broglie wavelength associated with a cricket ball, weighing 380 g thrown at a speed of 140 km per hour., Solution: Mass of the cricket ball = 380 g = 380 × 10−3 kg = 0.38 kg, Speed or Velocity = 140 km/hr = (140 × 1000)/3600, = 38.89 m s−1, The wavelength associated with the cricket ball will be, , λ=, , h, 6.626 × 10−34 JS, =, mv (0.380kg ) (38.89m s −1 ), , = 4.48 × 10−35 m (J = kg m2 s–2), , de-Broglie (1892-1987), de-Broglie proposed the theory of, wave-particle dualism as a part of, his PhD thesis in 1924. He got the, physics Nobel prize in 1929, , If the electrons show wave nature then a beam of these electrons is expected to, show diffraction which is a property of waves. In 1927 G.P. Thomson and C.J., Davisson demonstrated the diffraction of electron waves by the crystal lattice of, nickel ( Fig. 2.13). Thus electrons also show a dual nature. That is, sometimes, these show particle nature while at some other times they show wave nature., , Fig. 2.13: Electron diffraction pattern from nickel crystal, , CHEMISTRY, , 59
Page 89 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Atomic Structure, , 2.8 HEISENBERG’S UNCERTAINTY PRINCIPLE, An important consequence of the wave-particle duality, of matter and radiation was discovered by Werner, Heisenberg in 1927 and is called the uncertainty, principle. According to this principle it is not possible, to simultaneously measure both the position and, momentum (or velocity) of an electron accurately. In, simple words we may state that more accurately you, measure a particle’s position, the less accurately you’re, Werner Heisenberg, able to measure its momentum, and vice versa. (1901-1976) Heisenberg, Mathematically, the Heisenberg principle can be expressed got the physics. Nobel, in terms of an inequality, prize in 1932, Äx Äp ≥, , h, 4π, , …(2.11), , Where Äx and Äp are the uncertainities in the measurements of position and, momentum respectively. If the position of an object is known exactly (i.e., Äx =, 0), then the uncertainty in the momentum must be infinite, meaning that we cannot, say anything about the velocity. Similarly, if the velocity is known exactly, then, the position would be entirely uncertain and the particle could be anywhere. It, means that we cannot say anything about the position of the particle. In actual, practice none of the two properties can be measured with certainty. Due to the, small value of the Planck’s constant , h ( 6.626 x 10-34 J s) this principle is not, relevant while making measurements of large objects like car, bus or aeroplane, etc.. It is relevant, only when you are making measurements on very small objects, such as electrons., Heisenberg’s principle questioned the validity of Bohr’s model. It is so because, according to Bohr’s model we can precisely calculate the radius of the orbit (i.e.,, the position of the electron) and the velocity of electron in it. But it is not possible, according to Heisenberg’s principle. It motivated many scientists to develop, newer models of the atom using the dual nature of the electron. This resulted into, the development of a Quantum mechanical model or Wave Mechanical Model of, the atom discussed in the next section., , INTEXT QUESTION 2.5, 1. What do you understand by wave-particle duality?, 2. Name the experiment that established the wave nature of electron., 3. Compute the de-Broglie wavelength associated with an electron moving, with a velocity of 100 km /second? (me= 9.1 x 10-31kg), 4. State Heisenberg’s Uncertainty Principle?, 60, , CHEMISTRY
Page 90 :
Atomic Structure, , 2.9 WAVE MECHANICAL MODEL OF ATOM, Wave Mechanical Model of atom was proposed by Erwin Schrödinger- an Austrian, physicist in 1926. This model is basically a formalism or a mathematical recipe,, which is based on some postulates that have no foundation in classical physics., The correctness of these postulates can be justified in terms of the correctness of, the results predicted by them. According to this model, the motion of electron, inside an atom could be described in terms of a mathematical function called,, wave function, ø (Greek letter, psi). The wave functions are assumed to contain, all the information about the electron and are obtained by solving a differential, equation called Schrödinger wave equation (SWE). The square of the wave, function ø 2 is a measure of the probability of finding an electron in a three, dimensional space around the nucleus., , MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , On solving the SWE for hydrogen atom we get a number of wave functions,, which are characterized by three quantum numbers viz.,, •, , Principal quantum number, n, , •, , Azimuthal quantum number, l, , •, , Magnetic quantum number, ml, , These quantum numbers arise in the process of logically solving the wave equation., Every electron in an atom has a unique (different) set of quantum numbers which, help to describe the three dimensional region where there is maximum probability, of finding the electron. This region is called as atomic orbital or simply orbital., 2.9.1 Significance of Quantum Numbers, The three quantum numbers describe the size, shape, and orientation of the atomic, orbitals in space. There is an additional quantum number which does not arise, from the Schrödinger wave equation but is introduced to account for electron, spin. The fourth quantum number thus help in designating the electrons present, in the atom. Let us understand the significance of each of these quantum numbers., Principal quantum number, n, The principal quantum number, n describes the energy level (or principal, shell ) of the electron within the atom. n can have only positive non zero, integral values (i.e., n = 1,2,3,4……). This means that in an atom, the electron, can have only certain energies. Thus we may say that n quantizes energy of the, electron. The principal quantum number also determines the mean distance of the, electron from the nucleus, i.e., its size. Greater the value of n, farther is the, electron from the nucleus., , CHEMISTRY, , 61
Page 91 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Atomic Structure, , Each principal shell can accommodate a maximum of 2n2 electrons, i.e.,, n =1, , number of electrons : 2, , n =2, , number of electrons : 8, , n =3, , number of electrons : 18 and so on…, , Azimuthal quantum number, l, Notes, , The azimuthal quantum number, l is related to the geometrical shape of the, orbital. The value of l may be zero or a positive integer less than or equal to, (n–1) ( n is the principal quantum number), i.e., l = 0,1,2,3…… (n–1). Different, values of l correspond to different types of subshells and each subshell contains, orbitals of a given shape., l = 0 , corresponds to s-subshell and contains the orbital with spherical shape, called as s orbital., l = 1, corresponds to p-subshell and contains the orbitals with a dumb-bell, shape called as p-orbitals. There are three p-orbitals in each p-subshell, l = 2 , corresponds to d-subshell and contains the orbitals with a cloverleaf, shape called as d-orbitals., l = 3 , corresponds to f-subshell and contain f-orbitals. There are seven f-orbitals, in each f-subshell., The shapes of s, p and d orbitals will be discussed in the next subsection (3, 8, 2)., Magnetic quantum number, ml, The quantum number, ml, describes the direction or orientation of the orbital, in space. The quantum number ml may have any integral value from – l to + l ., For example, for l = 1 ; ml can have the values as –1,0 and 1., Magnetic spin quantum number, ms, The quantum number, ms ,describes the spin of the electron i.e., whether it is, clockwise or anticlockwise. The quantum number, ms does not arise while solving, SWE. The clockwise and anticlockwise direction of electron spin has arbitrarily, been assigned the values as +1/2 and –1/2 respectively., To sum up, let us take an example of an electron belonging to the third shell, (n = 3). This electron can be in an s-subshell (l = 0) or a p-subshell (l = 1) or a, d-subshell (l = 2). If it happens to be in a p-subshell it may be in any of the three, possible p orbitals ( corresponding to ml = –1, 0 + 1 directed along x , y or z– axis., And within the orbital it may have clockwise (ms = + ½)or anti-clockwise, (ms = -½) direction of electron spin. The possible values of different quantum, numbers for an electron belonging to the third shell are given in Table 2.3., , 62, , CHEMISTRY
Page 92 :
MODULE - 2, , Atomic Structure, Table 2.3 : The quantum numbers for an electron belonging to the third shell, Principal, quantum, number, n, , Azimuthal, quantum, number, l, , Magnetic, quantum, number, ml, , Magnetic spin, quantum, number, ms, , 3, , 0, , 0, , +1/2, , Atomic Structure and, Chemical Bonding, , −1/2, 1, , −1, , +1/2, −1/2, , 0, , Notes, , +1/2, −1/2, , +1, , +1/2, −1/2, , 2, , −2, , +1/2, −1/2, , −1, , +1/2, −1/2, , 0, , +1/2, −1/2, , +1, , +1/2, −1/2, , +2, , +1/2, −1/2, , You may note here that the third shell can contain a maximum of 18 electrons and, each of them, has a distinct set of four quantum numbers., , INTEXT QUESTION 2.6, 1. What do you understand by a Wave Function?, 2. What is the difference between an orbit and an orbital?, 3. What are quantum numbers? List different quantum numbers obtained from, Schrödinger Wave Equation?, 4. Give the significance of the principal, azimuthal and magnetic quantum, numbers?, 2.9.2 Shapes of Orbitals, We have defined an orbital as “the three dimensional region of space around, the nucleus where there is maximum probability of finding the electron”. Let, us try to understand the meaning of an orbital by taking the example of 1s orbital, CHEMISTRY, , 63
Page 93 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Atomic Structure, , ( n =1 ; l =0 ). This can be understood in terms of a radial probability curve. Such, a curve gives the variation of the probability of finding the electron as a function, of distance from the nucleus. For 1s orbital the radial probability curve (Fig. 2.14, (a)) shows that the probability of finding the electron in 1s orbital increases as we, move away from the nucleus and reaches a maximum at a certain distance (=, 0.0529 nm or 52.9 pm for hydrogen atom) and then decreases as we go further, away from it and at a certain distance it becomes close to zero. The curve shows, the radial probability for a given direction. The probability would be same for all, possible directions. If we put all such curves together it would give a spherical, distribution of the electron probability. Since the radial probability does not become, zero at any distance, we cannot specify the size of the sphere. Therefore, the, orbital is represented as a boundary surface diagram, which may be thought as a, region of space, which contains 95 % of the probability of finding the electron, as, indicated in Fig.2.14(b). Thus the 1s orbital is represented as a sphere., Radial probability, , 12, 8, 4, 0, 0, , 0.4, , 0.8, , Distance from the nucleus, (in nm), , (a), , (b), , Fig. 2.14: (a) Radial probability curve for 1s orbital (b) Boundary surface diagram for 1s orbital, , Similarly, the Fig. 2.15 (a) gives the radial probability curve for a 2s orbital while, the Fig. 2.15 (b) shows the boundary surface diagram for the same. You can note, two things here. First you may note that for a 2s orbital the boundary surface, diagram is bigger as compared to a 1s orbital. Secondly, the radial probability, curve shows two maxima. The probability initially increases, reaches a maximum, then it decreases and comes close to zero. It increases again and decreases as we, Radial probability, , 4, 3, 2, 1, 0, 0, , 0.4, 0.8, Distance from the nucleus, (nm), , (a), , (b), , Fig.3.15: (a) Radial probability curve for 2s orbital (b) Boundary surface diagram for 2s orbital, , 64, , CHEMISTRY
Page 94 :
MODULE - 2, , Atomic Structure, , move further away from the nucleus. The region where the probability comes, close to zero (before increasing again) is called a spherical node. There are n–l–, 1 spherical nodes in an orbital., , Atomic Structure and, Chemical Bonding, , A node is a region in space where the probability of finding the electron is, close to zero., p- orbital : Now when we draw the shape of a p-orbital (n =1 ; l =1) we get a, shape as shown in the Fig. 2.16. This picture shows the shape of one of the three, possible p-orbitals which is directed towards the z-axis; pz. You may note that the, probability picture for a pz orbital consists of two lobes ; one along the positive zaxis and the other along the negative z-axis. Another important feature of a porbital is the absence of the electron probability in the XY- plane. Such a plane is, called a nodal plane. The shapes of the three p-orbitals are given in Fig.2.17., , Y X, , X, X, , Y, Z, , Fig. 2.16 : A p orbital, surface diagrams (Shapes ), , Y, , Z, , Z, , Px, , Py, , Notes, , X, Z, Pz, , Fig. 2.17: The boundary showing a nodal plane, of the p-orbitals, , The Fig.2.18 gives the shapes of five possible d-orbitals. The d-orbitals also contain, nodal planes. The five d- orbitals have different shapes but they all have same, energies i.e., these are degenerate., Z, , Y, X, X, , Y, , Y, , dx – y, Z, 2, , Z, , dxy, , dz2, 2, , Z, dyz, X, dxz, , y, , x, , Fig. 2.18: The boundary surface diagrams (shapes) of the five d-orbitals, , CHEMISTRY, , 65
Page 95 :
MODULE - 2, , Atomic Structure, , Atomic Structure and, Chemical Bonding, , INTEXT QUESTIONS 2.7, 1. What are the shapes of s,p and d orbitals?, 2. Describe the shape of a 2s orbital. How is it different from that of a 1s orbital?, 3. What do you understand by, Notes, , (i) a spherical node?, (ii) a nodal plane?, 4. How many spherical nodes will be there in 3s orbital ?, , 2.10 ELECTRONIC CONFIGURATION OF ELEMENTS, You have so far learnt that an atom consists of a positively charged nucleus, surrounded by electrons present in orbitals of different shapes and sizes. These, orbitals are part of different shells and sub-shells and are characterized by the, three quantum numbers viz. n,l and ml. Let us now take up the distribution of, electrons in these shells and sub-shells. Such a distribution of electrons is called, Electronic Configuration and is governed by three basic rules or principles., 2.10.1 Aufbau (or building up) Principle, This principle is concerned with the energy of the atom and states that the electrons, should occupy. The electrons occupy the orbitals in such a way that the energy of, atom is minimum. In other words the electrons in an atom are filled in the, increasing order of their energies. Now, how does one know the increasing, order of the orbital energies? You have learnt above that the principal quantum, number determines the energy of the orbitals. Higher the value of n higher the, energy. This is true only for hydrogen atom. For other atoms, we need to consider, both n and l. This means that different sub-shells in a given shell have different, energies. The order of orbital energies can be determined by the following, (n + l) rules., Rule 1: An orbital with a lower value for (n + l) has lower energy. For example,, the 4s orbital (n + l = 4+0=4) will be filled before a 3d orbital (n + l = 3 + 2 =5)., Rule 2: If the value of (n + l) is same for two orbitals then the orbital with lower, value of n will be filled first. For example, the 3d orbital (n + l = 3+2=5) will be, filled before a 4p orbital (n + l = 4 + 1 =5)., Following these rules the increasing order of the orbital energies comes out to be, 1s < 2s < 2p < 3s < 3p < 4s < 3d < 4p < 5s < 4d < 5p < 6s, , 66, , CHEMISTRY
Page 96 :
MODULE - 2, , Atomic Structure, , Atomic Structure and, Chemical Bonding, , 2.10.2 Pauli’s Exclusion Principle, This principle concerns the spin of electrons present in an orbital. According to, the Pauli’s principle, no two electrons can have all the four quantum numbers, to be same. For example, if a given electron in an atom has the set of four quantum, numbers as n =2, l=1, ml=1 and ms= + ½ then no other electron in the atom can, have the same set of quantum numbers., As you know that an orbital is characterized by three quantum numbers so the, electrons occupying a given orbital would have same values of these three quantum, numbers. These electrons are distinguished in terms of their spin quantum number,, ms. Since the spin quantum number can have only two values so only two electrons, can occupy a given orbital. In fact this fourth quantum number was introduced, through Pauli’s principle only., , Notes, , 3.10.3 Hund’s Rule, This rule concerns the distribution of electrons in a set of orbitals of the same, energy, i.e. constituents of a subshell. According to this rule if a number of orbitals, of the same sub-shell are available then the electrons distribute in such a way that, each orbital is first singly occupied with same spin. For example, the six electrons, in carbon distribute as, 1s2 2s2 2p1x 2p1y2p0z, , and not as 1s2 .2s2 2p2x 2p0y2p0z, , Since electrons repel each other, they remain as far as possible from one another, by occupying different orbitals., The rules discussed above can be used to write the electronic configuration of, different elements. There are two common ways of representing the electronic, configurations. These are, a) Orbital notation method: In this method the filled orbitals are written in the, order of increasing energies . The respective electrons in them are indicated as, superscripts as shown in the example given below. For example, the electronic, configuration of nitrogen atom ( atomic number 7) is written as 1s22s22p1x 2p1y2p1z., b) Orbital diagram method: In this method the filled orbitals are represented, by circles or boxes and are written in the order of increasing energies. The, respective electrons are indicated as arrows whose direction represents their spin., For example, the electronic configuration of nitrogen in the orbital diagram, notation can be written as, 1s, , 2s, , 2p, , Electronic configurations can also be written in a short hand form . In this method, the last completed orbital shell is represented in terms of a noble gas. For, example, the electronic configuration of lithium and sodium can be written as, CHEMISTRY, , 67
Page 97 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Atomic Structure, , Li, , [He]2s1, , Na, , [Ne]3s1, , The electrons in the noble gas configuration are termed as core electrons while, the ones in the outer shell are called valence electrons., Notes, , 2.11 STABILITY OF COMPLETELY FILLED AND HALF, FILLED SUBSHELLS, Aufbau principle helps to determine the electronic configuration of an atom in, a periodic table but in certain cases it fails to predict the correct electronic, configuration where the energies of neighbouring subshells are quite close e.g.,, 4s, 3d; 5s, 4d; 4f, 5d etc. There is shifting of energy due to electron-electron, interactions e.g., in chromium, the Aufbau predicted configuration is 3d4 4s2, but the actual configuration is3d5 4s1. Similarly in copper, the Aufbau predicted, configuration is 3d9 4s2 but the actual configuration is 3d10 4s1. This is due to, extra stability of half filled or completed filled orbitals., 1. Symmetry of Orbitals, We know that symmetry leads to stability. Sometimes if we add or remove the, electron to make symmetrical distribution of the electrons in the orbital thus, the electronic configuration become more stable e.g., the Au'fbau predicted, electronic configuration of Chromium is [Ar] 4s23d4 but its actual configuration, is [Ar] 4s1 3d5., Predicted (a), Actual (b), Similarly in case of Copper., Predicted, Actual, , The half filled or completely filled 3d orbital is more symmetrical and more, stable., 2. Exchange Energy, The stability of half filled and completely filled orbtails is expained by exchange, energy i.e., (shifting of or exchange of electrons from one orbital to another, of the same subshell). Larger the number of electrons witht the same spin larger, is the exchange energy. Larger the exchange energy. Larger the exchange energy, stabler is the electronic configuration. Consider the outer electron configuration, 68, , CHEMISTRY
Page 98 :
MODULE - 2, , Atomic Structure, , of Cr which is according to Aufbau is 3d44s2 but in reality it is 3d5 4s1. In 3d4, 4s2 there are 4 electrons with same spin whereas in 3d5 4s1 there are 6 electrons, with same spin therefore exchange energy is larger in the latter case the preferred, configuration of Cr is 3d5 4s1. Consider the electronic configuration of, chromium Cr – 3d44s2, There are no. of possible ways by which electrons can exchange their position, in 3d subshell., , 3 possibility, of exchange, , 2 possibility, of exchange, , Atomic Structure and, Chemical Bonding, , Notes, , possibility, of exchange, , In 3d4 arrangements, electron can exchange in 3 + 2 + 1, λ′ = 6 ways, ie.e there, are six possibility of exchange electrons with parallel spins., In 3d5 electronic configuration the electron can exchange in 4 + 3 + 2 + 1 =, 10 ways or there are ten possibility of exchange electrons., , 4, , 3, , 2, , 1, , Thus there is increase in exhange energy from 3d4 4s2 to 3d5 4s1 configuration., Greater the exchange energy, greater is the stability of this configuration., , INTEXT QUESTION 2.8, 1., 2., 3., 4., , What do you understand by the electronic configuration of an atom?, What is Pauli’s exclusion principle?, What is Aufbau principle? What are ( n + l ) rules?, Which of the following orbitals will be filled first?, i) 2p or 3s, ii) 3d or 4s, 5. The electronic configuration of Cr is (Ar)3d54s1 not 3d44s2., , WHAT YOU HAVE LEARNT, z, , Atoms are made up of three fundamental particles namely, electrons, protons, and neutrons., , CHEMISTRY, , 69
Page 99 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Atomic Structure, z, , J.J Thomson made the first attempt to describe the structure of an atom in, terms of a model called plum pudding model. According to this atoms can, be considered as a large positively charged body (pudding) in which a number, of small negatively charged electrons ( plums) are scattered.., , z, , According to the Rutherford’s model, the positive charge of the atom and, most of its mass is contained in the nucleus and the rest of the atom is empty, space which contains the negatively charged electrons., , z, , Electromagnetic radiation is a kind of energy, which is transmitted through, space in the form of electric and magnetic fields. It travels with the speed of, light and does not need any medium to travel., , z, , The electromagnetic radiations are characterized by a number of parameters, like, amplitude, wavelength, frequency, wave number and velocity., , z, , Hydrogen gas gives a line spectrum consisting of distinct lines suggesting the, quantization of energy in hydrogen atom., , z, , In 1913, Niels Bohr proposed ‘Planetary Model’ for atom. According to the, model the electrons move in definite circular paths of fixed energy around a, central stationary nucleus. The electrons can change their orbits by absorbing, or emitting a photon of energy (= hí) equal to the difference of the energies, of the orbits., , z, , Bohr’s model did explain for the stability of atom and the line spectrum of, hydrogen. The model however was unable to explain the spectra of atoms, other than hydrogen., , z, , Louis de Broglie, argued for the dual nature of electron and proposed that, matter particles should have a wave nature. The associated wavelength is, , Notes, , h, h, or λ =, mv, p, This was experimentally verified by Thomson and Davisson by diffraction of, electron waves passing through the crystal lattice of nickel., given by the formula; λ =, , •, , 70, , z, , The wave-particle duality of matter led Werner Heisenberg to propose the, uncertainty principle. According to which it is not possible to measure, simultaneously both the position and momentum of a particle with a infinite, precision., , z, , The dual nature of electron and Heisenberg’s uncertainty principle led to the, development of wave mechanical model., , z, , According to the wave mechanical model, the motion of electron inside the, atom can be described in terms of a mathematical function called, wave, function, ø. This wave function contains all the information about the system, and can be found by solving a wave equation called Schrodinger wave equation., , CHEMISTRY
Page 100 :
Atomic Structure, z, , The square of the wave function, ø 2 is a measure of the probability of finding, the electron in a certain three dimensional space around the nucleus. This, region is called as atomic orbital or simply orbital., , z, , These wave functions are characterized by three quantum numbers. These, quantum numbers describe the size, shape, and orientation of the atomic, orbitals in space. Every electron in an atom has a unique set of quantum, numbers., , z, , The principal quantum number n concerns the quantisation of the energy of, the electron while the azimuthal quantum number , l is related to the shape, of the orbital. The magnetic quantum number ml describes the direction or, orientation of the orbital in space., , z, , An additional quantum number, ms is introduced to account for electron, spin. This quantum number does not follow from the wave mechanical model, and describes the spin of the electron., , z, , Different orbitals have different shapes. An s orbital is spherical; p-orbitals, are dumb-bell shaped ; d-orbitals have cloverleaf shape while f-orbitals, have a eight lobed shape., , z, , Electronic Configuration. Why are half filled and fully filled orbital more, stable, , z, , The distribution of electrons in the shells and subshells is called Electronic, Configuration. It is governed by three rules which are Aufbau principle ;, Pauli’s exclusion principle and Hund’s Rule of maximum multiplicity., , z, , According to Aufbau principle the electrons in an atom are filled in the, increasing order of their energies which is determined by (n + l) rules., , z, , According to the Pauli’s exclusion principle, no two electrons can have all, the four quantum numbers to be same., , z, , While filling electrons in the orbitals of same subshell, according to Hund’s, rule, each orbital is first singly occupied with same spin then the pairing up, takes place., , z, , Half filled and fully filled orbital are more stable., , MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , TERMINAL EXERCISE, 1., , (a) What are the three fundamental particles that constitute an atom?, (b) Compare the charge and mass of an electron and of a proton., , 2. What do you think is the most significant contribution of Rutherford to the, development of atomic structure?, CHEMISTRY, , 71
Page 101 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Atomic Structure, , 3. What experimental evidence shows the dual nature of light?, (a) Compute the energy of a FM radio signal transmitted at a frequency, of 100 MHz., (b) What is the energy of a wave of red light with l = 670 nm?, 4. In what way was the Bohr’s model better than the Rutherford’s model?, 5. What are the drawbacks of Bohr’s Model?, 6. What led to the development of Wave Mechanical Model of the atom?, 7. What do you understand by an orbital? Draw the shapes of s and p orbitals., 8. Explain the Hund’s rule of maximum multiplicity with the help of an example., , ANSWERS TO INTEXT QUESTIONS, 2.1, 1, , Proton is heavier than electron. The ratio of their masses is, = mp/me =, , 1.672 623 × 10−27 kg, 9.109 389 × 10−31 kg, , = 1836, 2 Main constituent particles like proton, neutron and electron etc. present in, the atom come in the category of fundamental particles., 3. Neutron, 2.2, 1 Electron, proton and neutron, 2 The aim of Rutherford’s experiment was to test the Thomson’s plum-pudding, model., 3 According to Rutherford’s model for atom, the positive charge of the atom, and most of its mass is contained in the nucleus. The rest of the atom is, empty space which contains the much smaller and negatively charged, electrons., 4 Rutherford’s model was rejected because it could not explain the stability of, the atom., 2.3, 1. Electromagnetic radiation is a kind of energy which is transmitted through, space in the form of electric and magnetic fields. It travels with the speed of, light and does not need any medium to travel., , 72, , CHEMISTRY
Page 102 :
Atomic Structure, , 2. The different characteristics of electromagnetic radiation are, , MODULE - 2, Atomic Structure and, Chemical Bonding, , i) Amplitude,, ii) Wavelength,, iii) Frequency,, iv) Wave number and, v) Velocity, , Notes, , 3. The wave number is defined as the number of waves per centimeter. It is, equal to the reciprocal of the wavelength., 4. A quantum of visible light is called photon. The energy of the quantum (or, photon) is proportional to the frequency of radiation., 2.4, 1. A line spectrum consists of a series of discrete lines of characteristic, wavelengths while a continuous spectrum contains a broad band of radiations, containing all possible wavelengths in the range i.e., the wavelengths of the, radiation varies continuously., 2. The main postulates of Bohr’s model are, i) The electrons move in a definite circular paths called as stationary, orbits or stationary states around a central stationary nucleus., ii) The electrons can change their orbits by absorbing or emitting a photon, of energy (= hí) equal to the difference of the energies of the orbits., iii) The angular momentum of the electron is quantised., 3. The energy of a Bohr’s orbit increases with an increase in the value of the, principal quantum number, n. In fact it becomes lesser and lesser negative., 2.5, 1. The wave-particle duality refers to the fact that light and the material particles, like electrons could sometimes behave as a particle and as a wave at other, times., 2. The wave nature of electron was established by the diffraction of electron, waves by the crystal lattice of nickel., 3., , Mass of the electron = 9.1 × 10−31 kg, Speed or Velocity = 100 km s–1 = 105 m s–1, , CHEMISTRY, , 73
Page 103 :
MODULE - 2, , Atomic Structure, , Atomic Structure and, Chemical Bonding, , Using equation , the wavelength associated with the electron will be, , λ =, , Notes, , h, 6.626 × 10-34 J s, =, = 7.28 ×10−9 m, –31, 5, –1, mv, (9.1 × 10 kg) ( 10 m s ), , 4. According to Heisenberg’s Uncertainty Principle it is not possible to measure, both the position and momentum of a particle with any degree of certainity., More accurately we measure a particle’s position, the less accurately we are, able to measure it’s momentum, and vice versa., 2.6, 1. It is a mathematical function that describes the motion of an electron inside, the atom. It contains all the information about the system and can be found by, solving a wave equation called Schrödinger wave equation., 2. An orbit refers to definite circular paths of fixed energy around a central, stationary nucleus while an orbital refers to the three dimensional region of, space around the nucleus where there is a probability of finding the electron., 3. The quantum numbers are integers that characterize the wavefunctions. These, are obtained in the process of solving Schrödinger wave equation and every, electron in an atom has a different set of quantum numbers. The three quantum, numbers obtained from Schrödinger Wave Equation are, (i) The principal quantum number, n, (ii) Azimuthal quantum number, l and, (iii) The magnetic quantum number ml, 4. The principal quantum number, n is concerned with the energy of the electron, in a shell. The quantum number l is related to the geometrical shape of the, orbital and the quantum, number, ml describes the orientation of the orbital, in space., 2.7, 1. s orbital, , : spherical;, , p orbitals : dumb-bell shaped ;, d orbitals : cloverleaf shaped., 2. The 2s orbital is spherical in shape similar to the 1s orbital . However there, are two differences. Firstly, the size of a 2s orbital is bigger as compared to a, 1s orbital and secondly, it contains a spherical node., 3., , 74, , (i) It is a spherical region of zero probability in an s orbital (other than, 1s)., CHEMISTRY
Page 104 :
Atomic Structure, , (ii) It is a planar region in an orbital (other than s orbitals )where the, probability of finding the electron is zero., , MODULE - 2, Atomic Structure and, Chemical Bonding, , 4. The 3s orbital will have two spherical nodes., 4. Because half filled d-orbital is more stable as compared to partially filled, orbital., Notes, , 2.8, 1. The distribution of electrons in the shells and subshells of an atom is called, Electronic Configuration., 2. Pauli’s principle states that in an atom no two electrons can have same set of, the four quantum numbers., 3. Aufbau principle states that the electrons in an atom are filled in the increasing, order of their energies which is determined by (n + l) rules., There are two ( n+l ) rules. These are, An orbital with a lower value for (n + l) is filled first., If the value of (n + l) is same for two orbitals then the orbital with lower, value of n will be filled first, 4. (i) 2p : (n + l) for 2p = 2 + 1 = 3 ; for 3s (n + l) = 3 + 0 = 3 ; Rule 2, (ii) 4s : (n + l) for 4s = 4 + 0 = 4 ; for 3d (n + l) = 3 + 2 = 5 ; Rule 1, , CHEMISTRY, , 75
Page 105 :
MODULE - 2, , Periodic Table and Periodicity in Properties, , Atomic Structure and, Chemical Bonding, , 3, Notes, , PERIODIC TABLE AND, PERIODICITY IN PROPERTIES, , We have seen different heaps of onions and potatoes at vegetable shop. Imagine,, they are lying mixed and you want to buy 1 kg of onion. What will happen? You, will have to wait for long to sort that and then weigh them. When you possess a, variety of material substances, you have to keep them classified for an easy access, and quick use. You cannot afford to mix clothes with eatables, cosmetics or books., Classification assures you that your eatbles are in the kitchen, books on the study, table or rack and your cosmetics are on the dressing table. Shopkeepers, business, houses, storekeepers, administrators, managers, information technology experts, and scientists etc. have to keep their materials duly classified., Chemists faced a similar problem when they were to handle a large number of, elements. The study of their physical and chemical properties and keeping a, systematic record of them had been a great challenge to chemists. Classification of, elements finally could be possible due to pioneering work of a few chemists. In the, present lesson we shall discuss the need, genesis of classification and periodic trends, in physical and chemical properties of elements., , OBJECTIVES, After reading this lesson, you will be able to:, , 76, , z, , recongise the need for classification of elements;, , z, , recall the earlier attempts on classification of elements;, , z, , define modern periodic law;, , z, , name the elements with atomic number greater than 100 according to IUPAC, nomenclature;, , CHEMISTRY
Page 106 :
MODULE - 2, , Periodic Table and Periodicity in Properties, z, , co-relate the sequence of arrangements of elements in periodic table with, electronic configuration of the elements;, , z, , recall the designations of the groups (1-18) in the periodic table;, , z, , locate the classifiction of elements into s-, p-, d- and f- blocks of the periodic, table; and, , z, , explain the basis of periodic variations of, , Atomic Structure and, Chemical Bonding, , Notes, , (a) atomic size, (b) ionic size, (c) ionization enthalpy, (d) electron gain enthalpy within a group or a period., (e) valence, , 3.1 EARLY ATTEMPTS, Attempts were made to classify elements ever since the discovery of metals or, may be even earlier. J.W. Dobereiner in 1817 discovered that when closely related, elements are grouped in a set of three, the atomic weight of the middle element, was almost the arithmetical mean of the other two elements in that group e.g.,, Element, Atomic weight, Mean atomic weight, , Lithium, 6.94, ------, , Sodium, 22.99, 23.02, , Potassium, 39.10, -------, , He called such a group of three elements a triad. He could group only a few, elements due to lack of knowledge of correct atomic weights of the elements at, that time., In 1863, J.A.R. Newlands, developed a system of classification of elements and, entitled it as Law of Octaves. He arranged the elements is such a way that every, eighth element had similar properties, like the notes of music. The law could not, apply to a large number of known elements. However, the law indicated very, clearly the recurrence of similar properties among the arranged elements. Thus, the periodicity was visualised for the first time in a meaningful way., Periodicity: Re-occurrence of properties after regular intervals., More significant results were obtained when Lother Meyer’s work reflecting the, periodicity was found to be based on physical properties of the elements. He, clearly showed that certain propertics showed a periodic trend., , 3.2 MENDELEEV’S PERIODIC TABLE, In 1869, Mendeleav, a Russian Chemist made a thorough study of the relation, between the atomic weights of the elements and their physical and chemical, CHEMISTRY, , 77
Page 107 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Periodic Table and Periodicity in Properties, , properties. He then constructed a table in which elements were arranged in order, of their increasing atomic weights. It was also found that every eighth element, had properties similar to that of the first element. Thus, there was a periodic, occurrence of elements with similer properties., One of the most striking applications of Mendeleev’s classification of elements, was that in his periodic table (Table 3.1) he left gaps for elements which were, yet to be discovered. He also predicted the properties of these elements., However, Mendeleev’s periodic table did not provide any place for isotopes, and noble gases which were discovered later on., Table 3.1 Mendeleev’s Table of 1871, Group, , I, , Oxide, Hydride, , II, , III, , R2O, RH, , IV, , RO, RH2, B A, , R2O3, RH3, B, , B, , RO2, RH4, , R2O5, RH3, , 2, , Li, 6.939, , Be, 9.012, , B, 10.81, , C, 12.011, , N, 14.007, , O, 15.999, , F, 18.998, , 3, , Na, 22.99, , Mg, 24.31, , Al, 29.98, , Sl, 28.09, , P, 30.974, , S, 32.06, , Cl, 35.453, , As, 74.92, , In, 114.82, , Sn, 118.69, , Sb, 121.75, , 6 First Cs, Ba, series: 132.90, 137.34, Second, Au, Hg, , La, 138.91, Tl, , Hf, 178.49, Pb, , Ta, 180.95, Bi, , 204.37, , 207.19, , 208.98, , 196.97, , 200.59, , Zr, 91.22, , V, 50.94, , 5 First Rb, Sr, series: 85.47, 87.62, Second, Ag, Cd, series:, 107.87, 112.40, , series:, , Y, 88.91, , Ge, 72.59, , B, , Nb, 92.91, , A, , R2O7, RH, , H, 1,008, , Ti, 47.90, , A, , RO3, RH2, , VIII, , 1, , Ga, 69.72, , B, , VII, , A, , Sc, 44.96, , A, , VI, , Periods, ↓, , 4 First K, Ca, series: 39.102, 40.08, Second, Cu, Zn, series:, 63.54, 65.37, , A, , V, , B A, , Cr, 50.20, Se, 78.96, Mo, 95.94, Te, 127.60, W, 183.85, , B, , RO4, Transition, series, , Mn Fe, Co, Ni, 54.94 55.85 58.93 58.71, Br, 79.909, Tc Ru, Rh, Pd, 99 101.07 102.91106.4, I, 126.90, Os, Ir, Pt, 190.2 192.2 195.09, , The extent of knowledge regarding the chemical properties of the elements and, his insight into the system of periodicty possessed by the elements under certain, arrangement have no parallel in the history of chemistry. This work laid strong, foundation of the fundamental principles of the periodic law. One of his most, important conclusions was that the elements if arranged according to their atomic, weights, exhibit an evident systematic reoccurence of properties (periodicity of, properties) and even the properties of some elements were listed much before, their discovery. Mendeleev’s periodic Table (Table 3.1) was quite useful till the, discovery of atomic number. There existed certain inherent defects which opposed, the system., 78, , CHEMISTRY
Page 108 :
Periodic Table and Periodicity in Properties, , 3.3 MODERN APPROACH, , MODULE - 2, Atomic Structure and, Chemical Bonding, , Atomic number was discovered in 1913 by a team lead by Mosely. The periodic, table based on atomic number is termed as Modern Periodic Table. Moseley, arranged all the elements according to increasing atomic number and showed, that the properties of elements are periodic function of their atomic numbers., Modern periodic law: The properties of the elements are periodic function of, their atomic numbers., , Notes, , 3.4 LONG FORM OF PERIODIC TABLE, The arrangement of elements in the long form of periodic table is a perfect matching, of electronic configuration of the elements on one hand and physical and chemical, properties of the elements on the other. Some important considerations of the, modern atomic structure applied to the classification of elements are discussed, below:, (i) An atom loses electron(s) from or gains electron(s) in the outermost shell of, an atom during a chemical reaction., (ii) The sharing of an electron or electrons by an atom with other atom or atoms, is largely through the outer most shell. Thus the electrons in the outermost, shell of an atom largely determine the chemical properties of the elements., We may therefore conclude that the elements possessing identical outer electronic, comfiguration should possess similar physical and chemical properties and, therefore they should be placed together for an easy and systematic study., Keeping in mind the reasoning given above, when all the known elements are, arranged in a table according to their increasing atomic number, the properties of, the elements show periodicity (reappear at definite intervals). The periodicity is, shown in Table in 3.2., , 3.5 STRUCTURAL FEATURES OF THE LONG FORM OF, PERIODIC TABLE, (i) In this table there are 18 vertical columns called GROUPS. They are, numbered from 1 to 18. Every group has a unique configuration., (ii) There are seven horizontal rows. These rows are called PERIODS. Thus, the periodic table has seven periods, numbered from 1 to 7., (iii) There are a total of 114 elements known to us till today. Of all the known, elements 90 are naturally occurring and others are made through nuclear, transformations or are synthesised artificially. Either way they are Man-made, Elements, but you will find the term specifically applied to transuranic, elements (elements listed after uranium) only., CHEMISTRY, , 79
Page 109 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Periodic Table and Periodicity in Properties, , (iv) First period consists of only two elements (very short period). Second and, third periods consist of only eight elements each (short periods). Fourth and, fifth periods consist of 18 elements each (long periods). Sixth period consists, of 32 elements (long period). Seventh period is yet incomplete and more and, more elements are likely to be added as the scientific research advances., (v) There are also nick names given to the groups or a cluster of groups on the, basis of the similarity of their properties, as given below:, Group 1 elements except hydrogen, are called Alkali Metals, Group 2 elements are called Alkaline Earth Metals., Group 3 to 12 elements are called Transition Metals., Group 16 elements are called Chalcogens, Group 17 elements are called Halogens, Group 18 elements are called Noble Gases., Apart from what has been said above elements with atomic numbers 58 to 71, are called Lanthanoids – or Inner Transition elements (First series). Elements, from atomic numbers 90 to 103 are called actinoids – Inner Transition elements, (Second series). All elements except transition and inner transition elements are, also collectively called Main Group Elements., , 3.6 POSITION OF METALS, NON-METALS AND, METALLOIDS, In order to locate the position of metals, non-metals and metalloids in the periodic, table, you may draw a diagonal line joining the element boron (At. no. 5) with, that of tellurium (At. no. 52) and passing through silicon and arsenic. Now we, are in a position to make the following observations., (i) The elements above the diagonal line and to the far right are non-metals, (except selenium which shows slightly metallic character also). The nonmetallic character is more marked the farther an element is from the diagonal, line and up., (ii) The elements below the diagonal line and to the left are metals. (Hydrogen is, a non-metal and is an exception)The metallic character is more marked the, farther an element is from the diagonal line and down. All lanthanoids and, actinoids are metals., (iii) The elements along the diagonal line are metalloids and possess the, characteristics of metals as well as of non-metals. In addition germanium,, antimony and selenium also show the characteristics of metalloids., , 80, , CHEMISTRY
Page 111 :
MODULE - 2, , Periodic Table and Periodicity in Properties, , Atomic Structure and, Chemical Bonding, , INTEXT QUESTIONS 3.1, 1. Classify the elements of group 14, 15 and 16 into metals, non-metals and, metalloids., 2. Compare the metallic character of aluminium and potassium., Notes, , 3. Name the group number for the following type of clements, (i) Alkaline earth metals, (ii) Alkali metals, (iii) Transition metals, (iv) Halogens, (v) Noble gases., 4. Name five man made elements., , 3.7 CATAGORISATION OF ELEMENTS INTO ‘s’, ‘p’, ‘d’,, AND ‘f’ BLOCKS, , S-Block, , Grouping of elements in the periodic table can be done in another way also,, which is more related to their electronic configuration. Under this categorisation,, the location of the differentiating electron (the last electron) is most important., If, for example, the electron has gone to ‘s-subshell’, the elements will fall in ‘sblock’ and if the last electron goes to ‘p-subshell’, then the element will belong to, p-block. Similarly if the defferentiating electron enters the ‘d-subshell’, of an, atom, then the elements comprising all such atoms will belong to d-block., , p-Block, , d-Block, , f-Block, , Fig. 3.1 : Blockwise categorization of elements., , 82, , CHEMISTRY
Page 112 :
MODULE - 2, , Periodic Table and Periodicity in Properties, , There are minor exceptions in Mn and Zn configurations. You will study more, about the reasons for such exceptions in Lesson 23., , Atomic Structure and, Chemical Bonding, , The grouping of elements explained above can be related to the type of elements, discussed earlier:, (i), , s-block elements: All alkali metals and alkaline earth metals., , (ii) p-block elements: All elements of group number 13 to group number 18., , Notes, , (iii) d-block elements: All elements from group no. 3 to group no. 12 except, Lanthanoids and Actinoides., (iv) f-block elements: Lanthanoids (atomic number 58 to 71) and Actinoids, (atomic number 90 to 103), This is shown in Fig. 3.1., Nomenclature of Elements with Atomic Numbers greater than 100, The naming of the new elements was earlier left entirely to its discoverer. The, suggested names were then later ratified by IUPAC. But due to certain disputes, that arose over the original discoverer of some of the elements of atomic numbers, greater than 104, the IUPAC in 1994 appointed a Commission on Nomenclature, of Inorganic Chemisty (CNIC). After consultation with the Commission and the, chemists around the world, the IUPAC in 1997 recommeded a nomenclature to, be followed for naming the new elements with atomic numbers greater than 103, until their names are fully recognised., z, , The names are derived directly from the atomic number of the element using, the following numerical roots for 0 and numbers 1–9., 0 = nil, , 3 = tri, , 6 = hex, , 1 = un, , 4 = quad, , 7 = sept, , 2 = bi, , 5 = pent, , 8 = oct, , 9 = enn, , z, , The roots are put together in the order of the digits which make up the, atomic number and ‘ium’ is added at the end., , z, , Names, thus derives, and the IUPAC approved names of some elements with, atomic numbers greater than 103 are listed in Table 3.3., , CHEMISTRY, , 83
Page 113 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Periodic Table and Periodicity in Properties, Table 3.3 : Nomenclature of elements with atomic numbers greater than 103, Atomic, number, , Notes, , Name, , Symbol, , IUPAC approved, name, , IUPAC, symbol, , 104, , Unnilquadium, , Unq, , Rutherfordium, , Rf, , 105, , Unnilpentium, , Unp, , Dubnium, , Db, , 106, , Unnilhexium, , Unh, , Seaborgium, , Sg, , 107, , Unnilseptium, , Uns, , Bohrium, , Bh, , 108, , Unniloctium, , Uno, , Hassium, , Hs, , 109, , Unnilennium, , Une, , Meitnerium, , Mt, , 110, , Ununnillium, , Uun, , –, , –, , 111, , Unununnium, , Uuu, , –, , –, , 112, , Ununbium, , Uub, , –, , –, , 113, , Ununtrium, , Uul, , –, , –, , 114, , Ununquadium, , Uuq, , –, , –, , 115, , Ununpentium, , Uup, , –, , –, , 3.8 PERIODICITY IN ATOMIC PROPERTIES, The term periodicity is used to indicate that some characteristic properties occur, in the periodic table after definite intervals, however with a varying magnitude., Thus after starting from a certain point on the periodic table, we are almost, certain that the movement in a particular direction will show steady increase or, decrease of a said property., , 3.9 ATOMIC SIZE, In homonuclear diatomic molecules the distance from the centre of one nuclus to, the centre of another nucleus gives the bond length and half of this bond length is, atomic radius (Fig. 3.2). The first member of each period is the largest in size., Thus we can say that the group 1 atom are the largest in their respective horizontal, rows. Similarly, atoms of group 2 elements are large but are definitely smaller, than the corresponding atoms of group 1. This is due to the reason that the extra, charge on the nucleus draws the electrons inward resulting in smaller size for the, atoms under reference. This trend of decrease in size of atoms, continues from, left to right. An example is shown in Fig. 3.3. However, there may be some, exceptions and there will be other reasons to explain them., A, , Fig 3.2 : Atomic radius =, , 84, , A, , 1, d, =r, 2 A–A, , CHEMISTRY
Page 114 :
MODULE - 2, , Periodic Table and Periodicity in Properties, , Atomic Structure and, Chemical Bonding, , Li, , Be B C N O F, , Fig. 3.3: From left to right, size of atoms decrease in the perodic table, , In going down the group of elements (in any particular column) the atomic size, increases at each step., , Notes, , This increase may be explained in terms of a new electron shell being added,, when we pass from one element to another in a group., , 3.10 IONIC SIZE, An ion is formed when an atom undergoes a loss or gain of electrons., → M+ (g) + e–, M (g) ⎯⎯, , (cation formation), , → M– (g), M (g) + e– ⎯⎯, , (anion formation), , A cation is formed when an atom loses the most loosely bound electron from its, outermost shell. The atom acquires a positive charge and becomes an ion (a, cation). A cation is smaller than its atom. On the removal of an electron, the, positive charge of the nucleus acts on lesser number of electrons than in the, neutral atom and thus greater pull is exerted by the nucleus, resulting in a smaller, size of the cation., An anion is bigger than its atom because on receipt of an electron in the outermost, orbit the number of negative charges increase and it outweighs the positive charges., Thus the hold of the nucleus on the shells decrease resulting in an increase in the, size of the anion., A cation is always smaller than its atom and an anion is always bigger than, its atom e.g. Na+ is smaller than Na, Cl- is bigger than Cl., z, , In the main groups, the ionic radii increase on descending the group. e.g., Li+, = 0.76 Å, Na+ = 1.02 Å, K+ = 1.38 Å, etc. It is due to the addition of extra, shell at each step., , z, , There is a decrease in the ionic radii of the positive ions on moving from, left to right across a period in the periodic table. e.g., Na+ = 1.02 Å, Mg2+ =, 0.72 Å, Al3+ = 0.535 Å, etc. It is due to the increase in the number of charges, on the nucleus and also due to the increase in the charge on the ion., , z, , The ionic radii of the negative ions, also decrease on moving from left to, right across a period. e.g., O2– = 1.40 Å, F– = 1.33 Å, etc. This is partly due, to increase in the number of charges on the nucleus and also due to the, decreasing charge on the ion., , CHEMISTRY, , 85
Page 115 :
MODULE - 2, , Periodic Table and Periodicity in Properties, , Atomic Structure and, Chemical Bonding, , INTEXT QUESTION 3.2, , Notes, , 1. Write the names of the elements with atomic numbers 105, 109, 112, 115, according to IUPAC nomenclature., 2. Arrange the following in the order of increasing size, Na+, Al3+, O2–, F–, 3. How does the size of atoms vary from left to right in a period and on, descending a group in the periodic table?, , 3.11 IONIZATION ENTHALPY, Ionization Enthalpy is the energy required to remove the most loosely bound, electron from an isolated atom in the gaseous state for one mole of an element. It, is expressed in kJ mol–1 (kilojules per mole)., M(g) + IE → M+(g) + e–, As we move from left to right in the periodic table, there is a nearly regular, increase in the magnitude of the ionization enthalpy of elements., Similarly, on moving down a group the magnitude of the ionization enthalpy, indicates a regular decline. The ionization enthalpy of the first member of any, group is the highest within that group and the ionization enthalpy of the last, member in the same group, is the least. This is shown in table 3.4., Table 3.4: First ionization enthalpies of the elements (in kJ mol-1), Group 1, 1, , 2, , 3, , 2, , 3, , 4, , 5, , 6, , 7, , 8, , 9, , 10, , 11, , 12, , 6, , 16, , 17, , 18, He, •, , 1311, , 2372, , Li, , Be, , B, , C, , N, , O, , F, , •, , •, , •, , •, , •, , •, , •, , Ne, •, , 520 899, , 801 1086 1403 1410 1681, , Na, , Mg, , Al, , Si, , P, , S, , Cl, , Ar, , •, , •, , •, , •, , •, , •, , •, , •, , 2081, , 577 786, , 1012 999 1255, , 1521, , K, , Ca, , Se, , Ti, , V, , Cr, , Mn, , Fe, , Co, , Ni, , Cu, , Zn, , Ga, , Ge, , As, , Se, , Br, , Kr, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , 656 650 652 717 762 758 736 745 906 579 760, , 947 941 1142, , 1351, , Rb, , Sr, , Y, , Zr, , Nb, , Mo, , Te, , Ru, , Rn, , Pd, , Ag, , Cd, , In, , Sn, , Sb, , Te, , I, , Xe, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , 403 549 616, , 674 664 685 703 711 720 804 731 876 558 708, , 834 869 1191, , 1170, , Cs, , Ba, , La, , Hf, , Ta, , W, , Re, , Os, , Ti, , Pt, , Au, , Hg, , TI, , Pb, , Bi, , Po, , At, , Rn, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , •, , 376 503 541, 7, , 15, , •, , 419 590 631, 5, , 14, , H, , 496 737, 4, , 13, , Fr, , 760 760 770 759 840 900 870 889 1007 589 1007 589 715 703, , •, 813, , Ra Ac, 912 1037, , 86, , CHEMISTRY
Page 116 :
Periodic Table and Periodicity in Properties, , The variation in the magnitude of ionization enthalpy of elements in the periodic, table is mainly dependent on the following factors:, , MODULE - 2, Atomic Structure and, Chemical Bonding, , (a) The size of the atom, (b) The magnitude of the nuclear charge on the atom,, (c) The extent of screening, (d) The type of orbital involved (s, p, d, or f)., In small atoms, the electrons are tightly held whereas in large atoms the, electron are less strongly held. Thus, the ionization enthalpy decreases as the, size of the atom increases., , z, , When an electron is removed from an atom, the effective nuclear charge, i.e.,, the ratio of the number of charges on the nucleus to the number of electrons,, increases. As a result the remaining electrons come closer to the nucleus and, are held more tightly. The removal of a second electron, therefore, requires, more energy. e.g., Mg+ is smaller than the Mg atom The remaining electrons, in Mg+ are more tightly held. The second ionisation enthalpy is, therefore,, more than the first ionisation enthalpy., , z, , Since the orbitals (s, p, d and f) have different shapes, the ionization enthalpy, depends on the type of electrons removed. e.g. an electron in an s orbital is, more tighly held as compared to an electron in a p orbital. It is because an s, electron is nearer to the nucleus as compared to a p electron. Similarily a pelectron is more tightly held than a d-electron, and a d-electron is more tightly, held than a f-electron. If all other factors are equal, the ionization enthalpies, are in the order s > p > d > f., , Ionization energy (kJ mol–1), , z, , Notes, , Atomic number, , Fig 3.4 : Variation of ioniztion enthalpy of elements., , These factors taken together contribute largely to decide the extent of the force, of attraction between the nucleus and the electrons around it. The resultant of, CHEMISTRY, , 87
Page 117 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Periodic Table and Periodicity in Properties, , these factors thus determine the magnitude of ionization enthalpy of any element., You can see the variation in the magnitude of the ionization enthalpy of elements, with atomic number in the Fig. 3.4., It is clear from Fig. 3.4 that, (i) the metals of group 1 (Li, Na, K, Rb, etc.) have the lowest ionization enthalpies, in their respective periods., , Notes, , (ii) the noble gases (He, Ne, Ar, Kr, Xe and Rn) have the highest ionization, enthalpies in their respective periods. It is because the energy required to, remove an electron from a stable fully filled shell is very large., (iii) The values of ionization energies do not increase smoothly. e.g. the first, ionization enthalpy of B (boron) is lower than that of Be (beryllium); the, ionization enthalpy of Al (aluminium) is lower than that of Mg (magnesium);, the first ionization enthalpy of O(oxygen) is lower than that of N (nitrogen)., It can be explained as follows., z, , The first ionization enthalpies of Be and Mg are higher than those of their, preceding elements because the elecrons are removed from the fully filled sorbitals., , z, , The first ionization enthalpy of N is higher than that of O because from N,, the electron is to be removed from a half-filled p-orbitals, Ionization enthalpy is the energy required to remove the most loosely, bound electron form an atom (in the gaseous state) for one mole of an, element. It is an absolute value and can be determined experimentally., , 3.12 ELECTRON GAIN ENTHALPY, Every atom, in general, has a tendency to gain or loose electrons in order to, acquire a noble gas configuration. The atom which have five, six or seven electrons, in their outermost shell show tendency to accept electrons and attain the nearest, noble gas configuration. Halogens, for example, have seven electrons in their, outermost orbit. Thus they show a tendency to accept one more electron and, attain the nearest noble gas configuration. The energy change (ΔE) for this process, is called electron gain enthalpy of that atom., Electron gain enthalpy is the energy released for one mole of neutral atoms, in a gaseous state when electron is accepted by each atom., → X –(g), X(g) + e– ⎯⎯, , where X represents an atom., → Cl– (g): ΔE = –349 kJ mol-1, Cl (g) + e– ⎯⎯, , 88, , CHEMISTRY
Page 118 :
MODULE - 2, , Periodic Table and Periodicity in Properties, , The negative value shows release of energy and hence tendency to greater, stabilisation. The electron gain enthalpy becomes more in negative from left to, right in a period. This is because it is easier to add an electron to a smaller atom, since the added electron would be closer to the positively charged nucleus., Halogens release maximum energy when they accept an electron. On the other, hand, metals do not accept electrons and show a high positive value for ΔE. Thus, electron gain enthalpy can be positive or negative., Electron gain enthalpies becomes less in negative as we go down the group, showing that the electropositive character of the atoms increases. This is because, the size of the atom increases down the group and the electron added goes to the, higher shells. Electron affinity values for some elements are shown in table 3.5,, along with their position in the periodic table. The electron gain enthalpy of chlorine, is more in negative value as compared to that of fluroine. This is due to the small, size of the F atom. As the electron approaches the small F atom, it expriences a, repulsion from other electrons., , Atomic Structure and, Chemical Bonding, , Notes, , Table 3.5: Electeron gain enthalpy in kJ mol-1, Group, Period 1, 1, 2, 3, 4, 5, , 2, , 13, , 14, , 15, , 16, , 17, , 18, , H, , He, , –73, , +98, , Li, , Be, , B, , C, , N, , O, , F, , Ne, , –59.6, , (0), , –26.7, , –154, , –7, , –111, , –328, , +116, , Na, , Cl, , Ar, , –53, , –349, , + 96, , K, , Br, , Kr, , –48, , –325, , + 96, , Rb, , I, , Xe, , –47, , –295, , + 77, , 6, , Rn, + 68, , 3.13 ELECTRONEGATIVITY, It is an indicator of the extent of attraction by which electrons of the bond pair, are attracted by an atom linked by this bond. The value of electronegativity is, assigned arbitrarily to one atom such as hydrogen. Then the value of, electronegativity is assigned to all other atoms with respect to hydrogen. One, such scale is the Pauling Scale of electronegativity (Table 3.6)., Electronegativity is defined as a measure of the ability of an atom to attract, the electron pair in a covalent bond to itself., , CHEMISTRY, , 89
Page 119 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Periodic Table and Periodicity in Properties, , In a homonuclear diatomic molecule such as hydrogen (H2) or fluorine (F2), the, electron pair of the covalent bond in each molecule experiences equal attraction, by each atom. Thus none of the two atoms is able to shift the bond pair of electrons, to itself. However in a heteronuclear diatomic molecule, the bond pair electrons, get shifted towards the atom which is more electronegative than the other. For, example, in HF or HCl the bond pair of electrons are not shared equally but the, more electronegative atom F or Cl is able to shift the bond pair towards itself,, resulting in the polarization of the molecule., A large difference between electronegativities of the two atoms indicates highly, ionic character of the bond between them, for example in Cs+F –. On the other, hand, zero difference in the electronegativities between the two atoms indicates, that the percentage ionic character is zero. Therefore the molecule is purly covalent, e.g. H2, Cl2, N2 etc., Table 3.6 : Electronegativities of elements on Pauling scale., Li, , Be, , B, , C, , N, , O, , F, , 1.0, , 1.5, , 2.0, , 2.5, , 3.0, , 3.5, , 4.0, , Na, , Mg, , Al, , Si, , P, , S, , Cl, , 0.9, , 1.2, , 1.5, , 1.8, , 2.1, , 2.5, , 3.0, , K, , Ca, , Se, , Ge, , As, , Sc, , Br, , 0.8, , 1.0, , 1.3, , 1.7, , 1.8, , 2.1, , 2.5, , Cs, , Ba, , 0.7, , 0.9, , The most electronegative elements have been placed on the farthest right hand, upper corner (noble gases are not included). The value of electronegativity, decreases as we go down in any group and increases from left to right in the, period. Thus fluorine is the most electronegative and caesium is the least, electronegative element. (We have not considered Francium being radioactive)., , 3.14 CONCEPT OF VALENCE OR VALENCY, You that that different elements have different number of electrons in the, outermost or the valence shell. These electrons in the outermost shell are known, as valence electrons. The number of valence electrons determines the, combining capacity of an atom in an element. Valence is the number of, chemical bonds that an atom can form with univalent atoms. Since hydrogen, is a univalent atom, the valence of an element can be taken by the number of, atoms of hydrogen with which one atom of the element can combine. For, example, in H2O, NH3, and CH4 the valencies of oxygen, nitrogen and carbon, are 2, 3 and 4, respectively., The elements having a completely filled outermost shell in their atoms show little, or no chemical activity. In other words, their combining capacity or valency is, zero. The elements with completely filled valence shells are said to have stable, 90, , CHEMISTRY
Page 120 :
Periodic Table and Periodicity in Properties, , electronic configuration. The main group elements can have a maximum of eight, electrons in their valence shell. This is called octet rule; you will learn more about, it in lesson 7. You will learn that the combining capacity or the tendency of an, atom to react with other atoms to form molecules depends on the ease with, which it can achieve octet in its outermost shell. The valencies of the elements, can be calculated from the electronic configuration by applying the octet rule., z, , If the number of valence electrons is four or less then the valency is equal, to the number of the valence electrons., , z, , In cases when the number of valence electrons is more than four then, generally the valency is equal to 8 minus the number of valence electrons., , MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Thus,, Valency = Number of valence electrons (for 4 or lesser valence electrons), Valency = 8 - Number of valence electrons (for more than 4 valence electrons), The composition and electronic configuration of the elements having the atomic, numbers from 1 to 18, along with their valencies is given in Table 3.7., Table 3.7: The composition, electron distribution and common valency, of the elements with atomic number from 1 to 18, , *However, the elements in the 3rd and higher periods may show higher valencie, than, predicted by octect rule since more than 8 electrons can be accommodate in their outermost, shells due to available d orbitas,., , CHEMISTRY, , 91
Page 121 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Periodic Table and Periodicity in Properties, , 3.14.1 Electron Configurations and the Periodic Table, By this time you can see a pattern develop among the ground state electron, configurations of the atoms. This pattern explains the periodic table. Consider, helium, neon, argon, and krypton, elements in Group 18 of the periodic table., Neon, argon, and krypton have configurations in which a p subshell has just, filled. (Helium has a filled 1s subshell; no lp subshell is possible.), , Notes, , helium, , 1s2, , neon, , 1s2 2s22p6, , argon, , 1s22s22p63s23p6, , krypton, , 1s22s22p6 3s23p63d104s241)6, , These elements are the members of the group called noble gases because of, their relative unreactivity., Look now at the configurations of beryllium, magnesium, and calcium, members, of the group of alkaline earth metals (Group 2), which are similar, moderately, reactive elements., beryllium, , 1s22s2, , magnesium 1s22s22p63s2, calcium, , Is22s22p6 3s23p64s2, , or, , [He]2s2, , or, , [Ne]3s2, , or, , [Ar]4s2, , Each of these configurations consists of a noble gas core, that is, an inner shell, configuration corresponding to one of the noble gases, plus two outer electrons, with an ns2 configuration., The elements boron, aluminum, and gallium (Group 13) also have similarities., Their configurations are, 2, , 2s22p], , or, , [He]2s22pI, , or, , [Ne]3s23p1, , boron, , Is, , aluminum, , ls2 2s22p6 3s23p], , gallium, , 2s2 2s22p6 3s2 3p6 3d104s2 4pl or, , [Ar]3d104s24p1, , Boron and aluminum have noble-gas cores plus three electrons with the, configuration ns2 npl. Gallium has an additional filled 3d subshell. The noblegas core together with (n - 1)d10 electrons is often referred to as a pseudonoble gas core, because these electrons usually are not involved in chemical, reactions., An electron in a core outside the noble-gas or pseudo-noble-gas core is called, a valence electron. Such electrons are primarily involved in chemical reactions,, and similarities among the configurations of valence electrons (the valence-shell, configurations) account for similarities in the chemical properties among the, groups of elements., 92, , CHEMISTRY
Page 122 :
MODULE - 2, , Periodic Table and Periodicity in Properties, , Atomic Structure and, Chemical Bonding, , INTEXT QUESTIONS 3.3, 1. What is the correlation between atomic size and ionization enthalpy., 2. Which species, in each pair is expected to have higher ionization enthalpy., (i), (iii), (v), (vii), , 3Li, 11Na, 20Ca, 12Mg, , (ii) 7N,, , 15P, , (iv), , 13Al, 14Si, , 17Cl, 18Ar, , (vi), , 18Ar, 19K, , Notes, , 13Al, 14C, , 3. Account for the fact that there is a decrease in first ionization enthalpy from, Be to B and Mg to Al., 4. Why is the ionization enthalpy of the noble gases highest in their respective, periods?, 5. Name the most electronegative element., , WHAT YOU HAVE LEARNT, z, z, z, z, z, z, z, z, z, z, z, , The classification of elements makes their study systematic., The arrangement of elements in the long form of the periodic table depends, on their electornic configuration., The properties of the elements are the periodic function of their atomic, number., All the known elements are arranged in 18 groups in the long form of periodic, table, There are seven horizontal rows (periods) in the long from of the periodic, table., Elements of groups 1 and 2 are known as alkali metals and alkaline earth, metals respectively., Elements of groups 17 and 18 are known as halogens and noble gases, respectively., s, p, d and f are the four blocks in the periodic table classified on the basis of, their outer most electrons residing in s, p, d or f sub-shell., The elements can be classified into metals, non-metals and metalloids on the, basis of their properties and their position in the periodic table., The atomic size, ionic size, ionization enthalpy, electron gain enthalpy and, electronegativity and valence show regular trends along a group and a period., Valence can be explained., , CHEMISTRY, , 93
Page 123 :
MODULE - 2, , Periodic Table and Periodicity in Properties, , Atomic Structure and, Chemical Bonding, , TERMINAL EXERCISE, 1. Define modern periodic law., 2. Refer the periodic table given in Table 3.2 and answer the following, questions., Notes, , (i) The elements placed in group number 18 are called ..............., (ii) Alkali and alkaline earth metals are collectively called ............... block, metals., (iii) The general configuration for halogens is ..............., (iv) Name a p-block element which is a gas other than a noble gas or a, hologen., (v) Name the groups that comprise the ‘s’ block of elements., (vi) Element number 118 has not yet been established, to which block, will, it belong?, (vii) How many elements should be there in total if all the 7s, 7p, 6d and, 5f, blocks are to be full?, 3. Describe the variation of electron affinity and ionization enthalpy in the, periodic table., 4. Define the following:, (a) Electron gain enthalpy, , (b) Ionization enthalpy, , (c) Ionic radius, , (d) Electronegativity., , 5. What is electronegativity? How is it related to the type of bond formed?, 6. Why is the electron gain enthalpy of Cl more in negative value as compared, to that of F?, , ANSEWERS TO INTEXT QUESTIONS, 3.1, 1., , 94, , Metals, , Non metals, , Metalloids, , Sn, Pb, , C, , Si, Ge, , Sb, Bi, , N, P, , As, , Te, Po, , O, S, , Se, , CHEMISTRY
Page 124 :
MODULE - 2, , Periodic Table and Periodicity in Properties, , Atomic Structure and, Chemical Bonding, , 2., , Potassium is more metallic than aluminum., , 3., , (i) 2, , (ii) 1, , (iii) 3 - 12, , (iv) 17, , (v) 18, , 4., , Np,, , Lr,, , No,, , Rf,, , Hs., , 3.2, 1., , (i) Unnilpentium,, , Notes, , (ii) unnilennium,, (iii) Ununbium,, (iv) Ununpentium, 2., , Al3+, Na+, F –, O2–, , 3., , The atomic size decreases from left to right across a period and increases, on moving down the group., , 3.3, 1., , Ionization enthalpy decreases with increase in atomic size and vice-versa., , 2., , (i) 3Li, , (ii) 7N, , (iii) 12Mg, , (iv) 14Si, , (v) 12Ar, , (vi), , 18, , Ar, , (vii) 6C, , 3., , The electronic configureation of Be is 1s2 2s2 whereas that of B is 1s2 2s2, 2p1. In case of Be, the electron is to be removed from completely filled s, orbital whereas in case of B it is to be removed from a singly occupied p, orbital. Fully-filled orbitals are more stable. Hence, ionization enthalpy, decreases from Be to B. Similarily it decreases from Mg to Al., , 4., , The noble gases have fully filled shells and are stable. Hence, they have, the highest ionization enthalpies in their respective periods., , 5., , Fluorine., , CHEMISTRY, , 95
Page 125 :
MODULE - 2, , Chemical Bonding, , Atomic Structure and, Chemical Bonding, , 4, Notes, , CHEMICAL BONDING, , In lesson 2, you have learnt about the structure of atom while in the lesson 3,, you studied about the classification of elements and periodicity in properties ., You know that molecules are obtained by the combination of two or more than, two atoms of the same or different elements. In this lesson you will study, z, , Why do atoms combine?, , z, , What are the different ways in which the atoms can combine?, and, , z, , What are the shapes of diffe rent molecules?, , The answers to these questions are of fundamental importance to the study of, chemistry, as you would discover while studying the later parts of this course., , OBJECTIVES, After reading this lesson you will be able to, , 96, , z, , explain the formation of bond in terms of potential energy diagram and octet, rule;, , z, , list different types of bonds;, , z, , define ionic bond and cite some examples;, , z, , write Lewis structures of some simple molecules;, , z, , list the characteristics of ionic compounds;, , z, , explain Born Haber Cycle;, , z, , define covalent bond and cite some examples;, , z, , list the characteristics of covalent compounds;, , z, , state valence shell electron pair repulsion (VSEPR) theory;, , CHEMISTRY
Page 126 :
Chemical Bonding, z, , explain bond polarity and dipole moment;, , z, , explain bond parameters;, , z, , predict the geometry of molecules with the help of VSEPR theory;, , z, , explain the hybridisation of atomic orbitals involving s, p and d orbitals and, illustrate with examples;, , z, , tabulate the geometry of some molecules showing sp, sp2, sp3, dsp2, and, dsp3 hybridisation;, , z, , explain the formation of σ and π bonds in CH4 , C2H4 and C2H2 ;, , z, , explain resonance;, , z, , explain molecular orbital theory;, , z, , write the molecular orbital configuration of H2, N2, O2 and F2 molecules;, , z, , define bond length and bond order and relate them and, , z, , explain hydrogen bonding with the help of examples., , MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , 4.1 VALENCE ELECTRONS, The electrons in the outer most shell take part in the bond formation and, determine the combining capacity or the ‘valency’ of the atom. Therefore, the, outer most shell of any atom is called its valence shell and the electrons present, in the valence shell are called the valence electrons., , 4.2 WHAT IS A CHEMICAL BOND?, When two atoms of same or different elements approach each other, the energy, of the combination of the atoms becomes less than the sum of the energies of the, two separate atoms at a large distance. We say that the two atoms have combined, or a bond is formed between the two. The bond is called a chemical bond. Thus, a chemical bond may be visualised as an effect that leads to the decrease in the, energy. The combination of atoms leads to the formation of a molecule that has, distinct properties different from that of the constituent atoms., A question arises, “ How do atoms achieve the decrease in energy to form the, bond”. The answer lies in the electronic configuration. As you are aware, the, noble gases do not react with other elements to form compounds. This is due to, their stable electronic configuration with eight electrons (two in case of helium), in their outermost shells. The formation of a bond between two atoms may be, visualised in terms of their acquiring stable electronic configurations. That is, when two atoms (other than that of noble gases) combine they will do so in such, a way that they attain an electronic configuration of the nearest noble gas., , CHEMISTRY, , 97
Page 127 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Chemical Bonding, , The stable electronic configuration of the noble gases can be achieved in a number, of ways; by losing, gaining or sharing of electrons. Accordingly, there are different, types of chemical bonds, like,, z, , Ionic or electrovalent bond, , z, , Covalent bond, , z, , Co-ordinate covalent bond, , In addition to these we have a special kind of bond called hydrogen bond. Let us, discuss about different types of bonds, their formation and the properties of the, compounds so formed., , 4.3 IONIC OR ELECTOVALENT BOND, According to Kossel’s theory, in the process of formation of ionic bond the atoms, acquire the noble gas electronic configuration by the gain or loss of electrons., Let us consider the formation of NaCl in terms of Kossel’s Theory., The electronic configuration of sodium atom (atomic number 11) is 2,8,1. Since, it is highly electropositive, it readily loses an electron to attain the stable, configuration of the nearest noble gas (neon) atom. It becomes a positively charged, sodium cation (Na+) in the process, +, –, Na ⎯⎯, → Na + e ;, , ΔH = 493.8 kJ mol–1, , 2,8,1, , ΔH is enthalpy change), (Δ, , 2,8, , On the other hand, a chlorine atom (electronic configuration: 2,8,7) requires one, electron to acquire the stable electronic arrangement of an argon atom. It becomes, a negatively charged chloride anion (Cl–) in the process., Cl, , ΔH = –379.5 kJ mol–1, , –, , + e– ⎯⎯, → Cl ;, , 2,8,7, , 2,8,8, , According to Kossel’s theory, there is a transfer of one electron from sodium, atom to chlorine atom and both the atoms attain noble gas configuration., Na, 2,8,1, , +, , Cl, 2,8, 7, , ¾¾® Na, 2,8, , +, , +, , Cl –, 2,8,8, , The positively charged sodium ion and the negatively charged chloride ion are, held together by electrostatic attractions. The bond so formed is called an, electrovalent or an ionic bond. Thus the ionic bond can be visualised as the, electrostatic force of attraction that holds the cation and anion together. The, compounds so formed are termed as ionic or electrovalent compounds., , 98, , CHEMISTRY
Page 128 :
MODULE - 2, , Chemical Bonding, , 4.3.1 Energetics of Ionic Compound Formation, We have just described the formation of an ionic compound (NaCl) as a result of, transfer of electrons as proposed by Kossel. You may raise a question here that, when more energy is required (ionisation energy) to form a sodium ion from, sodium atom, than that released (electron affinity) in the formation of chloride, ion from chlorine atom then how do we say that the formation of NaCl is, accompanied by a decrease in energy? Your question is quite justified but let us, assure you that there is no anomaly. Let us look at the whole process somewhat, closely to clarify your doubts., , Atomic Structure and, Chemical Bonding, , Notes, , Born Harber Cycle, The formation of NaCl from sodium and chlorine can be broken down into a, number of steps as :, a), , Sublimation of solid sodium to gaseous sodium atoms., ΔH = 108.7 kJ mol–1, , Na(s) ⎯⎯, → Na(g) ;, b), , Ionization of gaseous sodium atom to give sodium ion., +, –, Na(g) ⎯⎯, → Na (g) + e ;, , c), , Dissociation of gaseous chlorine molecule into chlorine atoms, 1, 2, , d), , ΔH = 120.9 kJ mol–1, , Cl2(g) ⎯⎯, → Cl(g) ;, , Conversion of gaseous chlorine atom to chloride ion (addition of electron), –, , Cl(g) + e– ⎯⎯, → Cl (g) ;, e), , ΔH = 493.8 kJ mol–1, , ΔH = –379.5 kJ mol–1, , Formation of NaCl from sodium and chloride ions.(Crystal or lattice, formation)., –, , –, , +, Na+(g) + Cl (g) ⎯⎯, → Na Cl (s) ;, , ΔH = –754.8 kJ mol–1, , The energy released in this step is lattice energy., The net reaction would be, Na(s) +, , 1, , +, –1, Cl2(g) ⎯⎯, → Na Cl (s) ; ΔH = –410.9 kJ mol, 2, –, , The overall energy change can be computed by taking the sum of all the energy, changes:, ΔH = ( 180.7 + 493.8 + 120.9 – 379.5 – 754.8 ) = - 410.9 kJ mol–1, Thus we see that the net process of formation of NaCl from sodium and chlorine, is accompanied by a large decrease in the energy. The approach we have just, followed is based on the law of conservation of energy and is known as BornHaber cycle., CHEMISTRY, , 99
Page 129 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Chemical Bonding, , Born Haber Cycle, ΔfH, , Na(s) + ½ Cl2(g), , Na+Cl−(s), , Δdiss H, Δsub H, , Cl(g), , Na(g), , Notes, , ΔegH, , ΔlatH, −, , +, , Cl (g) + Na (g), , Δion H, , Of the five different types of energies involved, two (sublimation and dissociation, energies) generally have low values than the rest. Therefore, the three energy, terms i.e., ionization energy, electron affinity and lattice energy are important in, determining the formation of an ionic compound. On the basis of the above, discussion we can say that the formation of an ionic compound is favoured by, i., , Low ionisation energy of the metal,, , ii., , High electron affinity of the other element (non-metal), and, , iii., , High lattice energy, , 4.3.2 Characteristic Properties of Ionic Compounds, z, , These exist as crystalline solids in which the ions are arranged in a regular, three dimensional structure. The ionic compounds are generally hard and, brittle in nature., , z, , These compounds have high melting and boiling points due to strong, electrostatic interactions between the ions., , z, , These are generally soluble in water and less soluble in non-polar solvents, like ether, alcohol, etc., , z, , These conduct electricity when in molten state or in aqueous solutions., , Kossel’s theory explains bonding quite well but only for a small class of solids, composed of electropositive elements of Group 1 and 2 with highly electronegative, elements. Secondly, this theory is incapable of explaining the formation of, molecules like, SO2 or O2 , etc. For example in case of O2, there is no reason to, expect that one atom of oxygen would lose two electrons while the other accepts, them. The problem was solved by Lewis theory of covalent bonding., , 4.4 COVALENT BOND, Like Kossel, Lewis also assumed that atoms attain noble gas electronic, configuration in the process of bond formation. However, the way the noble gas, electronic configuration is achieved, is different. Lewis proposed that this is, achieved by “sharing of a pair of electrons” between the two atoms. Both the, 100, , CHEMISTRY
Page 130 :
MODULE - 2, , Chemical Bonding, , atoms contribute an electron each to this pair. For example, two hydrogen atoms, form a molecule by sharing a pair of electrons. If electrons are indicated as dots,, formation of hydrogen molecule can be shown as, , Atomic Structure and, Chemical Bonding, , H . + . H ¾¾, ® H : H ¾¾, ®H—H, , This shared pair of electrons contributes towards the stability of both the atoms, and is said to be responsible for ‘bonding’ between the two atoms. Such a bond is, called covalent bond and the compounds so obtained are called covalent, compounds., , Notes, , 4.4.1 Lewis Structure, In the process of suggesting the process of chemical bonding Lewis provided a, very convenient way of representing bonding in simple molecules. This is called, Lewis electron-dot structures or simply Lewis structures., In Lewis structure each element is represented by a Lewis symbol. This symbol, consists of the normal chemical symbol of the element surrounded by number of, dots representing the electrons in the valence shell. Since the electrons are, represented by dots, these are called electron-dot structures. The Lewis symbols, of some elements are as:, , . Li, , ;, , . Be ., , ;, , .B., ., , ;, , ., ., .C. ; :N ., ., ., , ;, , .., , : O., , ., , ;, , :, , .., F, , .., , ., , .., , ;, , : Ne :, .., , You may note here that while writing the Lewis symbols, single dots are placed, first on each side of the chemical symbol then they are paired up. The Lewis, structure of a molecule is written in terms of these symbols, In terms of Lewis symbols the ionic bond formation in NaCl can be represented, as, Na . +, , .., . Cl :, .., , [ Na, , .., ., ] [ . Cl : ], .., +, , Cl, , and the covalent bond formation in HFl is represented as, H. +, , .., .F:, .., , .., , H, , :F :, .., , Sometimes the electrons contributed by different atoms are represented by different, symbols. For example, formation of HF may also be shown as, Hx +, , .., .F:, .., , .., ., Hx F :, .., , In this case the hydrogen electron is shown as a cross while the electrons of, fluorine are represented by dots. There is no difference between electrons; it is, just a presentation for the sake of convenience., CHEMISTRY, , 101
Page 131 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Chemical Bonding, , In terms of Lewis structures the formation of a chlorine molecule from two chlorine, atoms may be represented as, , :, , Notes, , .., , Cl, .. ., , +, , .., . Cl :, .., , .., , .. .., : Cl .. Cl :, .. .., , : Cl, .., , .., Cl, , .., , :, , Here each chlorine atom with seven valence electrons, contributes one electron, to the shared pair. In the process of bond formation both the chlorine atoms, acquire the electronic configuration of argon. In the same way, the formation of, oxygen molecule involves sharing of two pairs of electrons between the two, oxygen atoms. In this case both the atoms contribute two electrons each and, acquire eight electrons or an octet in their valence shell., , .., , :., , O, , ., , +, , ., , .., , .., , O, , O, , .:, , .., , ::, , .., , .., , .., , O, , O, , .., , .., , .., , O, , You may have noticed that in the process of bond formation, the elements of, second period acquire eight electrons in their valence shell. This is called ‘Octet, rule’. You may also note that in case of H2 and Cl2 the atoms are linked by a, single line while in case of O2 the atoms are linked by two lines. These lines, represent bonds. When two atoms are bound by sharing a single pair of electron,, they are said to be joined by a single bond. And when, two pairs of electrons are, shared (as in case of O2 ), the two atoms are said to be bound by a double bond., In nitrogen ( N2) the two atoms are joined by a triple bond as they share three, pairs of electrons., In a Lewis representation the electrons shown to be involved in the bond formation, are called bonding electrons; the pair of electrons is called ‘bond pair’ and the, pairs of electrons not involved in the bonding process are called ‘lone pairs’. The, nature of the electron pair plays an important role in determining the shapes of, the molecules. This aspect is discussed later in Section 4.4., 4.4.2 Coordinate Covalent Bond, You have learnt that in the formation of a covalent bond between the atoms, each, atom contributes one electron to the shared electron pair, However, in some, cases both the electrons of the shared pair are contributed by only one species, (atom, molecule or ion) A common example is the formation of a bond between, boron trifluoride (BF3) and ammonia (NH3). BF3 is an electron deficient molecule, and can accept a pair of electrons. The molecule of ammonia on the other hand is, electron rich. It has a lone pair of electrons on the nitrogen atom and that can be, donated. Electron rich ammonia donates a pair of electrons to electron deficient, BF3. Such electron donor-acceptor bonds are called coordinate covalent or dative, bonds., 102, , CHEMISTRY
Page 132 :
MODULE - 2, , Chemical Bonding, , H, , .., , H: N, , .., , .F., , :, , B, , .., , H, , Atomic Structure and, Chemical Bonding, , H, , :, , F, , OR, , F, , . . .F., H: N : B :, .. .., H, , F, , F, , A coordinate bond is normally represented by an arrow pointing from a donor, atom to the acceptor atom. A coordinate bond is identical to a covalent bond in, terms of its polarity and strength. The two are different only in the way they are, formed. We cannot distinguish between covalent and coordinate covalent bond,, once these are formed. HNO3 and NH4+ ion are some more common examples of, formation of a coordinate bond., , Notes, , +, , O, , H, H, , N, , H, , H, , O, , N, O, , H, , INTEXT QUESTION 4.1, 1. Define electrovalent bond., 2. Show the formation of a nitrogen molecule from two nitrogen atoms in terms, of Lewis theory., 3. What do you understand by a polar covalent bond? Give two examples., 4. What is a coordinate covalent bond ? How is it different from a covalent, bond?, 4.4.3 Characteristic properties of Covalent Compounds, z, , The covalent compounds have low melting and boiling points due to weak, forces of interaction between the molecules., , z, , The covalent compounds are poor conductors of electricity as these lack, ionic species., , z, , The covalent compounds are generally insoluble in water and dissolve in, nonpolar solvents like benzene, carbon tetrachloride etc., , 4.4.4 Polar Covalent Bond, In a chemical bond the shared electron pair is attracted by the nuclei of both the, atoms. When we write the electron dot formula for a given molecule this shared, electron pair is generally shown in the middle of the two atoms indicating that the, two atoms attract it equally. However, actually different kinds of atoms exert, different degrees of attraction on the shared pair of electrons. A more electroCHEMISTRY, , 103
Page 133 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Chemical Bonding, , negative atom has greater attraction for the shared pair of electrons in a molecule., As a consequence in most cases the sharing is not equal and the shared electron, pair lies more towards the atom with a higher electronegativity. For example, in, HCl, the shared pair of electron is attracted more towards more electronegative, chlorine atom. As a result of this unequal sharing of the electron pair , the bond, acquires polarity or partial ionic character., , Notes, H, , :, , .., Cl, , .., , :, , δ+, , δ–, , H ¯ Cl, , In an extreme case, the difference in the electronegativity may be so high that the, electron pair is practically under the influence of a single atom. In other words, the polarization of the bond is complete i.e., we have a case of ionic bonding., Thus , though the Lewis theory talks about covalent bonding it can account for, the formation of ionic compounds also., 4.4.5 Bond Polarity and Dipole Moment, A covalent bond is formed by sharing of a pair of electrons by the two atomseach one of which contributes one electron. If the two atoms have the same, electronegativity, then the sharing of the two bonded atoms is equal, see Fig., 4.1. This situation is encountered when both the atoms are of the same element, as in H2, Cl2, O2, N2 etc. Such a bond is pure covalent bond. It is non-polar, in nature. However, if the electronegativities of the two bonded atoms are, different as in HCl, then the shared pair of electrons lies more towards the more, electronegative atom i.e. chlorine. As a result, a small negative charge (δ−), develops on Cl atom and an equal positive charge (δ+) develops on H atom., The covalent bond in HCl is a polar covalent bond. It is not a pure covalent, bond as it has some ionic character., , Fig. 4.1: Non-polar or pure covalent bond; (b) polar covalent bond, , The extent of shift of the shared pair of electrons and hence the magnitude of, ionic character depends upon the difference in electronegativities of the two, bonded atoms. If it is 1.7, then the bond has 50% ionic character. If it is less, than 1.7, then ionic character is less than 50% and if the difference is more than, 1.7, then the ionic character is more than 50%., Dipole Moment, Charge separation in a covalent bond results in the formation of an electrical, dipole (two equal but opposite charges with a small distance between them)., Each electrical dipole is characterized by its dipole moment which is defined, as, 104, , CHEMISTRY
Page 134 :
MODULE - 2, , Chemical Bonding, , Dipole Moment (μ) = Magnitude of charge (Q) × Distance of Separation (r), , Atomic Structure and, Chemical Bonding, , It is commonly measured in the unit ‘debye’, symbol D. The SI unit of dipole, moment is Coulomb-metre (Cm). The two units are related as, 1D = 3.336 × 10–30 cm, The dipole moment depends upon the difference in electronegativities of the two, bonded atoms. It can be seen from the data given below, Bond, , Electronegativity of halogen atom, , Dipole moment/D, , H–F, H–Cl, H–Br, H–I, , 4.0, 3.0, 2.8, 2.5, , 1.90, 1.04, 0.79, 0.38, , Notes, , Dipole moment is a vector quantity since it has direction and magnitude both., By convention, the symbol (, ) is used to represent the dipole moment with, tail on the positive centre and the head pointing towards the negative centre., The dipole moment of HCl is represented as, H – Cl, Like forces, dipole moment vectors can be added and subtracted. By doing so,, the overall dipole moment of a molecule can be calculated. Let us consider some, examples to understand it., (i) Carbon dioxide, CO2, CO2 molecule is a linear triatomic molecule. Each C = O bond is polar with, the oxygen atom having the negative end of the bond dipole, O=C=O, The dipole moments of both the C = O bonds have the same magnitude but, are in opposite directions hence they cancel each other. The net dipole, moment of CO2 is zero., (ii) Water, H2O, , CHEMISTRY, , 105
Page 135 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Chemical Bonding, , H2O is a non-linear or bent diatomic molecule. Each H–O bond is a polar, covalent bond and has a dipole moment. Although dipole moments of both, the bonds are equal is magnitude, but due to non-linear shape, they do not, cancel each other. The net dipole moment of H2O molecule is 1.85D (or, 6.17 × 10–30 cm), (iii) Boron trifluoride, BF3, The net dipole moment for BF3 is zero due to its symmetrical planar structure, as shown below, although the B–F bonds are polar., F, , F, , B, F, F, Bond dipoles, , B, F, , (, , )=0, , +, , F, , (iv)Ammonia, NH3, NH3 has a pyramidal structure which makes the arrangement of three N–, H bonds unsymmetrical. In each N–H bond, nitrogen is the negative centre, and hydrogen is the positive centre. In addition to three N–H bonds, nitrogen, atom has a lone pair of electrons. which also has an orbital dipole moment, in which the electron pair is the negative centre., , H, , H, H, Bond and, orbital dipoles, , N, , N, , N, H, , H, , H, , Net bond dipole, and orbital dipole, , H, , H, , H, , Net dipole of, the molecule, , The net dipole moment of NH3 is 1.47D (4.90 × 10–30 Cm)., (v) Carbon tetrachloride, CCl4, It has a net zero dipole moment due to its symmetrical tetrahedral shape, Cl, C, , Net dipole, moment = O, Cl, , Cl, Cl, , 106, , CHEMISTRY
Page 136 :
Chemical Bonding, , 4.4.6 Covalent Character of Ionic Bond, In the earlier discussion, we have seen that most of the covalent bonds have, some ionic character. Similarly, ionic bonds also have some covalent character., The origion of covalent character of an ionic bond lies in the distortion of, electron cloud under the influence of an electric charge. This property of an, electron cloud to get distorted is called its polarizability and the process of, distortion of electron cloud is called polarization. The power of an electric, charge to distort an electron cloud is called its polarizing power. In an ionic, compound cations and anions are formed as a result of loss and gain of electrons,, respectively. Each ion tries to polarize the electron cloud of the other., , MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Generally, cations have greater polarizing power due to their small size and, hence high charge density. Anions are more easily polarized. Due to their large, size, the electrons in anions are more loosely bound to the nucleus than in, cations. Cation attracts the electron cloud of the anion and this results in its, polarization and the electron cloud spreads out towards cation in between the, two ions. Thus the electron lost by cation does not fully belong to the anion, but partially comes back towards cation and is shared by it. This results in some, covalent character in the ionic bond. The more the polarization, the more is the, covalent character., Fajan proposed some empiprical rules that deal with the polarization process., These rules are known as Fajan’s rules which are given below. The following, factors increase the covalent character of an ionic bond, (i) small cation, (ii) large anion, (iii) high positive charge on cation, (iv) cations with electronic configuration (n–1)dxns0 (typically transition metal, cations) as compared to cations with same size and charge but having ns2np6, (noble gas) configuration., 4.4.7 Covalent Bond Parameters, Each covalent bond is characterized by the following parameters which are, called covalent bond parameters., (1) Bond order, Bond order is the number of bonds present between two atoms in a molecule, or ion. Generally, bond order has an integral value. For example,, , CHEMISTRY, , 107
Page 137 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Chemical Bonding, , Bond order = 1, Bond order is 1 (one) when there is only one covalent bond (sigma bond), between the two bonded atoms. H2, Cl2, NH3 are some such examples. These, are called single bonds., Cl — Cl, (Cl2), , H—H, (H2), , Notes, , H—N—H, H (NH3), , In NH3 molecule, there are three single N–H bonds., Bond order = 2, Bond order is 2 (two) when there are two covalent bonds between the two, bonded atoms. Of the two, one is a sigma bond and the other is a pi bond. These, are called double bonds. Examples are as follows., H, , H, O O, (O2), , C O, (CO2), Two C = O bonds, O, , C, , C, , H, (C2H4), One C = C, , H, , Bond Order = 3, Bond order is 3 (three) when there are three bonds between the two bonded, atoms. Out of these, one is a sigma bond and two are pi bonds. These are called, triple bonds. Examples are given below., C, O, (CO), , H—C, C—H, (C2H2), , (2) Bond Length, Bond length is the distance between the nuclei of the two bonded atoms. It is, expressed in picometer (pm). Bond length depends upon the sizes of the bonded, atoms and the bond order of the bond linking them. The greater the bond order,, the smaller is the bond length., Bond, , 108, , Bond Length/pm, , Bond, , Bond Length/pm, , H–H, , 74, , O=O, , 121, , H–F, , 92, , N≡N, , 109, , H–Cl, , 127, , C–C, , 154, , H–Br, , 141, , C=C, , 134, , H–I, , 160, , C≡C, , 120, , C=O, , 122, , CHEMISTRY
Page 138 :
MODULE - 2, , Chemical Bonding, , Atomic Structure and, Chemical Bonding, , (3) Bond Angle, It is the angle between the two bonds in a molecule. Since covalent bonds are, formed through overlapping of orbitals, it may also be defined as the angle, between the two orbitals that contain bonding electrons and overlap with atomic, orbitals of other atoms. Bond angle between two bonds or bonding orbitals is, shown below, B, Bond, A, A, angle, , Notes, , The bond angle between two O–H bonds in H2O molecule is 104.5°, between, N–H bonds in NH3 molecule is 107.3° and between C–H bonds in CH4 is, 109°28’., (4) Bond Enthalpy ΔaH, It is the amount of energy required for breaking one mole of bonds of a particular, type in gaseous molecules. It is also called bond dissociation enthalpy, and is, applicable to simple molecules, containing only one bond of the particular type., For example, the bond enthalpy of Cl–Cl bond in Cl2 is 243 kJ mol–1, of O=O, in O2 is 498 kJ mol–1 and of N≡N bond in N2 is 946 kJ mol–1., A difficulty arises in using this definition in case of molecules that contain more, than one bond of the same type. H2O molecule has two O–H bonds and the, two bonds require different amounts of energies to break., H 2O(g) ¾¾, ® H(g) + OH(g) D a H10 = 502 kJ mol-1, OH(g) ¾¾, ® H(g) + O(g) D a H 02 = 427 kJ mol-1, , Likewise, there are three N–H bonds in NH3 and four C–H bonds in CH4. Each, one of these bonds requires a different amount of energy to break. In such cases,, average bond enthalpy is considered. It is defined as the average energy per, bond required to dissociate one mole of bonds in gaseous atoms., Total energy required to break, all the bonds of a particular type, in one mole of gaseous molecules, Average bond enthalpy =, Total no. of moles of bonds broken., For example in case of water molecule, Average bond enthalpy of O–H bond =, , 502 + 427, = 464.5 kJ mol–1, 2, , Some bond enthalpy values are given below:, , CHEMISTRY, , 109
Page 139 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Chemical Bonding, , Bond, , Bond Enthalpy/kJ mol–1, , Bond, , Bond Enthalpy/kJ mol–1, , H–H, , 436, , N≡N, , 946, , H–C, , 414, , O=O, , 498, , H–N, , 391, , C–C, , 347, , H–O, , 464, , C=C, , 611, , N–N, , 159, , C≡C, , 837, , O–O, , 138, , C≡N, , 891, , F–F, , 157, , C–Cl, , 330, , 4.5 HYDROGEN BONDING, It is a special type of attraction between a hydrogen atom bonded to a strongly, electronegative atom (like nitrogen, oxygen or fluorine) and the unshared pair of, electrons on another electronegative atom. Hydrogen bond is a weak bond, the, strength being just about 4-25 kJ mol–1. It is quite small as compared to the, covalent bond, which needs a few hundreds of kJ mol–1 of energy to break., However, it is strong enough to be responsible for the high boiling points of H2O, and HF etc. In fact it is due to hydrogen bonding only that water exists as a, liquid. The low density of ice also can be explained in terms of hydrogen bonding., , Due to the difference in the electronegativity between hydrogen and the other, electronegative atom, the bond connecting them becomes polar. The hydrogen, atom acquires a partial positive charge while the electronegative atom bears the, partial negative charge. Hydrogen bonding results from the electrostatic interaction, between the positively charged hydrogen atom and the negatively charged, electronegative atom. The second electronegative atom may be a part of the, same molecule or it may belong to a different molecule. Accordingly, there are, two types of hydrogen bonds. If the hydrogen bond is formed between two different, molecules it is called intermolecular hydrogen bond. When the hydrogen bond, exists within the same molecule, it is called intramolecular hydrogen bond., Salicyldehyde ad o-nitrophenol are two common examples of the molecules, showing intramolecular hydrogen bonding whereas in water, intermolecular, hydrogen bonding exists., , 110, , CHEMISTRY
Page 140 :
MODULE - 2, , Chemical Bonding, , H, , Atomic Structure and, Chemical Bonding, , H, O, , O, , O, , N, , O, C, H, , O, o-nitrophenol, , Salicyldehyde, , Hydrogen bonding plays an important role in the structure and function of many, biomolecules like proteins and nucleic acids., , 4.6, , Notes, , VALENCE SHELL ELECTRON PAIR REPULSION, (VSEPR) THEORY, , In a molecule the constituent atoms have definite positions relative to one another, i.e., the molecules have a definite shape. The theories of bonding that we have, discussed so far do not say anything about the shape of the molecules. A simple, theory called VSEPR theory was put forth by Sidgwick and Powell in 1940 to, explain the shapes of molecules. It was later refined and extended by Nyholm and, Gillespie in1957. This theory focuses on the electron pairs present in the valence, shell of the central atom of the molecule and can be stated in terms of two, postulates:, POSTULATE 1, The electron pairs (both bonding and non-bonding) around the central atom, in a molecule arrange themselves in space in such a way that they minimize, their mutual repulsion. In other words, the chemical bonds in the molecule will, be energetically most stable when they are as far apart from each other as possible., Let us take up some examples., BeCl2 is one of the simple triatomic molecules. In this molecule, the central, atom, beryllium has an electronic configuration of 1s2 2s2. That is it has two, electrons in its valence shell. In the process of covalent bond formation with two, chlorine atoms two more electrons are contributed (one by each chlorine atom), to the valence shell. Thus there are a total of 4 valence electrons or two pairs of, valence electrons. According to the postulate given above, these electron pairs, would try to keep as far away as possible. It makes the two electron pairs to be, at an angle of 180o which gives the molecule a linear shape., 180º, Cl, Cl, , CHEMISTRY, , Be, , Cl, , Be, , Cl, , linear molecule, , 111
Page 141 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Chemical Bonding, , Other molecules of this type would also have a similar shape., BF3 : In boron trifluoride, the central atom, boron has an electronic configuration, of 1s2 2s2 2p1. That is, it has three electrons in its valence shell. In the process of, covalent bond formation with three fluorine atoms three more electrons are, contributed (one by each fluorine atom ) to the valence shell. Thus there are a, total of 6 valence electrons or three pairs of valence electrons. According to the, VSEPR postulate, these electron pairs would try to keep as far apart as possible., It makes the three electron pairs to be located at an angle of 120o which gives, the molecule a planar trigonal shape., F, , B, F, , F, , Planar trigonal shape, , Thus different molecules would have different shapes depending on the number, of valence shell electrons involved. The geometric shapes associated with various, numbers of electron pairs surrounding the central atom are given in Table 4.1., Table 4.1: Geometric arrangements of electron pairs around central atom., Molecule, Type, , Number of, electron pairs, , Predicted, geometry, , AX2, , 2, , Linear, , AX3, , 3, , Planer, trigonal, , 4, , Tetrahedral, , AX5, , 5, , Trigonal, bipyramidal, , Examples, , 180º, X, , HgCl2, BeCl, BF3, BCl3, , A, , A, , CCl4, CH4, SiCl4, , :, , AX4, , Representative, structure, , 90°, , :, , :, , PCl5, PF5, , :, , 120°, , AX6, 112, , 6, , Octahedral, , A, , 90º, , SF6, PF6–, CHEMISTRY
Page 142 :
Chemical Bonding, , MODULE - 2, , POSTULATE 2, , Atomic Structure and, Chemical Bonding, , The repulsion of a lone pair of electrons for another lone pair is greater, than that between a bond pair and a lone pair which in turn is greater, than between two bond pairs. The order of repulsive force between different, possibilities is as under., lone pair - lone pair > lone pair - bond pair > bond pair - bond pair, The shapes of the molecules given in Table 4.1. correspond to the molecules, containing only bond pair electrons. The shapes of molecules containing a, combination of lone pairs and bond pairs would be distorted from the above, mentioned shapes., , Notes, , Let us take an example of three molecules namely, methane, ammonia and water., All the three contain a total of 4 electron pairs around their central atom. But the, nature of these is different in the three cases. In methane molecule the central, carbon atom has 4 valence electrons and it shares 4 electrons with four hydrogen, atoms. So there are a total of 4 bond pairs and according to Table 4.1 it should, have a tetrahedral shape. In case of ammonia also there are four pairs of electrons, but their nature is different. Three of these are bond pairs, while one is a lone pair., Similarly, in case of water again there are four pairs of electrons ; two are bond, pairs, while two are lone pairs. Due to the differences in the mutual repulsion, between bond pair - bond pair and lone pair - bond pair the molecular shape, would be slightly distorted from the expected tetrahedral shape. The number and, nature of electron pairs and the geometries of these three molecules are given in, Table 4.2., Table 4.2: Molecular geometries of molecules with 4 electron pairs, with different combinations of lone pairs and bond pairs., Molecule Number of, bond pairs, , Number of, lone pairs, , Molecular, geometry, , Molecular, Shape, , Bond angle, (in degrees), , H, CH4, , 4, , 0, , tetrahedral, , C, H, , H, H, , 109.5, , H, , 107.3, , .., .., , 104.5, , H, NH3, , 3, , 1, , trigonal pyramidal, , N, H, , .., H, , H2O, , 2, , 2, , angular or bent, , O, H, , We have so far learnt that a chemical bond formation between two atoms can, occur by transfer ( ionic bonding) or sharing (covalent bonding) of electrons., CHEMISTRY, , 113
Page 143 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Chemical Bonding, , The processes of bond formation and the bonding in simple molecules can be, conveniently represented in terms of electron dot structures. Further, the VSEPR, theory provides a good idea of the shapes of the molecules. But have you noticed, that we have been representing electrons as well defined dots i.e., localized, particles. This is in contradiction with the probabilistic (orbital) representation of, the electron that you have learnt in lesson 2. Let us learn how do we explain the, process of bond formation in terms of modern theories that incorporate the wave, mechanical representation of atom., , INTEXT QUESTION 4.2, 1. What are the basic postulates of VSEPR theory?, 2. Predict the shape of methane ( CH4 ) on the basis of VSEPR theory., 3. It is a molecule the difference between the electro-negativity of two atom, is 1.7. How much % will be ionic and covalent character?, , 4.7 MODERN THEORIES OF CHEMICAL BONDING, The theories of chemical bonding proposed (in 1916) by Kossel and Lewis are, known as classical theories of bonding. These do not take into account the wave, mechanical or quantum mechanical principles. After the development of quantum, mechanical description of atomic structure two more theories were proposed to, explain the bonding between atoms. These are called modern theories of chemical, bonding. These are Valence Bond Theory (VBT) and Molecular Orbital Theory, (MOT). Let us discuss these theories in brief., 4.7.1 Valence Bond Theory, , Potential Energy, , Valence bond theory was proposed, by Heitler and London in 1927, to, Distance between the hydrogen nuclei, describe the formation of hydrogen, molecule from its atoms. Linus, Pauling and others further developed, it. In this approach the process of, chemical bond formation can be, visualised as the overlapping of, atomic orbitals of the two atoms as, Bond Length, they approach each other. The, strength of the bond depends on the Fig. 4.2 : Formation of hydrogen molecule from, effectiveness or extent of the, overlapping of two hydrogen atoms, overlapping. Greater the overlapping, of the orbitals, stronger is the bond formed. Let us take the example of bonding, in hydrogen molecule to understand the VB approach., 114, , CHEMISTRY
Page 144 :
MODULE - 2, , Chemical Bonding, , Suppose that the two hydrogen atoms are at infinite distance from each other., Their electrons are in their respective 1s orbitals and are under the influence, of the corresponding nuclei. As the two atoms approach each other their 1s, orbitals begin to overlap which lead to decrease in energy, Fig. 4.1. At a distance, equal to the bond length the overlapping is maximum and the energy is minimum., The overlapping can be equated to the sharing of electrons between the atoms., The electrons occupying the shared region of orbitals are under the influence, of both the nuclei., , Atomic Structure and, Chemical Bonding, , Notes, , This simple approach can be used to explain the bonding in simple diatomic, molecules like HF,F2 etc.. However, to explain bonding in molecules containing, more than two atoms some additional concepts like excitation and hybridisation, need to be used., 4.7.1.1 Hybridisation, “Hybridisation is the process of forming new orbitals by mixing of atomic, orbitals in a particular atom. The new hybrid orbitals that are formed are all, equivalent orbitals and have the same energy. The phenomenon is know as, hybridisation”. Two main characteristics of hybridisation are:, (i) The number of hybrid orbitals formed is the same as the number of atomic, orbitals undergoing hybridisation., (ii) All the new hybrid orbitals that are formed are exactly identical in their, shapes and energy., Let us take up the example of bonding in a triatomic molecule; say, beryllium, hydride (BeH2) to understand the concept of hybridisation of orbitals and the, need for the same. The atomic number of beryllium is 4. Its electronic configuration, is 1s2 2s2. In order to form bonds with two hydrogen atoms the valence electrons, (2s2) of beryllium atom must overlap with the 1s electrons of the two hydrogen, atoms. Since the valence shell of beryllium atom contains both the electrons in, the same orbital (i.e., 2s) it cannot overlap with the 3p orbital of hydrogen atoms, containing one electron.[You know that an orbital can contain a maximum of, two electrons with opposite spin]. Pauling got over this problem by suggesting, that in the process of bond formation an electron from the 2s orbital of beryllium, atom gets momentarily excited to the empty 2p orbital as shown below., Excitation, Beryllium Atom, ( Ground state), 1s, , 2s, , 1s, , 2s, , 2p, , Beryllium Atom, ( Excited state), , CHEMISTRY, , 2p, , 115
Page 145 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Chemical Bonding, , Now the two valence electrons are in two singly occupied orbitals which can, overlap with the 1s orbitals of the two hydrogen atoms and form two bonds. The, problem is still not over. The two bonds formed by these overlaps would be of, different nature. One of these would involve overlapping of 2s orbital of beryllium, with 3p orbital of chlorine while the other would involve overlapping of 2p orbital, of beryllium with 2p orbital of chlorine. However, experimentally the two bonds, are found to be equivalent., This problem is solved with the help of a concept called hybridisation of orbitals., According to this two or more than two non equivalent orbitals (having different, energies and shapes ) of comparable energies mix or hybridize and give rise to an, equal number of equivalent ( same energies and shapes) hybrid orbitals., In case of BeCl2 the two singly occupied orbitals (2s and 2p) hybridize to give, two sp- hybrid orbitals. This is called sp hybridisation. These hybrid orbitals lie, along the z- direction and point in opposite directions., Hybridization, , Beryllium Atom, ( Excited state), 1s, , 2s, , Beryllium Atom, ( Hybridized ), 1s, , 2p, , sp, , 2p, , hybridized, orbitals, , These hybrid orbitals can now overlap with the 3p orbitals of chlorine atoms to, give the linear molecule of BeCl2. as shown below, Fig. 4.3 ., The concept of hybridisation as, illustrated above can be used to, describe the bonding and shapes, of different molecules by, considering hybridisation of, suitable orbitals. Let us take up, some more cases involving, hybridisation of s and p orbitals., , X, , X, Y, , 180º, , Y, Z, , s, , Z, , p, , + - - +, Linear, sp, , s, , s, Boron trichloride (sp 2, Z, +, +, +, +, hybridisation) : In boron there, Clpz, Clpz, Be, are five electrons and the, electronic configuration is 1s2,, Fig. 4.3 : Formation of BeCl2 ; sp, hybridisation, 2s2,2p1. There are three electrons, in the valence shell of boron, atom. In order to form bonds with three chlorine atoms one of the electrons from, the 2s orbital of boron atom is excited to its 2p orbital., , 116, , CHEMISTRY
Page 148 :
MODULE - 2, , Chemical Bonding, , Five sp3d hybrid orbitals are formed which are directed towards the corners of a, trigonal bipyramid (Fig. 4.5a). These orbitals overlap with singly filled p-orbitals, of five chlorine atoms and five σ bonds are formed. Thus PCl5 molecule has a, trigonal bipyramidal geometry. Three P–Cl bonds (equatorial) make an angle of, 120º with each other and lie in one plane. The other two P–Cl bonds (axial) are at, 90º to the equatorial plane, one lying above and the other lying below the plane., SF6 (sp3d2 hybridisation):, , Atomic Structure and, Chemical Bonding, , Notes, , s (ground state), s (excited state), sp3d2 hybridisation, , Six sp3d2 hybrid orbitals are formed which are directed towards the corners of a, regular octahedron. These orbitals overlap with singly filled orbitals of six F atoms, and form σ bonds giving a regular octahedral geometry (Fig. 4.5 b), F, Cl, , F, Cl, P, , F, S, , Cl, , F, , Cl, , F, , Cl, , F, Fig. 4.5 (a) : Trigonal bipyramidal geometry, of PCl5 molecule., , Fig. 4.5 (b) : Octahedral geometry of SF6, molecule, , 4.7.1.2 Hybridisation and Multiple Bonds, So far we have discussed the bonding in those molecules in which the orbitals on, a single central atom are hybridized. Let us see how does the concept of, hybridisation help us in understanding bonding between pairs of such atoms. In, the case of bonding in ethane (C2H6), two carbon atoms are bonded to each other, and each carbon atom is bonded to three hydrogen atoms. You would recall that, in the case of methane the valence orbitals of carbon atom undergo sp 3, hybridisation. In ethane each carbon atom undergoes sp3 hybridisation to give, four sp3 hybridized orbitals. The two carbon atoms form a carbon – carbon bond, by sp3 - sp3 overlapping. The remaining six sp3 hybridized orbitals overlap with, 1s orbitals of hydrogen atoms to give a molecule of ethane, C2H6.as shown in Fig., CHEMISTRY, , 119
Page 149 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Chemical Bonding, , 4.6. The C-C bond so formed is along, the internuclear axis. Such a bond is, called a ó bond., , 1s, 1s, , Notes, , sp3, , 1s, sp3, 1s, , sp3, sp3, Bonding in ethene: In case of ethene,, sp3, sp3 C, C, the relevant orbitals of the carbon, sp3, sp3, atoms undergo sp 2 hybridisation., Here, only two of the three p orbitals, 1s, 1s, of the carbon atoms hybridize with, Fig. 4.6 : Formation of ethane molecule, the 2s orbital to form three sp2 hybrid, orbitals each. The remaining porbitals (one on each carbon atom) do not take part in hybridisation. A carbon –, carbon bond is formed by overlapping of sp2 orbital on the two carbon atoms, [Fig. 4.7(a)]. The remaining four sp2 hybridized orbitals overlap with the 1s, orbitals of hydrogen atoms to give the basic skeleton of the molecule. This leaves, an un-hybridized p orbital each on both the carbon atoms[Fig. 4.7(b)]. These are, perpendicular to the molecular plane and undergo sideways overlap to give an, electron cloud in the plane above and below the molecule [Fig. 4.7(b and c)]., This is called a π- bond. In ethene there are two bonds between the carbon atoms, ( one sigma and one pi bond)., , Fig. 4.7 : Formation of ethylene molecule: (a) formation of the basic skeleton of the, molecule (b) sideways overlapping of the unhybridized p orbitals and (c) a π- bond (d) and, (e) complete picture of ethylene molecule., , 120, , CHEMISTRY
Page 150 :
MODULE - 2, , Chemical Bonding, , Bonding in ethyne ( acetylene): In case of acetylene the bonding can be explained, in terms of sp-hybridisation in carbon atoms. One 2s and one 2p orbitals hybridize, to give two sp-hybridized orbitals. This leaves two mutually perpendicular, unhybridised p orbitals each on both the carbon atoms. The carbon – carbon, bond is formed by sp - sp overlapping with each other. The remaining sp orbital, on each carbon overlaps with the 1s orbital of hydrogen to give C-H bonds (Fig., 4.8). The unhybridised p orbitals each on both the carbon atoms overlap sideways, to give two π-bonds., , Atomic Structure and, Chemical Bonding, , Notes, , Fig. 4.8 : Formation of acetylene molecule : (a) formation of the basic skeleton of the, molecule (b) sideways overlapping of two pairs of un-hybridized p orbitals and (c) two, mutually perpendicular π- bonds., , 4.7.1.3 Resonance, Some times it is possible to write more than one Lewis structure of a compound, that agree with the electronic requirements. For example, we can write two, Lewis structures for ozone molecules, O3., O, O, , O, , or, O, , O, , O, , Both of these structures satisfy the octet rule and have a double bond on one, side and single bond on the other side of the central oxygen. According to these, structures one oxygen-oxygen bond (O=O) would be shorter than the other (O–, O). However, this is not the case. Experimentally both the oxygen-oxygen bonds, are found to have the same bond length (128 pm) which is in between the bond, lengths of a (O=O) double bond (121 pm) and a (O–O) single bond (148 pm)., These experimental observations can be explained with the help of the concept, of resonance. The alternate Lewis structures are called canonical structures., These are separated by a double headed arrow(↔) All canonical structures must, have similar positions of nuclei, similar number of bonding and non-bonding, electrons and similar energy. The actual structure is the resonance hybrid of, all the contributing canonical or resonating structures. The structure of O3, molecule is represented as, , CHEMISTRY, , 121
Page 151 :
MODULE - 2, , Chemical Bonding, , Atomic Structure and, Chemical Bonding, , O, O, , O, , Notes, , O, , ¬¾®, , O, , O, , Resonance does not mean that the molecule constantly changes from one, canonical structure to the other. The structure is stable and the bond lengths, and energies have values which are intermediate between those of single and, double bonds. The concept of the resonance is necessary because of the, limitations in the way we write the structures. Some times the structure of a, resonance hybrid is shown with help of representation of bonds by broken lines, or dashes. The structure of resonance hybrid of O3 is shown below:, O, , O, , ¬¾®, , O, , O, , O, , O, , O, , or, , O, , O, , In molecules or ions containing more than two atoms and having double bonds, π) bonds. The electrons involved in π-bonds are, or triple bonds, there are pi (π, not localized between two atoms, but are spread over the entire structure (or, the portion of the structure) which has only sigma-(σ) bonds. This spreading, of π-electrons is known as the delocalization of the electrons. Broken lines or, dashes depict the region of delocalization of the π-electrons. Delocalization, results in stabilizing the structure. Therefore, the resonance hybrid is more stable, than the contributing canonical structures., Some examples of resonance structures:, , (, , 2–, (1) Carbonate ion CO 3, , ), , The three possible canonical structures of carbonate ion are, O, , O, ¬¾®, , C, –, , O, , O, , –, , –, , O, ¬¾®, , C, O, , O, , –, , –, , C, –, , O, , O, , (2) Sulphur dioxide (SO2), The two canonical structures of sulphur dioxide are shown below, S, O, , S, , ¬¾®, , O, , O, , O, , (3) Dinitrogen oxide (N2O), The three canonical structure of dinitrogen oxide are shown below, N, , 122, , N, , O, , ¬¾®, , N, , N, , O, , ¬¾®, , N, , N, , O, , CHEMISTRY
Page 152 :
MODULE - 2, , Chemical Bonding, , Atomic Structure and, Chemical Bonding, , INTEXT QUESTION 4.3, 1. What do you understand by the term, ‘hybridisation’?, 2. How would you explain the shape of ammonia molecule on the basis of, hybridisation?, 3. Draw the canonical structures of CO23 and SO2., , Notes, , 4.7.2 Molecular Orbital Theory, You have just learnt about valence bond theory. It describes bond formation as a, result of overlapping of the atomic orbitals belonging to the constituent atoms., The overlapping region responsible for bonding is situated between the two atoms, i.e., it is localised. Molecular orbital theory (MOT) was developed by F.Hund, and R.S.Mulliken in 1932. In contrast to the localized bonding in VBT, the, molecular orbital theory visualises the bonding to be delocalised in nature i.e.,, spread over the whole molecule. According to MOT, in the process of bond, formation, z, , The atomic orbitals of the constituent atoms combine to generate new, types of orbitals (called molecular orbitals). These are spread over the, whole molecule i.e., they are delocalised. In other words these new orbitals,, do not “belong” to any one atom but extend over the entire region of the, bonded atoms., , z, , These molecular orbitals are created by Linear Combination of Atomic, Orbitals (LCAO) approach in which, the atomic orbitals of comparable, energies and of suitable symmetry combine to give rise to an equal, number of molecular orbitals., , z, , The available electrons then fill these orbitals in the order of increasing, energy as in the Aufbau principle used in the electron configurations of, atoms., Molecular orbital, +, , Energy, , Atomic, orbital, , s1s, , 1s, , Atomic, orbital, , –, , 1s, atomic, orbital, , +, , 1s, atomic, orbital, , 1s, s1s, , +, , 1s, atomic, orbital, , +, , +, , +, , s*1s, antibonding sigma, molecular orbital, +, , +, , 1s, atomic, orbital, , s1s, bonding sigma, molecular orbital, , Fig. 4.9 : Formation of bonding (σ) and anti bonding (σ*) molecular orbitals, CHEMISTRY, , 123
Page 153 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Chemical Bonding, , Let us take the example of hydrogen molecule to understand the molecular orbital, approach to chemical bonding. The two hydrogen atoms have one electron each, in their respective 1s orbitals. In the process of bond formation the atomic orbitals, of two hydrogen atoms can combine in two possible ways. In one, the MO, wavefunction is obtained by addition of the two atomic wave functions whereas, in the other the MO is obtained by subtraction of the wave function. The, combination of the 1s orbitals on the two hydrogen atoms are shown in Fig. 4.9., The molecular orbital obtained by the addition of atomic orbitals is of lower, energy than that of the atomic orbitals and is called a bonding orbital. On the, other hand, the orbital obtained by subtraction of atomic orbitals is of higher, energy and is called an anti-bonding orbital. You can note here that the molecular, orbitals obtained here are symmetric around the bond axis (the line joining the, two nuclei). Such molecular orbitals are called sigma (σ) molecular orbitals., The bonding orbital obtained above is denoted as σ1s while the anti- bonding, orbital is denoted as σ*1s. Here σ indicates the type of molecular orbital; 1s tells, about the atomic orbital involved and * is indicative of the anti-bonding nature of, the MO. There are a total of 2 electrons in a hydrogen molecule; according to, Aufbau principle these are filled into σ1s orbital. Since the σ1s orbital is a bonding, orbital, its getting filled leads to stability or the bond formation., Like electronic configuration of atoms we write MO electronic configuration for, molecules. The MO configuration of hydrogen molecule is given as (σ1s)2. The, molecular orbital energy level diagram is given in Fig. 4.10(a)., Antibonding, MO, E, N, E, R, G, Y, , s*1s, Atomic, orbitals, 1s, , 1s, , s 1s2, , Bonding, MO, , (a), , Antibonding, MO, E, N, E, R, G, Y, , s* 1s2, Atomic, orbitals, 1s, , 1s, , s 1s2, , Bonding, MO, , (b), , Fig. 4.10 : Molecular orbital energy level diagram for a) H2 and b) He2 molecules, , Bond Order: we may define a new parameter called bond order as, Bond order = (b.o.) = ½(nb - na), Where, nb and na refer to the number of electrons present in bonding and, antibonding molecular orbitals, respectively. For hydrogen molecule the bond, order will be ½(2–0) = 1, i.e., there is a single bond between two hydrogen, atoms., 124, , CHEMISTRY
Page 154 :
MODULE - 2, , Chemical Bonding, , Helium (He2) molecule, , Atomic Structure and, Chemical Bonding, , In case of He2 also, there will be linear combination of 1s orbitals leading to the, formation of σ1s and σ*1s orbitals. The four electrons would be distributed as per, the MO electronic configuration : (σ1s)2 (σ*1s)2 . The molecular orbital, energy level diagram is given in Fig. 4.10 (b) .This gives a bond order of ½(2–2), = 0, that is there is no bond between two helium atoms. In other words He2, molecule does not exist., , Notes, , Li2 and Be2 molecules, The bonding in Li2 and Be2 can be explained by combining the 1s and 2s orbitals, to give appropriate MO’s. The molecular orbital diagrams for Li2 and Be2 are, given in Fig. 4.11., , s *2s, , s *2s, , 2s, E, N, E, R, G, Y, , 2s, , 2s, , 2s, , s 2s, , s 2s, , s *1s, , s *1s, , 1s, , 1s, , s 1s, , (a), , 1s, , 1s, , s 1s, , (b), , Fig. 4.11: Molecular orbital energy level diagram for a) Li2 and b) Be2 molecules, , 4.7.2.1 Molecular Orbital Bonding in Diatomic Molecules of Second, Period, So far we have talked about bonding in the elements in which the MO’s were, obtained from the linear combination of s orbitals. In case of the atoms of second, period (beyond Be) elements both s and p orbitals are involved in the formation, of molecular orbitals. In such a case a number of different molecular orbitals are, obtained depending on the type and symmetry of the atomic orbitals involved in, CHEMISTRY, , 125
Page 155 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Chemical Bonding, , the process. Let us try to understand the nature of MO’s obtained in this case., Here also the 1s and 2s orbitals of the two atoms would combine to give, corresponding bonding and anti-bonding molecular orbital as shown in Fig. 4.11, (b). Let us learn about the formation of MO’s from the combination of p orbitals, , Notes, , As mentioned above, in LCAO, the atomic orbitals of comparable energies and, of suitable symmetry combine to give molecular orbitals. A suitable symmetry, means that the combining orbitals should have same symmetry about the molecular, axis. It is nomally assumed that the bond formation takes place along the zdirection. You have learnt in the first unit that the three p orbitals are directed, towards three mutually perpendicular directions namely the x, y and z directions., Therefore the pz orbitals of the two atoms would combine along the bond axis to, give two molecular orbitals as shown below Fig. 4.12. Since these molecular, orbitals are symmetric around the molecular axis these are called σ orbitals. The, designation of the orbitals would be σ2pz and σ*2pz ., , (a), , (b), , Fig. 4.12: Overlapping of two 2pz orbitals to give molecular orbitals, , Combination of a pz -orbital with either a px or a py orbital would not lead to, any bonding. On the other hand a px orbital will combine with a px and the py, with a py as shown in Fig. 4.13., , Fig. 4.13 : Formation of molecular orbitals from two 2px atomic orbtials., , 126, , CHEMISTRY
Page 156 :
MODULE - 2, , Chemical Bonding, , You may note here that these orbitals combine in a lateral fashion and the resulting, molecular orbitals are not symmetric around the bond axis. These MO’s are called, π- molecular orbitals. These have large electron density above and below the, internuclear axis. The anti-bonding π orbital, π* 2px (or π* 2py) have a node (a, region of zero electron density) between the nuclei., The molecular orbitals obtained as a result of combination of respective AO’s of, two atoms can be represented in the form of following energy level diagram,, Fig. 4.14(a) . The MO’s obtained from the combination of 1s orbitals are not, shown.(these belong to the inner core and are completely filled ) The electrons in, these molecular orbitals are filled in accordance with Aufbau principle and Hund’s, rule., , s* 2pz, , s* 2pz, , Atomic Orbitals, , Atomic Orbitals, , 2py, , 2pz, , Atomic Orbitals, , Atomic Orbitals, , p * 2py, , 2py, , p 2py, , 2pz, E, N, E, R, G, Y, , 2py, , 2pz, , 2pz, , s* 2s, , 2s, , (a), , 2py, , p 2py, , p 2px, , s* 2s, , s 2s, , 2px, , 2px, , s 2pz, , s 2pz, , 2s, , p * 2py, , p * 2px, , 2px, , 2px, p 2px, , Notes, , Molecular Orbitals, , Molecular Orbitals, , p * 2px, , Atomic Structure and, Chemical Bonding, , 2s, , 2s, , s 2s, , (b), , Fig. 4.14: Molecular orbital energy level diagrams a) for O2 and F2 and b) for diatomic, molecules of lighter elements Li, Be, B, C and N, , However, this energy level diagram is valid for the diatomic molecules O2 and F2, only; For the diatomic molecules of the lighter elements like, B, C and N this, energy level diagram is somewhat modified. It is so because in case of lighter, elements the difference in the energy of 2s and 2p orbitals is very low and s and p, orbitals on the two atoms get mixed up. In place of normal pure 2s-2s or 2p-2p, combinations we may have s-p combinations; for example 2s orbital of first atom, can have a reasonable overlapping with 2pz, orbital of the second atom and vice, versa. The modified energy level diagram is given in Fig. 4.14 (b)., 4.7.2.2 MO Electronic Configuration and Properties of a Molecule, The MO energy level diagram discussed above can be used to find out the MO, electronic configuration of a molecule. This in turn provides the information about, CHEMISTRY, , 127
Page 157 :
MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , Chemical Bonding, , some properties of the molecule. Let us take the example of nitrogen molecule., An atom of nitrogen has five valence electrons; since there are two atoms, we, have a total of ten valence electrons that need to be filled in the MO’s. Using Fig., 4.14 , the MO electronic configuration can be written as σ2s2, σ*2s2, π 2px2, π, 2py2 , σ2pz2, Bond order : ½ [nb−na] = ½ [8−2] = ½ [6 ]= 3 ; this means that in nitrogen, molecule, a triple bond exists between the two nitrogen atoms., Magnetic nature: molecules show magnetic behaviour depending on their MO, electronic configuration. If all the MO’s are doubly occupied the substance shows, diamagnetic behaviour. In case one or more MO’s are singly occupied, the, substance shows paramagnetic behaviour. The MO electronic configuration of, O2 (with 12 valence electrons) is σ2s2, σ*2s2, σ2pz2, , π 2px2 , π 2py2, π* 2px1 , π*, 2py1 ; Since it contains unpaired electrons, oxygen shows paramagnetic behaviour., This has been found to be so experimentally also. In fact, the explanation of the, paramagnetic behaviour of oxygen is an achievement of MOT., The bond order and the magnetic behaviour of the diatomic molecular cations, and anions can also be obtained in the same way. In such cases we add one, electron for every negative charge and for every +ve charge we subtract an electron., For example, O22−( oxygen molecule dianion) would have a total of 14 valence, electrons ( 12 + 2) while oxygen molecule cation O2+ would have 12−1 = 11, valence electrons., , INTEXT QUESTION 4.4, 1. What is the basic difference between the valence bond and molecular orbital, theories?, 2. Calculate the bond orders for Li2 and Be2 molecules using the molecular, orbital diagrams given in Fig. 4.12., 3. Predict the magnetic behaviour of O2., , WHAT YOU HAVE LEARNT, z, z, z, , 128, , A chemical bond may be visualised as an effect that leads to the decrease in, the energy of the combination of two atoms when they come closer., The atoms combine in such a way so as to attain stable electronic, configuration of noble gases., According to Kossel, transfer of an electron from one atom to the other, achieves the stable configuration. This leads to formation of ions, which are, held together by electrostatic interactions called ionic bond., CHEMISTRY
Page 158 :
Chemical Bonding, z, , According to Lewis, the stable configuration is achieved by sharing of electron, pairs between the bonding atoms. This leads to the formation of a covalent, bond., , z, , Bonding in simple molecules can be conveniently represented in terms of, Lewis electron-dot structures., In some covalently bound atoms the shared pair of electron is more towards, the atom with greater electronegativity and leads to partial ionic character in, the molecule., , z, , z, , Valence shell electron pair repulsion (VSEPR) theory is very helpful in, predicting the shapes of simple molecules. It is based on the interactions, between the electron pairs around the central atom in the molecule., , z, , Valence bond theory (VBT) and Molecular orbital theory (MOT) are two, modern theories of chemical bonding. These are based on the wave, mechanical model of atom., , z, , According to the valence bond theory the process of chemical bond formation, can be visualised as the overlapping of atomic orbitals of the two atoms as, they approach each other. The overlap increases the electron charge density, in the inter-nuclear region., , z, , In order to explain bonding in molecules containing more than two atoms,, Pauling proposed the concept of hybridisation. In hybridisation, the atomic, orbitals of the valence shell of the central atom ‘ hybridise’ or merge and give, newer orbitals with proper orientations, which explain the shape of the, molecule., , z, , According to the Molecular orbital theory the atomic orbitals of comparable, energies and of suitable symmetry combine to give rise to an equal number, of molecular orbitals. These molecular orbitals extend over the entire region, of the molecule i.e., these are delocalised over the whole molecule., , z, , When two atomic orbitals combine it gives a pair of molecular orbitals; one, is called bonding molecular orbital of lower energy and the other of higher, energy is called anti-bonding orbital., , z, , The electrons present in the molecule are filled in these orbitals in the order, of increasing energy (Aufbau principle) to give the MO electronic, configuration., , z, , The number of bonds between the two atoms is called bond order and is, defined as Bond order = b.o. = ½(nb - na), , z, , The MO electronic configuration can be used to predict the magnetic nature, of the molecule. If all the MO’s are doubly occupied the substance shows, diamagnetic behaviour and if one or more MO’s are singly occupied the, substance shows paramagnetic behaviour., , CHEMISTRY, , MODULE - 2, Atomic Structure and, Chemical Bonding, , Notes, , 129
Page 159 :
MODULE - 2, , Chemical Bonding, , Atomic Structure and, Chemical Bonding, , TERMINAL EXERCISE, , Notes, , 1., , What do you understand by a chemical bond?, , 2., , Explain the process of bond formation as a decrease in energy., , 3., , What do you understand by the term, ‘bond length’ ?, , 4., , Describe the two possible ways in which the noble gas electronic, configuration is achieved in the process of bond formation., , 5., , What are Lewis electron dot symbols ? Show the formation of MgCl2 in, terms of Lewis symbols., , 6., , Define a coordinate bond and give some examples., , 7., , What is VSEPR theory ? predict the shape of SF6 molecule using this theory., , 8., , Why do we need the concept of hybridisation ? How does it help in explaining, the shape of methane ?, , 9., , Give the salient features of molecular orbital theory., , 10. Be2 molecule does not exist. Explain on the basis of molecular orbital theory., 11. Write down the molecular orbital electronic configuration of the following, species and compute their bond orders., O2 ; O2+ ; O2− ; O2 2−, 12. BF3 is a polar molecule but it does not show dipole moment. Why?, 13. Atom A and B combine to form AB molecule. If the difference in the, electronegativity between A and B is 1.7. What type of bond do you expect, in AB molecule?, 14. Write down the resonating structures of N2O, SO42–, CO32– and BF3., , ANSWERS TO INTEXT QUESTIONS, 4.1, 1., , 130, , An electrovalent bond is formed when one or more electrons are transferred, from one atom to another atom or atoms., , CHEMISTRY
Page 160 :
MODULE - 2, , Chemical Bonding, , 2., , ., ., : N. . + . N. :, , .., : N :: N :, , Atomic Structure and, Chemical Bonding, , :N, , N:, , 3., , In a covalent bond the shared pair of electrons is closer to the more, electronegative atom. This leads to charge separation in the molecule and, the bond becomes polar, , 4., , A bond in which both the bonding electrons are contributed by one atom, only., , Notes, , 4.2, 1., , The two postulates of VSEPR theory are, i) The electron pairs (both bonding and non-bonding) around the central, atom in a molecule arrange themselves in space in such a way that, they minimize their mutual repulsion, ii) The repulsion of a lone pair of electrons for another lone pair is greater, than that between a bond pair and a lone pair which in turn is stronger, than that between two bond pairs The order of repulsive force between, different possibilities is as under., lone pair - lone pair > lone pair - bond pair > bond pair - bond pair, , 2., , In methane the central carbon atom would have four pairs of electrons in its, valence shell. According to VSEPR theory these would be placed, tetrahedrally around the carbon atom. Hence the methane molecule would, have a tetrahedral shape., , 3., , 50% ionic and 50% covalent character., , 4.3, 1., , Hybridisation is a concept which is quite useful in explaining the shapes of, molecules. According to this two or more than two non equivalent orbitals, with comparable energies and different shapes mix and give rise to an equal, number of equivalent hybrid orbitals in an atom. The hybrid orbitals have, identical energies and shapes., , 2., , In ammonia the 2s and three 2p orbitals hybridize to give four sp3 hybridized, orbitals. Three of these overlap with the 1s orbitals of hydrogen and one, remains nonbonding containing a lone pair. The sp3 hybridized orbitals are, directed towards the corners of a regular tetrahedron. But due to the, difference in the repulsion between lone pair - bond pair and bond pair bond pair the ammonia molecule has a distorted tetrahedral shape which is, some what like a trigonal pyramid., , CHEMISTRY, , 131
Page 162 :
MODULE - III, STATES OF MATTER, 5. The Gaseous and Liquid State, 6. The Solid State, 7. Solutions, 8. Colloids
Page 164 :
MODULE - 3, , The Gaseous and Liquid State, , States of Matter, , 5, Notes, , THE GASEOUS AND LIQUID, STATE, , We know that matter exist in three* different states namely solid, liquid and gas., The most familiar example to show these different states of matter is water. Water, exists as a solid (ice), a liquid (water) and a gas (steam) under different conditions, of temperture and pressure. The difference between these three states of matter, is due to the difference in their intermolecular distances and intermolecular forces., In addition to these, temperture and pressure also play an important role in deciding, the states of matter., In this lesson we shall first discuss the differences in properties of the solid, liquid, and gaseous state and the factors due to which these differences arise. We shall, also study the effect of pressure and temperature on the volume of the given, amount of gas. These are governed by the gas laws namely Boyles’ law, Charles’, law and Avogadros’ law., You are familiar with gases, liquids and solids in your daily life. You are aware, that water can exist as a liquid, a solid (ice) or as a gas (vapour). These are called, three states of matter. The properties of gaseous state can be explained in terms, of large separation of molecules and very weak intermolecular forces. In this, lesson, we shall also study about the intermolecular forces in liquids and see how, their properties can be explained in terms of these forces., , OBJECTIVES, After reading this lesson, you will be able to:, z, , differentiate between the three states of matter - solid, liquid and gas;, , * There is fourth state of matter called Plasma. In this state matter exists as ionised gas. This, occur at very high temperature. For example matter in the sun is in the Plasma State., CHEMISTRY, , 135
Page 165 :
MODULE - 3, States of Matter, , Notes, , The Gaseous and Liquid State, z, , describe various intermolecular interactions;, , z, , list the characteristic properties of gases;, , z, , state the gas laws (Boyle’s law, Charle’s law, Gay Lussac's law and Avogadro’s, law) and express these mathematically;, , z, , draw the p-V, p-1/V, p-pV and V-T graphs;, , z, , interpret the effect of temperature and pressure on the volume of a gas from, the graph;, , z, , derive the ideal gas equation from the gas laws;, , z, , state the Dalton’s law of partial pressure and explain its significance;, , z, , state Graham’s law of diffusion;, , z, , explain the role of Gay Lussac's law of combining volumes and Avogtadso's, law in developing the concept of molecule;, , z, , state the postulates of Kinetic Molecular Theory of gases;, , z, , explain the Maxwell’s distribution of velocities; and effect of temperature, and molar mass of gas on it., , z, , differentiate between urms , ump and uav ;, , z, , explain the deviation of real gases from ideal behaviour in term of, compressibility factor;, , z, , state the van der Waal's equation and explain the significance of van der Waals, constants and, , z, , explain the liquifaction of gases with the help of Andrews curves., , z, , explain the properties of liquids in terms of their structure (molecular, arrangement and intermolecular forces);, , z, , differentiate between evaporation and boiling;, , z, , define vapour pressure of a liquid and correlate it with its boiling point;, , z, , define surface tension and explain the effect of various factors on it;, , z, , explain the consequences of surface tension and, , z, , define viscosity of a liquid and correlate it with intermolecular forces., , 5.1 THE THREE STATES OF MATTER, At any given conditions of temperature and pressure, matter exists in one of the, three states namely solid, liquid and gas. The characteristic properties of solid,, liquid and gaseous state are listed in Table 5.1., 136, , CHEMISTRY
Page 166 :
MODULE - 3, , The Gaseous and Liquid State, , States of Matter, , Table 5.1 : Properties of different states of matter, Property, , Solid, , Liquid, , Gas, , Shape, , Definite, , Indefinite; takes up the, shape of the vessel., , Indefinite, , Volume, , Definite, , Definite, , Density, , High, , Less than solids but, much higher than gases., , Indefinite (fills the, container completely), Low, , Compressibility, , Incompressible, , largely incompressible, , Notes, , Highly compressible, , The different characteristics of the three states of matter as listed above depend, upon the relative closeness of particles that make up the substance. In solid state,, the particles are held close together in a regular pattern by strong intermolecular, forces. In liquid state, intermolecular forces are weak as compared to solid state, hence the particles are less tightly held and allow them to move away from each, other. In the gaseous state, the molecules are farthest apart as compared to solid, and liquid states and the intermolecular forces are negligible so the particles move, randomly. A simplified picture of particles in solid, liquid and gaseous states is, represented in Fig. 5.1., , Solid, , Liquid, , Gas, , (a), , (b), , (c), , Fig. 5.1 : A simplified picture of particles in solid, liquid and gaseous state, , 5.2 INTERMOLECULAR INTERACTIONS, Intermolecular interactions are the interactions or forces that operate between, the constituent particles of a substance, which may be atoms, molecules or ions., These forces are very weak as compared to intramolecular forces–the forces, that operate within a molecule i.e. covalent and ionic bonds. These forces,, though weak, are responsible for the existence of condensed phases, namely,, liquids and solids., 5.2.1 Types of Intermolecular Interactions, There are four types of intermolecular interactions or forces: (i) London forces;, (ii) Dipole – dipole forces; (iii) Dipole – induced dipole forces and (iv) Hydrogen, CHEMISTRY, , 137
Page 167 :
MODULE - 3, States of Matter, , The Gaseous and Liquid State, , bonding. Out of these four, the first three are collectively called van der Waals, forces. Hydrogen bonding is a type of strong dipole – dipole forces and is not, included in van der Waals forces. Let us now learn about these forces., (i) London forces., , Notes, , Normally we assume that electrons are evenly distributed around nucleus (Fig., 5.2(a)). However, since electrons constantly move around the nucleus, at a, particular instant, the distribution of electrons may become uneven around the, nucleus (Fig. 5.2 (b)) and result in generation of a dipole with a slight negative, charge on one side and an equal positive charge on the opposite side. Thus,, an ordinarily non-polar species like an atom of a noble gas like argon or neon, becomes a polar species for an instant. This instantaneous dipole would induce, dipoles in its neighbours. (Fig 5.3). These dipoles attract each other as their, oppositely charged poles are near each other. The very next instant of time, this, dipole disappears as the electronic distribution once again becomes symmetrical, in this particular atom or molecule. With this, all the induced dipoles also, disappear. However, a new instantaneous dipole appears in some other atom, or molecule which induces dipoles in its neighbours., , d+ +, , +, , d–, , Figure 5.2: Development of instantaneous dipole, , d+ +, , d–, , Induced, depole, , d+ +, , d–, , Instantaneous, dipole, , d+ +, , d–, , Induced, dipole, , Fig. 5.3: Induced dipoles in neighbours of an atom or a molecule, with instantaneous dipole., , This phenomenon continues for ever. At any given instant, a large number of, such atoms or molecules distributed randomly develop instantaneous dipoles, which induce dipoles in their neighbours. This results in the presence of weak, interactions in the substance., These forces are named after the Germany born physicist Fritz London. London, forces are the weakest of all intermolecular forces and operate at extremely short, distances. These are also called dispersion forces. These are the only forces, that are present in atoms or non-polar molecules and are responsible for the, existence of their condensed phases. These forces are present universally in all, types of molecules, polar or non-polar and contribute majorly toward, intermolecular forces present in them., 138, , CHEMISTRY
Page 168 :
MODULE - 3, , The Gaseous and Liquid State, , States of Matter, , (ii) Dipole – Dipole Forces, If molecules of a substance are polar in nature, the positive (δ+) end of one dipole, attracts the negative (δ–) end of the other (Fig. 5.4) Magnitude of these forces, depends upon the dipole moment of the substance. These interactions are quite, strong as compared to London forces and increase the melting and boiling points, of polar compounds (such as ethers) as compared to those of similar non-polar, compounds (such as hydrocarbons) that have nearly the same molar mass, d+, , d–, , d+, , Notes, , d–, , Fig. 5.4: Dipole-dipole forces, , Compound, , Molar Mass Melting, /(g mol–1), Point/K, , Boiling, point/K, , n-Butane (non-polar) C4H10, , 58, , 135.2, , 272.6, , Propanone (polar), , 58, , 179.4, , 329.3, , (CH3)2C = O, , In addition to the dipole-dipole forces, London or dispersion forces also operate, between polar molecules., (iii) Dipole – Induced Dipole Forces, This type of forces operate in mixtures (solutions) of two substances, out of, which one is polar in nature and the other is non-polar. The polar molecules, induce a dipole in the neighbouring non-polar molecules and an electrostatic, force of attraction starts operating between them (Fig 5.5). Magnitude of these, forces depend upon two factors, , d–, , d+, (a), , d–, , d+, , d–, , d+, , (b), , Fig. 5.5 Dipole-induced dipole forces: (a) A pair of polar and a non-polar molecules, (b) Induced dipole in the non-polar molecule, , (a) Dipole moment of the polar molecule. Greater the dipole moment, stronger, is the attractive force., , CHEMISTRY, , 139
Page 169 :
MODULE - 3, States of Matter, , The Gaseous and Liquid State, , (b) Polarizability of the non-polar molecule. More polarizable is the non-polar, molecule, greater is the dipole moment of the induced dipole and stronger, is the attractive force., Dispersion or London forces also operate between polar and non-polar, molecules and they experience the cumulative effect of both types of forces., , Notes, , (iv) Hydrogen Bonding, Hydrogen boding is responsible for abnormally high melting and boiling points, of water, ethanol, ammonia, hydrogen fluoride etc. The following table gives, the boiling points of hydrides of group 14, 15, 16, and 17 elements. In each, group the boiling point of hydrides is increasing with molar mass from top to, bottom. The trend is clearly seen in hydrides of group 14. In other groups, the, trend can be seen in all the hydrides, except that of the first element in each, group i.e. NH3 (Group 15), H2O(Group 16) and HF (Group 17) respectively., Their boiling points are exceptionally high due to the presence of hydrogen, bonding in these hydrides., Boiling Points of some Hydrides, Group 14, , Group 15, , Group 16, , Group 17, , Hydride B. P./K Hydride B.P./K Hydride B.P./K Hydride B.P./K, CH4, , 109, , NH3, , 240, , H2O, , 373, , HF, , 293, , SiH4, , 161, , PH3, , 183, , H2S, , 212, , HCl, , 188, , GeH4, , 183, , AsH3, , 218, , H2Se, , 232, , HBr, , 207, , SnH4, , 221, , SbH3, , 255, , H2Te, , 271, , HI, , 238, , Hydrogen bonding is responsible for some unusual properties of water, like its, existence in liquid state over a wide range of one hundred degrees (273 K to, 373 K), high heats of fusion and vaporization and its solid form (ice) being lighter, than liquid water. Hydrogen bonding plays a crucial role in the structure of DNA, by holding together the two helical nucleic acid chains., , 5.3 GENERAL BEHAVIOUR OF GASES: THE GAS LAWS, The volume of a given mass of a gas depends upon the temperature and pressure, under which the gas exists. It is, therefore, possible to describe the behaviour of, gases in terms of the four variables: temperature, T ; pressure p; volume V and amount, (number of moles, n). For a given amount of gas the volume of gas changes with, change in variables such as temperture and pressure. The relationship between, any two of the variables is studied, keeping the other variable constant by various, laws which are described below., 140, , CHEMISTRY
Page 170 :
MODULE - 3, , The Gaseous and Liquid State, , States of Matter, , 5.3.1 Effect of Pressure on the Volume of the Gas (Boyle’s law), The effects of pressure on the volume of gas for a given amount of gas at constant, temperture was studied by Robert Boyle in 1662 for different gases. He observed, that if the volume of gas is doubled the pressure is halved and vice versa. Boyle’s, law states that at constant temperature, the volume of a given amount of a gas is, inversely proportional to its pressure., , Notes, , Mathematically Boyle’s law is expressed as shown below:, 1, , V ∝ p, or, , (at constant T and n), , p1 V1 = p2V2, , when the pressure of the gas, p is plotted against volume of the gas, V the, exponential curve is obtained (Fig. 5.6). However when the pressure, p of the gas, 1, is plotted against a straight line is obtained (Fig. 5.7). If the product of pressure, V, and volume (pV) is plotted against pressure (p) a straight line parellel to x-axis, (pressure is axis) is obtained (Fig. 5.8)., T n = contant, , T, n = contant, , p®, , p®, , 1, , v®, Fig. 5.6 : A graph of p versues V, , ⎛1⎞, ⎟, ⎝V ⎠, , Fig. 5.7 : A graph of p versues ⎜, , T n = contant, pV, , 1, , Pressure (p), Fig. 5.8 : A graph of pV versues p, CHEMISTRY, , 141
Page 171 :
MODULE - 3, States of Matter, , The Gaseous and Liquid State, , Example 5.1 : The volume occupied by a given mass of a gas at 298 K is 24 mL, at 1 atmospheric pressure. Calculate the volume of the gas if the pressure is, increased to 1.25 atmosphere keeping temperature constant., Solution : Given that, , Notes, , V1 = 25 mL, , p1 = 1 atm, , V2 = ?, , p2 = 1.25 atm, , According to Boyle’s Law, p1V1 = p2 V2, substituting the values of p1, V1 and p2 in the above expression we get, V2 =, , p1 V1, (1 atm) (25mL), =, = 20 mL, (1.25 atm), p2, , The volume occupied by the gas is 20 mL at 298 K and 1.25 atm pressure., Example 5.2 : The volume of a certain amount of a gas is decreased to one fifth, of its initial volume at a constant temperature. What is the final pressure?, Solution : Let, Initial volume = V1, , Initial pressure = p1, , Final volume V2 = V1 /5, , Final pressure = p2, , By Boyle’s law, we know that at constant temperature, p1V1 = p2 V2, p1 V1, , p2 = V, 2, Thus when volume is decreased to, , =, , p1 × V1, = 5p1, V1, 5, , 1, th of its initial volume, the pressure is, 5, , increased by 5 times of the initial voluem., 5.3.2 Effect of Temperature on the Volume of Gas (Charles’ Law), The effects of temperature on the volume of the gas was studied by Jacques, Charles in 1787 and Gay Lussac in 1802 at constant pressure for different gases., Their conclusion can be given as Charles’ law which states that at a constant, pressure, the volume of a given amount of gas is directly proportional to the, absolute temperature., So, according to Charles’ Law, the volume of a gas increases as its absolute, temperature is being raised, if its absolute temperature is lowered, its volume will, consequently decrease. Mathematically, Charles’ Law is expressed as shown below:, V ∝ t, V = k .t, 142, , (at constant p and n), (k is a constant), CHEMISTRY
Page 172 :
MODULE - 3, , The Gaseous and Liquid State, , Therefore,, , States of Matter, , V1/t1 = V2/t2, , Graphical representation of Charles’ Law is a straight line pointing away from, the origin of the graph as shown in Fig. 5.9., Here graph of the volume of a gas (V) plotted against its temperature at constant, pressure and amount (in moles). Notice that the graph is a straight line with a, positive gradient (slope)., , Notes, , V, , P, n = constant, , –273ºC, , Temperature T, , Fig. 5.9 : A graph of V versues T, , Mathematically volume of a gas at temperature t is given as, vt = v0 +, Thus at, , t ⎞, ⎛, ⎛ 273 + t ⎞, v0, = v0 ⎜, × t = v0 ⎜1 +, ⎟, ⎝, ⎝ 273 ⎟⎠, 273 ⎠, 273, , t = –273ºC, ⎛, vt = v0 ⎜⎝, , 273 − 273 ⎞, ⎟ =0, 273 ⎠, , This means that at –273ºC, the volume of the gas is reduced to zero i.e., the gas, ceases to exist. Thus this temperature (–273ºC) at which the gas hypothetically, ceases to exist is called Absolute zero. It is represented by zero K., This is the theoretically lowest possible temperature. In actual practice, we cannot, reduce the temperature of the gas to zero kelvin., Kelvin Scale of Temperatue, The scale of temperature which has –273ºC as zero is called Kelvin Scale. Degree, celsius is converted to Kelvin by adding 273. Thus, t/ºC + 273 = T/K, where, , T = temperature in Kelvin, t = temperature in celcius, , For example 15ºC can be converted in K by adding 273 to 15., , CHEMISTRY, , 143
Page 173 :
MODULE - 3, States of Matter, , The Gaseous and Liquid State, , 5.3.3 Effect of Temperature on Pressure (Gay-Lussac's Law), This law states that., Pressure of given amount of a gas at constant volume is directly proportional, to its absolute temperature., p α T, , Notes, , p = kT, Example 5.3 : A given amount of a gas is maintained at constant pressure and, occupies a volume of 2 litres at 1000ºC. What would be volume if gas is cooled, to 0ºC keeping pressure constant., Solution : Given that,, Initial volume V1 = 2L, , T1 = 1000 + 273 = 1273 K, , Final volume V2 = ?, , T2 = 0 + 273 = 273 K, , Now using Charle’s Law V1/T1 = V2/T2 or V2 = (V1/T1) × T2, On substituting the values we get, V2 = (V1/T1) × T2 = (2L /1273 K) × 273 K = 0.4291 L, 5.3.4 Avogadros’ Law, The Italian physicist Amadeo Avogadro was the first to propose, in 1811, a, relationship between the volume of a gas and the number of molecules present in, it. This, relationship is known as Avogadros’ Law. It states that :, Equal volumes of all gases at the same temperature and pressure contain equal, number of molecules., Mathematically, Avogadros’ law is expressed as :, V α N (at constant temperature and pressure), Where V and N are volume and number of molecules respectively., At a given temperature and pressure, the number of molecules present in the gas, is directly proportional to the number of moles., therefore,, , N∝ n, , Where n is the number of moles, ∴, or, , 144, , V ∝ n, V, = constant, n, , CHEMISTRY
Page 174 :
MODULE - 3, , The Gaseous and Liquid State, , V1, V2, =, n1, n2, , Also, , States of Matter, , He also found that the number of molecules present in 1 mole of any substance, (22.4 litre of any gas at 273 K temperature and 1 atmosphere pressure) is 6.022, × 1023 molecules. This number is known as Avogadros’ number., It is the number of molecules (6.022 × 1023) of any gas present in a volume of, 22.4 L (at 273 K and 1 atm) and it is the same for the lightest gas (hydrogen) as, for a heavy gas such as carbon dioxide or bromine., , Notes, , Example 5.4 : 0.965 mol of a gas occupies a volume of 5.0 L at 298 K temperature, and 1 atm pressure. What would be the volume of 1.80 mol of the gas at the same, temperature and pressure?, Solution :, , V1 n2 = V2 n1, V2 =, , V1n2, (5.0 L) (1.8 mol), =, n1, (0.965 mol), , V2 = 9.33 L, Example 5.5 : Compare the volumes of 16 g of oxygen and 14 g nitrogen at the, same temperature and pressure., Solution :Number of moles of O2 = 16 g/32 g mol–1 = 0.5 mol, Number of moles of N2 = 14 g/28 g mol–1 = 0.5 mol, Since the two gases are at the same temperature and pressure, and contain equal, number of mole, hence according to the Avogadro’s Law they should also occupy, the same volume., , INTEXT QUESTION 5.1, 1. The density of a gas is usually less than that of the liquid. Explain., 2. Calculate the pressure (atm) required to compress 500 mL of gas at 0.20 atm, into a volume of 10 mL., 3. Equal volumes of oxygen gas and an unknown gas weigh 2.00 and 1.75 g, respectively under the same experimental conditions. What is the molar mass, of the unknown gas?, 4. What type of intermolecular interactions are present in (a) Ne gas (b) Carbon, monoxide., CHEMISTRY, , 145
Page 175 :
MODULE - 3, , The Gaseous and Liquid State, , States of Matter, , 5.3 THE IDEAL GAS EQUATION, Boyle’s Law, Charles’ Law and Avogadro’s Law can be combined to give a single, equation which represents the relation between the pressure, volume and kelvin, temperature of a given amount of a gas under different conditions. Thus, , Notes, , V ∝ 1/p, , at constant temperature (Boyles’ Law), , V∝T, , at constant pressure (Charles’ Law), , V∝n, , at constant temperature and pressure (Avogadros’ Law), , All the three expressions can be combined into a single expression, V ∝ nT/P or pV ∝ n T, or pV = constant × n T, The constant in this equation is called ‘universal gas constant’ or ‘molar gas, constant’, represented by R. Thus we can write for 1 mole of a gas, pV = RT, Correspondingly, for n moles of a gas we have, pV = n RT, This is known as the ideal gas equation because it holds only when gases are, behaving as ‘ideal’ gases., Since for a given mass of gas we can write, pV/T = a constant, we have, p1 V1/T1 = p2 V2/T2, Where p1, V1 and T1 refer to one set of conditions and p2, V2 and T2 refer to a, different set of conditions., The numerical value of R can be found by substituting experimental quantities in, the equation. At STP, T = 273.15 K, p = 1 bar (105 Pa) and for 1 mol of gas (n =, 1), V = 22.711 L. Consequently,, R = pV/nT = (105 Pa) (22.711 × 10–3 m3) / (1 mol) (273.15K), = 8.314 J K–1 mol–1, At STP conditions used earlier (0°C and 1 atom pressure) the volume occupied, by 1 mol of gas is 22.414 L, The value of R depends on the units adopted for the quantities in the equation, pV = nRT. The various values are:, , 146, , CHEMISTRY
Page 176 :
MODULE - 3, , The Gaseous and Liquid State, , States of Matter, , R = 8.314 J K–1 mol–1, R = 0.082057 L atm K–1 mol–1 (for calculation purpose the value is, taken as 0.0821 L atm K–1 mol–1), R = 8.314 × 107 erg K–1 mol–1, R = 1.987 cal K–1 mol–1, Example 5.6 : At 273 K, 10 mol of a gas is confined in container of volume, 224 L. Calculate the pressure of the gas. R = 0.0821 L atm mol–1 K–1., , Notes, , Solution : The ideal gas equation pV = nRT will be used here, n = 10 mol, R = 0.0821 atm L K–1 mol–1, V = 224 L T = 273K, , p=?, , On substituting these values in the above equation we get, p = nRT/V = (10 mol × 0.0821 atm L mol–1 K–1 × 273K) / 224L = 0.99998 atm, = 1 atm, , 5.4 DALTONS’ LAW OF PARTIAL PRESSURE, The behaviour observed when two or more non-reacting gases are placed in the, same container is given by Dalton’s Law of partial pressures. Dalton’s Law states, that., The total pressure exerted by a mixture of non-reacting gases is equal to the sum, of the partial pressures of the various gases present in the mixture., The partial pressure is defined as the pressure the gas would exert if it was alone, in the container. Suppose a sample of hydrogen is pumped into a one litre box, and its pressure is found to be 0.065 atm. Suppose, further a sample or argon is, pumped into a second one litre box and its pressure is found to be 0.027 atm. If, both samples are now transferred to a third one litre box, the pressure is observed, to be 0.092 atm. For the general case, Daltons’ Law can be written as, ptotal = pA + pB + pC +...., Where pA, pB, pC,.... are the partial pressure of gases A, B, C, .... respectively., This gas laws provide a simple way of calculating the partial pressure of each, component, given the composition of the mixture and the total pressure. First we, introduce the mole fractions XA and XB. These are defined as, XA =, , nA, n, , and XB =, , nB, n, , Where nA and nB are the number of moles of gas A and B respectively and n = nA, + nB., CHEMISTRY, , 147
Page 177 :
MODULE - 3, , The Gaseous and Liquid State, , States of Matter, , Since, , pA = nA RT/V, pB = nB RT/V and p = n RT/V,, , it follows that, pA = XA p and pB = XB p, This is an exceptionally useful (and simple) way of calculating at partial pressures, when the composition and total (measured) pressure of a mixture of gas is known., Notes, , Example 5.7 : Atmosphere is often considered mainly as a mixture of nitrogen, and oxygen: 76.8% by mass of nitrogen and 23.2% by mass of oxygen. Calculate, the partial pressure of each gas when the total pressure is 1 atm., Solution : The number of moles of each component is, pN2 = 76.8 g / 28 g mol–1 = 2.74 mol, pO2 = 23.2 g / 32 g mol–1 = 0.725 mol, , The mole fractions of the components are therefore, X N2 =, , 2.74, 0.725, = 0.791 ; X O2 =, = 0.209, 2.74 + 0.725, 2.74 + 0.725, , The partial pressures are therefore given by, pN2 = 0.791 × 1 atm = 0.791 atm, , pO2 = 0.209 × 1 atm = 0.209 atm, , 5.5 GRAHAM’S LAW OF DIFFUSION, If we open a bottle of perfume in one corner of a room or burn an incense stick, we can feel the smell of the perfume or the incense stick all over the room also., The smell of perfume or incense stick spreads from one point of the room to the, other by mixing with air. This free intermingling of gases when placed in contact, with each other is known as diffusion., Diffusion occurs in liquids as well as in gases. Effusion is the escape of a gas, through a small hole, as in case of a puncture in a tyre., The experimental observation of the rate of effusion of gases through a small, hole in the side led Graham (1829) to formulate the following law:, At constant temperature and pressure, the rate of diffusion of a gas is inversely, proportional to the square root of its density. Graham’s law is applicable to both, diffusion and effusion., If the time for a given volume of gas A to escape is tA, while the time for the same, volume of gas B to escape is tB, it follows, that,, 148, , CHEMISTRY
Page 178 :
MODULE - 3, , The Gaseous and Liquid State, , tA/tB = (rate)B / (rate)A = ρ A / ρB where ρA and ρB are the densities of gases A and, B respectively., , States of Matter, , The ratio of the densities of the gases is the same as the ratio of the molecular, masses of the gases at the same temperature and pressure., hence, tA/tB = (rate)B / (rate)A = ρA / ρB = M A /M B where MA and MB are the, molecular masses of gases A and B respectively., , Notes, , INTEXT QUESTIONS 5.2, 1. What is the difference between diffusion and effusion., 2. Explain why Daltons’ law is not applicable to a system of ammonia and, hydrogen chloride gas., 3. The rates of diffusion of CO2 and O3 were found to be 0.29 and 0.271. What, is the molecular mass of O3 if the molecular mass of CO2 is 44., 4. Calculate the pressure exerted by 5.0 mol of carbon dioxide in a 1 litre flask, at 47ºC using ideal gas equation., , 5.6 GAY LUSSAC’S LAW OF COMBINING VOLUMES, One of the laws of chemical bomination is Gay Lussac's law of combining, volumes., This law states that in any chemical reaction involving gases the volumes of, gaseous reactants and products (if any) bear a simple ratio to one another, when, measured under similar conditions of pressure and temperature in the reaction, N2(g) + 3H2(g) ⎯→ 2NH3(g), 1 vol, , 3 vol, , 2 vol, , The ratio of volumes of nitrogen, hydrogen and ammonia is 1 : 3 : 2 when their, volumes are measured at the same temperature and pressure. Similarly, in the, reaction, 2H2(g) + O2(g) ⎯→ 2H2O(l), 2 vol, , 1 vol, , hydrogen and oxygen always react in the ratio 2 : 1 by volume., This law is nothing but the law of definite proportions stated in terms of volume., Gay Lussac's law when combined with gas laws led to the concept of molecules., Gay Lussac’s Law and the concept of molecule, Prior to the Avogadro’s law, a similar hypothesis was put forward by Berzelius., “Equal volumes of all gases, under similar conditions of temperature and, CHEMISTRY, , 149
Page 179 :
MODULE - 3, States of Matter, , The Gaseous and Liquid State, , pressure contain equal number of atoms.” This hypothesis, when combined with, the Gay-Lussac’s law led to conclusions which contradicted Dalton’s atomic, theory. For example consider the gas phase reaction between hydrogen and, chlorine to form hydrogen chloride, Hydrogen(g) + Chlorine(g) ⎯→ Hydrogen chloride(g), 1 vol, , Notes, , 1 vol, , 2 vol (same T and P), , Gay Lussac’s law (experimental result), Berzelius, hypothesised, , x number of, hydrogen atoms, , x number of, chlorine atoms, , 2x number of, compound atoms, of hydrogen chloride, , Divided by x, , 1 atom of, hydrogen, , 1 atom of, chlorine, , 2 compound atoms, of hydrogen chloride, , 1, atom of, 2, hydrogen, , 1, atom of, 2, chlorine, , 1 compound atom, , Divided by 2, , of hydrogen chloride, , ('Compound atom' was the term used by Dalton for the smallest particle of, compounds before of molecule was developed), Thus, one compound atom of hydrogen chloride contains one-half atom of each, of hydrogen and chlorine. This contradicts the Dalton’s atomic theory according, 1, to which atoms are indivisible. Therefore fractional atoms ( atom each of H, 2, and Cl) cannot be present in hydrogen chloride. Due to this contradiction, Avogadro modified the Berzelius hypothesis by replacing the term ‘atom’ by, ‘molecule’. According to Avogadro’s law, equal volumes of all gases, under, similar conditions of temperature and pressure contain equal number of, molecules”. This law when applied to the same reaction as earlier, modifies the, conclusion., Hydrogen (g) + Chlorine (g) ⎯→ Hydrogen chloride (g), 1 vol, , 1 vol, , 2 vol, , Gay Lussac’s Law, Avogadros law ‘x’ molecules, of hydrogen, Divide by 2x, , 1, molecule, 2, of hydrogen, , ‘x’ molecules, of chlorine, 1, molecule, 2, of chlorine, , ‘2x’ molecules of, hydrogen chloride, 1 molecule of, hydrogen chloride, , Thus, now, one molecule of hydrogen chloride, is made from one-half molecule, each of hydrogen and chlorine. ‘Molecule’ was accepted as the smallest stable, 150, , CHEMISTRY
Page 180 :
MODULE - 3, , The Gaseous and Liquid State, , particle of matter (element or compound) which has the same properties as the, bulk of the matter. Further, it was suggested that each molecule of an element, may contain more than one atom of the element. Later studies showed that both,, hydrogen and chlorine molecules are diatomic and contain two atoms each. Their, chemical formulae are H2 and Cl2 respectively; one-half molecule, now, would, mean one atom of H or Cl. Thus one molecule (rather than compound atom), of hydrogen chloride would be formed by one atom each of hydrogen and, chlorine and its formula becomes HCl. Now we may write the reaction as, H2(g), 1 vol, ‘x’ molecules, 1, molecule, 2, 1 atom, , +, , Cl2(g), , ⎯→, , 1 vol, ‘x’ molecules, 1, molecule, 2, 1 atom, , States of Matter, , Notes, , 2HCl(g), 2 vol, ‘2x’ molecules, 1 molecule, 1 molecule, , Thus Gay-Lussac’s law and Avogadro’s Law led to the concept of ‘molecule’., , 5.7 KINETIC MOLECULAR THEOY OF GASES, (ACCOUNTING FOR THE GAS LAWS), To explain the behaviour of the gases theoretically, Clausius, Maxwell and, Boltzmann made the following assumptions:, (1) Gases consist of large number of tiny particles called molecules., (2) The gas molecules are so small and so far apart that the total volume of the, molecules is a negligible fraction of the total volume occupied by the gas., (3) The molecules are in a state of constant, rapid and random motion colliding, with one another and with the walls of the container., (4) There are no attractive or repulsive forces between the molecules of the gas., (5) The collisions of the molecules among themselves and with the walls of the, containing vessel are perfectly elastic, so that there is no loss of energy, during collisions., (6) The pressure exerted by a gas is due to the bombardment of the molecules, on the walls of the containing vessel., (7) The kinetic energy of a gas is a directly proportional to the absolute, temperature of the gas., On the basis of this model, it is possible to derive the following expression for a, gas:, pV =, , 1, m NC2, 3, , Where p is pressure, V denotes volume, m is the mass of a gas molecule, N is the total, number of molecules, and C is the root mean square velocity of the gas molecules., CHEMISTRY, , 151
Page 181 :
MODULE - 3, States of Matter, , The Gaseous and Liquid State, , 5.7.1 Root Mean Square Velocity, Root mean square velocity is the square root of the average of the squares of all, the molecular velocities. Mathematically,, RMS Velocity =, , (C, , 2, 1, , + C 22 + ...+ C 2N ) /N, , where C1, C2.... CN the molecular velocities., Notes, , 5.7.2 Average Velocities, This is defined as, uav =, , u1 + u2 + ... + u N, N, , and is given by the expression, uav =, , 8 RT, πM, , 5.8 DISTRIBUTION OF MOLECULAR SPEEDS, According to Maxwell in a gas all the molecules are in a state of constant rapid, random motion and they collide with one another and with the walls of the vessel., During collision the resdistribution of the energy takes place. As a result their, speed and the kinetic energy changes. Therefore at any instant different molecules, have different speed and hence different kinetic energy. At the given temperature, even though the speed of the individual molecule constinuously changes, the, fraction of the molecules having the same speed remains constant and this is, known as Maxwell–Bolttzmann Distribution Law., At the given temperature this fraction is denoted by, , dN, where dN is number of, N, , Fraction of molecules, dN ¾®, N, , molecules having the same velocity and N is the total number of the molecules, present in the gas. At the given temperature this fraction of the molecule is plotted, against the molecular speed as shown as figure 5.10., , T = Constant, , cmp cav crms, , Fig. 5.10: Maxwells distribution of speeds of molecules at a constant temperature, , 152, , CHEMISTRY
Page 182 :
MODULE - 3, , The Gaseous and Liquid State, , In the above Fig. 5.10, the maximum in the distribution curve corresponds to, the speed possessed by the highest fraction of molecules. This is known as the, most probable speed cmp. Also shown in the figure are the average speed,, cav and the root mean square (rms) speed crms These types of speeds are, related to the temperatures, T and the molar mass, M of the gas by the following, relations, , cmp =, , 2RT, M, , cav =, , 8RT, πM, , crms =, , 3RT, M, , States of Matter, , Notes, , The relative values of these speeds are, cmp : cav : crms, 1 : 1.13 : 1.22, Or, , 0.82 : 0.92 : 1, , From any of the above relations it can be seen that these speeds are related to, the temperature and molar mass of the gas., Dependence of molecular speeds on temperature, The temperature dependence of molecular speeds is shown in Fig. 5.11. On, increasing the temperature the fraction of molecules with higher speeds increases, and with somes speeds decreases. The maxima shifts to higher velocity side but, its height decreases., , T3 > T2 > T1, dN, N, , Velocity, Fig. 5.11: Effect of temperature on distribution of velocities., CHEMISTRY, , 153
Page 183 :
MODULE - 3, States of Matter, , The Gaseous and Liquid State, , Dependence of molecular speeds on molar mass of the gas, At a constant temperature any of the three types of speeds is inversely, proportional to the square root of the molar mass of the gas, that is,, , 1, M, Hence, at the same temperature, the most probable speed of a lighter gas would, be more than that of a heavier gas. Figure 5.12 a shows the distribution curves, of H2 and N2 gases., Cmp ∝, , Notes, , Fraction of molecules, dN ¾®, N, , H2, T = Constant, N2, , Molecular speed C ¾®, , Fig. 5.12 A: Effect of molar mass of the gas on distribution of speeds., , 5.9 DEVIATION FROM IDEAL GAS BAHAVIOUR, The gas laws mentioned above are strictly valid for an ideal gas under all conditions, of temperature and pressure. Real gases show deviations from these laws at low, temperature and high pressure. These deviations can be shown clearly by plotting, pV, as a function of pressure at constant temperature,, nRT, Vobserved, pV, =, = Z (compressibility factor), Videal, nRT, , Such a plot is shown in Fig. 5.13 for an ideal gas and a few real gases. you may, note that for in ideal gas pV = nRT hence, , pV, = z = 1. Thus a straight line, nRT, , obtained for ideal gas in this plot, but for real gases diferent curves are obtained., Gases deviate from ideal behaviour due to the following faulty assumptions of, kinetic theory :, 1. Volume of the molecules of a gas is negligibly small in comparison to the, space occupied by the gas., 2. There is no force of attraction between the molecules of a gas., 154, , CHEMISTRY
Page 184 :
MODULE - 3, , The Gaseous and Liquid State, , Contrary to assumption(1), the volume occupied by the molecules of a gas becomes, significant at high pressures. If nb is the volume occupied by the molecules, the actual, volume of the gas is (V – nb). Assumption (2) too doesn’t hold good as at high, pressures molecular interactions start operating. Molecules are dragged back by other, molecules which affects the pressure exerted by them on the walls of the container., Pideal = Preal +, (Preal is observed pressure and, , an 2, V2, , States of Matter, , an 2, V2, , Notes, , is correction term), , In view of the corrections for pressure and volume, ideal gas equation can be, rewritten as, ⎛, an 2 ⎞, ⎜⎝ p + 2 ⎟⎠ (V – nb) = nRT, V, , This is known as van der Waals' equation, Ethene, Methane, n, , roge, , Volume, , Hyd, , Ammonia, N, , Idealgas, , 0, , Pressure, 200, , 400, , 600, , 800, , 1000, , Fig. 5.13 : The plot volume versus P for real gases, , 5.10 LIQUEFACTION OF GAS, Any gas can be liquified at atmospheric pressure if it is cooled sufficiently. Many, gases (but not all) can be liquified at ordinary temperature by compressing them., The conditions of temperature and pressure under which gases liquify were first, investigated by Andrews in 1869., Andrews subjected CO2 to various pressures at different temperatures and plotted, the effect of pressure on volume (Fig. 5.14). The curve obtained at a given, temperature is called an isotherm. As can be seen in the figure, at 321 K the, volume of the gas decreased with the increased pressure approximately in, accordance with the Boyle’s Law. At 294 K, however, the volume first decreases, in accordance with Boyle’s Law until the pressure was increased to about 60, atm. At this pressure there was a sudden break in the curve and liquid carbon, dioxide appeared. The pressure remained constant until all the gas had been, converted into liquid. Subsequent increase of pressure caused practically no change, CHEMISTRY, , 155
Page 185 :
MODULE - 3, States of Matter, , The Gaseous and Liquid State, , in volume. In accordance with the general rule that extremely high pressures are, required to compress liquids appreciably., , Pressure ¾¾®, , Liquefaction of Gases, , Notes, , 343 K, 323 K, Liquid, , 304.1 K, Liquid and Gas, Gas, , 294 K, 286 K, , Volume ¾¾®, , Figure 5.14: Isotherms of carbon dioxide, , Similar changes took place when the isotherms were constructed for temperatures, below 294 K, except that the pressure required to liquify the gas became smaller, as the temperature decreased. Andrews found that liquifaction could be brought, about at all temperatures below 304.1 K. But above this temperature no liquifaction, occured no matter how much pressure was increased. This temperature was, therefore called the critical temperature for CO2. The pressure required to liquify, the gas at the critical temperature was called critical pressure, and the volume, of 1 mole of the substance at the critical temperature and pressure, the critical, volume., The temperature above which a gas cannot be liquified, however large the, pressure may be is known as critical temperature., Table 5.2 list values of the critical temperature and pressure critical volume for, some common substances., Table 5.2 : Critical temperatures and critical pressures, Substance, , Water, H2O, Sulphur dioxide, SO2, Ammonia, NH3, Hydrogen Chloride, HCl, Carbon dioxide, CO2, Oxygen, O2, Nitrogen, N2, Hydrogen, H2, , 156, , Critical, Temperature (K), , Critical, Pressure (atm), , 647, 430, 406, 324, 304, 154, 126, 33, , 217.7, 77.7, 112.5, 81.6, 73.0, 49.7, 33.5, 12.8, , CHEMISTRY
Page 186 :
MODULE - 3, , The Gaseous and Liquid State, , States of Matter, , INTEXT QUESTIONS 5.3, 1. What are the conditions under which real gases behave as ideal gas., 2. Which term in van der waals equation accounts for the molecular volume., 3. Calculate the root mean square velocity of ozone kept in a closed vessel at, 20ºC and 1 atm pressure., , Notes, , 4. What is compressibility factor., , 5.11 NATURE OF LIQUIDS, Look at Figure 5.1 in which the molecular arrangement has been shown in the, three states of matter. What do you notice?, In figure 5.1a, you would find that the molecules, are far apart. A gaseous state can be represented, by this arrangement. In liquid state (figure 5.1b),, molecules are closer as compared to gaseous, state. You would notice that they have very, little spaces between them. However, there is, no order in arrangement of molecules. Further, we say that, these molecules can move about,, but with lesser speeds than those in gases. They, can still collide with one another as in the gaseous, state. You would recall that the molecules in, gases have very little attraction between them., But in liquid state the attraction between the, molecules is comparatively much stronger as, compared to that in the gaseous state. The, attractions are strong enough to keep the, molecules in aggregation. Contrary to this, in, solids (Fig. 5.1a) you notice that the molecules, are arranged at the closest possible distance., Solid state is a well ordered state and has very, strong intermolecular forces. You would learn, more about solids in lesson 8., , (a), , (b), , (c), Fig. 5.15 : Arrangement of, molecuels in (a) solids;, (b) liquids and (c) gases, , We would say, in a gas there is complete chaos, due to very weak intermolecular forces, whereas, in solids there is a complete order due to strong forces. Liquids fall between, gases and solids. Liquid molecules have some freedom of gases state and some, order of solid state. Intermolecular forces in liquids are strong enough to keep, the molecules close to one another but not strong enough to keep them in perfect, order., CHEMISTRY, , 157
Page 187 :
MODULE - 3, , The Gaseous and Liquid State, , States of Matter, , 5.12 PROPERTIES OF LIQUIDS, In this section you would learn how the properties of liquids can be explained in, terms of molecular arrangement and intermolecular forces. Let us consider a few, properties of liquids as examples., , Notes, , 5.12.1 Volume and Shape, You would recall that the liquids (for example water) take the shape of the, container in which they are kept. However, they have a definite volume. How can, you explain the properties of definite volume and variable shape? In liquids, the, attractive forces are strong enough to keep the molecules moving within a definite, boundary. Thus, they maintain a definite volume. These intermolecular forces are, not strong enough to keep them in definite positions. The molecules can, therefore,, move around and take the shape of the container in which they are kept., 5.12.2 Compressibility, Compressibility of a substance is its ability to be squeezed when a force is applied on, it. Let us study the compressibility of liquids with the help of the following activity., , ACTIVITY 5.1, Aim: To study the compressibility of water., What is required?, A 5 mL syringe and water., What to do?, (i) Take the syringe and fill it with water by pulling out the plunger., (ii) Note the volume of water., (iii) Press the plunger while blocking the nozzle of the syringe with a finger., What to observe?, Observe the volume of water in the syringe while pressing the plunger. Does the volume, of water change by pressing the plunger? You would observe that it does not change., The above activity clearly shows that liquids are largely incompressible. It is, because there is very little empty space between the molecules. In contrast, the, gases are highly compressible because of large empty spaces between their, molecules., 158, , CHEMISTRY
Page 188 :
The Gaseous and Liquid State, , The large difference in the free space in gaseous and liquid states becomes, evident from the fact that the volume occupied by a given amount of a, substance in liquid state is 100-1000 times less than that in the gaseous state., , MODULE - 3, States of Matter, , 5.12.3 Diffusion, Diffusion is the process of spreading of a substance from a region of higher, concentration to a region of lower concentration. Let us study the phenomenon, of diffusion in liquids with the help of the following activity., , Notes, , ACTIVITY 5.2, Aim : To study the phenomenon of diffusion through water., What is required?, A glass, water, blue ink and a dropper., What to do?, (i) Take some water in the glass., (ii) Add a few drops of blue ink into water with the help of a dropper., What to observe?, Observe the water and ink in the beaker., Initially the ink does not mix with water. After some time it starts spreading, slowly. After a few hours the whole of water in the glass becomes coloured due, to diffusion of ink through water., The above activity demonstrates that diffusion occurs in liquids. Why does it, happen? Because the molecules of both the liquids are moving and help in the, diffusion process., 5.12.4 Evaporation, You know that water left in an open pan evaporates slowly until the pan becomes, dry. Evaporation is the process by which a liquid changes into vapour. It occurs, at all temperatures from freezing point to boiling point of the liquid., In a liquid, at any temperature, a small fraction of the molecules is moving with, relatively high velocity. Such molecules have high kinetic energy. These can, overcome the intermolecular attractive forces and escape through the surface of, the liquid., Rate of evaporation of a liquid depends on a number of factors. For example,, more is the surface area, faster will be the evaporation. For faster drying, we, CHEMISTRY, , 159
Page 189 :
MODULE - 3, States of Matter, , Notes, , The Gaseous and Liquid State, , increase the surface area by spreading the wet clothes. If we supply heat to the, liquid, evaporation is faster. The wet clothes dry faster in the sun. The increase in, temperature increases the kinetic energy of the molecules of the liquid and the, liquid evaporates at a faster rate. We feel cool after the bath. Why do we feel so? It, is because during evaporation water takes the heat from our body and we feel cold., Now let us compare the rate of evaporation of two liquids, for example, water and, alcohol. Which of these two liquids evaporates faster? You must have experienced, that alcohol evaporates faster. Why does this happen? The number of molecules, escaping from a liquid depends upon the attractive forces. When these forces are, stronger, fewer molecule escape. In alcohol, these attractive forces are weaker than, those in the water. Hence, alcohol evaporates faster than water., , 5.13 VAPOUR PRESSURE AND BOILING POINT, In the previous section you have learnt that liquids evaporate when kept in an, open vessel. Different liquids evaporate to different extent under similar conditions., The extent of evaporation of a liquid is measured with the help of vapour, pressure of a liquid. In this section, you will study about it and also about the, boiling point of a liquid., 5.13.1 Vapour Pressure of a Liquid, You know that a liquid placed in an open vessel evaporates completely. If,, however, the liquid is allowed to evaporate in a closed vessel, say in stoppered, bottle or a bell jar, evaporation occurs, but after sometime the level of the liquid, does not change any further and becomes constant. Let us understand how does, it happen. In the closed vessel, the molecules evaporating from the liquid surface, are confined to a limited space. These molecules may collide among themselves, or with the molecules of air and some of them may start moving towards the, surface of the liquid and enter into it. This is known as condensation. In the, , Initially molecules, evaporate, , After some time, condensation begins, , At equilibrium the rates of, eavporation and condensation, become equal, , Fig. 5.16 : Establishing (vapour liquid) equilibrium under a evacuated jar, , 160, , CHEMISTRY
Page 190 :
MODULE - 3, , The Gaseous and Liquid State, , beginning, rate of evaporation is greater than the rate of condensation. But as, more and more molecules accumulate in the space above the liquid, rate of, condensation gradually increases. After some time, rate of evaporation becomes, equal to the rate of condensation and an equilibrium state is reached, (Fig. 5.16). The number of molecules in the vapour above the liquid becomes, constant. These molecules exert certain pressure over the surface of the liquid., This pressure is known as equilibrium vapour pressure, saturated vapour, pressure or simply as vapour pressure. The vapour pressure of a liquid has a, characteristic value at a given temperature. For example, vapour pressure of, water is 17.5 Torr and that of benzene is 75.00 Torr at 20º C. The vapour, pressure of a liquid increases with increase in temperature. It is so because at a, higher temperature more molecules have sufficiently high energy to overcome, the forces of attraction and escape to form vapour. A plot of vapour pressure as, a function of temperature is called vapour pressure curve. Figure 5.17 depicts, the vapour pressure curves of some liquids., , Ethanal, , Notes, , Water, , Vapour Pressure/torr ®, , Diethyl, ether, , States of Matter, , 760, , T1, T2, T3, Temperature / °C ®, , Fig. 5.17 : Vapour pressure curves of some liquids., , What would happen if we remove some of the vapour from the closed vessel., Would the vapour pressure of the liquid increase, decrease or remain constant?, Vapour pressure of the liquid would remain constant at that temperature. In the, beginning, the vapour pressure would decrease after the removal of the vapour,, but soon more liquid would evaporate to maintain the equilibrium and the, original vapour pressure would be restored. So the vapour pressure of a liquid, has a definite value at a particular temperature., 5.13.2 Boiling, You must have seen the formation of bubbles at the base of a vessel, in which a, liquid is heated. The rate of formation of bubbles increases with increse in heat, supplied. What are the bubbles made up of? The first bubbles that you see are of, the air, which is driven out of the liquid by increase in temperature. After some, CHEMISTRY, , 161
Page 191 :
MODULE - 3, States of Matter, , Notes, , The Gaseous and Liquid State, , time, bubbles of the liquid are formed throughout vapour. These bubbles rise to, the surface and break. When this happens, we say that the liquid is boiling. The, bubbles in the liquid the liquid rise and break form only if its vapour pressure is, equal to the atmospheric pressure., The temperature at which boiling occurs is called the boiling point of the liquid., At this temperature the vapour pressure of the liquid is equal to the atmospheric, pressure. The boiling point, therefore, depends upon the atmospheric pressure., For example, water boils at 100ºC at 760 Torr and at 97.7ºC at 700 Torr., The normal boiling point of a liquid is defined as the temperature at which, the vapour pressure of a liquid is equal to one atmosphere or 760 Torr., The boiling point of a liquid depends upon its nature. A more volatile liquid, would boil at a lower temperature than a less volatile liquid. You can again refer, to figure 5.3 and note that diethyl ether boils at a much lower temperature than, water, because it is highly volatile liquid. The boiling point of ethanol lies in, between those of diethyl ether and water. Vapour pressures or boiling points of, liquids give us an idea of the strength of attractive forces between molecules in, liquids. Liquids having lower boiling points have weaker attractive forces in, comparison to those having higher boiling points., You can make a liquid boil at temperature other than its normal boiling point., How? Simply alter the pressure above the liquid. If you increase this pressure,, you can increase the boiling point and if you can decrease this pressure you, decrease the boiling point. On the mountains, the atmospheric pressure decreases, and therefore boiling point of water also decreases. People living on hills face, problem in cooking their meals. They, therefore, use pressure cooker. How food, is cooked faster in it? The lid of pressure cooker does not allow water vapours to, escape. On heating the water vapours accumulate and the inside pressure, increases. This makes the water boil at a higher temperature and the food is, cooked faster., 5.13.3 Evaporation and Boiling, Evaporation and boiling, both involve conversion of a liquid into vapour and, appear to be similar. However, they differ from each other in some aspects., Evaporation occurs at all temperatures from freezing point of a liquid to its, boiling point, while boiling occurs at a definite temperature only i.e, at its boiling, point. Evaporation occurs slowly while boiling is a fast process. Evaporation of a, liquid occurs at its surface alone while boiling occurs throughout the liquid., These differences between evaporation and boiling have been summarized in, Table 5.3., , 162, , CHEMISTRY
Page 192 :
MODULE - 3, , The Gaseous and Liquid State, , States of Matter, Table 5.3 : Differences between evaporation and boiling, S.No., , Evaporation, , Boiling, , 1., , It takes place at all temperatures., , It takes place at a definite temperature., , 2., , It is a slow process., , It is a fast process, , 3., , It occurs only at the surface of, the liquid., , It occurs throughout the liquid., , Notes, , INTEXT QUESTIONS 5.1, 1. Match the following., Column I, , Column II, , (i) Liquids have a definite, volume., , (A), , The molecules in a liquid can, move about., , (ii) Liquids acquire the shape of, their container., , (B), , The molecules in liquids are, close and have very little free, space., , (iii) Liquids are largely, incompressible., , (C), , The inter molecular forces, liquids strong enough to keep, the molecules moving with in, a definite space., , 2. When a liquid is heated till it starts boiling., (i) What are the small bubbles that appears initially at the bottom and, sides of the vessel made up of?, (ii) What are the large bubbles that form in the boiling liquid made up, of?, 3. Liquids A, B and C boil at 65ºC, 120ºC and 90ºC respectively. Arrange them, in the decreasing order of the strength of intermolecular forces., , 5.14 SURFACE TENSION, Liquids show the effects of inter molecular forces most dramatically in another, property, namely, surface tension. Any molecule in the interior of liquid is, equally attracted by neighbour molecules from all sides and it does not experience, any ‘net’ force. On the other hand, any molecule at the surface of a liquid is, attracted by other molecules at the surface of the liquid or below it. Due to the, CHEMISTRY, , 163
Page 193 :
MODULE - 3, States of Matter, , Notes, , The Gaseous and Liquid State, , imbalance of forces, any molecule at the surface experiences a net inward pull, (Figure 5.18). As a result, the surface is under tension as if the liquid were, covered with a tight skin (or stretched membrane). The phenomenon is called, surface tension. Quantitatively, the surface tension is defined as the force, acting on an imaginary line of unit length drawn on the surface of the liquid and, acting perpendicular to it towards the liquid side as shown in Figure 5.5. It is, represented by the Greek letter gamma, γ. Its SI unit is newton per metre (N m–, 1, ) and CGS unit is dyne per centimetre (dyne cm–1). The two units are related as, : 1 N m–1 = 103 dyne cm–1, , Fig. 5.18 : Forces acting on molecules, at the surface and in bulk of liquids, , Surface molecules of a liquid experience a constant inward force. Therefore they, have a higher energy than the molecules in the bulk of the liquid. Due to this, reason liquids tend to have minimum number of molecules at their surface. This is, achieved by minimising the surface area. In order to increase the surface area, more molecules must come to the surface. This can happen only if some energy is, supplied or work is done. The energy supplied (or work done) for increasing the, surface area of a liquid by a unit amount is known as its surface energy. Its, units are joule per square metre J m–2 or N m–1 (since 1J = 1N m). Thus, dimensionally, the surface tension and surface energy are similar quantities and, they have the same numerical value., , Fig. 5.19 : Surface tension force acting on the surface of a liquid., , 164, , CHEMISTRY
Page 194 :
MODULE - 3, , The Gaseous and Liquid State, , States of Matter, , Effect of Temperature, On raising the temperature surface tension of a liquid decreases. It completely, vanishes at the critical temperature. This happens due to the following two, factors:, (i) On heating, the liquids expand. This increases the intermolecular distances., (ii) On heating, the average kinetic energy of molecules and hence their chaotic, motion increases., , Notes, , Due to both of these factors, the intermolcular forces become weak and the, surface tension decreases., Effect of Adding Surface Active Solutes, The solutes which get more concentrated on the surface of the liquid than in the, bulk are called surface active solutes or surfactants. Alcohols are examples of, such substances. Their addition to a liquid lowers its surface tension. The, cleaning action of soaps and detergents is based on this fact., Some Effects of Surface Tension, Surface tension results in many interesting and important properties of liquids., Let us now study some of them., (i) Spherical Shape of liquid drops, wetting, liquid, , non-wetting, liquid, , You have already learnt that liquids tend to, have a minimum surface area. For a given, volume, the geometrical shape having, Fig. 5.20 : Wetting and non-wetting, minimum surface area is a sphere. Hence,, liquids on the surface of a solid., liquids have a natural tendency to form, spherical drops, when no external force acts on them. Rain drops are distorted, spheres and the distortion is due to the friction of air., (ii) Wetting and Non-wetting properties, When a drop of liquid is placed on a solid surface, the force of gravity should, cause it to spread out and form a thin layer (Fig. 5.20). Such a liquid is called a, wetting liquid. This happens in case of most of the liquids. For example, drops, of water or alcohol spread out on the surface of glass. Some liquids behave, differently. When a drop of mercury is placed on the surface of glass, it does not, spread out (Fig. 5.20). Such liquids are called non-wetting liquids., , CHEMISTRY, , 165
Page 195 :
MODULE - 3, States of Matter, , Notes, , The Gaseous and Liquid State, , Wetting or non-wetting nature of a liquid depends upon two types of forces. The, intermolecular attractive forces between molecules of a liquid are called cohesive, force while those between the molecules of the liquid and the solid (whose, surface is in contact with the liquid) are called adhesive forces. If adhesive, forces are stronger than cohesive forces, the liquid would be wetting in nature, and when cohesive forces are stronger than adhesive forces it would be nonwetting in nature on the surface of a particular solid., (iii) Capillary Action, Let us carry out the following activity., , ACTIVITY 5.3, Aim : To study the capillary action., What is required?, Glass capillary tubes, water, mercury and two petri dishes., What to do?, (i) Take some water in a petri dish, (ii) Dip one end of a 3-4 cm long capillary in it., (iii) Take some mercury in another petri dish., (iv) Dip one end of another 3-4 cm long capillary in it., What to observe?, Observe the levels of water and mercury in the capillaries. Is it below or above, the levels of the liquids in petri dishes?, , (a), , (b), Fig. 5.21 : Capillary Action, , You would observe that when one end of a capillary tube is dipped in water, it, rises in the capillary as shown in Fig. 5.21(a). On the other hand when one end of, 166, , CHEMISTRY
Page 196 :
MODULE - 3, , The Gaseous and Liquid State, , a capillary tube is dipped in mercury, its level falls in the capillary as in Fig., 5.21(b)., The phenomenon of rise or fall of a liquid in a capillary is known as capillary, action. The rise of water in the glass capillary is due to its wetting nature as the, adhesive forces are stronger than cohesive forces. Water tends to increase the, area of contact with glass wall of the capillary by rising in it. Mercury being nonwetting with respect of glass (its cohesive forces are stronger than adhesive, forces) tends to minimise the area of contact by depressing inside the capillary, , States of Matter, , Notes, , (iv) Curved meniscus, When a wetting liquid such as water is taken in a glass tube, the liquid tends to, rise slightly along the walls of the tube for increasing its area of contact with, glass. The surface of the liquid (meniscus) becomes curved. It is concave in shape, [Fig. 5.22(a)]. When a non-wetting liquid like mercury is taken a glass tube, it, tends to decrease its area of contact and depresses along the walls of the glass, tube. The meniscus is convex in shape in this case [Fig. 5.22(b)]., , (a) Concave, meniscus, , (b) Convex, meniscus, , Fig. 5.22 : Curved meniscus of liquids, , 5.15 VISCOSITY, Every liquid has the ability to flow. It is due to the fact that molecules in a liquid, move freely, although within a limited space. Water flows down a hill under, gravitational force or through pipes when forced by a pump. Some external, force is always required for a liquid to flow. Some liquids like glycerol or honey, flow slowly while others like water and alcohol flow rapidly. This difference is, due to the internal resistance to flow which is called viscosity. The liquids with, higher viscosity flow slowly and are more viscous in nature like glycerol or, honey. Water and alcohol have lower viscosity and are less viscous in nature., CHEMISTRY, , 167
Page 197 :
MODULE - 3, States of Matter, , The Gaseous and Liquid State, , They flow more rapidly., , Notes, Surface of a solid, , Fig. 5.23 : Flow of different layers of a liquid, , The viscosity is related to the intermolecular forces. Stronger the intermolecular, forces more viscous are the liquids. Let us understand this with the help of Figure, 5.9. When a liquid flows steadily, it flows in different layers with one layer sliding, over the other. Such a flow is known as laminar flow. Consider a liquid flowing, steadily on a plane surface. The layer closest to it is almost stationary due to, adhesive forces. As the distance of the layer from the surface increases, is, velocity increases. Thus different layers move with different velocities. Due to, intermolecular forces (cohesive forces) each layer experiences a force of friction, from its adjacent layers. This force of friction, f between two layers depends, upon:, (i) area of contact between them A., (ii) distance between the layers, dx., (iii) difference in velocity between the layers, du., These quantities are related as, f=ηA, , du, dx, , du, , Here η (Greek letter ‘eeta’) is called the coefficient of viscosity and, is the, dx, velocity gradient between the layers., If A = 1 cm2, du = 1 cm s–1 and dx = 1 cm, then, f=η, Thus, coefficient of viscosity is the force of friction between two parallel layer of, the liquid which have 1cm2 area of contact, are separated by 1cm and have a, velocity difference of 1cm s–1. It may be noted that f is also equal to the external, force which is required to overcome the force of friction and maintain the steady, flow between two parallel layers having A area of contact, and which are dx, distance apart and moving with a velocity difference of du., 168, , CHEMISTRY
Page 198 :
The Gaseous and Liquid State, , MODULE - 3, States of Matter, , Units, CGS unit of viscosity is dyne cm–2 s. This unit is also known as poise (P). The SI, unit of viscosity is N m–2s or Pa s. The two units are related as :, 1 Pa s = 10 P, The unit poise is found to be too large and its submultiples centipoise (1 cP = 10–, P) and milli poise (1 mP = 10–3 P) are used for liquids and micropoise (μP = 10–, 6, P) is used for gases., 2, , Notes, , Effect of Temperature, Viscosity of a liquid decreases on raising the temperature. It is due to decrease in, intermolecular forces on heating as discussed in previous section (Section 5.4)., , INTEXT QUESTIONS 5.5, 1. Fill in the blanks., (i) A molecule at the surface of a liquid has ............... energy than the, one within the liquid., (ii) Surface tension of liquid .................... on cooling., (iii) Meniscus of a non-wetting liquid is ............... in shape while that of, a wetting liquid is ..................... in shape., (iv) When one end of a glass capillary tube was dipped in a liquid, the, level of liquid inside the capillary was observed to fall. The adhesive, forces in this liquid are ................. than the cohesive forces between, the liquid and glass., (v) Liquid X is more viscous than liquid Y. The intermolecular forces in, Y are ................... than in X., 2. What are the SI units of, (i) Surface tension., (ii) Coefficient of viscosity, 3. Why do liquids have a tendency to acquire minimum surface area?, , WHAT YOU HAVE LEARNT, z, , Matter exists in three states, namely, solid, liquid and gas., , z, , The three states of matter differ in the relative closeness of the molecules, constituting them., , CHEMISTRY, , 169
Page 199 :
MODULE - 3, States of Matter, , Notes, , The Gaseous and Liquid State, z, , Intermolecular interactions present depend upon the nature of the substance., Various types of interactions are London or dispersion forces, dipole-dipole, dipode induced dipole forces and hydrogen bonding., , z, , There exists a definite relationship between the pressure, volume, temperature, and number of moles of a gas and they are given by Boyle’s law, Charle’s law, and Avogadro’s law., , z, , The gases obeying gas laws are known as ideal gases., , z, , Dalton’s law give the relationship between partial pressures exerted by the, non-reacting gases to the total pressure., , z, , Gay Lussac's law of combining volume and Avogadre's law led to the concept, of molecule., , z, , Most of the gases deviate from the ideal behaviour. The deviations of gases, from ideal behaviour is due to the wrong assumptions of kinetic molecular, theory., , z, , Real gases can be liquified under appropriate conditions., , z, , In liquids the intermolecular force are quite strong as compared to gases but, weak enough to allow the molecules to move within a limited space and the, intermolecular distance is short., , z, , Liquids have definite volume but no definite shape, are almost incompressible, and can diffuse., , z, , Liquids evaporate and exert a definite vapour pressure at specified temperature., , z, , Boiling point is the temperature at which the vapour pressure of the liquid, becomes equal to the external pressure., , z, , Surface tension is the force acting on an imaginary line of unit length drawn, on the surface of the liquid and acting perpendicular to it towards the liquid, side., , z, , Due to surface tension, liquids tend to have minimum surface area and show, the phenomena of capillary rise or fall and curved meniscus., , z, , Viscosity is the internal force of friction to the flow of liquid., , TERMINAL EXERCISE, 1. Draw the graphs of the following :, a) p vs V at constant T and n, b) 1/V vs p at constant T and n, c) T vs V at constant p, 170, , CHEMISTRY
Page 200 :
MODULE - 3, , The Gaseous and Liquid State, , 2. What is the volume occupied by one mole of a gas at STP (0°C, 1 bar pressure)?, , States of Matter, , 3. The volume of a sample of a gas is 500 mL at a pressure of 1.5 atm. If the, temperature is kept constant, what will be the volume of that gas at, (i) 1 atm., (ii) 5.0 atm., 4. List the wrong assumptions of kinetic theory of gases which led to van der, Waal's equation., 5. What is the standard temperature and pressure?, , Notes, , 6. What is the lowest possible temperature?, 7. CO2 can not be liquefied at 35ºC, however large the pressure may be, Why?, 8. A sample of nitrogen gas weighing 9.3 g at a pressure 0.99 atm occupies a, volume of 12.4 litres when its temperature is 55K. What will be its volume, when the temperature is 220 K? Assume pressure is kept constant., 9. Calculate the volume of one mole of oxygen at 27ºC and 2 atm pressure,, given that the volume of oxygen at STP is 22.4 litres., 10. What is the Maxwell-Boltzmann Law?, 11. Explain the following properties of liquids on the basis of their structure:, (i) Volume (ii) Shape (iii) Compressibility, , (iv) Ability to flow, , 12. Why diffusion can occur in liquids. Explain., 13. Define (i) vapour pressure and (ii) boiling point., 14. Differentiate between evaporation and boiling., 15. Explain the effect of temperature on vapour pressure of a liquid., 16. Define surface tension and give its CGS and SI units., 17. What is surface energy?, 18. Why is energy required to increase the surface area of a liquid?, 19. What is the effect of addition of a surface active substance on the surface, tension of a liquid., 20. Why are liquid drops spherical in shape?, 21. What are wetting and non-wetting liquids?, 22. The cohesive forces acting in liquids A and B are C1 and C2 respectively and, C1 > C2 Which of them would have higher surface tension., 23. Liquid A rises in glass capillary tube. If one drop of it is put on a plane glass, surface, would it spread out or not. Explain., 24. A liquid forms a convex meniscus in glass tube. Comment on its nature., 25. Define viscosity., 26. What is coefficient of viscosity?, , CHEMISTRY, , 171
Page 201 :
MODULE - 3, States of Matter, , The Gaseous and Liquid State, , 27. Give CGS and SI units of coefficient of viscosity., 28. What is the effect of temperature on (i) vapour pressure (ii) surface tension, and (iii) viscosity of a liquid?, , ANSWERS TO INTEXT QUESTIONS, , Notes, 5.1, , 1. Due to more intermolecular distances in gaseous molecule compared to liquid., 2. Boyle Law equation is, p1V1 = p2V2, (.20 atm) (500 mL) = p2 (10 mL), p2 =, , (0.20 atm) (500 ml), 10 ml, , p2 = 10 atm., 3. By Avogadro’s Law, moles of O2 = moles of unknown gas, 2.00 g, 1.75 g, −1 =, 32 g mole, Molecular weight of unknown gas, , Molar mass of unknown gas =, , 1.75 × 32, = 28 g mol–1, 2.00, , Molar mass of unknown gas is 28., 4. (a) Dispersion or London Forces (b) dipole-dipole interactions and dispersion, forces., 5.2, 1. Movement of gas molecules through another gas is called diffusion., When gas escapes from a container through a very small opening it is called, effusion., 2. Ammonia and hydrogen chloride gases are reacting gases and Dalton’s Law, is applicable to mixture of non-reacting gases., 3., , rO3, rCO2, , ⎛ M CO2 ⎞, = ⎜ M ⎟, ⎝ O3 ⎠, , ⎛ 44 ⎞, 0.271, = ⎜M ⎟, 0.290, ⎝ O3 ⎠, , 172, , 1/ 2, , 1/ 2, , CHEMISTRY
Page 202 :
The Gaseous and Liquid State, , MODULE - 3, States of Matter, , Squaring both sides, 44, (0.271) 2, 2 =, M O3, (0.290), M O3 =, , 44 × 0.29 × 0.29, 0.271 × 0.271 = 50.4, , Molecular mass of O3 = 50.4, 4. By ideal gas equation, pV = nRT, p × 1.0 = (5.0 mol) (0.0821 L atm K–1 mol–1) 320 K, p =, , Notes, , (5.0 mol) (0.0821 L atm K –1 mol –1 ) 320 K, 1.0 L, , p = 131.3 atm., 5.3, 1. Low pressure and high temperature., 2. b, 3., , urms =, , =, , =, , 3RT, M, 3 (8.314 Jk –1 mol –1 ) (293 K), (0.048 kg mol –1 ), , (8.314 Kg m 2s −2 K −1 mol −1 ) (293 K), 0.048 Kg mol −1, , = 390.3 ms–1, 4. Z =, , pVm, = where Vm = molar volume, RT, , Z is compressibility factor., 5.4, 1. (i) C;, , (ii) A; (iii) B, , 2. (i) Air (ii) Liquid., 3. B > C > A, , CHEMISTRY, , 173
Page 203 :
MODULE - 3, States of Matter, , The Gaseous and Liquid State, , 5.5, 1. (i) more, (ii) increases, (iii) convex; concave, (iv) stronger, , Notes, (v) weaker, 2. (i) N m–1;, , (ii) N m–2 s, , 3. Molecules in the surface of a liquid have higher energy due to an inward force, on them. Therefore liquids tend to have minimum number of molecules in the, surface or have minimum surface area., , 174, , CHEMISTRY
Page 204 :
MODULE - 3, , The Solid State, , States of Matter, , 6, Notes, , THE SOLID STATE, , You are aware that the matter exists in three different states viz., solid, liquid and, gas. In these, the constituent particles (atoms, molecules or ions) are held together, by different forces of attraction between them. However, the nature and magnitude, of the forces varies. In the first two lessons of this module you have learnt about, the gaseous and the liquid states of matter. In this lesson you would learn about, solid state- a compact state of matter. The solids are distinguished from a liquid, or gas in terms of their rigidity which makes them occupy definite volume and, have a well defined shape. In solid state, the constituent particles are in close, contact and have strong forces of attraction between them. Here, you would, learn about structure, classification and properties of solids., , OBJECTIVES, After reading this lesson, you should be able to:, z, , explain the nature of solid state;, , z, , explain the properties of solids in terms of packing of particles and intermolecular attractions;, , z, , differentiate between crystalline and amorphous solids;, , z, , explain the melting point of a solid;, , z, , classify the crystalline solids according to the forces operating between the, constituent particles;, , z, , define the terms crystal lattice and unit cell;, , z, , describe different types of two dimensional and three dimensional unit cells;, , z, , explain different types of packing in the solids;, , z, , define coordination number;, , CHEMISTRY, , 175
Page 205 :
MODULE - 3, States of Matter, , Notes, , The Solid State, z, , calculate the number of particles in simple cubic, face-centrad cubic and, body centered cubic unit cells;, , z, , relate the density of a solid to the properties of its unit cell;, , z, , calculate the packing efficiency of simple cubic body central cubic and CCP/, HCP lattices;, , z, , define radius ratio;, , z, , correlate the radius ratio with the structure of solids;, , z, , explain the structure of simple ionic compounds;, , z, , explain Frenkel and Schottky defects;, , z, , classify solids on the basis of their electrical and magnetic properties; and, , z, , explain the effect of doping of semiconductors by electron deficit and electron, rich impurities., , 6.1 NATURE OF SOLID STATE, You have learnt in lesson 6 that according to Kinetic Molecular Theory, the, gases consist of a large number of molecules, which are in constant random motion in all directions in the available space. These molecules have very weak or, negligible forces of attraction between them. A sample of gas can be compressed,, as there is a lot of free space between the molecules Fig. 6.1(a) In liquids Fig., 6.1(b) on the other hand the molecules are also in constant motion but this motion is relatively restricted. Since there is very little free space available between, the molecules the liquids are relatively incompressible., , (a), , (b), , (c), , Fig. 6.1: A pictorial representation of the three states of matter: (a) gas, (b) liquid and (c) solid state., , In solid state the constituent particles are arranged in a closely packed ordered, arrangement Fig. 6.1 (c) with almost no free space. They can just vibrate about, their fixed positions. These are in close contact and cannot move around like the, molecules of a gas or a liquid. As a consequence, the solids are incompressible,, rigid and have a definite shape. Like liquids, the volume of a solid is independent, of the size or the shape of the container in which it is kept., , 176, , CHEMISTRY
Page 206 :
The Solid State, , MODULE - 3, States of Matter, , 6.2 CLASSIFICATION OF SOLIDS, On the basis of nature of arrangements of the constituent particles the solids, are classified into amorphous and crystalline solids., 6.2.1 Amorphous and Crystalline Solids, In crystalline solids the constituent particles are arranged in a regular and periodic pattern and give a well defined shape to it. The term ‘crystal’ comes from the, Greek word, krustallos meaning ice. The regular pattern extends throughout the, solid and such solids are said to have long range order. On the other hand, some, solids have only a short range of order. This means that the particles are arranged regularly in only some regions of the solid and are relatively disordered in, other regions. Such solids are called amorphous solids. In Greek, a means, without and morph means form. Thus the word amorphous means without, form. Sodium chloride and sucrose are common examples of crystalline solids, while glass, fused silica, rubber and high molecular mass polymers are some examples of amorphous solids., , Notes, , An important difference between the amorphous and crystalline solids is that, while amorphous solids are isotropic in nature (i.e., these exhibit same value of, some physical properties in all directions) the crystalline solids are anisotropic, (i.e., the values of some physical properties are different in different directions)., Refractive index and coefficient of thermal expansion are typical physical properties, which have different values when measured along different directions of a, given crystal. Another difference between amorphous and crystalline solids is, that while crystalline solids have a sharp or definite melting point, whereas the, amorphous solids do not have definite melting point, these melt over a range of, temperature., The crystalline solids can be further classified on the basis of nature of interaction between the constituent particles as discussed below., 6.2.2 Classification of Crystalline Solids, In crystalline solids the constituent particles are arranged in an ordered arrangement and are held together by different types of attractive forces. These forces, could be coulombic or electrostatic, covalent, metallic bonding or weak intermolecular in nature. The differences in the observed properties of the solids are due, to the differences in the type of forces between the constituting particles. The, types of forces binding the constituent particles can be used as a basis for classification of crystalline solids. On this basis, the crystalline solids can be classified, into four different types- ionic, molecular, covalent and metallic solids. The characteristics and the properties of different types of solids are compiled in Table, 6.1., , CHEMISTRY, , 177
Page 207 :
MODULE - 3, , The Solid State, , States of Matter, , Table 6.1: Characteristics and properties of different types of solids., Type of, Solid, , Constituent, Particles, , Nature of, interaction, between the, particles, , Appearance Melting, Point, , Ionic, , Ions, , Coulombic, , Hard and, brittle, , High, , Sodium chloride,, zinc sulphide, etc, , van der Waals, Dipole-dipole, , Soft, brittle, , low, , Iodine, naphthalene, water carbon dioxide., , Hard, , Very high Diamond, graphite,, silica, etc., , Notes, Molecular Molecules, Non polar, Polar, Covalent, , Atoms, , Covalent bonding, , Metallic, , Atoms, , Metallic bonding Hard and, malleable, , Variable, , Examples, , Copper, silver, etc., , Sodium chloride is an example of an ionic solid because in this case the sodium, ions and chloride ions are attracted to each other by electrostatic interactions., Iodine on the other hand is an example of a molecular solid because in this the, molecules are held together by weak van der Waals forces. Diamond, with strong, covalent bonds between the constituent carbon atoms is an example of covalent, solids while in metals a large number of positive cores of the atoms are held, together by a sea of electrons., , 6.3 PROPERTIES OF CRYSTALLINE SOLIDS, You are familiar with the following properties of solids on the basis of handling solids in day to day work., z, , Solids are rigid in nature and have well defined shapes, , z, , Solids have a definite volume irrespective of the size and shape of the, container in which they are placed, , z, , Solids are almost incompressible., , You are familiar with a number of crystalline solids like sugar, rock salt, alum,, gem stones, etc. You must have noticed that such solids have smooth surfaces., These are called ‘faces’ of the crystal. These faces are developed in the process of, crystal formation by ordered arrangements of the constituent particles. It is generally observed that the faces of crystals are developed unequally. The internal, angle between a pair of faces is called interfacial angle and is defined as the, angle between the normals to the intersecting faces. An important characteristic, of crystalline solids is that irrespective of the size and shape of the crystal of a, given substance, the interfacial angle between a pair of faces is always the same., 178, , CHEMISTRY
Page 208 :
MODULE - 3, , The Solid State, , This fact was stated by Steno as the law of constancy of interfacial angles (Fig., 6.2)., , Fig. 6.2 The constancy of interfacial angles, , States of Matter, , Notes, , 6.3.1 Melting Point of a Solid, What is the effect of heat on a solid? You would have observed that when a solid, is heated it becomes hot and eventually gets converted into a liquid. This process, of conversion of a solid to a liquid on heating is called melting. You would also, have observed that different solids need to be heated to different extents to convert them to liquids. The temperature at which a solid melts to give a liquid is, called its melting point. Every solid is characterized by a definite melting point., This in fact is a test of the purity of the solid. The melting point of a solid gives, us an idea about the nature of binding forces between constituent particles of the, solid. Solids like sodium chloride ( m.p = 1077 K ) have very high melting points, due to strong coulombic forces between the ions constituting it. On the other, hand molecular solids like naphthalene ( m.p. = 353 K) have low melting points., The effect of heat on a solid can be understood in terms of energy and motion of, the constituent particles. You are aware that in a solid the constituent particles, just vibrate about their mean position. As the heat is supplied to the solid, the, constituent particles gain energy and start vibrating more vigorously about their, equilibrium positions. As more and more heat is supplied, the energy keeps on, increasing and eventually it becomes greater than the binding forces between, them. As a consequence the solid is converted into a liquid., , INTEXT QUESTIONS 6.1, a) Differentiate between solid, liquid and gaseous state., b) How are solids classified on the basis of the intermolecular forces., c) What is Steno’s law of constancy of interfacial angles?, , 6.4 CRYSTAL LATTICE AND UNIT CELLS, You know, the crystalline solids have long-range order and the closely packed, constituent particles are arranged in an ordered three dimensional pattern. The, CHEMISTRY, , 179
Page 209 :
MODULE - 3, States of Matter, , The Solid State, , structure of the crystalline solids can be represented as an ordered three dimensional, arrangement of points. Here each point represents the location of a constituent, particle and is known as lattice point and such an arrangement is called a crystal, lattice or space lattice or simply a lattice., 6.4.1 Two Dimensional Lattice and Unit Cells, , Notes, , To understand the meaning of the term lattice, let us first start with a two, dimensional lattice. A two dimensional lattice has a set of points arranged in, a regular pattern on a plane or a surface (in two dimensions). One such lattice, is shown in Figure 6.4(a). The arrangement of lattice points is as shown in Fig., 6.4(a). When these lattice points are joined, the geometry of the lattice becomes, clear (Fig. 6.4(b). The entire pattern can be generated by repeating a set of four, points. On joining these points we get a unit cell of the lattice (shown in dark)., The unit cell of a two dimensional lattice is a parallelogram which is defined, in terms of two sides a and b and the angle between them γ. Only five types, of unit cells are possible in two dimensional lattice. These are shown in Fig., 6.4(b)., , b, , y, a, (b), , (a), , Fig. 6.3 (a) A two dimensional lattice (b) and its unit cell, , a, , a, , b, , y, , 90°, a, a=b, y = 90°, , 180, , 90°, a, a¹b, y = 90°, , a, a=b, y ¹ 90°, , CHEMISTRY
Page 210 :
MODULE - 3, , The Solid State, , States of Matter, , b, a, y, a, a¹b, y ¹ 90°, , 60°, a, a=b, y = 60°, , Notes, , Fig. 6.4: Five types of two dimensional unit cells, , 6.4.2 Three Dimensional Lattice and Unit Cells, In three dimensions, the crystal structure of a solid is represented as a three, dimensional array of lattice points. Remember that the lattice points represent the, positions of the constituent particles of the solid (Fig. 6.5(a))., , (a), , (b), , Fig. 6.5: Schematic representation of a three dimensional crystal lattice., , In a crystal lattice we can select a group of points which can be used to generate, the whole lattice. Such a group is called repeat unit or the unit cell of the crystal, lattice. The shaded region in the Fig. 6.5(a) represents a unit cell of the crystal, lattice. The unit cell is characterized by three distances along the three edges of, the lattice (a, b and c) and the angles between them (α, β and γ) as shown in the, Fig. 6.5(b). We can generate the whole crystal lattice by repeating the unit cell in, the three directions., On the basis of the external appearance the known crystals can be classified into, seven types. These are called crystal systems. In terms of the internal structure, also the crystal lattices contain only seven types of unit cells. The seven crystal, systems and the definition of their unit cells in terms of their unit distances and, the angles are compiled in Table 6.2. The seven simple unit cells are given in Fig., 6.6., , CHEMISTRY, , 181
Page 211 :
MODULE - 3, , The Solid State, , States of Matter, , Table 6.2: The seven crystal systems and their possible lattice types., Systems, , Notes, , Axes, , Angles, , Possible lattice, types, , Cubic, , a=b=c, , a = β = γ = 90°, , P, F, I, , Tetragonal, , a=b≠c, , a = β = γ = 90°, , P, I, , Orthorhombic, , a≠b=c, , a = β = γ = 90°, , P, F, I, C, , Rhombohedral, , a=b=c, , a = β = γ ≠ 90°, , P, , Hexagonal, , a=b≠c, , a = β = 90°; γ = 120°, , P, , Monoclinic, , a≠b=c, , a = γ = 90°; β ≠ 90°, , P, I, , Triclinic, , a≠b≠c, , a ≠ β ≠ γ ≠ 90°, , P, , * P= primitive, I= body centered, F= face centered and C= side centered, , The unit cell shown in Fig. 6.5 and the ones given in Fig..6.5 have the lattice, points at the corners only., , a, , c, , c, , a, a, , Cubic, , 120o, , b, , a, , a, , a, , Hexagonal, , Orthorhombic, , a, , a, b g, a, Triclinic, , c, , c, b, , b, a, Monoclinic, , b, , c, a, Tetragonal, , a, , a, , a, , a, , Rhombohedral, , Fig. 6.6 : The primitive unit cells; the relative dimensions of the three repeat distances (a, b, and c) and the angles between them (a, β and γ) are given in Table 6.2., , Such unit cells are called primitive (P) unit cells. Sometimes, the unit cell of a, crystal contains lattice point(s) in addition to the ones at the corners. A unit cell, containing a lattice point each at the centers of its faces in addition to the lattice, points at the corners is called a face centered (F) unit cell. On the other hand a, unit cell with lattice points at the center of the unit cell and at the corners is, called a body centered unit cell (I). In some cases, in addition to the lattice, points at the corners there are two lattice points located at the centers of any two, opposite faces. These are called as end centered (C) unit cells. The possible, lattice types in different crystal systems are also indicated in Table 6.2. The seven, crystal systems when combined with these possibilities give rise to 14 lattice, types. These are called Bravais lattices., , 182, , CHEMISTRY
Page 212 :
MODULE - 3, , The Solid State, , States of Matter, , 6.5 NUMBER OF ATOMS IN CUBIC UNIT CELLS, Of the seven crystal systems, let us discuss unit cells belonging to the cubic crystal system in somewhat details. As you can see from Table 6.2 that in the cubic, crystal system the three repeat distances are equal and all the three angles are, right angles. The unit cells of three possible lattice types viz., primitive or simple, cubic, body centered cubic and the face centered cubic, belonging to cubic crystal system are shown in Figure 6.7., , (a), , (b), , Notes, , (c), , Fig. 6.7: (a) primitive or simple (b) body centered and (c) face centered, cubic unit cells., , Number of atoms per unit cell, As you know that in unit cells the atoms can be on the corners, in the body, center and on face centers. All the atoms do not belong to a single unit cell., These are shared amongst different unit cells. It is important to know the number, of atoms per unit cell. Let us learn how to compute these for different cubic unit, cells., (a) Simple Cubic Unit Cell, The simple or primitive unit cell has the atoms, at the corners of the cube (Fig. 6.7 (a)). A lattice, point at the corner of the unit cell is shared by, eight unit cells as you can see from the encircled, atom in the Fig. 6.8. Therefore, the contribution, of an atom at the corner to the unit cell will be, 1/8. The number of atoms per unit cell can be, calculated as follows:, Number of corner atoms = 8, Contribution of each corner atom =, , Fig. 6.8: A corner lattice point is, shared by eight unit cells, , 1, 8, , The number of atoms in a simple cubic unit cell = 8 ×, , 1, =1, 8, , (b) Body Centered Cubic Unit Cell, A body centered cubic (bcc) unit cell has lattice points not only at the corners but, CHEMISTRY, , 183
Page 213 :
MODULE - 3, States of Matter, , The Solid State, , also at the center of the cube( Fig. 6.7 (b)). The atom in the center of the cube, belongs entirely to the unit cell, i.e., it is not shared by other unit cells. Each, corner atom, on the other hand, as in the case of simple cubic unit cell, is shared, by eight unit cells. Thus the number of atoms per unit cell can be calculated as, Number of corner atoms = 8, , Notes, , Contribution of each corner atom =, , 1, 8, , ∴ Contribution of all the corner atoms to the unit cell = 8 ×, , 1, =1, 8, , Number of atoms at the center of the cube = 1, Contribution to the unit cell = 1 ( as it is not shared), The number of atoms in a body centered cubic unit cell = 1 + 1 = 2, (c) Face Centered Cubic Unit Cell, A face centered cubic ( fcc) unit cell has atoms not only at the corners but also at, the center of each face. Thus it has eight lattice points at the corners and six at, the face centers (Fig. 6.7 (c)). A face centered lattice point is shared by two unit, cells, as shown in Fig. 6.9., , Fig. 6.9: A face centered lattice point is shared by two unit cells, , Number of corner atoms = 8, Contribution of each corner atom =, , 1, 8, , Contribution of all the corner atoms to the unit cell = 8 ×, , 1, =1, 8, , Number of atoms at the face center = 6, Contribution of each atom at the face centre =, , 1, 2, , Contribution of all the face centered atoms to the unit cell = 6 ×, , 1, =3, 2, , The number of atoms points in a face centered cubic unit cell = 1 + 3 = 4, , 184, , CHEMISTRY
Page 214 :
MODULE - 3, , The Solid State, , The number of atoms per unit cell in different types of cubic unit cells is given in, Table 6.3., , States of Matter, , Table. 6.3: Number of atoms per unit cell, S.No., , Type of unit cell, , No. of atoms per unit cell, , 1., , Simple cubic, , 1, , 2., , Body centered cubic, , 2, , 3., , Face centered cubic, , 4, , Notes, , 6.6 CALCULATION OF DENSITY OF UNIT CELL, Density =, , Mass, Volume, , (i) Volume of Unit cell, If the edge length of the cubic unit cell is ‘a’ its volume is a3, (ii) Mass of the Unit cell, Let the molar mass of substance = M, ∴ Mass of one atom or molecule =, , M, NA, , Where NA = Avogadro’s constant., Let the number of atoms or molecules of the substance present per unit cell = z, (See table 6.3 for values of z for different type of cubic unit cells), ∴ Mass of unit cell = ( number of atoms/molecules present per unit cell ), , × ( mass of one atom/molecule ), , =, , z⋅M, NA, , (iii) Density, Density =, , d=, , Mass of one unit cell, Volume of one unit cell, , z⋅M, a3 ⋅ N A, , Density of the unit of cell of a substance is the same as the density of the, substance. In case of ionic substances, the formula unit is taken as one particle., (For example, formula unit of NaCl = 1Na+ + 1Cl– ions; of Na2SO4 = 2Na+, + 1SO42– ions)., , CHEMISTRY, , 185
Page 215 :
MODULE - 3, States of Matter, , The Solid State, , The above relation can be used to calculate z and hence the nature of cubic lattice, if d and M are known or M if d and z are known, Example. The unit cell of a metallic element is face centred cubic and the side, of the cube is 540.2 pm. Calculate the density of the metal in gcm–3 if its relative, atomic mass is 202.4., , Notes, , Solution, , d=, , z⋅M, a3 ⋅ N A, , Given:, Side of the cube = a = 540.2 pm = 540.2 × 10–10 cm, Number of atoms per unit cell of a face-centred cubic unit cell = z = 4, Molar mass of the element, M = 202.4 g mol–1, Putting these values in the above relation, d=, , 4 × 202.4 g mol−1, (540.2 × 10−10 cm)3 × (6.022 × 10−23 mol−1 ), , = 8.53 g cm −3, , 6.7 CLOSE PACKED STRUCTURES OF SOLIDS, In the process of the formation of a crystal the constituent particles get packed, quite closely. The crystal structures of the solids can be described in terms of a, close packing of identical spheres as shown in Fig. 6.10. These are held together, by forces of attraction. Let us learn about the possible close packed structures of, solids and their significance., , Fig. 6.10 : Arrangement of identical spheres in one dimension, , A linear horizontal arrangement of identical spheres in one dimension forms a, row (Fig. 6.10). A two dimensional close packed structure can be obtained by, arranging a number of such rows to form a layer. This can be done in two possible ways. In one of these, we can place these rows in such a way that these are, aligned as shown in (Fig. 6.11 (a)). In such an arrangement each sphere is in, contact with four other spheres. This arrangement in two dimensions is called, square close packing., 186, , CHEMISTRY
Page 216 :
MODULE - 3, , The Solid State, , States of Matter, , Notes, (a), , (b), , Fig. 6.11 : (a) Square close packing and (b) hexagonal close packing of identical, spheres in two dimensions, , In the other way we can place the spheres of the second row in the depressions, of the first row and so on and so forth (Fig. 6.11(b)). You may notice that, in such an arrangement each sphere is in contact with six other spheres. Such, an arrangement in two dimensions is called hexagonal close packing. In such, a packing, the spheres of the third row are aligned with the first row. You may, also have noticed that in the hexagonal close packed the spheres are more, efficiently packed. In Fig. 6.11 an equal number of identical spheres are arranged, in two different types of packing., A three dimensional structure can be generated by placing such two dimensional, layers on top of each other. Before we move on to the three dimensional packing, let us look at the hexagonal close packed layer some what more closely (Fig., 6.12)., You may note from Fig. 6.12 that in a hexagonal close packed layer there are, some unoccupied spaces or voids. These are triangular in shape and are called, trigonal voids. You can further note that there are two types of triangular voids,, one with the apex pointing upwards and the other with the apex pointing downwards. Let us call these as X type and Y type voids respectively as marked in the, Fig. 6.12., IIIrd row, IInd row, , Ist row, , Fig. 6.12 : A hexagonal Close Packed layer showing two types of triangular voids., , Close Packed Structures in three dimensions, Let us take a hexagonal close packed layer and call it A layer and place another, hexagonal close-packed layer (called the B layer) on it. There are two possibilities., CHEMISTRY, , 187
Page 217 :
MODULE - 3, States of Matter, , Notes, , The Solid State, , 1. In one, we can place the second layer in such a way that the spheres of the, second layer come exactly on top of the first layer., 2. In other, the spheres of the second layer are in such a way that these are on, the depressions of the first layer. The first possibility is similar to square, close packing discussed above and is accompanied by wastage of space. In, the second possibility when we place the second layer into the voids of the, first layer, the spheres of the second layer can occupy either the X or Y type, trigonal voids but not both. You may verify this by using coins of same, denomination. You would observe that when you place a coin on the trigonal, void of a given type, the other type of void becomes unavailable for placing, the next coin (Fig. 6.13)., , Fig. 6.13 : Two layers of close packed spheres , the second layer occupies only one, type (either X or Y ) of triangular voids in the first layer., , In this process, the sphere of second layer covers the trigonal voids of the first, layer. It results into voids with four spheres around it, as shown in Fig. 6.14(a)., Such a void is called a tetrahedral void since the four spheres surrounding it are, arranged on the corners of a regular tetrahedron, Fig. 6.14(b). Similarly, the trigonal, voids of the second layer will be placed over the spheres of the first layer and give, rise to tetrahedral voids., , (a), , (b), Fig. 6.14 : A tetrahedral void, , In a yet another possibility, the trigonal voids of the first layer have another, trigonal void of the opposite type (X type over Y and Y type over X type) from, the second layer over it. This generates a void which is surrounded by six spheres,, Fig..6.15 (a). Such a void is called an octahedral void because the six spheres, 188, , CHEMISTRY
Page 218 :
MODULE - 3, , The Solid State, , surrounding the void lie at the corners of a regular octahedron, Fig..6.15 (b)., , States of Matter, , Notes, (a), Fig. 6.15 :, , (b), An octahedral void, , A closer look at the second layer reveals that it has a series of regularly placed, tetrahedral and octahedral voids marked as ‘t’ and ‘o’ respectively in Fig. 6.16., , o, t, , Fig. 6.16 : The top view of the second layer showing the tetrahedral, and octahedral voids., , Now, when we place the third layer over the second layer, again there are two, possibilities i.e., either the tetrahedral or the octahedral voids of the second layer, are occupied. Let us take these two possibilities. If the tetrahedral voids of the, second layer are occupied then the spheres in the third layer would be exactly on, top (i.e., vertically aligned) of the first or A layer The next layer ( 4th layer), which is then placed would align with the B layer. In other words, every alternate, layer will be vertically aligned. This is called AB AB …. pattern or AB AB …., repeat. On the other hand if the octahedral voids of the second layer are occupied, the third layer is different from both the first as well as the second layer. It, is called the C layer. In this case the next layer, i.e., the fourth layer, howsoever, it is placed will be aligned with the first layer. This is called ABC ABC …. pattern, or ABC ABC.. …. repeat. In three dimensional set up the AB AB …. pattern or, repeat is called hexagonal closed packing ( hcp) (Fig. 6.10 (c) ) while the ABC, ABC …. pattern or repeat is called cubic closed packing (ccp) (Fig. 6.17 (a))., , CHEMISTRY, , 189
Page 219 :
MODULE - 3, , The Solid State, , States of Matter, A Layer, A-Layer, C Layer, , A Layer, B-Layer, C Layer, , B Layer, , Notes, , A-Layer, , B Layer, , A Layer, A Layer, , (a), , (b), , (c), , Fig. 6.17 : (a) Cubic closed packing (ccp) as a result of ABC pattern of close packed spheres;, (b) the layers in (a) tilted and brought closer to show fcc arrangement, (c) hexagonal closed packing (hcp) as a result of ABAB pattern of close packed, spheres., , This process continues to generate the overall three dimensional packed structure. These three dimensional structures contain a large number of tetrahedral, and octahedral voids. In general there is one octahedral and two tetrahedral voids, per atom in the close packed structure. These voids are also called as interstices., As mentioned earlier, the identical spheres represent the positions of only one, kind of atoms or ions in a crystal structure. Other kind of atoms or ions occupy, these interstices or voids., In the close packed structures (hcp and ccp) discussed above, each sphere is in, contact with six spheres in its own layer ( as shown in Fig. 6.12) and is in contact, with three spheres each of the layer immediately above and immediately below it., That is, each sphere is in contact with a total of twelve spheres. This number of, nearest neighbor is called its coordination number. The particles occupying the, interstices or the voids will have a coordination number depending on the nature, of the void. For example an ion in a tetrahedral void will be in contact with four, neighbors i.e., would have a coordination number of four. Similarly the atom or, ion in an octahedral void would have a coordination number of six., , INTEXT QUESTIONS 6.2, (a) What is the difference between the square close packed and hexagonal close, packed structures?, (b) Which of the above two, is more efficient way of packing?, (c) Clearly differentiate between, trigonal, tetrahedral and octahedral voids., , 190, , CHEMISTRY
Page 220 :
MODULE - 3, , The Solid State, , States of Matter, , 6.8 PACKING EFFICIENCY, In all closed packed structures there are always some voids or empty spaces., The percentage of the total space that is filled by the constituent particles is, called the packing efficiency. It is calculated from the following relation:, Packing efficiency =, , filled space, × 100%, total space, , Notes, , It depends upon the nature of crystal lattice. For the three types of cubic lattices,, the calculations are given below:, (1) Packing Efficiency of Simple Cubic Lattice, In simple cubic lattice, the constituent particles occupy only the corner positions, of the cubic unit cell. These particles touch one another along the edge of the, cube as shown in the Figure 6.18. Let a be the edge length of the cube and, r the radius of the particle then, a = 2r, C, , B, , H, , A, , F, , C, , E, , D, , Fig. 6.18: Simple cubic unit cell, , The volume of the cube = (edge length/side)3 = a3 = (2r)3 = 8r3, No. of constituent particles present in each unit cell = 1, The volume of the filled space = volume of 1 particle =, , 4 3, πr, 3, , filled space, × 100, total space, 4 3, πr, π, 3, =, × 100 = × 100, 3, 6, 8r, , Packing efficiency =, , = 52.36% = 52.4%, , CHEMISTRY, , 191
Page 221 :
MODULE - 3, , The Solid State, , States of Matter, , (2) Packing Efficiency of Body Centred Cubic Lattice, G, , B, , H, , A, , Notes, , c, F, E, , c, C, , b, a, , D, , Fig. 6.19. Body centred cubic until cell., , The body centred cubic unit cell has constitutent particles present at all its, corners as well as at its body centre. The particle at the body-centre touches, the particle at corner positions. Thus, the particles are in contact along the bodydiagonal of the cube. Consider the body-diagonal AF along which the contact, between the central particles with those at corners A and F has been shown in, Fig. 6.19., ∴ Length of body-diagonal AF = c = 4 r, , (6.1), , (Whole of the central particle (2r) and one-half of each of the two corner particle, (r + r) occupy the body-diagonal), Length of the body-diagonal, In the triangle EFD on the face CDEF, EF ⊥ ED., ∴, , FD2 = EF2 + ED2, b 2 = a 2 + a 2 = 2a 2, , Where a is the edge-length or side of the cube., In the triangle AFD, AD ⊥ FD, ∴, , AF2 = AD2 + FD2, c 2 = a 2 + b 2 = a 2 + 2a 2 = 3a 2, , ∴, , c = 3a, , (6.2), , But c = 4r, , 192, , CHEMISTRY
Page 222 :
MODULE - 3, , The Solid State, , States of Matter, , ∴, , 4r, a=, 3, , (6.3), , The number of constituent particles in a body-centred cubic unit cell = 2, 4, ∴ Volume of filled space = 2 × π r 3, 3, , ⎛ 4r ⎞, Volume of the cubic unit cell = a3 = ⎜, ⎟, ⎝ 3⎠, , (6.4), Notes, 3, , (6.5), , 4, 2 × π r3, filled space, 3, Packing efficiency =, × 100 =, × 100, 3, total space, ⎛ 4r ⎞, ⎜, ⎟, ⎝ 3⎠, 8 3, πr, 3π, 3, =, ×100 =, × 100 = 68.0%, 64 3, 8, r, 3 3, , (3) Packing Efficiency of CCP and HCP Lattices, Cubic close packed or face centred cubic (FCC) and hexagonal close packed, lattices have equal packing efficiency. Packing efficiency of face centred cubic, (FCC) or cubic close packing (CCP) unit cell can be calculated with the help, of Figure 6.20. Let the edge length or the side of the cubic unit cell be a and, its face diagonal AC be b. In the triangle ABC, AB ⊥ BC, therefore,, AC2 = AB2 + BC2, b 2 = a 2 + a 2 = 2a 2, , b = 2a., , or, , (6.6), , G, , B, A, , H, b, , C, , F, E, , D, , Fig. 6.20: Face centred cubic or cubic close packing unit cell, , CHEMISTRY, , 193
Page 223 :
MODULE - 3, States of Matter, , The Solid State, , Unit cell of fcc (or ccp) lattice has constituent particles at all the corners and, at the centre of each face., The particle of face-centre touches the particles occupying the corner positions, of the same face., ∴ Length of the face diagonal AC = b = 4r, , Notes, , (6.7), , Where r is the radius of the constitutent particle (whole of central particle, 2r,, and one-half of each of the two corner particles, r + r), From equations 1 and 2, , b = 4r = 2a, a=, , or, , 4r, = 2 2r, 2, , (6.8), , The number of constituent particles in a face centred cubic unit cell = 4, 4, ∴ The Volume of filled space = 4 × π r 3, 3, , Volume of the cubic unit cell = (side)3 = a3 = (2 2r )3, 4, 4 × π r3, filled space, 3, Packing efficiency =, × 100 =, ×100, total space, (2 2r )3, 16, π, π, 3, =, × 100 =, = 74%, 16 2, 3 2, Packing efficiencies of the three types of cubic lattices are summarized in the, table 6.4., Table 6.4: Packing efficiency of cubic unit cells, , S.No., , 194, , Lattice, , Packing Efficiency %, , 1., , Simple Cubic, , 52.4%, , 2., , Body Centred Cubic, , 68.0%, , 3., , Face Centred Cubic or, Cubic close packing, , 74.0%, , CHEMISTRY
Page 224 :
MODULE - 3, , The Solid State, , States of Matter, , 6.9 STRUCTURES OF IONIC SOLIDS, In case of ionic solids that consist of ions of different sizes, we need to specify, the positions of both the cations as well as the anions in the crystal lattice. Therefore, structure adopted by an ionic solid depends on the relative sizes of the two, ions. In fact it depends on the ratios of their radii (r+/r-) called radius ratio. Here, r+ is the radius of the cation and r- is that of the anion. The radius ratios and the, corresponding structures are compiled in Table 6.5., , Notes, , Table 6.5 : The radius ratios (r + /r–) and the corresponding structures, Radius ratio (r+/r–), , Coordination number, , Structure adopted, , 0.225 – 0.414, , 4, , Tetrahedral, , 0.414 – 0.732, , 6, , Octahedral, , 0.732 – 0.91, , 8, , Body centered cubic, , >=1.00, , 12, , Cubic Close Packed structure, , The common ionic compounds have the general formulae as MX, MX2, and, MX3, where M represents the metal ion and X denotes the anion. We would, discuss the structures of some ionic compounds of MX and MX2 types., 6.9.1 Structures of the Ionic Compounds of MX Type, For the MX type of ionic compounds three types of structures are commonly, observed. These are sodium chloride, zinc sulphide and caesium chloride structures. Let us discuss these in some details., (a) Caesium Chloride Structure, In CsCl the cation and the anions are, of comparable sizes (the radius ratio, = 0.93) and has a bcc structure in, which each ion is surrounded by 8, ions of opposite type. The Cs+ ions, is in the body center position and, eight Cl– ions are located at the, corners (Fig. 6.21) of the cube. Thus, it has a coordination number of 8., , Caesium Ion, , Chloride Ions, , Fig. 6.21: Caesium chloride structure, , (b) Sodium Chloride Structure, In case of NaCl the anion (Cl–) is much larger than the cation (Na+). It has a, radius ratio of 0.52. According to Table 3.3 it should have an octahedral arrangement. In sodium chloride the( Cl–) form a ccp (or fcc) structure and the, sodium ion occupy the octahedral voids. You may visualise the structure having, CHEMISTRY, , 195
Page 225 :
MODULE - 3, States of Matter, , The Solid State, , chloride ions at the corners and the face centers and the sodium ions at the edge, centers and in the middle of the cube (Fig. 6.22)., , Notes, , Fig. 6.22 : Sodium chloride structure., , (c) Zinc Sulphide Structure, In case of zinc sulphide the radius ratio is just = 0.40. According to Table 3.3, it should have an tetrahedral arrangement. In Zinc sulphide structure, the, sulphide ions are arranged in a ccp structure. The zinc ions are located at the, corners of a tetrahedron, which lies inside the cube as shown in the Fig. 6.23., These occupy alternate tetrahedral voids., , Fig. 6.23 : Zinc Sulphide structure., , 6.9.2 Structure of Ionic Compounds of MX2 type, (a) Calcium fluoride or fluorite structure, In this structure the Ca2+ ions form a fcc arrangement and the fluoride ions are, located in the tetrahedral voids (Fig. 6.24)., 196, , CHEMISTRY
Page 226 :
The Solid State, , MODULE - 3, States of Matter, , Notes, , Fig. 6.24 : Calcium fluoride or Fluorite structure; calcium ions occupy the corners of the, cube and face centers The F- ions are on the corners of the smaller cube which, dipict the positions of tetrahedral void., , (b) Antifluorite Structure, Some of the ionic compounds like Na2O have antifluorite structure. In this structure the positions of cations and the anions in fluorite structures are interchanged., That is why it is called antifluorite structure. In Na2O the oxide ions form the, ccp and the sodium ions occupy the tetrahedral voids (Fig. 6.25)., , Fig. 6.25: Antifluorite structure adopted by Na2O; The oxide ions occupy the corners, of the cube and face centers and the Na+ ions ( shown in black ) are on, the corners of the smaller cube., , 6.10 DEFECTS IN IONIC CRYSTALS, You have learnt that in a crystalline solid the constituent particles are arranged in a ordered three dimensional network. However, in actual crystals, such a perfect order is not there. Every crystal has some deviations from the, perfect order. These deviations are called imperfections or defects. These, defects can be broadly grouped into two types. These are stoichiometric and, CHEMISTRY, , 197
Page 227 :
MODULE - 3, States of Matter, , Notes, , The Solid State, , non-stoichiometric defects depending on whether or not these disturb the, stoichiometry of the crystalline material. Here, we would deal only with stoichiometric defects. In such compounds the number of positive and negative, ions are in stoichiometric proportions. There are two kinds of stoichiometric, defects, these are, z, , Schottky defects, , z, , Frenkel defects, , (a) Schottky defects : This type of defect are due to the absence of some, positive and negative ions from their positions. These unoccupied lattice sites, are called holes. Such defects are found in ionic compounds in which the, positive and negative ions are of similar size e.g., NaCl and CsCl. The number of missing positive and negative ions is equal. The presence of Schottky, defects decreases the density of the crystal [Fig. 6.26(a)]., (b) Frenkel defects : This type of defect arise when some ions move from, their lattice positions and occupy interstitial sites. The interstitial sites refer, to the positions in between the ions. When the ion leaves its lattice site a hole, is created there. ZnS and AgBr are examples of ionic compounds showing, Frenkel defects. In these ionic compounds the positive and negative ions are, of quite different sizes. Generally the positive ions leave their lattice positions, as these are smaller and can accommodate themselves in the interstitial, sites. The Frenkel defects do not change the density of the solids [Fig. 6.26(b)]., A–, , B–, , A–, , –, , –, , B–, , A–, , A–, , –, , –, , B–, , A–, , B–, , A–, , A, –, , B, , A, , A–, –, , B, , B, , A–, A, , –, , –, , B, , (a), , B–, A, , –, , –, , –, , –, , B, , B, , A, , A–, , A–, , B–, , A–, , B–, , A–, , –, , A–, , B, , –, , A–, , B, , –, , B, , B, , B, , B, , –, , (b), , Fig. 6.26: Stoichiometric defects a) Schottky and b) Frenkel defects, , These defects cause the crystal to conduct electricity to some extent. The, conduction is due to the movement of ions into the holes. When an ion moves, into a hole it creates a new hole, which in turn is occupied by another ion, and the, process continues., , 198, , CHEMISTRY
Page 228 :
The Solid State, , MODULE - 3, States of Matter, , INTEXT QUESTIONS 6.3, (a) What do you understand by crystal lattice?, b), , What is a unit cell ?, , c), , How many atoms are there in a fcc unit cell?, , Notes, , 6.11 ELECTRICAL PROPERTIES, Conductance of electricity is an important property of a substance. Solids show, a very wide range of conductivities from a high of 107 to a low of 10–20 s m–, 1, thus spanning 27 orders of magnitude. Based upon their ability to conduct, electricity, solids may be classified into three categories; conductors, insulators, and semiconductors., 6.11.1 Conductors' Insulators and Semiconductors, (i) Conductors, These are the solids with conductivities ranging from 104 to 107 S m–1. Metals, conduct electricity through movement of their electrons and are called electronic, conductors. Ionic solids conduct electricity when in molten state or dissolved, in water, through movement of their ions. They are called electrolytic conductors., (ii) Insulators, These are the solids with extremely low conductivities ranging from 10–20 to, 10–10 S m–1. Insulators are used to provide protective covering on conductors., (iii) Semiconductors, These are the solids with intermediate conductivities ranging from 10–6 to 104, S m–1. Although semiconductors have low conductivities, they find vide, applications in solid state devices like diodes and transistors. Their conductivities, can be modified by introduction of a suitable impurity., 6.11.2 Conduction of Electricity in Metals, Metals conduct electricity through movement of their electrons. In unit 5 you, have learnt that when two atoms come closer, their atomic orbitals overlap and, they form an equal number of molecular orbitals. One-half of these have lower, energy while the other half have higher energy than the energy of the atomic, orbitals. As the number of molecular orbitals increases, the energy-separation, between them decreases., CHEMISTRY, , 199
Page 229 :
MODULE - 3, States of Matter, , Notes, , The Solid State, , In a piece of metal, the number of metal atoms is very large and so is the number, of their valence orbitals. This results in formation of an equally large number, of molecular orbitals which are so close to one-another that they form a, continuous band. The band in which the valence electrons are present is called, valence band. Electrons present in this band are strongly bound to the nucleus, and cannot conduct electricity. The band formed by vacant molecular orbitals, of higher energy is called conduction band. When electrons reach conduction, band from valence band on excitation, they become loosely bound to the nucleus, and can conduct electricity by moving under the influence of an electric field., Such electrons are also called free electrons. Conductivity of a solid depends, upon how easy or difficult it is for the valence electrons to jump to the, conduction band. See figure 6.27., Conduction band, Empty, band, , Emergy, , Forbidden band, (Large energy gap), , Empty, band, Small energy gap, , Filled, band, Partially, filled, band, , Overlapping, bands, Metal, (a), , Insulator, (b), , Semiconductor, (c), , Fig. 6.27: Valence and conduction bands in (a) metals, (b) insulators and, (c) semi conductors., , (i) In conductors either the valence band is only partially filled or it overlaps, a vacant conduction band of slightly higher energy. In both the cases its, electrons can easily flow under the influence of electric field and the solid, behaves as a conductor (Fig. 6.27(a)), (ii) In insulators the gap between the valence band and conduction band is, large. Due to this the valence electrons cannot jump to the conduction band, and conduct electricity (Fig. 6.27(b)), (iii) In semiconductors the gap between the valence band and nearest, conduction band is small (Fig. 6.27(c)). On applying the electric field, some, electrons can jump to the conduction band and provide low conductivity., On increasing the temperature more electrons can jump to the conduction, band and the conductivity increases. Silicon and germanium show this type, of behaviour. They are called intrinsic semiconductors., 200, , CHEMISTRY
Page 230 :
MODULE - 3, , The Solid State, , States of Matter, , 6.11.3 Doping of Semiconductors, Conductivities of silicon and germanium are too low to be put to any practical, use. Their conductivities can be improved by introduction of controlled, quantities of impurities which are either electron-rich or electron-deficit with, respect to these elements. This process is known as doping., (a) Doping with electron–rich impurities, , Notes, Silicon and germanium both belong to group 14 of the periodic table and have, 4 valence electrons each. In their crystal lattice each silicon (or germanium) atom, forms four covalent bonds with its neighbours (Fig. 6.23(a)) when it is doped, with a group 15 element like As or P, whose each atom carries 5 valence electrons, which is one more than Si. After sharing its four electrons with four neighbouring, Si atoms, it has 9 electrons in its valence shell (Fig. 6.28(b)) which is highly, unstable electronic configuration. The ninth electron being highly unstable roams, freely in the whole of the crystal lattice randomly. In the presence of an electric, field this electron (mobile electron) moves from negative to positive terminal and, increases the conductivity of Si (or Ge). Since the increased conductivity of Si, (or Ge) is due to negatively charged mobile electrons, the Si doped with electron, rich impurity becomes a n-type semiconductor., , :, , :, , :, , :, , :, , :, , :, :, , :, , :, , :, :, , :, , :, :, , :, , B, , :, :, , :, , :, :, , :, , :, :, , :, , :, , :, , :, :, , :, , :, , :, , :, , n-type, (b), , :, , :, :, , :, , :, , :, , :, , :, , Perfect crystal, (a), , :, , :, , :, , :, , :, , :, , :, , :, , :, , :, , :, , :, :, , :, , :, , :, , :, , :, , : As :, :, , :, :, , :, , :, , :, , :, , :, , :, , :, :, , :, :, , :, , :, , :, :, , :, , :, :, , :, , :, , :, , Positive hole, (no electron), , Mobile electron, , Silicon atom, , p-type, (c), , Fig. 6.28: Creation of n-type and p-type semiconductors, , (b) Doping with electron-deficit impurities. When Si (or Ge) is doped with, a group 13 element like B or Al containing 3 valence electrons (1 electron less, than Si/Ge), this results in creation of one-electron vacancy in the structure, which is called an electron hole (Fig. 6.28(c)). An electron from its neightbouring, atom can come and occupy it, leaving a hole at its original position. Electrons, can randomly occupy holes and the hole would appear to move randomly. On, applying an electric field, the electrons move from negative to positive terminal, and the hole would appear move in the opposite direction, i.e., from positive, to negative terminal and would behave as if it is positively charged. Such, semiconductors are called p-type semiconductors. The movement of electrons, and the hole increases the conductivity of Si. Semiconductors like Si, doped, , CHEMISTRY, , 201
Page 231 :
MODULE - 3, States of Matter, , The Solid State, , with electron-deficit or electron-rich impurities are called extrinsic, semiconductors., Applications of n-type and p-type semiconductors, Due to their special properties, n-and p-type semiconductors find several, applications., , Notes, , (i) Diodes: Diode is a combination of a n-type and a p-type semiconductor., Diodes are used as rectifier to convert an AC signal to a DC signal., (ii) Transistors. Transistors are made by combination of 3 layers of, semiconductors. Transistor of n-p-n type is made by sandwiching a layer, of p-type semiconductor between two layers of n-type semiconductors and, of p-n-p type by sandwiching a layer of n-type semiconductors between, two layers of p-type semiconductor. Transistors are used as detectors and, amplifiers of radio or audio frequency signals. They are used in circuits of, solid state devices., (iii) Solar cells. Solar cells are photo diodes which have a special property of, emitting electrons when sunlight falls on them. They are used to convert, solar energy into electricity., , 6.12 MAGNETIC PROPERTIES, All substances are affected (attracted or repelled) by a magnetic field. Magnetic, properties present in any substance are due to the electrons present in it. Each, electron in an atom behaves like a tiny magnet. Electrons are negatively charged, particles. When an electron revolves around nucleus and spins about its own, axis, two types of magnetic moments are created – orbital magnetic moment, due to its revolution around the nucleus and spin magnetic moment due to its, spin. Overall magnetic properties of a substance depend upon the orientation, of these tiny magnets., On the basis of magnetic properties, all substances can be classified into five, categories:, (i) paramagnetic, (ii) diamagnetic, (iii) ferromagnetic, (iv) antiferromagnetic and, (v) ferrimagnetic, 6.12.1 Paramagnetic Substances, On placing these substances in a magnetic field, these are weakly attracted by, it. O2, Cu2+, Fe3+ and Cr3t are some examples of paramagnetic substances, Paramagnetism is due to the presence of one or more unpaired electrons in an, atom, molecule or ion., , 202, , CHEMISTRY
Page 232 :
The Solid State, , 6.12.2 Diamagnetic Substances, , MODULE - 3, States of Matter, , When placed in a magnetic field, diamagnetic substances are weakly repelled, by it. H2O, NaCl and C6H6 are some examples of such substances. Diamagnetism, is shown by substances in which all the electrons are paired., 6.12.3 Ferromagnetic Substances, When placed in a magnetic field, ferromagnetic substances are strongly attracted, by it. Fe, Ni, Co, Gd, MnAs, CrBr3 and CrO2 are such substances. These, substances can be permanently magnetized. In solid state, the metal ions of, ferromagnetic substances are grouped together into small regions called, domains. In each domain, the individual magnetic moments of the metal ions, are directed in the same direction and they add up. As a result, each domain, acts as a tiny magnet. Ordinarily, these domains are randomly oriented which, cancels out their magnetic moment. When, , Notes, , (a), , (b), , (c), , Fig. 6.29 Arrangement of magnetic moments of domains in (a) ferromagnetic, (b) anti, ferromagnetic and (c) ferrimagnetic substances, , placed in a magnetic field, all the domains get oriented in the direction of the, magnetic field. This adds up their magnetic moments and makes them strong, magnets (Fig. 6.29(a)). They lose their ferromagnetism on being given mechanical, jerks or on heating above a certain temperature, called Curie temperature they, become paramagnetic., 6.12.4 Antiferromagnetic Substances, Some substance that have domains in them like ferromagnetic substances but, their domains are oppositely oriented and cancel out the magnetic moments of, each other (Fig. 6.29 (b)) are caled antiferro magnetic substances. FeO, MnCl2,, MnO, Mn2O3 and MnO2 are examples of antiferromagnetic substances. They, also become paramagnetic on heating above a certain temperature., 6.12.5 Ferrimagnetic Substances., Some substances like Fe3O4 (magnetite) and ferrites (MFe2O4 where M is a, bivalent cation like Cu2+, Zn2+) show an intermediate behavior between that, CHEMISTRY, , 203
Page 233 :
MODULE - 3, States of Matter, , The Solid State, , of ferromagnetic and antiferromagnetic substances. These substances are quite, strongly attracted by magnetic field as compared to paramagnetic substances, but weakly as compared to ferromagnetic substances. Their domains are aligned, in parallel and antiparallel directions in unequal numbers (Fig. 6.29(c)). They, also become paramagnetic on heating above a certain temperature., , Notes, , WHAT YOU HAVE LEARNT, , 204, , z, , In solid state the constituent particles are arranged in a closely packed ordered arrangement with almost no free space. These are held together by, strong forces of attraction and vibrate about their fixed positions. Solids are, incompressible and rigid and have definite shapes., , z, , Solids are classified into amorphous and crystalline solids. The crystalline, solids have long range order while amorphous solids have only short range, order., , z, , The crystalline solids can be classified into four different types- ionic, molecular, covalent and metallic solids on the basis of nature of forces of, attraction between the constituent particles., , z, , The temperature at which a solid melts to give a liquid is called its melting, point., , z, , The three dimensional internal structure of a crystalline solid can be represented in terms of a crystal lattice in which the location of each constituent, particle is indicated by a point., , z, , The whole crystal lattice can be generated by moving the unit cell in the, three directions., , z, , The crystal structures of the solids can be described in terms of a closepacking of identical spheres., , z, , In three dimensions there are two ways of packing identical spheres. These, are hexagonal closed packing (hcp) and cubic closed packing (ccp). The hcp, arrangement is obtained by ABAB repeat of the two dimensional layers, whereas the ccp arrangement is obtained by ABCABC repeat., , z, , On the basis of the external appearance the known crystals can be classified, into seven types called crystal systems., , z, , The unit cells of cubic crystal system has three possible lattice types. These, are simple cubic, body centered cubic and the face centered cubic., , z, , The atoms at the corner of a cubic unit cell is shared by eight unit cells while, a face centered atom is shared by two unit cells. The atom at the body center,, on the other hand is exclusive to the unit cell as it is not shared., , CHEMISTRY
Page 234 :
The Solid State, z, , The number of atoms per unit cell for the simple cubic, bcc and fcc unit cells, are 1,2 and 4 respectively., , z, , The structure adopted by an ionic solid depends on the ratios of their radii, (r+/r-), called radius ratio., , z, , The structures of some simple ionic solids can be described in terms of ccp, of one type of ions and the other ions occupying the voids., , z, , Actual crystals have some kind of imperfections in their internal structure., These are called defects., , z, , There are two types of defects called stoichiometric and non-stoichiometric, defects depending on whether or not these disturb the stoichiometry of the, crystalline material., , z, , There are two kinds of stoichiometric defects, these are called Schottky, defects and Frenkel defects., , z, , Solid can be classified as conductors, insulators and semiconductors on the, basis of their electrical conductivities., , z, , Electrical properties of solids can be explained with the help of band theory., , z, , On the basis of their interaction with external magnetic field, solids can be, classified as paramagnetic, diamagnetic, ferromagnetic, ferrimagnetic and, antiferromagnetic substances., , MODULE - 3, States of Matter, , Notes, , TERMINAL EXERCISES, 1. Outline the differences between a crystalline and an amorphous solid., 2. How can you classify solids on the basis of the nature of the forces between, the constituent particles?, 3. What do you understand by the melting point of a solid ? What information, does it provide about the nature of interaction between the constituent particles, of the solids?, 4. What do you understand by coordination number? What would be the coordination number of an ion occupying an octahedral void.?, 5. Explain the following with the help of suitable examples., (a) Schottky defect, (b) Frankel defect, 6. Explain why a particular solid behaves as conductor or semiconductor or, insulator on the basis of band theory., 7. What are (i) paramagnetic (ii) diamagnetic and (iii) ferromagnetic substances?, , CHEMISTRY, , 205
Page 235 :
MODULE - 3, , The Solid State, , States of Matter, , ANSWERS TO INTEXT QUESTIONS, 6.1, 1. Solids have definite shape and definite volume., Notes, , Liquids have indefinite shape but define volume., Gases have indefinite shape and indefinite volume., 2. Coulombic forces, dipole-dipole attractions, covalent bonding and metallic, bonding., 3. Irrespective of the size and shape of the crystal of a substance, the interfacial, angle between a pair of faces is always the same, 6.2, 1. Refer to section 6.7., 2. Hexagonal close packed., 3. Refer to sections 6.7., 6.3, 1. Ordered three dimensional arrangement of points representing the location, of constituent particles., 2. A select group of points which can be used generate the whole lattice. Unit, cell is characterised by three edges of the lattice and angles between them., 3. Four., , 206, , CHEMISTRY
Page 236 :
MODULE - 3, , Solutions, , States of matter, , 7, Notes, , SOLUTIONS, , You know that when sugar or salt is added to water, it dissolves. The resulting, mixture is called a solution. Solutions play an important role in our life. In industry,, solutions of various substances are used to carry out a large number of chemical, reactions., Study of solutions of various substances is very interesting., In this lesson, let us learn about the various components of a solution and the ways in, which concentration of solutions is expressed. We shall also learn about some properties, of solutions which are dependent only on the number of solute particles. (you will learn, about solute in this lesson), , OBJECTIVES, After reading this lesson, you will be able to:, z, , identify the components of different types of solution;, , z, , express the concentration of solutions in different ways;, , z, , list different types of solutions;, , z, , state Henry’s law;, , z, , define vapour pressure;, , z, , state and explain Raoult’s law for solutions;, , z, , define ideal solutions;, , z, , give reasons for non-ideal behaviour of solutions;, , z, , state reasons for positive and negative deviations from ideal behaviour;, , z, , explain the significance of colligative properties;, , CHEMISTRY, , 207
Page 237 :
MODULE - 3, States of matter, , Notes, , Solutions, z, , state reasons for the elevation of boiling point and depression in freezing point of, solutions;, , z, , explain the abnormal colligative properties;, , z, , define osmosis and osmotic pressure;, , z, , define Van’t Hoff factor;, , z, , correlate the degree of dissociation of solute and, , z, , solve numerical problems., , 7.1 COMPONENTS OF A SOLUTION, When we put sugar into water, it dissolves to form a solution. We do not see any more, sugar in it. Like sugar, a large number of other substances such as common salt , urea,, potassium chloride etc dissolve in water forming solution . In all such solutions , water, is the solvent and substances which dissolve are the solutes ., Thus, solute and solvent are the components of a solution . Whenever a solute mixes, homogeneously with a solvent , a solution is formed, solute + solvent → solution, A solution is a homogeneous mixture of two or more substances ., Solvent is that component of a solution that has the same physical state as the, solution itself., Solute is the substance that is dissolved in a solvent to form a solution., 7.1.1 The Concentration of a Solution, Some of the properties of solutions , e.g.. the sweetness of a sugar solution or the, colour of a dye solution , depend on the amount of solute compared to that of the, solvent in it. This is called the solution concentration . There are several ways, for describing concentration of solution . They include molarity, molality,, normality, mole fraction and mass percentage., Molarity: Molarity is defined as the number of moles of solute dissolved per, litre of solution and is usually denoted by M. It is expressed as :, M =, , n, V, , Where n is the number of moles of solute and V is the volume of the solution in, litres. A 2.0 molar solution of sulphuric acid would be labelled as 2.0 M H2SO4., , 208, , CHEMISTRY
Page 238 :
MODULE - 3, , Solutions, , It is prepared by adding 2.0 mol of H2SO4 to water to make a litre of solution., Molarity of a solution changes with temperature because of expansion or, contraction of the solution., , States of matter, , Molality : It is defined as the number of moles of solute dissolved per kilogram, of solvent., It is designated by the symbol m. The label 2.0 m H2SO4 is read “2 molal sulphuric, acid” and is prepared by adding 2.0 mol of H2SO4 to 1 kg of solvent. Molality is, expressed as:, m=, , Notes, , 1000 nB, WA, , where nB is the number of moles of the solute and WA is the mass in grams of, solvent. The molality of a solution does not change with temperature., Example 7.1 : Find out the molarity of the solution which contains 32.0 g of, methyl alcohol (CH3OH) in 200 mL solution., Solution : Molar mass of CH3OH = 12 + 1 × 3 + 16 + 1 = 32 g mol–1, Number of moles of CH3OH =, , 32 g, = 1 mol, 32 g mol –1, , Volume of the solution = 200 mL = 0.2 litre, ∴, , Molarity =, , 1, No. of moles of solute, =, =5M, 0.2, Volume of solution in litres, , Example 7.2 : What is the molality of a sulphuric acid solution of density 1.20, g/cm3 containing 50% sulphuric acid by mass., Solution : Mass of 1cm3 of H2SO4 solution = 1.20 g, Mass of 1 litre (1000 cm3) of H2SO4 solution = 1.20 × 1000 = 1200 g, Mass of H2SO4 in 100 g solution of H2SO4 = 50 g, Mass of H2SO4 in 1200 g solution of H2SO4 =, , 50, × 1200 = 600 g, 100, , ∴ Mass of water in the solution = 1200 – 600 = 600 g, Molar mass of H2SO4 = 98 g mol–1, No. of moles of H2SO4 =, , CHEMISTRY, , Mass in grams, 600 g, =, Molar mass, 98 g mol –1, , 209
Page 239 :
MODULE - 3, , Solutions, , States of matter, , No. of moles of H 2SO 4, Molarity = Mass of water in grams × 1000, , ∴, , =, , Notes, , 600, 1, ×, × 1000 = 6.8 m, 98, 600, , Normality : Normality is another concentration unit. It is defined as the number, of gram equivalent weights of solute dissolved per litre of the solution., The number of parts by weight of a substance (element or compound) that, will combine with or displace, directly or indirectly 1.008 parts by weight of, hydrogen, 8 parts by weight of oxygen and 35.5 parts by weight of chlorine, is known as equivalent weight. Like atomic weight and molecular weight,, equivalent weight is also a number and hence no units are used to express, it. However, when equivalent weight is expressed in grams, it is known as, gram equivalent weight of the substance., Equivalent weight =, , Atomic or molecular weight, Valency, , Equivalent weight of an acid =, , Molecular weight, Basicity, , Equivalent weight of a base =, , Molecular weight, Acidity, , Equaivalent weight of a salt =, , Molecular weight, Total valency of the metal atom, , oxidising and reducing agents may have different equivalent weights if they, react to give different products under different conditions. Thus, the equivalent, weight of such substances can be calculated from the reactions in which they, take part., Normality is denoted by the symbol N., ∴ Normality (N) =, , No. of gram equivalent weights of the solute, volume of the solution in litres, , =, , 1, Mass of the solute in grams, × volume of the solution in litres, Equivalent weight of the solute, , =, , Strength of solution in grams/litre, Equivalent weight of the solute, , The label 0.5 N KMnO4 is read “0.5 normal” and represents a solution which, contains 0.5 gram equivalent of KMnO4 per litre of solution., 210, , CHEMISTRY
Page 240 :
MODULE - 3, , Solutions, , Mole Fraction : The mole fraction of a component in a solution is the ratio of its, number of moles to the total number of moles of all the components in the solution., If a solution contains 2 mol of alcohol and 3 mol of water, the mole fraction of, alcohol is, , States of matter, , 2, 3, , and that of water . The sum of mole fractions of all the components, 5, 5, , of a solution is equal to one. The mole fraction (xA) of a component A in solution, with B is :, xA =, , Notes, , nA, nA + nB, , Where nA and nB are the number of the moles of A and B respectively., Mass Percentage : Mass percentage is the mass of solute present in 100 g of, solution. Thus 5% solution of KMnO4 in water means that 5 g of KMnO4 is, present in 100 g of the aqueous solution of KMnO4., Example 7.3 : A solution contains 36.0 g water and 46.0 g ethyl alcohol, (C2H5OH). Determine the mole fraction of each component in the solution., Solution : Molar mass of water = 18 g mol–1, Molar mass of C2H5OH = 46 g mol–1, No. of moles of water =, , 36 g, 18 g mol –1, , = 2.0 mol, , 46 g, , No. of moles of C2H5OH = 46 g mol –1 = 1.0 mol, Total number of moles in the solution = 2.0 + 1.0 = 3.0, Mole fraction of water =, , 2.0, No. of moles of water, =, = 0.67, Total no. of moles in the solution, 3.0, , Mole fraction of C2H5OH =, , 1.0, No. of moles of C2 H5OH, =, = 0.33, Total no. of moles in the solution, 3.0, , Example 7.4 : Calculate the normality of a solution of NaOH if 0.4 g of NaOH, is dissolved in 100 ml of the solution., Solution : Mass of NaOH present in 100 mL of the solution = 0.4 g, ∴ Mass of NaOH present in 1000 mL of the solution =, , 0.4, × 1000 = 4.0 g, 100, , Mol. wt. of NaOH = 23 + 16 + 1 = 40 amu, , CHEMISTRY, , 211
Page 241 :
MODULE - 3, , Solutions, , States of matter, , Mol. Wt., , 4.0, , Eq. wt. of NaOH = Acidity =, = 40, 1, ∴ Normality =, , Notes, , Strength in g/litre, 4, 1, =, = N, Eq. wt., 40, 10, , Hence, the normality of the solution =, , 1, N or 0.1 N, 10, , INTEXT QUESTIONS 7.1, 1. List the various methods of expressing the concentration of a solution?, 2. Define the following, (i) Molarity, , (ii) Molality, , (iii) Normality, , 7.2 TYPES OF SOLUTIONS, Solutions can be solid, liquid or gaseous. Depending upon the physical state of, the solute and the solvent, there are nine possible types of solutions consisting of, two components (binary solutions) Different types of solutions are given in Table, 7.1., Table 7.1 Different Types of Solutions, SOLUTE, , SOLVENT, , SOLUTION, , Gas, , Gas, , Air, , Gas, , Liquid, , Soda water, , Gas, , Solid, , Hydrogen in palladium, , Liquid, , Gas, , Humidity in air, , Liquid, , Liquid, , Alcohol in water, , Liquid, , Solid, , Mercury in gold, , Solid, , Gas, , Camphor in air, , Solid, , Liquid, , Sugar in water, , Solid, , Solid, , Alloys such as brass (zinc in copper), and bronze (tin in copper), , Generally, we come across only the following three types of solutions:, (a) Liquids in Liquids: In the solution of liquids in liquids such as alcohol in, water, the constituent present in smaller amounts is designated as solute, and the constituent present in larger amounts is called the solvent. When, two liquids are mixed, three different situations may arise:, 212, , CHEMISTRY
Page 242 :
Solutions, , (i) Both the liquids are completely miscible, i.e., when, two liquids are mixed,, they dissolve in each other in all proportions, e.g., alcohol and water,, benzene and toluene., , MODULE - 3, States of matter, , (ii) The liquids are partially miscible, i.e., they dissolve in each other only to, a certain extent, e.g., water and phenol., (iii) The liquids are immiscible, i.e., they do not dissolve in each other, e.g.,, water and benzene, water and toluene., , Notes, , The solubility of liquids in liquids generally increases with rise in temperature., (b) Gases in Liquids: Gases are generally soluble in liquids. Oxygen is sufficiently, soluble in water, which allows the survival of aquatic life in ponds, rivers and, oceans. Gases like CO2 and NH3 are highly soluble in water. The solubility of a, gas in a liquid depends on the pressure, temperature and the nature of the gas, and the solvent. These factors are discussed below in detail :, (i) Effect of Pressure: The variation of solubility of a gas in a liquid with pressure, is governed by Henry’s law. Henry’s law states that, The mass or mole fraction, of a gas dissolved in a solvent is directly proportional, to the partial pressure of the gas., Henry’s law is represented by, x = Kp, where K is a constant, p is the partial pressure of the gas and x is the mole fraction of, the gas in the solution. Let us now see what are the conditions for the validity of, Henry’s law., (i), , Conditions for validity of Henry’s law: It is found that gases obey Henry’s, law under the following conditions., (i) the pressure is not too high., (ii) the temperature is not too low., (iii) the gas does not dissociate, associate or enter into any chemical reaction, with the solvent., , (ii) Effect of temperature: The solubility of a gas in a liquid at constant pressure, decreases with rise in temperature. For example, the solubility of CO2 in water, at 20°C is 0.88 cm3 per cm3 of water, where as it is 0.53 cm3 per cm3 of, water at 40°C. This happens because on heating a solution, containing a, dissolved gas, some gas is usually expelled from the solution., (iii) Effect of the nature of the gas and the solvent: Gases like CO2, HCl and,, NH3 are highly soluble in water where as H2, O2 and N2, are sparingly soluble., , CHEMISTRY, , 213
Page 243 :
MODULE - 3, , Solutions, , States of matter, , (c) Solids in liquids: When a solid is dissolved in a liquid, the solid is referred as the, solute and the liquid as the solvent. For example, in a solution of sodium chloride, in water, the solute is sodium chloride and water is the solvent. Different substances, dissolve to different extent in the same solvent., , 7.3 VAPOUR PRESSURE, Notes, , If we keep an inverted beaker over a small beaker containing a pure liquid, it is found, that the molecules of the liquid start evaporating in the form of vapours and fill the, empty space above the beaker containing the liquid. A time comes when the number, of molecules evaporating per unit time is equal to the number of molecules condensing, during that time (Fig. 7.1). An equilibrium is thus established between the vapour and, the liquid phase. The pressure exerted by the vapour of the liquid in such a case is, called the vapour pressure of the liquid., , Fig.7.1 : Vapour pressure of a liquid, , 7.4, , RAOULT’S LAW FOR SOLUTIONS, , Did you ever think that if you mix two miscible volatile liquids A and B, what would be, the vapour pressure of the resulting solution? The relationship between vapour pressure, of a liquid and its mole fraction is given by Raoult’s law., Raoult’s law states that for a solution of volatile liquids, the partial vapour pressure of, each liquid in the solution is directly proportional to its mole fraction. ., Raoult’s law is applicable only if the liquids are miscible. The vapour phase now consists, of vapours of both the liquids A and B. The partial vapour pressure of each liquid will, depend upon its mole fraction in the solution. Let the mole fractions of the liquids A, and B be XA and XB respectively. Also, if PA and PB are the partial vapour pressures of, A and B respectively, then, PA ∝ XA or PA = P0 A XA, Similarly,, , PB = p0 B XB, , where pA0 and pB0 represent the vapour pressures of pure liquids A and B respectively., , 214, , CHEMISTRY
Page 244 :
MODULE - 3, , Solutions, , If the values of PA and PB are plotted against the values of XA and XB for a solution,, two straight lines are obtained as shown in Fig. 7.2 the total vapour pressure P of the, solution is given by the sum of partial vapour pressures PA and PB., , States of matter, , Thus,, P = PA + PB, p = p 0A XA + pB0 XB, , or, , Notes, , The total vapour pressure (P) of a solution is represented by the line joining p 0A, and p0B. The solutions which obey Raoult’s law are known as ideal solutions., A solution which obeys Raoult’s law over the entire range of concentration, at all temperatures is called an ideal solution., pB0, p = pA, , + pB, , pB, , 0, , pB, , pA, , Vapour pressure, , pA, , XA = 1, XB = 0, , pA, , p = pA + pB, Mole fraction, , XA = 0, XB = 1, , Fig. 7.2 : Relationship between vapour pressure and mole fraction in a solution, , INTEXT QUESTIONS 7.2, 1. State Raoult’s law., 2. State Henry’s law and list the conditions necessary for the validity of Henry’s, law, , 7.5 RAOULT’S LAW FOR SOLUTIONS CONTAINING, NON-VOLATILE SOLUTE, If we have an aqueous solution containing a non-volatile solute, such as sugar or, salt, what do you think about the vapour pressure exerted by such a solution?, The vapour phase of such a solution consists of vapours of solvent (A) only, because the solute is non-volatile. Since the mole fraction of the solvent in solution, is less than one, therefore according to RaouIt’s law, the vapour pressure of the, CHEMISTRY, , 215
Page 245 :
MODULE - 3, States of matter, , Solutions, , solution will be less than the vapour pressure of the pure solvent. If the total vapour, pressure of the solution is P, then, PA = pA0 XA, , ...(7.1), , for a binary mixture, XA + XB = 1, Notes, , therefore,, XA = 1 – XB, Substituting the value of XA in equation (7.1) we get, PA = p0A(1 – XB), PA, PA0, , therefore,, , p0A − p A, PA0, , = 1 – XB, = XB, , In the above equation, (P0A – PA) represents the lowering of the vapour pressure, and, , p0A − p A, PA0, , is called the relative lowering of the vapour pressure of the solution., , An alternative statement of Raoult’s law for solutions of non-volatile solute is :, The relative lowering of vapour pressure for a solution is equal to the mole, fraction of the solute, when only the solvent is volatile., , 7.6 IDEAL AND NON-IDEAL SOLUTIONS, Ideal solutions obey Raoults Law and during their formation there is no, change in heat and volume., Non-ideal solutions are those solutions which do not obey Raoult’s law and, whose formation is accompanied by changes of heat and volume., Most of the real solutions are non-ideal. They show considerable deviation from, the ideal behaviour. Generally deviations are of two types;, (i) Positive deviation: Positive deviations are shown by liquid pairs for which the, A-B molecular interactions are weaker than the A-A and or B-B molecular interactions., The total vapour pressure for such solutions is greater than predicted by RaouIts law., The total vapour pressure for such a solution will be maximum for a particular, intermediate composition (Fig. 7.3), Examples of non-ideal solutions showing positive deviation from the ideal, behaviour are mixtures of liquids such as water-propanol, ethanol-chloroform,, acetone- carbon disulfide, ethanol-cyclohexane etc., 216, , CHEMISTRY
Page 246 :
MODULE - 3, , Solutions, , States of matter, pB0, , p, p = pA + B, , pA0, , pB, , Vapour pressure, , pA, pB, , pA, , Notes, , Mole fraction, , XA = 1, XB = 0, , XA = 0, XB = 1, , Fig. 7.3 : Positive deviation for a liquid pair, , (ii) Negative Deviation: Negative deviations are shown by liquid pairs for which the, A-B molecular interactions-are stronger than A-A or B-B molecular interactions. The, total vapour pressure for such solutions is less than that predicted by Raoult’s law. For, a particular intermediate composition, the total vapour pressure of such a solution will, be minimum (Fig. 7.4). Examples of such liquid pairs are chloroform acetone, watersulphuric acid, phenol-aniline, water- HCI etc., pB0, p = pA +, , pA0, , pB, , Vapour pressure, , pB, , pA, , pB, , XA = 1, XB = 0, , pA, , Mole fraction, , XA = 0, XB = 1, , Fig. 7.4 : Negative deviation for a liquid pair, , 7.7, , COLLIGATIVE PROPERTIES, , Do you know that there are certain properties of dilute solutions which depend only, on the number of particles of solute and not on the nature of the solvent and the solute?, Such properties are called colligative properties. There are four colligative properties, : relative lowering of vapour pressure, elevation in boiling point, depression in freezing, point and osmotic pressure., We shall discuss these colligative properties in detail in the following sections., , CHEMISTRY, , 217
Page 247 :
MODULE - 3, States of matter, , Solutions, , 7.7.1 Relative Lowering of Vapour Pressure, According to Raoult’s law for solutions containing non- volatile solute, Ρ 0A − PA, , = XB (see section 7.5), , PA0, , Notes, , ...(i), , nB, , Also, , XB = n + n, A, B, , In a dilute solution nB << nA Therefore the term nB can be neglected in the, denominator., , Hence,, , XB, , WB, nB M B, W .M, = n =W = B A, A, WA . M B, A, MA, , Therefore equation (i) can be written as, Ρ0A − Ρ A, Ρ 0A, , WB . M A, , = XB = W . M, A, B, , The above expression can be used to determine the molecular mass of the solute, B, provided the relative lowering of vapour pressure of a solution of known, concentration and molecular mass of the solvent are known. However, the, determination of molecular mass by this method is often difficult because the, accurate determination of lowering of vapour pressure is difficult., Example 7.5: The relative lowering of vapour pressure produced by dissolving, 7.2 g of a substance in 100g water is 0.00715. What is the molecular mass of the, substance?, Solution: We know that, PA0 − PA, PA0, , W, , M, , B, A, = M ×W, B, A, , Substituting the values we get, 7.2 × 18, , 0.00715 = M × 100, B, ∴, 218, , 7.2 × 18, , or MB = 0.00715 × 100, , Molecular mass of the substance = 181.26 amu, CHEMISTRY
Page 248 :
MODULE - 3, , Solutions, , States of matter, , 7.7.2 Elevation of Boiling Point, Boiling point of a liquid is the temperature at which the vapour pressure of the liquid, becomes equal to the atmospheric pressure., As you know, the vapour pressure of a pure solvent is always higher than that of its, solution. So, the boiling point of the solution is always higher than that of the pure, solvent. If you see the vapour pressure curves for the solvent and the solution (Fig., 7.5), you will find that there is an elevation in the boiling point of the solution., , Notes, , Vapour pressure, , 1 Atmosphere, , Sol, , ven, , t, , tion, , Solu, , DTb, , B.P. of, solvent, , Temperature K, , B.P. of, solution, , Fig. 7.5 : Vapour pressure curves for solvent and solution, , Now let ΔTb be the elevation in boiling point and Δp be the lowering in vapour pressure., Then,, ΔTb α Δp α XB or ΔTb = K XB, , (i), , K is the proportionality constant, As you know, , nB, , XB = n + n, A, B, , In a dilute solution, nB << nA and thus the term nB is neglected in the denominator., , Thus,, , XB =, , nB, nA, , WB, WB, MA, MA, M, = B = M × W = nB × W, WA, B, A, A, MA, , Substituting the value of XB in the equation (i) we get, MA, , ΔTb = K × nB × W, A, CHEMISTRY, , 219
Page 249 :
MODULE - 3, , Solutions, , States of matter, , If we take the mass of the solvent WA in kilograms the term, , nB, is molality m. Thus, WA, , ΔTb = K MA . m = KB m, , Notes, , The constant Kb is called the molal elevation constant for the solvent. Kb may be, defined as the elevation in boiling point when one mole of a solute is dissolved, in one kilogram of the solvent. Kb is expressed in degree per molality., 7.7.3 Depression in Freezing Point, Freezing point is the temperature at which the solid and the liquid forms have the same, vapour pressure, , Vapour pressure, , The freezing point of the solution is always less than that of the pure solvent. Thus,, there is a depression in the freezing point of the solution This is because the vapour, pressure of the solution is always less than that of the pure solvent., , Solven, , l, So, , id, , S, , ve, ol, , t, , nt, , Solutio, , n, , DTf, , FP of FP of, solution solvent, , Temperature K, , Fig. 7.6 : Vapour pressure curves for solid, solvent and solution, , Let ΔTf be the depression in freezing point. Then:, ΔT f α XB, or, , ΔT f = KXB, , ...(ii), , Where K the proportionality constant, You know that, , nB, , XB = n + n, A, B, , In dilute solutions nB << nA, Therefore, the term nB can be neglected from the denominator. Thus,, , 220, , CHEMISTRY
Page 250 :
MODULE - 3, , Solutions, , States of matter, , n, W / MB, M, W, M, XB = B = B, = B × A = nB × A, MB, WA, WA, nA WA / M A, ⎛, WB ⎞, ⎜ sin ce n B =, ⎟, MB ⎠, ⎝, , Substituting the value of XB in equation (ii) we get, MA, , ΔTf = K × nB × W, A, , Notes, nB, , If the mass of the solvent WA is taken in kg, then the term W becomes, A, molality m., Thus,, , ΔTf = K MA . m = Kf . m, , The constant (Kf ) for a solution is known as molal depression constant or, molal cryoscopic constant for the solvent. Kf may be defined as the depression, in freezing point of a solution when one mole of a solute is dissolved in 1, kilogram of the solvent., Example 7.6: Find the (i) boiling point and (ii) freezing point of a solution, containing 0.520 g glucose (C6H12O6) dissolved in 80.2g of water.(Kf = 1.86 K/, m, Kb = 0.52 k/m.), Solution:, , Molality of glucose =, , =, , Wt. of glucos e, 1000, ×, mol. Wt., Wt. of solvent, , 0.52 1000, ×, = 0.036, 180 80.2, , ΔTb = Kbm = 0.52 × 0.036 = 0.018 K, ∴, (ii), , Boiling point = 373 + 0.018 = 373.018 = 373.02 K, Kf = 1.86 K/m, m=, , 0.52 1000, ×, = 0.036, 180 80.2, , ∴, , ΔTf = 1.86 × 0.036 = 0.66 K, , ∴, , Freezing point = 273 – 0.66 = 272.34 K, , 7.7.4 Osmosis and Osmotic Pressure, You must have observed that if rasins are soaked in water for some time, they, swell. this is due to the flow of water into the rasins through its skin which acts, , CHEMISTRY, , 221
Page 251 :
MODULE - 3, States of matter, , Notes, , Solutions, , as a semipermeable membrane (permeable only to the solvent molecules). This, phenomenon is also observed when two solutions of different concentrations in, the same solvent are separated by a semipermeable membrane. In this case the, solvent flows from a solution of lower concentration to a solution of higher, concentration. The process continues till the concentrations of the solutions on, both sides of the membrane become equal., The spontaneous flow of the solvent from a solution of lower concentration (or pure, solvent) to a solution of higher concentration when the two are separated by a, semipermeable membrane is known as osmosis., The flow of solvent into the solution of higher concentration from a solution of lower, concentration (on pure solvent) can be stopped if some extra pressure is applied to, that side of the solution which has a higher concentration. The pressure that just stops, the flow of the solvent is called osmotic pressure., Thus, osmotic pressure may be defined as the excess pressure that must be applied, to the solution side to just prevent the passage of pure solvent into it when the, two are seprated by a perfect semipermeable membrane. This is illustrated in, Fig. 7.7., h, , Solvent, Solution, Semipermeable, membrane, , Fig. 7.7 : Osmosis, , The pressure that must be applied to the solution side to prevent it from rising in the, tube is the osmotic pressure. It is also equal to the hydrostatic pressure of the liquid, column of height h., If the two solutions have the same osmotic pressure, they are known as isotonic, solutions. The osmotic pressure is a colligative propertly. It depends on the number, of particles of solute present in the solution and not on their nature. At a given, temperature T, the osmotic pressure (π) of a dilute solution is experimentally, found to be proportional to the concentration of the solution in moles per litre., Mathematically,, , π = CRT, , where π is the osmotic pressure and R is the gas constant, or, , π=, , nB, RT, V, , where nB is the number of moles of solute present in V litres of the solution, 222, , CHEMISTRY
Page 252 :
MODULE - 3, , Solutions, , States of matter, , or, , w, , πV = M, RT, solute, , where w in the mass of solute dissolved in V litres of the solution and Msolute is, the molar mass of the solute. Thus, knowing π, V and w, the molar mass of the, solute can be calculated., Thus, the molar masses of the solutes can be determined by measuring the osmotic, pressure of their solutions. This method has been widely used to determine the, molar masses of macromolecules, proteins, etc., which have large molar masses, and limited solubility. Therefore their solutions have very low concentrations, and the magnitudes of their other colligative properties are too small to measure., yet their osmotic pressures are large enough for measurements. As the osmotic, pressure measurements are done at around room temperature, this method is, particularly useful for determining the molar masses of biomolecules as they are, generally not stable at higher temperature., , Notes, , Example 7.7 : The osmotic pressure of an aqueous solution of a protein containing, 0.63 g of a protein in 100 g of water at 300 K was found to be 2.60 × 10–3 atm., Calculate the molar mass of the protein. R = 0.082 L atm K–1 mol–1., We know that osmotic pressure of a solution in given by the expression, w, , πV = M, RT, solute, or, , Msolute =, , w, RT, πV, , Substituting the values, we get, Msolute =, , (0.63g) × (0.082 L – atm K –1mol –1 ) × (300 K), (2.60 × 10 −3 atm) × (0.100 L), , = 61022 g mol–1, Thus, molar mass of the protein is 61022 g mol–1., Reverse Osmosis and Water Purification, If a pressure higher than the osmotic pressure is applied to the solution side, the, direction of flow of the solvent can be reversed. As a result, the pure solvent, flows out of the solution through the semipermeable membrane. This process is, called reverse osmosis. It is of great practical application as it is used for, desalination of sea water to obtain pure water., , CHEMISTRY, , 223
Page 253 :
MODULE - 3, , Solutions, , States of matter, , INTEXT QUESTIONS 7.3, 1. Define colligative property. List two colligative properties., 2. What type of liquid pairs show (i) positive deviations (ii) negative deviations., Notes, , 3. Why is the determination of osmotic pressure a better method as compared to, other colligative properties for determining the molar masses of biomolecules., , 7.8, , ABNORMAL COLLIGATIVE PROPERTIES, , The colligative properties of the solutions depend only upon the number of solute, particles present in the solution and not on their nature But sometimes while measuring, colligative properties abnormal results are obtained due to the following reasons :, (i), , If the solution is very concentrated, the particles of the solute start interacting, with each other. Therefore, the solution should not be concentrated., , (ii), , In case of association two or more solute molecules associate to form a bigger, molecule. The number of effective molecules in the solution, therefore decreases., Consequently, the value of the collgative property (relative lowering of vapour, pressure, elevation of boiling point, depression of freezing point, osmotic pressure), is observed to be less than that calculated on the basis of unassociated molecules. Since, the colligative property is inversely proportional to the molar mass,, the molar mass of such solutes calculated on the basis of collgative property will, be greater than the true molar mass of the solute., , (iii) In case of dissociation of the solute in the solution, the number of effective, solute particles increases. In such cases the value of the observed collgative, property will be greater than that calculated on the basis of undissociated solute, particles. The molar mass of the solute calculated from the measurement of, collgative property will be lower than the true molar mass of the solute., Van’t Hoff factor, In order to account for extent of association or dissociation Van’t Hoff introduced a, factor ‘i’., i=, , Observed colligative property, Normal (calculated or expected) colligative property, , Since the colligative property is proportional to the number of solute particles or the, number of moles of solute, Total number of moles of solute in the solution, , i = Expected (calculated) number of moles of solute, 224, , CHEMISTRY
Page 254 :
MODULE - 3, , Solutions, , Also, since colligative properties vary inversely as the molar mass of the solute, it, follows that, i=, , States of matter, , Normal (calculated or expected) molar mass, Observed molar mass, , Here the observed molar mass is the experimentally determined molar mass whereas, the normal molar mass is the molar mass calculated on the basis of chemical formula of, the solute. In case of association the value of van’t Hoff factor, i, is less than unity while, for dissociation it is greater than unity. For example, benzoic acid associates in benzene, to form a dimer. The value of i is, therefore, close to 1/2. The value of i for aqueous, NaCl is close to 2.0 because NaCl dissociates in water to form Na+ and Cl– ions., , Notes, , The inclusion of van’t Hoff factor, i, modifies the equations for the colligative properties, as follows :, PA0 – PA, PA0, , = i XB, , ΔT b = i Kb m, ΔT f = i Kf m, , π V = i CRT, Degree of Association, Degree of association may be defined as the fraction of the total number of molecules, which associate to form a bigger molecule. Let us consider the association of benzoic, acid in benzene. In benzene two molecules of benzoic acid associate to form a dimer., It can be represented as, ZZX (C6H5COOH)2, 2C6H5COOH YZZ, , If x represents the degree of association of benzoic acid in benzene (i.e out of one, molecule of benzoic acid, x molecules associate to form a dimer), then at equilibrium., No. of moles of unassociated benzoic acid = 1 – x, No of moles of associated benzoic acid =, , x, 2, , Total number of effective moles of benzoic acid = 1 – x +, , CHEMISTRY, , x, x, =1–, 2, 2, , 225
Page 255 :
MODULE - 3, States of matter, , Solutions, , According to definition, Van’t Hoff factor is given by, Total number of moles of solute in the solution, i=, =, Expected (calculated) number of moles of solute, , Notes, , 1−, , x, 2, , 1, , Example 7.8 : Acetic acid (CH3COOH) associates in benzene to form double, molecules 1.60 g of acetic acid when dissolved in 100 g of benzene (C6H6) raised the, boiling point by 273.35 K. Calculate the van’t Hoff factor and the degree of association, of benzoic acid. Kb for C6H6 = 2.57 K kg mol–1., ΔTb = i kb m =, , Solution :, , 1000 i Kb WB, WA M B, , Normal molar mass (MB) of CH3COOH = 60 g mol–1, ΔTb × WA × M B, , Van’t Hoff factor, i, is = 1000 × K × W, b, B, , 0.35 × 100 × 60, , = 1000 × 2.57 × 1.60, = 0.51, Since, acetic acid associates in benzene to form double molecules, the following, equilibrium exists in the solution., ZZX (CH COOH), 2CH3COOH YZZ, 3, 2, , If x represents the degree of association of the solute, then we would have (1 –x) mol, of acetic acid left unassociated and x/2 moles of acetic acid at equilibrium., Therefore, total number of particles at equilibrium, , = 1 – x + x/2, = 1−, , x, 2, , The total number of partilces at equilibrium equal van’t Hoff factor. But van’t Hoff, factor (i) is equal to 0.51., ∴, , or, , 1−, , x, = 0.51, 2, x, = 1 – 0.51 = 0.49, 2, , x = 0.49 × 2 = 0.98, 226, , CHEMISTRY
Page 256 :
MODULE - 3, , Solutions, , States of matter, , Therefore, degree of association of acetic acid in benzene is 98%,., Degree of dissociation, Degree of dissociation may be defined as the fraction of the total number of particles, that dissociate, i.e., break into simpler ions. Consider a solution of KCl in water., When KCl is dissolved in water, it dissociates into K+ and Cl– ions., , Notes, , ZZX K+ + Cl–, KCl YZZ, , let x be the degree of dissociation of KCl, then at equilibrium, number of moles of, undissociated KCl = 1 – x, According to the dissociation of KCl shown above, when x mol of KCl dissociates,, x moles of K+ ions and x mol of Cl– ions are produces, Thus, the total number of moles in the solution after dissociation, =1–x+x+x=1+x, Hence,, , Total number of moles of solute in the solution, , i = Expected (calculated) number of moles of solute =, , 1+ x, 1, , Example 7.9 : A 0.5 percent aqueous solution of potassium chloride was found to, freeze at 272.76 K. Calculate the van’t Hoff factor and the degree of dissociation of, the solute at this concentration. (Kf for H2O = 1.86 K kg mol–1)., Solution : Normal molecular weight of KCl = 39 + 35.5 = 74.5 g mol–1, Observed molecular weight, MB =, , =, , 1000 × WB × K f, ΔTf × WA, 1000 × 0.5g × 1.86 K kg mol−1, (0.24 K) × 100 g, , = 38.75 g mol–1, Normal molecular weight, , van’t Hoff factor (i) = Observed molecular weight, , =, , CHEMISTRY, , 74.5 g mol −1, 38.75 g mol −1, , = 1.92, , 227
Page 257 :
MODULE - 3, States of matter, , Solutions, , Potassium chloride in aqueous solution dissociates as follow., ZZX K+ + Cl–, KCl YZZ, , Let x be the degree of dissociation of KCl. Thus at equilibrium,, No. of moles of KCl left undissociated = (1 – x) mol, Notes, , No. of moles of K+ = x mol, No. of moles of Cl– = x mol, Total number of moles at equilibrium = 1 – x + x + x = 1 + x, ∴, or, , Van’t Hoff factor =, , 1+ x, = 1.92, 1, , x = 1.92 – 1 = 0.92, , ∴ Degree of dissociation of KCl = 92%, , WHAT YOU HAVE LEARNT, , 228, , z, , Solution is a homogeneous mixture of two or more substances., , z, , Solvent is that component of a solution that has the same physical state as the, solution itself., , z, , Solute is the substance that is dissolved in a solvent to form a solution., , z, , Molarity is expressed as the number of moles of solute per litre of solution., , z., , Molality is expressed as the number of moles of solute per kilogram of solvent., , z, , Normality is a concentration unit which tells the number of gram equivalents of, solute per litre of solution., , z, , Mole fraction is the ratio of the number of moles of one component to the total, number of moles in the solution., , z, , Solutions can be solid, liquid or gaseous., , z, , Henry’s law states that mass or mole fraction of a gas dissolved in a solvent is, directly proportional to the partial pressure of the gas., , z, , Raoult’s law states that for a solution of volatile liquids, the partial pressure of, each liquid in the solution is directly proportional to its mole fraction., , z, , A solution which obeys Raoult’s law over the entire range of concentration at all, temperatures is called an ideal solution., , CHEMISTRY
Page 258 :
MODULE - 3, , Solutions, z, , The relative lowering of vapour pressure for a solution is equal to the mole fraction, of the solute, when only the solvent is volatile., , z, , Those properties of dilute solutions which depend only on the number of particles, of solute and not on their nature are known as colligative properties., , z, , Molal elevation constant is the elevation in boiling point when one mole of solute, is dissolved in one kilogram of the solvent., , z, , Boiling point of a liquid is the temperatures at which the vapour pressure of the, liquid becomes equal to the atmospheric pressure., , z, , Freezing point is the temperature at which the solid and the liquid forms of the, substance have the same vapour pressure., , z, , Abnormal result are obtained when the solute associates or dissociates in the, solution., , z, , Van’t Hoff factor is defined as the ratio of normal molar mass to experimentally, determined molar mass., , States of matter, , Notes, , TERMINAL EXERCISE, 1. What do you understand by ideal and non-ideal solutions?, 2. Define freezing point and boiling point., 3. Derive the relationship ΔTb = Kb m, 4. A solution containing 7 g of a non-volatile solute in 250 g of water boils at 373.26, K. Find the molecular mass of the solute., 5. 2 g of a substance dissolved in 40 g of water produced a depression of 274.5 K, in the freezing point of water. Calculate the molecular mass of the substance. The, molal depression constant for water is 274.85 K per molal., 6. Calculate the mole fraction of the solute in a solution obtained by dissolving 10 g, of urea (mol wt 60) in 100 g of water., 7. A solution containing 8.6 g of urea (molar mass = 60 per dm3) was found to be, isotonic with a 5 per cent solution of an organic non-valatile solute. Calculate the, molar mass of the non-valatile solute., 8. 2 g of benzoic acid (C6H5COOH) dissolved in 25 g of benzene shows a depression, in freezing point equal to 1.62 K. Molar depression constant for benzene in 4.9 K, kg mol–1. What is the percentage association of C6H5COOH if it forms double, molecules in solution., , CHEMISTRY, , 229
Page 259 :
MODULE - 3, States of matter, , Solutions, , 9. The freezing point depression of 5.0 × 10–3 M solution of Na2SO4 in water was, found to be 0.0265ºC. Calculate the degree of dissociation of the salt at this, concentration. (Kf for H2O is 1.86 K kg mol–1), , ANSWERS TO INTEXT QUESTIONS, Notes, 7.1, 1. Molarity, Molality, Normality, Mole fraction, Mass percentage., Molarity is the number of moles of solute dissolved per litre of the solution., 2. Molality is the number of moles of solute dissolved per kg of solvent., Normality is the number of gram equivalents of solute dissolved per litre of, solution., 7.2, 1. For a solution of volatile liquids the partial vapour pressure of each liquid is, proportional to its mole fraction., 2. The mass of a gas dissovled in a solvent is directly proportional to its partial, pressure. Pressure should not be too high Temperature should not be too, low. The gas should not associate or dissociate., 7.3, 1. Properties that depend upon the number of particles of solute and not on the, nature of solute. e.g. Elavation of boiling point, depression of fruzing point., 2. For which A–B molecular interactions are:, (i) weaker than A–A and B–B interactions., (ii) stronger than A–A and B–B interactions., 3. At low concentration the magnitude of osmotic pressure is large enough for, measurement., , 230, , CHEMISTRY
Page 260 :
MODULE - 3, , Solutions, , States of Matter, , 8, Notes, , COLLOIDS, , You are familiar with solutions. They play an important role in our life. A large, number of substances such as milk, butter, cheese, cream, coloured gems, boot, polish, rubber, ink also play an important role in our daily life. They are mixtures of, special type. They are colloidal solutions. The term colloid has been derived from, two terms, namely colla and oids. ‘Kolla’ means glue and ‘Oids’ means like i.e., glue-like. The size of the particles in colloidal solutions is bigger than the size of, particles present in solutions of sugar or salt in water but smaller than the size of, particles in suspensions. In this lesson you will learn about the methods of preparation,, properties and applications of colloidal solutions., , OBJECTIVES, After reading this lesson you will be able to:, z explain the difference between true solution, colloidal solution and suspension;, z identify phases of colloidal solution;, z classify colloidal solutions;, z describe methods of preparation of colloids;, z explain some properties of colloidal solutions;, z explain Hardy Schultz Rule;, z recognise the difference between gel and emulsion;, z cite examples of the application of colloids in daily life; and, z define nano materials and list some of their properties., , 8.1 DISTINCTION BETWEEN A TRUE SOLUTION,, COLLOIDAL SOLUTION AND SUSPENSION, You may recall that solution of sugar in water is homogeneous but milk is not., When you closely look at milk you can see oil droplets floating in it. Thus, although, it appears to be homogenous it is actually heterogenous in nature. The nature of, CHEMISTRY, , 231
Page 261 :
MODULE - 3, States of Matter, , Solutions, , the solution formed depends upon the size of the solute particles. If the size of, the solute particles is less than 1 nm it will form true solution but when the size, is between 1 to 100 nm then it will form colloidal solution. When the size of, solute particles is greater than 100 nm it will form a suspension. Therefore we, may conclude that colloidal solution is an intermediate state between true solution, and suspension (Table 8.1)., , Notes, , Table 8.1: Some important properties of true solutions, colloids, and suspensions, , S.No., , Name of, Property, , True Solution, , Colloids Solution, , Suspension, , 1., , Size, , Size of particles is, less than 1 nm, , Size of particles is, between 1nm and, 100 nm., , Size of particles is, greater than 100, nm., , 2., , Filterability, , Pass through, ordinary, filter paper and also, through animal, membrane., , Pass through, ordinary filter paper, but not through, animal membrane., , Do not pass, through filter paper, or, animal membrane., , 3., , Settling, , Particles do not, settle down on, keeping, , Particles do not settle, down on their own, but can be made to, settle down by, centrifugation., , Particles settle, down on their own, under gravity., , 4., , Visibility, , Particles are, invisible, to the naked eye as, well as under a, microscope., , Particles are invisible, to the naked eye but, their scattering effect, can be observed with, the help of a, microscope., , Particles are visible, to the naked eye., , 5., , Separation, , The solute and, solvent cannot be, separated by, ordinary filteration, or by ultra, filteration., , The solute and, solvent cannot be, separated, by ordinary filteration, but can be separated, by ultra-filteration., , The solute and, solvent can be, separated by, ordinary filteration., , 6., , Diffusion, , Diffuse quickly, , Diffuse slowly, , Do not diffuse, , 8.2 PHASES OF COLLOIDS SOLUTION, Colloids solutions are heterogenous in nature and always consist of at least two, phases : the dispersed phase and the dispersion medium., z, , 232, , Dispersed Phase : It is the substance present in small proportion and consists, of particles of colloids size (1 to 100 nm)., CHEMISTRY
Page 262 :
MODULE - 3, , Solutions, z, , Dispersion Medium : It is the medium in which the colloids particles are, dispersed. For example, in a colloidal solution of sulphur in water, sulphur, particles constitute the ‘dispersed phase’ and water is the ‘dispersion medium’., , Each of the two phases namely, dispersed phase and dispersion medium can be, solid, liquid or gas. Thus, different types of colloidal solutions are possible, depending upon the physical state of the two phases. Different types of colloidal, solutions and their examples are shown in Table 8.2. You should note that gases, cannot form a colloidal solution between themselves, because they form, homogenous mixtures., , States of Matter, , Notes, , Table 8.2 : Types of Colloidal Solutions, S.No., , Dispersed, Phase, , Dispersion, Medium, , Type of, Colloidal Solution, , Examples, , 1., , Solid, , Solid, , Solid sol., , Gemstones,, , 2., , Solid, , Liquid, , Sol, , Paints, muddy water,, gold sol, starch sol,, arsenious sulphide sol., , 3., , Solid, , Gas, , Aerosol of solids, , Smoke, dust in air, , 4., , Liquid, , Solid, , Gel, , Jellies, Cheese, , 5., , Liquid, , Liquid, , Emulsion, , Milk, Cream, , 6., , Liquid, , Gas, , Aerosol, , Mist, fog, cloud, , 7., , Gas, , Solid, , Solid foam, , Foam rubber,, pumice stone, , 8., , Gas, , Liquid, , Foam, , Froth, whipped cream, , Out of the various types of colloidal solutions listed above, the most common are, sols (solid in liquid type), gels (liquid in solid type) and emulsions (liquid in, liquid type). If the dispersion medium is water then the ‘sol’ is called a hydrosol;, and if the dispersion medium is alcohol then the ‘sol’ is called an alcosol., , INTEXT QUESTIONS 8.1, 1. Classify the following into suspension, colloidal solution and true solution., milk, sugar in water, clay in water, blood, boot polish, sand in water, face, cream, jelly, foam., 2. Give one example each of, (a) Sol (b) Gel (c) Aerosol (d) Emulsion, 3. What is the difference between an alcosol and hydrosol?, 4. How does colloidal solution differ from true solution., CHEMISTRY, , 233
Page 263 :
MODULE - 3, , Solutions, , States of Matter, , 8.3 CLASSIFICATION OF COLLOIDS, Colloidal solutions can be classified in different ways :, (a) on the basis of interaction between the phases., (b) on the basis of molecular size., Notes, , 8.3.1 Classification Based Upon Interaction, Depending upon the interaction between dispersed phase and the dispersion, medium colloidal solutions have been classified into two categories., (a) Lyophilic colloids : The word 'Lyophilic' means solvent lover. Lyophilic, colloidal solutions are those in which the dispersed phase have a great, affinity (or love) for the dispersion medium. Substances like gum, gelatine,, starch etc when mixed with suitable dispersion medium, directly pass into, colloidal state and form colloidal solution. Therefore, such solutions are, easily formed simply by bringing dispersed phase and dispersion medium, in direct contact with each other. However, these colloidal solutions have, an important property i.e. they are reversible in nature. This means that once, lyophilic colloidal solution has been formed then dispersed phase and, dispersion medium can be separated easily. Once separated these can again, be formed by remixing the two phases. These sols are quite stable., If water is used as dispersion medium then it is termed as hydrophilic, colloid., (b) Lyophobic Colloids : The word 'Lyophobic' means solvent hating. Lyophobic, colloidal solutions are those in which the dispersed phase has no affinity, for the dispersion medium. Metals like Au, Ag and their hydroxides or, sulphides etc., when simply mixed with dispersion medium do not pass, directly into colloidal state. These sols have to be prepared by special, methods. These sols can be readily precipitated and once precipitated they, have little tendency to go back into the colloidal state. Thus these sols are, irreversible in nature. Also they are not very stable and require a stabilizing, agent to remain in the colloidal form. In case water is used as dispersion, medium it is called as hydrophobic sol., 8.3.2 Classification Based on Molecular Size, Depending upon the molecular size the colloids have been classified as, (a) Macromolecular colloids – In this type of colloids the size of the particles of, the dispersed phase are big enough to fall in the colloidal dimension as, discussed earlier (i.e. 1–100 nm), Examples of naturally occurring macromolecular colloids are starch,, cellulose, proteins etc., , 234, , CHEMISTRY
Page 264 :
MODULE - 3, , Solutions, , (b) Multi molecular colloids – Here individually the atoms are not of colloidal, size but they aggregate to join together forming a molecule of colloidal, dimension. For example sulphur sol contains aggregates of S8 molecules, which fall in colloidal dimension., (c) Associated colloids – These are substances which behave as normal, electrolyte at low concentration but get associated at higher concentration, to form miscelle and behave as colloidal solution. Soap is an example. Soap, is sodium salt of long chain fatty acid R COONa. When put in water, soap, forms RCOO— and Na+. These RCOO— ions associate themselves around, dirt particles as shown below forming a miscelle (Fig. 8.1)., , States of Matter, , Notes, , +, , Na, Water COO–, +, , Na, COO–, , –, , COO, , +, , Miscelle, +, COO– Na, , –, , COO, Na+, , COO–, Na+, , Na, , COO– +, Na, –, , COO, Na+, , Fig.8.1 : Aggregation of RCOO– ions to form a micelle., , 8.4 PREPARATION OF COLLOIDAL SOLUTIONS, As discussed earlier, the lyophilic sols can be prepared directly by mixing the, dispersed phase with the dispersion medium. For example, colloidal solutions of, starch, gelatin, gum etc. are prepared by simply dissolving these substances in, hot water. Similarly, a colloidal sol of cellulose nitrate is obtained by dissolving it, in alcohol. The resulting solution is called collodion., However, lyophobic colloids cannot be prepared by direct method., Hence two types of methods are used for preparing lyophobic colloids. These, are:, (i) Physical methods, (ii) Chemical methods, (i) Physical methods, These methods are employed for obtaining colloidal solutions of metals like gold,, silver, platinum etc. (Fig. 8.2), , CHEMISTRY, , 235
Page 265 :
MODULE - 3, , Solutions, , States of Matter, , +, , Electrodes, , –, , Dispersion medium, Ice-Bath, , Notes, , Fig. 8.2 : Preparation of colloidal solution by Bredig’s Arc Method, , An electric arc is struck between the two metallic electrodes placed in a container, of water. The intense heat of the arc converts the metal into vapours, which are, condensed immediately in the cold water bath. This results in the formation of, particles of colloidal size. We call it as metal sol. e.g. gold sol., Peptisation : Peptisation is the process of converting a freshly prepared precipitate, into colloidal form by the addition of a suitable electrolyte. The electrolyte is, called peptising agent. For example when ferric chloride is added to a precipitate, of ferric hydroxide, ferric hydroxide gets converted into reddish brown coloured, colloidal solution. This is due to preferential adsorption of cations of the electrolyte, by the precipitate. When FeCl3 is added to Fe(OH)3, Fe3+ ions from FeCl3 are, adsorbed by Fe(OH)3 particles. Thus the Fe(OH)3 particles acquire + ve charge, and they start repelling each other forming a colloidal solution., (ii) Chemical Methods : By oxidation, Sulphur sol is obtained by bubbling H2S gas through the solution of an oxidizing, agent like HNO3 or Br2 water, etc. according to the following equation :, Br2 + H2S → S + 2 HBr, 2 HNO3 + H2S → 2 H2O + 2 NO2 + S, Fe(OH)3 sol, As2 S3 sol can also be prepared by chemical methods., , 8.5 PURIFICATION OF COLLOIDAL SOLUTION, When a colloidal solution is prepared it contains certain impurities. These impurities, are mainly electrolytic in nature and they tend to destabilise the colloidal solutions., Therefore colloidal solutions are purified by the following methods:, (i) Dialysis, (ii) Electrodialysis, Dialysis : The process of dialysis is based on the fact that colloidal particles, cannot pass through parchment or cellophane membrane while the ions of the, 236, , CHEMISTRY
Page 266 :
MODULE - 3, , Solutions, , electrolyte can. The colloidal solution is taken in a bag of cellophane which is, suspended in a tub full of fresh water. The impurities diffuse out leaving pure, coloidal solution in the bag (Fig. 8.3). This process of separating the particles of, colloids from impurities by means of diffusion through a suitable membrane is, called dialysis., , States of Matter, , Notes, Water, , Dialysis, Bag, , Fig. 8.3 : A dialyser, , Electrodialysis : The dialysis process is slow and to speed up its rate, it is carried, out in the presence of an electrical field. When the electric field is applied through, the electrodes, the ions of the electrolyte present as impurity diffuse towards, oppositely charged electrodes at a fast rate. The dialysis carried out in the presence, of electric field is known as electrodialysis (Fig. 8.4)., –, , +, , Addition of, Impure sol, , Water, , Funnel, , Electrodes, Colloid, Crystalloid, Solution of Crystalloid, in water, Cellophane bag or, Parchment paper bag, , Fig. 8.4 : Electrodialysis, , CHEMISTRY, , 237
Page 267 :
MODULE - 3, States of Matter, , Solutions, , The most important use of dialysis is the purification of blood in the artificial, kidney machine. The dialysis membrane allows the small particles (ions etc.) to, pass through, whereas large size particles like haemoglobin do not pass through, the membrane., , Notes, , INTEXT QUESTIONS 8.2, 1. Name two colloids that can be prepared by Bredig’s Arc method., 2. Name two colloids that can be prepared by chemical methods., 3. Differentiate between (a) Lyophilic and Lyophobic sol. (b) macromolecular, and multimolecular colloids., 4. Explain the formation of miscelle., , 8.6 PROPERTIES OF COLLOIDS, The properties of colloids are discussed below :, a), , Heterogeneous character : Colloidal particles remain within their own, boundary surfaces which separates them from the dispersion medium. So a, colloidal system is a heterogeneous mixture of two phases. The two phases, are dispersed phase and dispersion medium., , b), , Brownian movement : It is also termed as Brownian motion and is named, after its discoverer Robert Brown (a Botanist.), Brownian Motion is the zig-zag movement of colloidal particles in continuous, and random manner (Fig. 8.5). Brownian motion arises because of the impact, of the molecules of the dispersion medium on the particles of dispersed, phase. The forces are unequal in different directions. Hence it causes the, particles to move in a zig-zag way., , Fig. 8.5 : Brownian Movement, , 238, , CHEMISTRY
Page 268 :
MODULE - 3, , Solutions, , c), , Tyndall Effect : Tyndall in 1869, observed that if a strong beam of light is, passed through a colloidal solution then the path of light is illuminated. This, phenomenon is called Tyndall Effect. This phenomenon is due to scattering, of light by colloidal particles (fig.8.6). The same effect is noticed when a, beam of light enters a dark room through a slit and becomes visible. This, happens due to the scattering of light by particles of dust in the air., +, , –, , States of Matter, , Notes, , Electrode, , Coagulated sol, particles, As2S3 sol, (negatively charged), , Fig. 8.6 : The Tyndall Effect, , d), , Electrical Properties : The colloidal particles are electrically charged and, carry the same type of charge, either negative or positive. The dispersion, medium has an equal and opposite charge. The colloidal particles therefore, repel each other and do not cluster together to settle down. For example,, arsenious sulphide sol, gold sol, silver sol, etc. contain negatively charged, colloidal particles whereas ferric hydroxide, aluminium hydroxide etc. contain, positively charged colloidal particles. Origin of charge on colloidal particles, is due to:, +, , –, Electrode, , Coagulated sol, particles, As2S3 sol, (negatively charged), , Fig. 8.7 : A set up for Electrophoresis, , CHEMISTRY, , 239
Page 269 :
MODULE - 3, States of Matter, , Solutions, , (a) Preferential adsorption of cations or anions by colloidal particles., (b) Miscelles carry a charge on them due to dissociation., (c) During the formation of colloids especially by Bredig arc method,, colloidal particles capture electrons and get charged. The existence, of charge on a colloidal particle is shown by a process called, electrophoresis., , Notes, , Electrophoresis is a process which involves the movement of colloidal, particles either towards cathode or anode under the influence of, electrical field. The apparatns used is as shown in Fig 8.7., , 8.7 COAGULATION OR PRECIPITATION, The stability of the lyophobic sols is due to the presence of charge on colloidal, particles. If, somehow, the charge is removed, the particles will come nearer, to each other to form aggregates (or coagulate) and settle down under the force, of gravity., The process of settling of colloidal particles is called coagulation or, precipitation of the sol., The coagulation of the lyophobic sols can be carried out in the following ways:, (i) By electrophoresis: The colloidal particles move towards oppositely, charged electrodes, get discharged and precipitated., (ii) By mixing two oppositely charged sols: Oppositely charged sols when, mixed in almost equal proportions, neutralise their charges and get partially, or completely precipitated. Mixing of hydrated ferric oxide (+ve sol) and, arsenious sulphide (–ve sol) bring them in the precipitated forms. This type, of coagulation is called mutual coagulation., (iii) By boiling: When a sol is boiled, the adsorbed layer is disturbed due to, increased collisions with the molecules of dispersion medium. This reduces, the charge on the particles and ultimately lead to settling down in the form, of a precipitate., (iv) By addition of electrolytes: When excess of an electrolyte is added, the, colloidal particles are precipitated. The reason is that colloids interact with, ions carrying charge opposite to that present on themselves. This causes, neutralisation leading to their coagulation., The ion responsible for neutralisation of charge on the particles is called, the coagulating ion. A negative ion causes the precipitation of positively, charged sol and vice versa., , 240, , CHEMISTRY
Page 270 :
MODULE - 3, , Solutions, , It has been observed that, generally, the greater the valence of the, flocculating ion added, the greater is its power to cause precipitation., , States of Matter, , This is known as Hardy-Schulze rule. In the coagulation of a negative, sol, the flocculating power is in the order:, Al3+>Ba2+>Na+, Similarly, in the coagulation of a positive sol, the flocculating power is in, the order:, , Notes, , [Fe(CN)6]4– > PO43– > SO42– > Cl–, The minimum concentration of an electrolyte in millimoles per litre required, to cause precipitation of a sol in two hours is called coagulating value. The, smaller the quantity needed, the higher will be the coagulating power of, an ion., 8.7.1 Coagulation of lyophilic sols, There are mainly two factors which are responsible for the stability of lyophilic, sols. These factors are the charge and solvation of the colloidal particles. When, these two factors are removed, a lyophilic sol can be coagulated. This is done, (i) by adding an electrolyte and (ii) by adding a suitable solvent. When solvents, such as alcohol and acetone are added to hydrophilic sols, the dehydration of, dispersed phase occurs. Under this condition, a small quantity of electrolyte can, bring about coagulation., Protection of colloids, Lyophilic sols are more stable than lyophobic sols. This is due to the fact that, lyophilic colloids are extensively solvated, i.e., colloidal particles are covered, by a sheath of the liquid in which they are dispersed., Lyophilic colloids have a unique property of protecting lyophobic colloids. When, a lyophilic sol is added to the lyophobic sol, the lyophilic particles form a layer, around lyophobic particles and thus protect the latter from electrolytes., Lyophilic colloids used for this purpose are called protective colloids., , 8.8 APPLICATIONS OF COLLOIDAL SOLUTIONS, Colloids play a very important role in our daily life. Some of these applications, are discussed below:, (i), , Sewage disposal : Colloidal particles of dirt, etc. carry electric charge. When, sewage is allowed to pass through metallic plates kept at a high potential,, , CHEMISTRY, , 241
Page 271 :
MODULE - 3, , Solutions, , States of Matter, , the colloidal particles move to the oppositely charged electrode and get, precipitated there. Hence sewage water is purified., (ii) Purification of Water in Wells : When alum is added to muddy water, the, negatively charged particles of the colloid are neutralized by Al3+ ions. Hence, the mud particles settle down and the water can be filtered and used., , Notes, , High voltage electrode, (30000 volts or more), Gases free from, carbon particles, , Smoke, Precipitated carbon., X X XX X X X X XXX X X X, , Fig.8.8 : Cottrell smoke precipitator, , (iii) Smoke Precipitation : Smoke particles are actually electrically charged, colloidal particles of carbon in air. Precipitation of this carbon is done in a, Cottrell’s Precipitator. Smoke from chimneys is allowed to pass through a, chamber having a number of metallic plates connected to a source of high, potential as shown in Fig. 8.8. Charged particles of smoke get attracted to, the oppositively charged electrode and get precipitated and hot purified air, passes out., Other applications in day to day life are :, (i), , Photography : A colloidal solution of silver bromide in gelatin is applied on, glass plates or celluloid films to form photo-senstive plates in photography., , (ii) Clotting of Blood : Blood is a colloidal solution and is negatively charged., On applying a solution of FeCl3 bleeding stops and clotting of the colloidal, particles of blood takes place., (iii) Rubber Plating : Latex is a colloidal solution of negatively charged rubber, particles. The object to be rubber plated is made the anode in the rubber, plating bath. The negatively charged rubber particles move towards the anode, and get deposited on it., , 242, , CHEMISTRY
Page 272 :
Solutions, , (iv) Blue Colour of Sky : Have you ever wondered why is the sky blue? It is, because the colloidal dust particles floating about in the sky scatter blue, light, that makes the sky appear blue. In the absence of these colloidal particles, the sky would have appeared dark throughout., , MODULE - 3, States of Matter, , 8.8 EMULSION AND GEL, Emulsions are colloidal solutions in which both the dispersed phase and dispersion, medium are liquids. However, the two liquids are immiscible, as miscible liquids, will form true solution., , Notes, , Emulsion are of two kinds :, (a) Oil-in-water emulsion : Here the dispersed phase is oil while the dispersion, medium is water. Milk is an example of this kind as in milk liquid fats are, dispersed in water. Vanishing cream is another example., (b) Water-in-oil emulsion : Here dispersed phase is water and dispersion, medium is oil. Butter, cod- liver oil, cold creams are examples of this type., The liquids forming emulsion i.e. oil and water will separate out on keeping as, they are immiscible. Therefore an emulsifying agent or emulsifier is added to, stabilise the emulsion. Soap is a common emulsifier. The preparation of emulsion, in the presence of an emulsifier is called emulsification., How does an emulsifier work? It is believed that an emulsifier gets concentrated, at the interface between oil and water i.e. the surface at which oil and water come, in contact with each other. It acts as a binder between oil and water., Applications of Emulsions - Emulsions play very important role in our daily, life. Some of the common applications are given below :, 1., , The cleansing action of soap and synthetic detergents for washing clothes,, bathing etc is based upon the formation of oil in water type emulsion., , 2., , Milk is an emulsion of fat in water. Milk cream and butter are also emulsions., , 3., , Various cold creams, vanishing creams, body lotions etc. are all emulsions., , 4., , Various oily drugs such as cod liver oil are administered in the form of, emulsion for their better and faster absorption. Some ointments are also in, the form of emulsions., , 5., , The digestion of fats in the intestine occurs by the process of emulsification., , 6., , Emulsions are used for concentrating the sulphide ores by froth flotation, process. Finely powdered ore is treated with an oil emulsion and the mixture, is vigorously agitated by compressed air when the ore particles are carried, to the surface and removed., , CHEMISTRY, , 243
Page 273 :
MODULE - 3, States of Matter, , Notes, , Solutions, , Gels - Gels are the type of colloids in which the dispersed phase is a liquid and the, dispersion medium is a solid. Cheese, jelly, boot polish are common examples of, gel. Most of the commonly used gels are hydrophilic colloidal solution in which, a dilute solution, under suitable conditions set as elastic semi solid masses. For, example 5% aqueous solution of gelatin in water on cooling forms the jelly block., Gels may shrink on keeping by loosing some of the liquid held by them. This is, known as syneresis or resetting on standing., Gels are divided in two categories elastic gels and non elastic gels. Elastic gels, are reversible. When partly dehydrated on loosing water, they change back into, the original form on addition of water. The non elastic gels are not reversible., Gels are useful in many ways. Silica, cheese, jelly, boot polish, curd are commonly, used gels. Solidified alcohol fuel is a gel of alcohol in calcium acetate., , 8.9 NANO MATERIALS, In recent past nano materials have attracted enormous interest because of their, potential for wide ranging applications in number of fields like medicine,, electronics and various industries. They can be metals, ceramics, polymeric, materials or composite materials., A material containing particles with dimension in the size range 1 nm – 100, nm in atleast one direction is termed as nano material. One nanometre is, 10–9m which is extremely small in size. It is about the size of three to five atoms, lined up in a row., Nano materials have been produced and in use for hundreds of years. The, beautiful ruby red colour of some types of glass is due to the presence of nano, particles of gold in it. The decorative lusture found on some articles of medieval, pottery is due to nano particles of some metals in the glaze., Nano materials fall in two categories (i)fullerenes and (ii) inorganic nano, materials., (i) Fullerenes, Fullerenes are allotropes of carbon which are hollow carbon spheres consisting, of large number of carbon atoms bonded chemically like C60., (ii) Inorganic nano particles, Inorganic nano particles are made of metals, semiconductors or oxides with, peculiar electrical, mechanical, optical or chemical properties., , 244, , CHEMISTRY
Page 274 :
MODULE - 3, , Solutions, , Properties, , States of Matter, , Nanomaterials are available in great variety and the range of their properties, and possible applications is enormous., (i) They are used for making miniature batteries, super absorbents, extremely, tiny electronic devices, parts of automobiles and as packaging films., (ii) Nanocapsules and nanodevices present new possibilities for drug delivery,, gene therapy, and medical diagnostics., , Notes, , (iii) Nanocomposites are obtained by mixing a small quantity of nano material, to polymers. For example, adding such small amounts as 2% by volume, of silicate nanoparticles to a polyimide resin increases the latter’s strength, by 100%. Addition of nanoparticles not only improves the mechanical, properties, but has also been shown to improve thermal stability., (iv) Nano materials generally have high plasticity., (v) Due to their large surface, nanoparticles made of transition element oxides, exhibit catalytic properties, (vi) Magnetic nanoparticles show super paramagnetism and have lead to the, discovery of a new class of permanent magnetic materials., , WHAT YOU HAVE LEARNT, z, , Size of the particles in the colloidal state is intermediate between that of, suspension and true solution., , z, , There are eight different types of colloidal systems., , z, , Sols are classified on the basis of (a) interaction between dispersed phase and, dispersion medium (b) molecular size of dispersed phase., , z, , Colloidal solutions are prepared by physical and chemical methods., , z, , The zig zag motion of colloidal particles is called Brownian motion., , z, , Colloidal size particles scatter light and so the path of light becomes visible in, a semi darkened room due to dust particles., , z, , Colloidal particles may carry electric charge., , z, , A colloidal dispersion of a liquid in another liquid is called an emulsion., , z, , A colloidal solution of a liquid dispersed in a solid medium is called a gel., , z, , Colloids are extremely useful to mankind both in daily life and in industry., , z, , Nano materials contain particles with dimension of 1–100 mm in atleast in, one direction. They have special properties and find many applications., , CHEMISTRY, , 245
Page 275 :
MODULE - 3, , Solutions, , States of Matter, , TERMINAL EXERCISE, 1., , List three differences between a true solution and a colloidal solution., , 2., , Describe one method of preparation of, (a) a lyophilic colloid, , Notes, , (b), , a lyophobic colloid, , 3., , What are associated colloids?, , 4., , What is Brownian motion? How does it originate?, , 5., , Why bleeding from a fresh cut stops on applying alum?, , 6., , Two beakers A and B contain ferric hydroxide sol and NaCl solution, respectively. When a beam of light is allowed to converge on them, (in a, darkened room), beam of light is visible in beaker A but not in breaker B., Give the reason. What is this effect called?, , 7., , Define the following terms and give two examples of each, (i) Gel, , (ii) Sol, , 8., , Describe two important applications of colloidal solutions., , 9., , Give two examples of emulsions used in daily life., , 10. Explain the role of emulsifier in an emulsion?, 11. What are nano materials? Give their three applications., , ANSWERS TO THE INTEXT QUESTIONS, 8.1, 1., , 2., , 3., , 246, , Suspension, , – Clay in water, Sand in water, , Colloidal, , – Milk, Blood, Boot polish, Face Cream, Jelly, Foam., , True Solution, , – Sugar in water, , Sol, , – Starch in water, , Gel, , – Silica gel, , Aerosol, , – Fog, , Emulsion, , – Milk, , Alcosol, , – When alcohol is the dispersion medium., , Hydrosol, , – When water is the dispersion medium., , CHEMISTRY
Page 276 :
MODULE - 3, , Solutions, , 4., , True solution, , Colloidal solution, , 1. Size of solute in less than 1 nm., , 1. Particle size (1–100) nm., , 2. Form transparent solution and, allows light to pass through them., , 2. Path of light becomes visible., , States of Matter, , 8.2, 1., , Gold sol, Platinum sol, , 2., , As2S3, Fe(OH)3 (Arsenious sulphide sol, ferric hydroxide sol), , 3., , (a) Lyophilic sol :, , Notes, , 1. easy to prepare, 2. affinity between dispersed phase and dispersion medium., 3. Reversible, Lyophobic, 1. special method used for preparation, 2. No affinity between the two phases., 3. Not reversible, (b) Macromolecular – The size of the colloidal particles large enough to fall in, the colloidal dimensions., Multimolecular – Individually the particles are not of colloidal dimensions, but they aggregate to join together to form molecules of colloidal size., 4., , Refer to section 8.3.2 (c), , CHEMISTRY, , 247
Page 278 :
MODULE - IV, CHEMICAL ENERGETICS, 09. Chemical Thermodynamics, 10. Spontaneity of Chemical Reactions
Page 280 :
MODULE - 4, , Chemical Thermodynamics, , Chemical Energetics, , 9, Notes, , CHEMICAL, THERMODYNAMICS, , When a chemical reaction occurs, it is accompanied by an energy change which, may take any of several different forms. For example, the energy change involved, in the combustion of fuels like kerosene, coal, wood, natural gas, etc., takes the, form of heat and light. Electrical energy is obtained from chemical reactions in, batteries. The formation of glucose, C6H12O6 by the process of photosynthesis, requires the absorption of light energy from the sun. Thus, we see that the energy, change that accompanies a chemical reaction can take different forms. In this, lesson, you shall study the reactions in which heat is either evolved or absorbed., , OBJECTIVES, After reading this lesson, you will be able to:, z, , define the commonly used terms in thermodynamics;, , z, , differentiate between exothermic and endothermic reactions;, , z, , explain the first law of thermodynamics;, , z, , explain internal energy;, , z, , define enthalpy and enthalpy change;, , z, , state the relationship between enthalpy change and internal energy change;, , z, , define enthalpies of formation, combustion, neutralisation, atomisation,, transition, solution and ionisation;, , z, , state the relationship between enthalpy of reaction and enthalpies of formation, of reactants and products;, , CHEMISTRY, , 251
Page 281 :
MODULE - 4, Chemical Energetics, , Chemical Thermodynamics, z, , solve numerical problems based on the enthalpy changes;, , z, , state Lavoisier-Laplace law and Hess’s law;, , z, , calculate enthalpy of a reaction using Hess’s law;, , z, , define bond enthalpy and bond dissociation enthalpy and, , z, , calculate enthalpy of a reaction using bond enthalpy data., , Notes, , 9.1 SOME COMMONLY USED TERMS, In this lesson you would come across some frequently used terms. Let us, understand the meaning of these terms first., 9.1.1 System and Surrounding, If we are studying the reaction of two substances A and B kept in a beaker, the, reaction mixture of A and B is a system and the beaker and the room where it is, kept are surroundings as shown in figure 9.1, , System, , Surrounding, A+B, , Fig 9.1 : System and surroundings, , System is the part of the physical universe which is under study, while the, rest of the universe is surroundings., You know that hot tea/milk (let us call it a system) kept in a stoppered thermos, flask remains hot for a couple of hours. If this flask is made of perfect insulating, material, then there would be no exchange of matter or energy between the system, and the surroundings. We call such a system an isolated system., Isolated system is a system which can exchange neither matter nor energy, with the surroundings., If we keep hot tea/milk in a stoppered stainless steel flask, it will not remain hot, after some time. Here energy is lost to the surroundings through the steel walls,, but due to stopper, the matter will not be lost. We call this system a closed system., Closed system is a system which can exchange energy but not matter with, the surroundings., , 252, , CHEMISTRY
Page 282 :
MODULE - 4, , Chemical Thermodynamics, , If we keep stainless steel flask or thermos flask open, some matter will also be, lost due to evaporation along with energy. We call such a system an open system., Plants, animals, human beings are all examples of open systems, because they, continuously exchange matter (food, etc) and energy with the surroundings., , Chemical Energetics, , Open system is a system which can exchange both energy and matter with, surroundings., Notes, , 9.1.2. State of a System, We describe the state of a system by its measurable properties. For example, we, can describe the state of a gas by specifying its pressure, volume, temperature, etc. These variable properties are called state variables or state functions. Their, values depend only on the initial and final state of the system and not on the path, taken by the system during the change. When the state of a system changes, the, change depends only on the initial and the final state of the system (Fig 9.2)., B (p2 , T2 ), Final State, , I, II, III, , R, , A (p1 , T1 ), Initial State, , T, , Fig. 9.2 : Change of state from initial state to final state through three paths I, II and, III. The difference p2 – p1 and T2 – T1 are independent of the path since pressure and, temperature are state functions., , State functions are those functions which depend only on the state of the, system., Change in state of a system is defined by giving the initial and the final state of the, system. We can understand it by considering another example. We travel from, one point to another. The distance travelled depends on the path or the route we, take. But the separation between these two points on the earth is fixed. Thus,, separation is a state function, but not the distance travelled., 9.1.3 Properties of a System, As stated earlier, the measurable properties of a system are called state variables., They may be further divided into two main types., , CHEMISTRY, , 253
Page 283 :
MODULE - 4, Chemical Energetics, , Chemical Thermodynamics, , (i) Extensive property (variable) is one whose value depends upon the size, of the system. For example, volume, weight, heat, etc., (ii) Intensive property (variable) is one whose value is independent of the size, of the system. For example, temperature, pressure, refractive index,, viscosity, density, surface tension, etc., , Notes, , You may note that an extensive property can become an intensive property by, specifying a unit amount of the substance concerned. For example, mass and, volume are extensive properties, but density (mass per unit volume) and specific, volume (volume per unit mass) are intensive properties., 9.1.4 Types of Processes, Let us first understand what do we mean by a process. Suppose we want to raise, the temperature of the system. We may do it by heating it. Here, heating is the, process., The method of bringing about a change in state is called process., Processes could be of different types. The different types of processes are explained, below., (i) Isothermal process: Ice melts at 273 K and 1 atm pressure. The temperature, does not change as long as the process of melting goes on. Such processes, are examples of isothermal process. We can define isothermal process as, followes., When the temperature of the system remains constant during various, operations, then the process is said to be isothermal. This is attained either, by removing heat from the system or by supplying heat to the system., (ii) Adiabatic Process: If an acid is mixed with a base in a closed thermos, flask, the heat evolved is retained by the system. Such processes are known, as adiabatic processes because the thermos flask does not allow exchange, of heat between the system and the surroundings. Adiabatic process can, be defined as follows:, In an adiabatic process there is no exchange of heat between the system and, the surroundings. Thus, in adiabatic processes there is always a change in, temperature., (iii) Reversible Process: In a reversible process, the initial and the final states, are connected through a succession of equlibrium states. All changes, occuring in any part of the process are exactly reversed when it is carried, out in the opposite direction. Thus both the systems and its surroundings, must be restored exactly to their original state, when the process has been, performed and then reversed., , 254, , CHEMISTRY
Page 284 :
MODULE - 4, , Chemical Thermodynamics, , Let us understand it by an example. Imagine a liquid in equlibrium with its vapour, in a cylinder closed by a frictionless piston, and placed in a constant temperature, bath as shown in figure. 9.3. If the external pressure on the piston is increased by, an infinitesimally small amount, the vapours will condense, but the condensation, will occur so slowly that the heat evolved will be taken up by the temperature, bath. The temperature of the system will not rise, and the pressure above this, liquid will remain constant. Although condensation of the vapor is taking place,, the system at every instant is in the state of equilibrium. If the external pressure is, made just smaller than the vapour pressure, the liquid will vaporize extremely, slowly, and again temperature and pressure will remain constant., , Chemical Energetics, , Notes, , Cylinder with piston, Vapour, Liquid, , Constant temperature bath, , Fig 9.3 : Reversible process, , Reversible processes are those processes in which the changes are carried, out so slowly that the system and surroundings are always in equilibrium., (iv) Irreversible Processes: In the above example rapid evaporation or, condensation by the sudden decrease or incease of the external pressure,, will lead to non-uniformity in temperature and pressure within the system, and the equilibrium will be disturbed. Such processes are called as, irreversible processes., 9.1.5 Standard States, You have seen that a system is described by the state variables. In order to compare, the energies for different compounds, a standard set of conditions is chosen. This, refers to the condition of 1 bar pressure at any specified temperature, with a, substance in its most stable form., , 9.2 EXOTHERMIC AND ENDOTHERMIC REACTIONS, (i) Add a few cm3 of dilute hydrocholoric acid in a test tube containing a few, pieces of granulated zinc and observe the evolution of a gas. Feel the test, tube. It would be hot., CHEMISTRY, , 255
Page 285 :
MODULE - 4, Chemical Energetics, , Chemical Thermodynamics, , (ii) You must have observed that when some water is added to quick lime to, prepare white wash, a lot of heat is evolved., (iii) When a fuel like cooking gas or coal is burnt in air, heat is evolved besides, light. Many chemical reactions lead to release of energy (heat) to the, surroundings. We call these type of rections as exothermic reactions., , Notes, , Exothermic reactions are those reactions which proceed with the evolution, of heat., Let us now consider the following reactions:, (i) Add a small amount of solid ammonium chloride in a test tube half-filled, with water. Shake and feel the test tube. It will feel cold., (ii) Similarly repeat this experiment with potassium nitrate and feel the test, tube, it will feel cold., (iii) Mix barium hydroxide with ammonium chloride in small quantities in water, taken in a test tube. Feel the test tube. It will be cold., In all the above processes we see that heat is absorbed by the system from the, surroundings. Such reactions are called endothermic reactions., Endothermic reactions are those reactions which proceed with the absorption, of heat from the surroundings., , 9.3 THERMOCHEMICAL EQUATIONS, You are familiar with equations for chemical reactions. Now we shall write the, chemical equations which will specify heat energy changes and states of the, reactants and products. These are called the thermochemical equations. For writing, these equations, we follow the conventions listed below:, (i), , The heat evolved or absorbed in a reaction is affected by the physical state, of the reacting substances. Therefore, gaseous, liquid and solid states are, represented by putting symbols (g), (l), and (s) along side the chemical, formulae respectively., For example, to represent burning of methane in oxygen, we write, CH4(g) + 2O2 (g) → CO2 (g) + 2H2O (l) + heat, In writing thermochemical reactions, we denote the amount of heat evolved, or absorbed by a symbol ΔH. The amount of heat evolved or absorbed is, written after the equation followed by semicolon. ΔH is negative for, exothermic reactions and it is positive for endothermic reactions., For example:, An exothermic reaction is written as, CH4 (g) + 2O2 (g) → CO2 (g) + 2H2O (l) ; ΔH = – 891 kJ, , 256, , CHEMISTRY
Page 286 :
MODULE - 4, , Chemical Thermodynamics, , Chemical Energetics, , Whereas an endothermic reaction is written as, H2 (g) + I2 (g) → 2HI (g) ; ΔH = 52.2 kJ, (ii) In case of elements which exhibit allotropy, the name of allotropic, modification is mentioned. For example,, C (graphite), C (diamond), etc., (iii) The substances in aqueous solutions are specified using the symbol (aq)., For example NaCl (aq) stands for an aqueous solution of sodium chloride., , Notes, , (iv) Thermochemical equations may be balanced even by using fractional, coefficients, if so required. The coefficients of the substances of the chemical, equation indicate the number of moles of each substance involved in the, reaction and the ΔH values given correspond to these quantities of substances., (v) In case the coefficients are multipied or divided by a factor, ΔH value must, also be multiplied or divided by the same factor. In such cases, the ΔH value, will depend upon the coefficients. For example, in equation., H2 (g) +, , 1, 2, , O2 (g) → H2O (g) ; ΔH = – 242 kJ, , If coefficients are multiplied by 2, we would write the equation, 2H2 (g) + O2 (g) → 2H2O (g) ; ΔH = 2 (– 242) = – 484 kJ, , 9.4 THE FIRST LAW OF THERMODYNAMICS, You have learnt that chemical reactions are accompanied by energy changes., How do we determine these energy changes? You know that we cannot create or, destroy energy. Energy only changes from one form to another. This is the, observation made by many scientists over the years. This observation has taken, the form of first law of thermodynamics. It has been found valid for various, situations. We state this law as follows:, Energy can neither be created nor destroyed. The total energy of the universe, or an isolated system is constant., Mathematically the first law of thermodynamics is stated as:, ΔU = q + w, , (9.1), , where ΔU = change in internal energy, q = heat absorbed by the system, and w =, work done on the system. These terms are explained as:, 9.4.1 Internal Energy (U), Every system has a definite amount of energy. This amount is different for different, substances. It includes translational, vibrational and rotational energies of, molecules, energy of electrons and nuclei., , CHEMISTRY, , 257
Page 287 :
MODULE - 4, Chemical Energetics, , Notes, , Chemical Thermodynamics, , The internal energy may be defind as the sum of the energies of all the, atoms, molecules or ions contained in the system., It is a state variable. It is not possible to measure the absolute values of internal, energy. However, we can calculate the change in internal energy. If the internal, energy of the system in the initial state is U1 and that in the final state is U2, then, change in internal energy Δ U is independent of the path taken from the intial to, the final state., We can write this change as:, Δ U = U2 – U1, The internal energy of the system can be changed in two ways :, (i) either by allowing heat to flow into the system or out of the system; and, (ii) by work done on the system or by the system, 9.4.2 Heat(q) and Work(w), Heat and work are not state functions. This is because the values of both q and w, depend upon the way in which the change is carried out., Since the law deals with the transfer of heat and work, we assign some signs to, these quantities. Any thing which increases the internal energy of a system is, given a positive sign., Heat given to the system (q) and work done on the system (w) are given positive, signs. Let us illustrate this with an example., If a certain change is accompanied by absorption of 50 kJ of heat and expenditure, of 30 kJ of work,, q = + 50 kJ, w = – 30 kJ, Change in internal energy ΔU = (+ 50 kJ) + (– 30 kJ) = = + 20 kJ, Thus the system has undergone a net increase in the internal energy of –20 kJ., Change in the internal energy of the surroundings wll be 20 kJ., 9.4.3 Work of Expansion, Let us assume that pressure p is constant and the volume of the system changes, from V1 to V2. The work done by a system is given as, w = – p (V2 – V1) = – p Δ V, , 258, , (9.2), , CHEMISTRY
Page 288 :
MODULE - 4, , Chemical Thermodynamics, , (Here we have taken minus sign, because the work is done by the system). Let us, substitute the expression given for w in equation 9.1., , Chemical Energetics, , We get, ΔU=q–pΔV, , (9.3), , If the process is carried ot at constant volume, i.e. Δ V = 0, then, Δ U = qv, , (9.4), , Notes, , The subscript v in qv denotes that volume is constant., The equation 9.4 shows that we can determine internal energy change if we, measure the heat gained or lost by the system at constant volume. However, in, chemistry, the chemical reactions are generally carried out at constant pressure, (atmospheric presure). What do we do then? Let us define another state function,, called, enthalpy., 9.4.4 Enthalpy (H), For measuring heat lost or gained at constant pressure, we define a new state, function called enthalpy. It is denoted by the symbol H and is given by, H=U+pV, , (9.5), , Enthalpy change, Δ H, is given by, Δ H = Δ U + Δ(pV), , (9.6), , or Δ H = Δ U + p Δ V + V Δ p, If the change is carried out at constant pressure, then Δ p = 0. The equation 9.6, will become, Δ H = Δ U + p Δ V (at constant pressure), , (9.7), , Substituting the value of Δ U from equation 9.3 in equation 9.7, we get, ΔH=q–pΔV+pΔV, = q (at constant pressure), We denote q at constant pressure by qp hence, Δ H = qp, , (9.8), , Equation 9.8 shows that by measuring heat lost or gained at constant pressure,, we can measure enthalpy change for any process., 9.4.5 Relation between ΔH and ΔU, For liquids and solids, the difference between ΔrH and ΔrU is not significant but, for gasses, the difference is significant as we will see here., , CHEMISTRY, , 259
Page 289 :
MODULE - 4, Chemical Energetics, , Chemical Thermodynamics, , Let VA be the total volume of the gaseous reactants,, VB the total volume of the gaseous products,, nA the number of moles of gaseous reactants,, and nB the number of moles of gaseous products, at constant pressure and, temperature., , Notes, , Then, using ideal gas law, we can write, p VA = nA RT, , (9.9), , p VB = nB RT, , (9.10), , Subtracting equation 9.9 from equation 9.10, we get, p VB – pVA = nB RT – nA RT = (nB – nA) RT, p (VB – VA) = p Δ V = Δ ngRT, At constant pressure, ΔH=ΔU+pΔV, Therefore Δ H = Δ U + Δ ng RT, Here Δ ng = (number of moles of gaseous products) - (number of moles of gaseous, reactants), Thus we can find the value of Δ H from Δ U or vice versa., For solids and liquids Δ V is very small. We can neglect the term p Δ V, hence Δ H, is nearly the same as Δ U., , INTEXT QUESTIONS 9.1, 1., , Which of the following is false ?, (a) The reaction, H2(g) + Cl2(g) → 2HCl (g) + 185 kJ, is endothermic., (b) Enthalpy change is a state function., (c) Standard state condition for a gaseous system is 1 bar pressure at a, specified temperature., , 2., , For the reaction at 298 K,, 1, 3, N2(g) + H2 (g) → NH3(g) ; Δ H = - 46 kJ, 2, 2, , 260, , CHEMISTRY
Page 290 :
Chemical Thermodynamics, , (a) What is the value of Δng ?, , MODULE - 4, Chemical Energetics, , (b) Calculate the value of Δ U at 298 K?, 3., , Which of the following will increase the internal energy of a system?, (a) Heat given to the system, (b) Work done by the system, , Notes, , 9.5 STANDARD ENTHALPY OF REACTIONS, Let as denote total enthalpy of reactants as Hreactants and total enthalpy of products, as Hproducts. The difference between these enthalpies, Δ H, is the enthalpy of the, reaction, Δ rH = Hproducts – Hreactants, When Hproducts is greater than H reactants then Δ H is positive and heat is absorbed in, the reaction, and the reaction will be endothermic. For example,, H2(g) + I2(g) → 2HI (g); ΔrH = 52.5 kJ, When Hproducts is less than Hreactants then Δ H is negative and heat is evolved in the, reaction, and the reaction will be exothermic. For example,, CH4(g) + 2O2(g) → CO2(g) + 2Η2Ο (l); ΔrH = – 890.4 kJ, Enthalpy of a reaction changes with pressure and temperature. It is convenient to, report enthalpies of the reactions in terms of standard state of substances as we, have defined earlier. When substances are in their standard states, we call the, enthalpy of reaction as standard enthalpy of reaction. It is defined as the enthalpy, change for a reaction, when the reactants and the products are in their standard, states. It is donoted by Δr H 0., 9.5.1 Enthalpy of formation (Δ, Δf H°), The enthalpy change when one mole of a pure compound is formed from its, elements in their most stable states is called the enthalpy of formation and is, denoted by Δf H0., When the reacting elements and the products formed are all in their standard, states, the enthalpy change accompanying the chemical reaction is called the, standard enthalpy of formation and is denoted by Δf H 0. By convention, we take, the standard enthalpy of formation of an element in its most stable state as zero., For example:, C(Graphite) + O2(g) → CO2 (g) ; Δf H0 = – 393.5 kJ mol–1, , CHEMISTRY, , 261
Page 291 :
MODULE - 4, Chemical Energetics, , Chemical Thermodynamics, , This means that carbon dioxide is formed from its elements in their most stable, states. Carbon in the form of graphite and at room temperature and gasesous O2, and CO2 being at 1 bar., 9.5.2 Enthalpy of Combustion (Δ, Δcomb H°), , Notes, , Enthalpy of combustion is the enthalpy change (heat evolved) accompanying, the complete combustion of 1 mole of a substance in oxygen at a given, temperature and 1 bar pressure., For example :, C2H5OH(l) + 3O2(g) → 2CO2(g) + 3H2O(l) ; Δcomb H 0 = – 1365.6 kJ, Enthalpy of combutsion of C2H5OH(l) is – 1365.6 kJ mol–1, 9.5.3 Enthalpy of Neutralization (Δ, Δneut Hº), Enthalpy of neutralization is the enthalpy change (heat evolved) when one mole, of hydrogen ions (H+)is neutralized by one mole of hydroxyl ions (OH-) in dilute, aqueous medium to form water., For example:, H+ (aq) + OH– (aq) → H2O (l) ; Δneut H = –57 kJ/mol, Enthalpy of neutralization of a strong acid with a strong base is always constant, having a value of –57 kJ. However, enthalpy of neutralization of strong acid with, a weak base or weak acid with a strong base will be different, because of varying, degree of ionization of weak acids and bases., 9.5.4 Enthalpy of atomisation (Δ, ΔaH0), It is the change in enthalpy when one mole of a substance is converted into its, atoms in gaseous state at a given temperature and 1 bar pressure, Examples:, (i) C(graphite) ⎯→ C(g), , Δa H 0 = 716.68 kJ mol–1, , In case of elements in solid state, it is equal to the enthalpy of sublimation., (ii) CH4(g) ⎯→ C(g) + 4H(g), , Δa H 0 = 1660 kJ mol–1, , (iii) Hg(l) ⎯→ Hg(g), , Δa H 0 = 61.32 kJ mol–1, , For elements in liquid state, it is equal to the enthalpy of vaporization., 9.5.5 Enthalpy of transition (phase transformation) (Δ, ΔtrsH0), It is the change in enthalpy when one mole of substance changes from one phase, to another at a constant temperature and under 1 bar pressure. Phase transition/, transformation is a general term that includes the following:, 262, , CHEMISTRY
Page 293 :
MODULE - 4, , Chemical Thermodynamics, , Chemical Energetics, , 9.6 LAWS OF THERMOCHEMISTRY, There are two laws of thermochemistry : The Lavoisiter–Laplace law and the, Hess’s Law of Constant Heat Summation., Lavoisier – Laplace Law : When a chemical equation is reversed, the sign of, ΔrH is changed. For example,, Notes, , N2 (g) + O2 (g) → 2NO (g); ΔrH = 180.5 kJ, 2NO (g) → N2 (g) + O2 (g); ΔrH = –180.5 kJ, Hess’s Law of constant heat summation: Hess’s law states that the enthalpy of, reaction is independent of the number and the nature of the intermediate steps., You have learnt that standard enthalpy change of the reaction, C (graphite) + O2(g) → CO2(g), is equal to – 393.5 kJ mol–1. This value can be determined with the help of a, calorimeter. However, there are some reactions for which the direct measurement, of enthalpy in the laboratory is not possible. For example the standard enthalpy, change for the reaction,, C (graphite) +, , 1, O (g) → CO (g), 2 2, , cannot be to determined with the help of calorimeter because the combustion of, carbon is incomplete unless an excess of oxygen is used. If excess of oxygen is, used, some of the CO is oxidized to CO2. How can then we determine the enthalpy, change for such reactions when direct measurement is not possible?, Since Δ H is a state function, it is not dependent on the way the reactions are, carried out. Let us carry out the reactions as follows., (1) First carry out the following reaction and let ΔrH10 be the enthalphy change, of the reaction., C (graphite) + O2 (g) → CO2 (g); ΔrH10 = –393.5 kJ/mol., (2) Now let us write the reaction for which we have to determine the enthalpy, change of the reaction and let it be ΔrH2º, C (graphite) +, , 1, O (g) → CO(g); ΔrH20 = ?, 2 2, , (3) Let us carry out the following reactions and let ΔrH30 be the enthalpy change, of the reaction, CO(g) +, , 264, , 1, O (g) → CO2(g); ΔrH30 = – 283.0 kJ, 2 2, CHEMISTRY
Page 294 :
MODULE - 4, , Chemical Thermodynamics, , We have obtained the products CO2(g) from carbon and oxygen through two, routes, namely first as in step (1) and second as in step (2) + (3)., , Chemical Energetics, , According to Hess’s Law, Δr H10 = Δr H20 + Δr H30, Δr H20 = Δr H10 – Δr H30, , or, , Fig 9.4 shows alternate paths for the conversion of carbon and oxygen to carbon, dioxide., , Notes, , Reactants = C (graphite) + O2(g), , D,H°2, C (graphite) + O2(g), , Path I, , D,H°1, , 1, C(graphite) + 2 O2(g) ® CO(g), , D,H°3, , 1, CO(g) + 2 O2(g), Path II, , Products = CO2(g), , Fig 9.4 : Alternate paths for the conversion of carbon and oxygen to carbon dioxide, , The result of Hess’s law is that thermochemical equations can be added and, substracted just like algebraic equations to get the desired reaction. A useful, practical application of this law is that we can calculate enthalpy changes for the, reactions which cannot be studied directly as in the above case., To obtain the enthalpy change for the reactions, we algebraically combine the, known values of Δr H0 in the same way as the reactions themselves,, C(graphite) + O2 (g) → CO2 (g) ; ΔrH10 = –393.5 kJ/mol, , Thus, , – [CO(graphite) +, C(graphite) +, , 1, O (g) → CO2 (g)]; Δr H30 = – 283.0 kJ/mol, 2 2, , 1, O (g) → CO(g) ; Δr H20 = [ (−393.5) − (−283.0)], 2 2, , = –110.5 kJ/mol, Example 9.1 The heat evolved in the combustion of glucose is shown in the, following equation:, C6H12O6 (s) + 6O2(g) → 6CO2 (g) + 6H2O(l); ΔcombH = – 2840 kJ/mol, How much energy will be required for the production of 1.08 g of glucose?, Soluton: Glucose will be prepared by the reverse reaction., , CHEMISTRY, , 265
Page 296 :
Chemical Thermodynamics, , MODULE - 4, Chemical Energetics, , INTEXT QUESTIONS 9.2, 1. Which of the following is true?, (a) Enthalpy of formation is the heat evolved or absorbed when one gram, of a substance is formed from its elements in their most stable states., (b) When one mole of H+ (aq) and 1 mole of OH— (aq) react, 57.1 kJ of, energy is absorbed., , Notes, , (c) In the thermochemical equation,, C(graphite) + O2 (g) → CO2(g) ; Δf H0 = –394 kJ mol–1, Δ fH0 is known as enthalpy of formation of CO2(g), 2. Calculate the enthalpy change for complete combustion of 29.0 g of butane,, if, C4H10(g) +, , 3, O (g) → 4CO2 (g) + 5H2O (l) ; Δcomb H0 = – 2658 kJ, 2 2, , 3. Calculate the standard enthalpy of the reaction, 2H2S(g) + SO2(g) → 3S (s) + 2H2O (l), given that, Δf H0 (H2S) = – 20.6 kJ mol–1, Δf H0 (SO2) = – 296.9 kJ mol–1, Δf H0 (H2O) = – 289.9 kJ mol–1, , 9.7 BOND ENTHALPIES, In a chemical reaction, you have seen that energy is either absorbed or evolved., Do you know the origin of this change in energy? You know that bonds are, broken and new bonds are formed in chemical reactions. Energy changes take, place in breaking some bonds of the reactants and in forming new bonds of the, products. So the energy changes in a chemical reaction are a result of energy, changes in breaking and forming of bonds. Let us consider the gaseous reactions,, because in these, we encounter the energy changes due to breaking and forming, of bonds only., At high temperature, hydrogen molecules dissociate into atoms as, H2(g) → H (g) + H(g) ; ΔrH = 435 kJ/mol, The heat absorbed in this reaction is used to break the chemical bonds holding the, hydrogen atoms together in the H2 molecules. For a diatomic molecule like H2(g),, CHEMISTRY, , 267
Page 297 :
MODULE - 4, Chemical Energetics, , Chemical Thermodynamics, , we define bond dissociation energy as the enthalpy change of the reaction in, which one mole of the gaseous molecules are dissociated into gaseous atoms., Now, let us consider a polyatomic molecule like H2O(g). The dissociation may, involve fragmenting the molecules into an atom and a group of atoms, called a, radical, as in, , Notes, , H2O (g) → H (g) + OH (g) ; ΔrH0 = 502 kJ/mol, OH(g) → O(g) + H (g) ; ΔrH0 = 427 kJ/mol, In the first reaction, one of the two OH bonds in H2O (g) dissociates with an, enthalpy change of 502 kJ/mol and in the second reaction, second OH bond, dissociates with an enthalpy change of 427 kJ/mol. It is clear that the dissociation, energy of the O – H bond is sensitive to its environment. However, the difference, is not very large. We take the average value (464.5 kJ/mol in this case) in case of, polyatomic molecules and call it bond enthalpy., Bond enthalpy is defined as the average amount of enthalpy change involved, in the dissociation of one mole of bonds present in different gaseous, compounds., Now you know the distincton between bond dissociation enthalpy and bond, enthalpy. Bond dissociation enthalpy refers to breaking a particular bond in a, particular molecule whereas bond enthalpy is the average value of bond dissociation, energies for a given type of bond. The bond enthalpies of some bonds are listed in, table 9.1., By using bond enthalpies (B.E.) it is possible to estimate the energy released, when a gaseous molecule is formed from its gaseous atoms. For example, the, energy released at constant pressure for the reaction (ΔrH),, 3H(g) + C(g) + Cl(g) → CH3Cl(g), is the sum of the energies of three C – H bonds, and one C – Cl bond, all taken, with a negative sign because energy is released. Using the values of bond enthalpies, (B.E.) from table 9.1 we get,, ΔrH = – 3 × B.E. (C – H) – B.E. (C – Cl), = (–3 × 415 – 335) kJ mol–1, = (–1245 – 335) kJ mol–1, = – 1574 kJ mol–1, We will now show you how to use bond enthalpy data to estimate the enthalpy of, a reaction, when direct calorimetric data are not available. Note that in section, 9.7 we used enthalpy of formation data to estimate enthalpy of a reaction. In, , 268, , CHEMISTRY
Page 298 :
MODULE - 4, , Chemical Thermodynamics, , principle, bond enthalpy data can be used to calculate ΔrH for a chemical reaction, occuring in gaseous state by making use of difference in energy absorbed in, breaking the bonds in reactants and energy released in formation of bonds in, products., ΔrH = Σ B.E. (reactants) – Σ B.E. (products), , Chemical Energetics, , (9.10), , Table 9.1 : Average Bond enthalpies, , Notes, -1, , BOND, , BOND ENTHALPY /(kJ mol ), , H–H, , 435, , C–H, , 415, , C – Br, , 284, , C–C, , 356, , C=C, , 598, , Br – Br, , 193, , Cl – Cl, , 242, , C – Cl, , 339, , F–F, , 155, , H – Cl, , 431, , H–O, , 462, , H–N, , 390, , H–F, , 563, , H – Br, , 366, , H–I, , 296, , C–O, , 355, , C=O, , 723, , C–N, , 391, , C=N, , 619, , C ≡ N, , 878, , C ≡ C, , 832, , Example 9.3: Use bond enthalpy data given in table 9.1 to calculate the enthalpy, of the reaction., CH3 (g) + Cl2 (g) → CH3Cl (g) + HCl (g), Solution: 1. Write the equation using structural formula, H, , H, , H – C – H + Cl – Cl ® H – C – Cl + H – Cl, H, , CHEMISTRY, , H, , 269
Page 299 :
MODULE - 4, Chemical Energetics, , Chemical Thermodynamics, , 2. List the bonds broken and bonds formed under the equation as shown, H, , H, H – C – H + Cl – Cl, , H – C – Cl + H – Cl, H, , H, , Notes, , number of bonds broken, , number of bonds formed, , C–H=4, , C – Cl = 1, , Cl – Cl = 1, , H – Cl = 1, C–H=3, , 3. Look up the values of bond enthalpies for the bonds in the reactants and, products and list them as shown, Reactants, , Products, –1, , B.E. (C – H) = 435 kJ mol, , B.E. (Cl – C) = 339 kJ mol–1, , B.E. (Cl – Cl) = 242 kJ mol–1, , B.E. (H – Cl) = 431 kJ mol–1, B.E. (C – H) = 435 kJ/mol, , 4. Use equation 9.10,, Enthalpy of the rection Δr H = Σ B.E. (reactants) - Σ B.E. (products), = 4[B.E. (C – H) + B.E. (C – Cl)] – [B.E. (C – Cl) + B.E., (H – Cl) + 3 B.E. (C – H)], = [4 × 435 + 242] – [339 + 431 + 3 × 435] = – 93 kJ, Now let us take one example in which we calculate the enthalpy of a reaction, using, (i) Enthalpy of formation data, (ii) Bond enthalpy data., Example 9.4: Calculate Δr H° for the reaction, Cl2 (g) + 2HF (g) → 2HCl (g) +F2 (g), (a) Using enthalpy of formation data, Δf H° (HCl) = – 92.5 kJ, Δf H° (HF) = – 269 kJ, (b) Using bond enthalpy data, B.E. (H – Cl) = 431 kJ mol–1, B.E. (F – F) = 155 kJ mol–1, , 270, , CHEMISTRY
Page 301 :
MODULE - 4, , Chemical Thermodynamics, , Chemical Energetics, , 1, H (g) → H (g);, 2 2, , Δr H0 = 218 kJ mol–1, , 1, N (g) → N(g);, 2 2, , Δr H0 = 973 kJ mol–1, , 3. Calculate the enthalpy of the reaction, Notes, , H2 (g) + Cl2 (g) → 2HCl (g), given;, Bond enthalpy (H - H) = 435 kJ mol-1, Bond enthalpy (Cl - Cl) = 242 kJ mol-1, Bond enthalpy (H - Cl) = 431 kJ mol-1, , WHAT YOU HAVE LEARNT, , 272, , z, , System is the part of the physical universe which is under study, while the, rest of the universe is surroundings., , z, , Isolated system is a system which can exchange neither matter nor energy, with the surroundings., , z, , Closed system is a system which can exchange energy but not the matter, with the surroundings., , z, , Open system is a system which can exchange both energy and matter with, the surroundings., , z, , State functions are those functions which depend only on the state of the, system., , z, , Extensive properties depend upon the size of the system whereas intensive, properties do not depend upon the size of the system., , z, , When the temperature of the system is kept constant during various operations, then the process is said to be isothermal., , z, , In an adiabatic process there is no exchange of heat between the system and, the surroundings., , z, , Reversible processes are those processes in which the changes are carried, out so slowly that the system and surrounding are always in equilibrium., , z, , Exothermic reactions are those reactions which proceed with the evolution, of heat., , CHEMISTRY
Page 302 :
MODULE - 4, , Chemical Thermodynamics, z, , Endothermic reactions are those which proceed with absorption of heat from, the surroundings., , z, , First law of Thermodynamics states that energy can neither be created nor, destroyed., , z, , Internal energy is the sum of the energies of all the atoms, molecules or ions, contained in the system., , z, , The state function enthalpy (H) is given by the relation H = U + PV., , z, , When a chemical equation is reversed, the sign of Δ H is also reversed., , z, , The enthalpy of reaction is independent of the number and nature of the, intermediate steps., , z, , Bond enthalpy is the average amount of bond dissociation enthalpies for a, given type of bond present in different gaseous compounds, when one mole, of bonds is broken in the gaseous state., , Chemical Energetics, , Notes, , TERMINAL EXERCISE, 1., , Enthalpy of combustion of ethyl alcohol, C2H5OH, is – 950 kJ mol–1. How, much heat is evolved when one gram of ethyl alcohol burns?, , 2., , Given :, C2H2(g) +, , 5, O (g) → 2CO2 (g) + H2O(l) ; ΔcombH = – 1299 kJ/mol, 2 2, , C(graphite) + O2 (g) → CO2(g) ; ΔfH = – 393 kJ/mol, H2(g) +, , 1, O (g) → H2O (l) ; ΔfH = – 285.5 kJ/mol, 2 2, , Calculate enthalpy of formation of C2H2(g), 3., , Calculate the enthalpy of combustion of propane, C3H8 (g) + 5O2(g) → 3CO2(g) + 4H2O (l), Given the following:, H2(g) +, , 1, O (g) → H2O (l);, 2 2, , ΔfH = – 285.5 kJ/mol, , C(s) + O2(g) → CO2(g);, , ΔfH = – 393 kJ/mol, , 3C (s) + 4H2(g) → C3H8(g);, , ΔfH = – 104 kJ/mol, , CHEMISTRY, , 273
Page 305 :
MODULE - 4, , Spontaneity of Chemical Reactions, , Chemical Energetics, , 10, Notes, , SPONTANEITY OF CHEMICAL, REACTIONS, , We have studied about the first law of thermodynamics in lesson 09. According, to this law the processes occur in such a way that the total energy of the universe, remains constant. But it does not tell us whether a specified change or a process, including a chemical reaction can occur spontaneously i.e., whether it is feasible, or not. For example, the first law does not deny the possibility that a metal bar, having a uniform temperature can spontaneously become warmer at one end and, cooler at the other. But it is known from experience that such a change does not, occur without expenditure of energy from an external source., The first law also states that energy can be converted from one form into an, equivalent amount of energy of another form. But it does not tell that heat energy, cannot be completely converted into an equivalent amount of work without, producing some changes elsewhere. In this lesson you shall learn to predict whether, a given process or a chemical reaction can occur spontaneously or not., , OBJECTIVES, After reading this lesson you will be able to :, z, z, , define entropy;, recognise that entropy change in a system is given by, ΔS=, , z, , z, , 276, , qrev, T, , state entropy criterion for a spontaneous process Δ Suniverse > 0 and at, equilibrium Δ Suniverse = 0, state the second law of thermodynamics., CHEMISTRY
Page 306 :
Spontaneity of Chemical Reactions, z, , state the third law of thermodynamics;, , z, , state the relationship between G, H and S ;, , z, , derive the relation Δ Gsystem = T Δ Ssystem ;, , z, , state Gibbs energy criterion for spontaneous process, , MODULE - 4, Chemical Energetics, , Δ G < 0 for a spontaneous process, Δ G = 0 at equilibrium, , Notes, , Δ G > 0 for a non-spontaneous process, z, , define standard Gibbs energy of formation of a subtance;, , z, , relate the standard Gibbs energy change with the equilibrium constant and, , z, , solve numerical problems., , 10.1 SPONTANEOUS AND NON-SPONTANEOUS, PROCESSES, We know that hot water kept in a container cools down by losing heat to the, surroundings. On the other hand, water at room temperature cannot become hot, by gaining heat from the surroundings. It can be made hot by heating it over a gas, burner. The cooling down of hot water is an example of a spontanneous process., Heating of water (at room temperature) is an example of a non-spontaneous, process because an outside agency (gas burner) has been used., A spontaneous process is a process that occurs in a system by itself; once started,, no action from outside the system (outside agency) is necessary to make the, process continue. A non-spontaneous process will not take place unless some, external action is continuously applied. Let us consider another example, we, know that when iron objects are exposed to moist atmosphere, rusting of iron, takes place. Although the rusting of iron is a slow process but it always takes place in, the same direction. We say that the rusting of iron is a spontaneous process. During, rusting of iron, iron is oxidised to iron (III) oxide., → 2Fe2O3 (s), 4 Fe(s) + 3O2(g) ⎯⎯, , The reverse of the above reaction is also possible but it is non-spontaneous. An, external agency has to be used to reduce iron (III) oxide to iron., From our discussion it can be concluded that, z, , if a process is spontaneous, the reverse process is non-spontaneous., , z, , all the spontaneous processes and most of the non-spontaneous processes, are possible., , z, , spontaneous processes occur naturally whereas non-spontaneous processes, require the help of an outside agency to occur., , CHEMISTRY, , 277
Page 307 :
MODULE - 4, , Spontaneity of Chemical Reactions, , Chemical Energetics, , 10.2 ENTROPY, In fig 10.1(a) the bulb ‘I’ contains 1 mol of an ideal gas ‘A’ at a pressure of 1 bar, and the bulb II contains 1 mol of another ideal gas ‘B’ at 1 bar. The two bulbs are, joined together through a valve., , Notes, , I, , (a), , II, , (b), Fig 10.1, , When the valve between the two bulbs is opened [Fig 10.1 (b)], the two gases, mix spontaneously. The mixing of gases continues until the partial pressure of, each gas becomes equal to 0.5 bar in each bulb i.e., the equilibrium is attained., We know from experience that the process cannot be reversed spontaneously the gases do not unmix on their own. What is the driving force behind this process?, We know that the internal energy (U) and enthalpy (H) of an ideal gas depend, only upon the temperature of the gas and not upon its pressure or volume. Since, there are no intermolecular forces in ideal gases, Δ U = Δ H = 0 when ideal gases, mix at constant temperature. Thus, energy change is not the driving force behind, the spontaneous mixing of ideal gases. The driving force is simply the tendency, of the molecules of the two gases to achieve maximum state of mixing, i.e.,, disorder. The thermodynamic property related to the disorder of the system is, called entropy. It is denoted by the symbol S., The entropy is the measure of disorder or randomness in a system. The, greater the disorder in a system, the greater is the entropy of the system., For a given substance,, (i) the crystalline state is the most ordered state, hence its entropy is the lowest., (ii) the gaseous state is the most disordered state, hence its entropy is the, maximum, and, (iii) the disorder in the liquid state is intermediate between the solid and the, gaseous state., Entropy of any substance increases on heating. In a chemical reaction, entropy, increases if there is an increase in number of moles of gases during the reaction, and vice-versa., When a system changes from one state to another, the change of entropy ΔS is, given by, ΔS=, , qrev, T, , (10.1), , where qrev is the heat supplied reversibly at a constant temperature T., 278, , CHEMISTRY
Page 308 :
MODULE - 4, , Spontaneity of Chemical Reactions, , Chemical Energetics, , 10.3 CRITERIA FOR SPONTANEOUS CHANGE : THE, SECOND LAW OF THERMODYNAMICS, So far we have studied about internal energy, enthalpy and entropy. Can we define, the spontaneity of a process in terms of these properties? Let us see whether, these changes in properties can be used as a criterion for determining the, spontaneity of a process or not., , Notes, , (i) We know that most of the processes which occur spontaneously are, exothermic. Water kept in a container at room temperature evaporates, spontaneously. It is an endothermic process. Thus enthalpy change cannot, be used as a criteria for spontaneous change., (ii) Can we use the increase of entropy as a criteria for the spontaneous change?, Then how do we explain the spontaneous freezing of water at –10°C? We, know that crystalline ice is more ordered then the liquid water and therefore, the entropy must decrease. The answer to this question is that we must, consider simultaneously two entropy changes:, (a) the entropy change of the system itself, and, (b) the entropy change of the surroundings, Δ Stotal = Δ Suniv = Δ Ssystem + Δ Ssurroundings > 0, , (10.2), , The equation is one of the many forms of the second law of thermodynamics., According to the second law of thermodynamics all spontaneous or natural, processes produce an increase in entropy of the universe., Thus, for a spontaneous process when a system is at equilibrium, the entropy is, maximum, and the change in entropy is zero, Δ S = 0 (at equilibrium), , (10.3), , 10.4 ENTROPY CHANGE IN PHASE TRANSITIONS, When a solid melts and produces liquid, the process occurs at the melting point, of the solid. For example, ice melts at 273 K and produces water at the same, temperature., 273K, H 2 O(s) ⎯⎯, ⎯→ H 2 O(l ), , The heat involved in the process of melting a solid is called enthalpy of fusion, (ΔfusH). Therefore, the entropy of fusion (ΔfusS) is given by, ΔfusS =, , Δfus H, T, , (Q qrev at const p = ΔfusH), , where T is the melting point of the solid., CHEMISTRY, , 279
Page 309 :
MODULE - 4, Chemical Energetics, , Spontaneity of Chemical Reactions, , Similarly, for the equilibrium, 373K, H 2 O(l) ⎯⎯, ⎯→ H 2 O(g), , ΔvapS =, Notes, , Δ vap H, T, , , (T is the boiling point of the liquid.), , Example 10.1: The enthalpy change for the transition of liquid water to steam at, 373 K is 40.8 kJ mol-1. Calculate the entropy change for the process, 373 K, Solution: H2O (l) YZZZ, ZZZZ, X, Z H2O(g), , Δ vapS =, , Δ vap H, T, , Δ vapH = 40.8 kJ mol-1 = 40.8 × 103 J mol-1, T = 373 K, 40.8×103 J mol-1, = 109 J K-1 mol-1, Δ vapS =, 373 K, , INTEXT QUESTIONS 10.1, 1. The enthalpy change for the transition of ice to liquid water at 273 K is 6.02, kJ mol–1. Calculate the entropy change for the process., 2. Arrange the following systems in the order of increasing randomness,, (i) 1 mol of gas A, (ii) 1 mol of solid A, (iii) 1 mol of liquid A, 3. Indicate whether you would expect the entropy of the system to increase or, decrease, (a) 2SO2 (g) + O2 (g) → 2SO3 (g), (b) N2 (g) + 3H2 (g) → 2NH3 (g), (c) O2 (g) → 2O (g), , 10.5 THIRD LAW OF THERMODYNAMICS AND, ABSOLUTE ENTROPY, When temperature of a substance is increased, the translational, vibrational and, rotational motions become more vigorous. It leads to greater disorder and as a, , 280, , CHEMISTRY
Page 310 :
MODULE - 4, , Spontaneity of Chemical Reactions, , result the entropy of the substance increases. Thus, on increasing the temperature, of a substance the entropy of a substance increases. It decreases on decreasing, the temperature of a substance., The entropy of a pure and perfectly crystalline solid at absolute zero temperature, is zero. This is known as the Third law of thermodynamics. This third law of, thermodynamics helps us to calculate the absolute value of molar entropies (Sm), of substances at different temperatures. The standard molar entropies of some, substances at 298 K are given in Table 10.1., , Chemical Energetics, , Notes, , Table 10.1 : Standard molar entropies (Sm0/J K–1 mol–1) at 298 K, Solids, , Entropy, , Liquids, , Entropy, , Gases, , Entropy, , C (graphite), , 5.7, , H2O, , 69.9, , H2, , 130.7, , C (diamond), , 2.4, , Hg, , 76.0, , O2, , 205.1, , Fe, , 27.3, , C2H5OH, , 160.7, , N2, , 191.6, , Pb, , 64.8, , C6H6, , 173.3, , CO2, , 213.7, , Cu, , 33.1, , CH3COOH, , 159.8, , NO2, , 240.1, , Al, , 96.2, , N 2O 4, , 304.3, , 360.8, , NH3, , 192.3, , 92.9, , CH4, , 186.2, , C12H22O11, CaCO3, , Entropy Change for a Reaction, The absolute entropies can be used for calculating standard entropies changes, accompanying chemical reaction. It can be determined by subtracting the standard, entropies of reactants from those of products. Thus, for a general reaction, aA + bB + …… → pP + qQ + …, ΔSm0 = [pSm0 (P) + qSm0 (Q) + ....] – [aSm0 (A) + bSm0 (B) + ...], ΔrSm0 = Σ Sm0 (products) – Σ Sm0 (reactants), Example 10.2 : Calculate the entropy change, ΔrSm0 for the following reaction, at 298K., 4Fe(s) + 3O2(g) → 2Fe2O3(s), Given that the standard molar entropies of Fe(s), O2(g), and Fe2O3(s) at 298 K, are 27.3, 205.0 and 87.4 J K–1 mol–1 respectively., Solution : 4Fe(s) + 3O2(g) → 2Fe2O3(s), Δ r S o = ∑ vP Smo (products) − ∑ vR S o m (reactants), , CHEMISTRY, , 281
Page 312 :
MODULE - 4, , Spontaneity of Chemical Reactions, , Since H, T and S are state functions, it follows that G is also a state function., Comparing equations 10.7 and 10.9, we find that, Δ G = – T Δ Stotal, , Chemical Energetics, , (10.10), , We have seen that if Δ Stotal is positive, the change will be spontaneous. Equations, 10.10 can be used to predict the spontaneity of a process based on the value of, ΔG., , Notes, , The use of Gibbs energy has the advantage that it refers to system only. Thus for, a process occurring at constant temperature and pressure, if, Δ G < 0 (negative), the process is spontaneous, Δ G > 0 (positive), the process is non-spontaneous, Δ G = 0 (zero), the process is at equilibrium, In deciding the spontaneity of a chemical reaction, the equation Δ G = Δ H –T Δ, S takes into account two factors (i) the energy factor ΔH, and (ii) the entropy, factor ΔS. Based on the signs of Δ H and Δ S there are four possibilities for Δ G., These possibilities are outlined in table 10.2, ΔS, Table 10.2 Criterion for spontaneous change : ΔG = ΔH –TΔ, S.No, , ΔH, , ΔS, , ΔG, , 1, , –, , +, , –, , Spontaneous at all temperatures., , 2., , –, , –, , –, , Spontaneous at low temperatures., , +, , Non-spontaneous at high temperatures., , +, , Non-spontaneous at low temperatures., , –, , Spontaneous at high temperatures., , +, , Non-spontaneous at all temperatures., , 3., , 4., , +, , +, , +, , –, , Result, , Example 10.3 : For the reaction, 2NO (g) +O2 (g) → 2NO2 (g), Calculate ΔrG at 700 K when enthalpy and entropy changes (ΔrH and ΔrS) are, respectively – 113.0 kJ mol-1 and –145 JK-1 mol-1, Solution : Given that, Δ H = – 113.0 kJ mol–1, Δ S = – 145 JK–1 mol–1 = – 145 × 10–3 kJ K–1 mol–1, T = 700 K, Substituting the values in, ΔG = ΔH–TΔS, , CHEMISTRY, , 283
Page 313 :
MODULE - 4, Chemical Energetics, , Spontaneity of Chemical Reactions, , Δ G = (– 113.0 kJ mol–1) – (700 K) (– 145 × 10–3 kJ K–1 mol–1), = (– 113.0 kJ mol–1) + (101.5 kJ mol–1), = – 11.5 kJ mol–1, , Notes, , INTEXT QUESTIONS 10.2, 1. Determine whether the following reaction, CCl4 (l) + H2 (g) → HCl (g) +CHCl3 (l), is spontaneous at 298 K if ΔrH = 91.35 kJ mol–1 and ΔrS = 41.5 JK–1 mol–1, for this reaction., 2. Which of the following conditions would predict a process that is always, spontaneous?, (i) ΔH > 0, Δ S > 0, (ii) Δ H > 0, Δ S < 0, (iii) Δ H < 0, Δ S > 0, (iv) Δ H < 0, Δ S < 0, , 10.7 STANDARD GIBBS ENERGY CHANGE (Δ, ΔGº) AND, EQUILIBRIUM CONSTANT (K), The standard Gibbs energy change is defined as the change in Gibbs energy for, the process in which the reactants in their standard states are converted into the, products in their standard states. It is denoted by the symbol ΔrG°., The value of ΔrG° can be found from the standard Gibbs energy of formation of, substances., The standard Gibbs energy of formation of a compound is defined as the change, in Gibbs energy when 1 mole of the compound is formed from its constituent, elements in their standard states. Like the standard enthalpy of formation of an, element, the standard Gibbs energy of formation of an element in its standard, state is taken as zero., Thus for a reaction, aA + bB + .... → pP + qQ + ...., ΔrG° = (p Δf GPo + q Δf GQo + ....) – (a Δf GAo + b Δf GBo + ....), ΔrG° = ∑ vR ΔfG° (products) – ∑ vR Δ f G° (reactants), 284, , CHEMISTRY
Page 314 :
MODULE - 4, , Spontaneity of Chemical Reactions, , The standard Gibbs energy change (ΔrG°) is related to the equilibrium constant, (K) of the reaction by the expression, , Chemical Energetics, , ΔrGo = – RT ln K = – 2.303 RT log K, Example 10.4 : The equilibrium constant of the reaction, P(s) +, is 2.00 ×, , 1024, , 3, Cl2 (g), 2, , PCl3 (g), , Notes, , at 500 K. Calculate the value of ΔrG°., , Solution : Given that, K = 2.00 × 1024, T = 500 K, ΔrG° = – 2.303 RT log K, = – 2.303 × (8.314 J mol–1 K–1) (500 K) log 2.0 × 1024, = – 2.303 × (8.314 J mol–1 K–1) (500 K) 24.30, = – 232.6 kJ mol–1, Example 10.5 : Calculate the standard Gibbs energy change for the reaction, CH4 (g) + 2O2 (g) ⎯⎯, → CO2 (g) + 2H2O (l), at 298K. The standard Gibbs energies of formation of CH4, CO2 and H2O at, 298K are – 50.8 kJ mol–1, –394.4 kJ mol–1, and –237.2 kJ mol–1 respectively., Solution : CH4 (g) + 2O2 (g) → CO2 (g) + 2H2O (l), ΔrG° = ΔfG°(CO2) + 2 ΔfG° (H2O) – ΔfG° (CH4) – 2ΔfG° (O2), = – 394.4 + 2 × (– 237.2) – (–50.8) – 2 × 0, = – 394.4 – 474.4 + 50.8, = –818 kJ mol–1, , INTEXT QUESTIONS 10.3, 1., , What is the relationship between the standard Gibbs energy change and the, equilibrium constant of the reaction?, , 2., , The standard Gibbs energy change for the reaction, CO (g) + 2H2 (g) ⎯⎯, → CH3OH (l), at 298 K is – 24.8 kJ mol-1. What is the value of the equilibrium constant at, 298 K?, , CHEMISTRY, , 285
Page 315 :
MODULE - 4, , Spontaneity of Chemical Reactions, , Chemical Energetics, , WHAT YOU HAVE LEARNT, , Notes, , z, , All spontaneous processes lead to an increase in disorder or randomness, , z, , The thermodynamic function related to disorder in a system is called entropy,, S., , z, , According to the second law of thermodynamics for a spontaneous change, the total entropy change of the system and the surroundings must increase., , z, , Absolute entropies of different substances can be calculated with the help of, the third law of thermodynamics which states that the entropy of a pure and, perfectly cystalline solid is zero at absolute zero temperature., , z, , Gibbs energy is defined as G = H – TS, , z, , At a constant temperature, the change in Gibbs free energy is related to, enthalpy and entropy changes by the expression, ΔG = ΔH – T ΔS, For a spontaneous change, there must be a decrease in Gibbs energy, i.e.,, ΔG < 0., At equilibrium ΔG = 0, The standard Gibbs energy change is related to the equilibrium constant of, the reaction by the expression, ΔrG° = –2.303 RT log K, , •, , The standard Gibb’s energy change is given by, ΔrG° = ∑ vP Δ f G 0 (products) – ∑ vR Δ f G 0 (reactants), , TERMINAL EXERCISE, 1., , What do you call the measure of disorder or randomness in a system?, , 2., , Predict the sign of Δ S for each of the following processes., (i) H2 (g) → 2H (g), (ii) O2 (g, 300 K) → O2 (g, 500 K), , 286, , 3., , Define entropy., , 4., , Explain why entropy is not a good criteria for determining the spontaneity, of a process?, CHEMISTRY
Page 316 :
Spontaneity of Chemical Reactions, , 5., , What is the relationship between the enthalpy and the entropy change for a, system at equilibrium?, , 6., , For the reaction, , MODULE - 4, Chemical Energetics, , O3 (g) + O (g) → 2O2 (g), ΔrH = - 391.9 kJ mol–1 and ΔrS = 10.3 J K-1 mol–1 at 298 K. Calculate ΔrG, at this temperature and state whether the reaction is spontaneous or not., 7., , Notes, , What happens to ΔrG during, (a) a spontaneous process, (b) a non-spontaneous process, (c) a process at equilibrium, , 8., , Calculate ΔrG° at 298 K for the reaction, 2NO2 (g) → N2O4 (g), Given ΔrH = - 57.20 kJ mol–1 and ΔrS = - 175.8 J K-1 mol–1, Is this reaction spontaneous?, , 9., , The standard Gibbs energies of formation at 298 K are – 202.85 kJ mol-1 for, NH4Cl (s), – 16.45 kJ mol–1 for NH3 (g) and – 95.3 kJ mol–1 for HCl (g), (a) What is ΔrG° for the reaction, NH4Cl (s) → NH3 (g) + HCl (g), (b) Calculate the equilibrium constant for this decomposition., , 10. For the following reaction, CCl4 (l) + H2 (g) → HCl (g) + CHCl3 (l), ΔrG° = – 103.7 kJ mol–1 at 298 K. Calculate the equilibrium constant for, this reaction., , ANSWERS TO INTEXT QUESTIONS, 10.1, 1., , 6.02 kJ mol−1, 6.02 × 103 J mol−1, Δ fus H, ΔfusS =, =, =, 273 K, 273 K, T, , = 22.0 J mol-1 K-1, , CHEMISTRY, , 287
Page 317 :
MODULE - 4, Chemical Energetics, , Spontaneity of Chemical Reactions, , 2., , 1 mol of solid, 1 mol of liquid, 1 mol of gas., , 3., , Decrease (b) Decrease (c) Increase, , 10.2, , Notes, , 1., , ΔG = –103.7 kJ. Therefer the reaction is spontaneous., , 2., , (iii), , 10.3, , 288, , 1., , ΔGº = –2.303 RT log K, , 2., , 2.2 × 104, , CHEMISTRY
Page 318 :
MODULE - V, CHEMICAL DYNAMICS, 11. Chemical Equilibrium, 12. Ionic Equilibrium, 13. Electrochemistry, 14. Chemical Kinetics, 15. Adsorption and Catalysis
Page 320 :
MODULE - 5, , Chemical Equilibrium, , Chemical Dynamics, , 11, Notes, , CHEMICAL EQUILIBRIUM, , When reactants are mixed in exact stoichiometric proportion to perform a, chemical reaction, it is believed that all the reactants would be converted into, products with the release or absorption of energy. This is not true in all cases., Many chemical reactions proceed only to a certain extent and stop. When analysed,, the resulting mixture contains both the reactants and products. It is because when, reactants combine to form products, the products also start combining to give back, the reactants., When such opposing processes take place at equal rates, no reaction appears to, take place and it is said that a state of equilibrium has reached. In this lesson, we, will examine many aspects of chemical equilibrium. We shall also discuss how, can we control the extent to which a reaction can proceed by changing the various, conditions of the equilibrium., , OBJECTIVES, After reading this lesson you will able to :, z, differentiate between static and dynamic equilibrium;, z, z, z, z, z, z, , identify and differentiate between reversible and irreversible reactions;, list and explain characteristics of equilibrium state;, explain the equilibria involving physical changes and their characterstics;, differentiate between equilibrium in homogeneous and tieterogeneous, symbols;, state and explain the law of mass action;, apply the law of equilibrium and write expression of equilibrium constant, for different types of chemical reactions namely, homogeneous and, heterogenous;, , CHEMISTRY, , 291
Page 321 :
MODULE - 5, Chemical Dynamics, , Chemical Equilibrium, z, z, , state and derive the relation between Kc and Kp and carry out some calculations, involving them and, list the factors which affect the state of equilibrium and state and apply, Le-Chatelier principle., , 11.1 STATIC AND DYNAMIC EQUILIBRIUM, Notes, , The state of equilibrium can be observed in physical and chemical systems. Also,, equilibrium can be static or dynamic in nature. A book lying on the table is an, example of static equilibrium. The forces of action and reaction cancel each, other and no change takes place. Thus it is a case of static equilibrium. On the, other hand, when an escalator is coming down and a passenger is going up at the, same speed it is a case of dynamic equilibrium. Here, because both are moving, in opposite directions and at the same speed, no net change takes place. The, equilibrium established in the above examples are in physical systems., , 11.2 REVERSIBLE AND IRREVERSIBLE REACTIONS, Chemical reactions can be classified as : Reversible and Irreversible reactions., 11.2.1 Reversible reactions, Consider the reaction between ethanol and acetic acid. When mixed in the, presence of dilute sulphuric acid they react and form ethyl acetate and water., +, , H, C2H5OH(l) + CH3COOH (l) ⎯⎯, → CH3COO C2H5(l) + H2O(l), , On the other hand, when ethyl acetate and water are mixed in the presence of, dilute sulphuric acid the reverse reaction occurs., +, , H, CH3COOC2H5(l) + H2O(l) ⎯⎯, → CH3COOH(l) + C2H5OH(l), , It may be noted here that the second reaction is reverse of the first one and under, the same conditions, the two reactions occur simultaneously. Such reactions which, occur simultaneously in opposite directions are called reversible reactions., A reaction is said to be reversible if under certain conditions of temperature, and pressure, the forward and reverse reactions occur simultaneously., Reversible reactions are indicated by placing two half arrows pointing in opposite, directions ( U ) between the reactants and products. Thus the above reaction is, more appropriately written as, CH3COOH (l) + C2H5OH (l) U CH3COOC2H5 (l) + H2O(l), When ethyl acetate and water are formed in the forward reaction the reverse, reaction also starts in which ethanol and acetic acid are formed. After some time, the concentrations of all the reactants and products become constant. This happens, when the rates of forward and reverse reactions become equal; and all the, properties of the system become constant. It is said that the system has attained, 292, , CHEMISTRY
Page 322 :
MODULE - 5, , Chemical Equilibrium, , state of equilibration. However it may be noted that the state of equilibrium is reached, only if the reaction is carried out in a closed system. At the time of equilibrium, forward, and reverse reactions are taking place and it is in a state of dynamic equilibrium because, no change is taking place., A reversible reaction is said to be in the equilibrium state when the forward, and backward reaction occur simultaneously at the same rate in a closed, system and the concentrations of reactants and products do not change with, time, ZZX C + D, A common example of reversible reactions of the type A + B YZZ, , Chemical Dynamics, , Notes, , ZZX CH COOH + H O, CH3COOH + C2H5OH YZZ, 3, 2, The following graphs Fig. 11.1 shows the equilibrium state in a reversible reaction., , Concentration, , C or D, , A or B, , Time, , Equilibrium, , Fig. 11.1 : Equilibrium in reversible reaction, , The graph depicts that the rate of forward reaction gradually decreases while the rate, of backward reaction increase till they become constant and equal to each other., 11.2.2 Irreversible Reactions, Most of the reactions occur only in one direction. They are called irreversible, reactions. For example when carbon is burnt in air to form carbon dioxide the, reaction goes only in one direction i.e. in the direction of formation of carbon, dioxide, C (s) + O2 (g) ⎯⎯, → CO2 (g), Strictly speaking all reactions are considered to be reversible. But the rate of, reaction in one particular direction is extremely small compared to the other., Thus the reaction proceeds practically in one direction to near completion, leaving, a negligibly small amount of reactant at the end., , CHEMISTRY, , 293
Page 323 :
MODULE - 5, Chemical Dynamics, , Chemical Equilibrium, , When hydrochloric acid is mixed with sodium hydroxide, a base, in equimolar quantities,, a neutralisation reaction takes place; with the formation of salt and water., HCl (aq) + NaOH (aq) ⎯⎯, → NaCl (aq) + H2O (l), , Notes, , This reaction proceeds to completion in the forward direction. Similarly when a, solution of silver nitrate is added to a solution of sodium chloride silver chloride, is precipitated immediately., NaCl (aq) + AgNO3 (aq) ⎯⎯, → AgCl (s) + NaNO3 (aq), , 11.3 CHARACTERISTICS OF EQUILIBRIUM STATE, 1. The state of chemical equilibrium is reached in a reversible reaction when;, (i) the temperature of the system attains a constant value., (ii) the pressure of the system attains a constant value., (iii) the concentrations of all the reactants and products attain constant, values., The state of equilibrium has following characteristics properties :, (i) Chemical Equilibrium is dynamic in nature, The chemical equalibrium is the result of two equal but opposite processes, occurring in the forward and reverse directions and there is no “net” change, occurring in the system., (ii) Equilibrium can be attained from either side, The same state of equilibrium (characterized by its equilibrium constant which, is discussed later can be reached whether the reaction is started from the reactants, or products side. For example, the same equilibrium, ZZX 2NO (g), N2O4 (g) YZZ, 2, is established whether we start the reaction with N2O4 or NO2., (iii) Equilibrium can be attained only in a closed system, Equilibrium can be attained only if no substance among, reactants or products,, is allowed to escape i.e. the system is a closed one. Any system consisting of, gaseous phase or volatile liquids must be kept in a closed container, e.g., ZZX 2NH (g), N2(g) + 3H2(g) YZZ, 3, A system consisting of only non-volatile liquid and solid phases can be kept, even in an open container because such substances have no tendency to escape,, e.g., ZZX Fe (SCN)3 (s) + 3 NH4Cl(aq), FeCl3(aq) + 3 NH4SCN(aq) YZZ, , 294, , CHEMISTRY
Page 324 :
MODULE - 5, , Chemical Equilibrium, , (iv) A catalyst can not change the equilibrium state, , Chemical Dynamics, , Addition of a catalyst speeds up the forward and reverse reactions by same extent, and help in attaining the equilibrium faster. However, the equilibrium, concentrations of reactants and products are not affected in any manner., , 11.4 EQUILIBRIUM IN PHYSICAL PROCESSES; PHASE, EQUILIBRIUM, , Notes, , State of equilibrium can also be reached in physical processes., 11.4.1 Liquid – Vapour Equilibrium, Let us take some quantity of a liquid in an empty container and close it. Initially, the vapour pressure above the liquid will be zero. The liquid will evaporate and, its vapour will fill the empty space above it., Liquid → Vapour, The rate of evaporation is maximum in beginning. As vapours build up, its, pressure increases and the rate of evaporation slows down. Also the reverse, process of condensation begins (Fig. 11.2)., Vapour → Liquid, Vapour, , Liquid, , Fig. 11.2 : Liquid Vapour equilibrium, , and its rate gradually increases with the increase in the vapour pressure. After some, time the two rates (of evaporation and condensation) become equal and the following, equilibrium is established., Liquid U Vapour, At equilibrium the vapour pressure reaches its maximum value and is known as, the saturated vapour pressure or simply the vapour pressure. At a fixed, temperature, each liquid has its own characteristic vapour pressure. The vapour, pressure of a liquid increases with rise in temprature., 11.4.2 Solid – Vapour Equilibrium, Volatile solids sublime to form vapour. The situation is just similar to the liquid, vapour system. When kept in a closed container at a constant temperature the, following equilibrium is established., Solid U Vapour, CHEMISTRY, , 295
Page 325 :
MODULE - 5, , Chemical Equilibrium, , Chemical Dynamics, Iodine, Vapour, , Solid, Iodine, , Notes, Fig. 11.3 : Solid vapour equilibrium, , Such an equilibrium can be established by keeping some solid iodine in a gas jar, covered with a lid. (Fig. 11.3). Gradually the purple coloured iodine vapours fill, the jar and the following equilibrium is established., I2(s) U I2(g), 11.4.3 Solid – Liquid Equilibrium, Below its freezing point a liquid freezes spontaneously, Liquid → Solid, When heated above its melting point the solid melts spontaneously :, Solid → Liquid, At the melting point, the two phases are in equilibrium, Solid, , Liquid, , because the above two processes occur simultaneously and at the same rate., Such an equilibrium is characterized by its temperature i.e. the melting point of, the solid., 11.4.4 Solute – Solution Equilibria, , Saturated, Solution, Undissdued, Sugar, , Fig. 11.4 : Solute - Solution Equilibrium, , 296, , CHEMISTRY
Page 326 :
Chemical Equilibrium, , When sugar crystals are put in a saturated solution of sugar in water; it will appear that, no change is taking place and sugar appears to remain undissolved. Actually, the, undissolved sugar does dissolve in the saturated sugar solution; and an equal amount, of sugar seperates out from the solution. The solid sugar and the sugar solution form an, equilibrium system which is dynamic in nature., , MODULE - 5, Chemical Dynamics, , sugar (s) U sugar solution (saturated), The equilibrium is established when the rate of dissolution of sugar becomes, equal to the rate of crystallisation. In general such equilibrium can be represented, as, , Notes, , solute (s) U solution (saturated), This equilibrium is known as Solubility Equilibrium., 11.4.5, , Phase and Phase Equilibrium, , You must have noticed in each of the above equilibria the system consists of two, distinct parts; solid, liquid, solution or vapour. Each of these parts is called a, phase., A phase is defined as a homogenous part of a system which has uniform, composition and properties throughout., A phase is not the same as physical state. A mixture of two solids, even when, powdered finely is a two phase system. This is because particles of the two, solids have different chemical compositions and physical properties. Completely, miscible liquids, solutions and all gaseous mixture constitute only one phase, each., All the cases of physical equilibrium are in fact the systems in which different, phases are in equilibrium; only if they contain, at least one common component., A dynamic exchange of the common component between two phases takes place., When the rates of exchange becomes equal the equilibrium is established. In, solid solute and solution equilbrium the example given earlier, sugar is the, common component., , 11.5 EQUILIBRIUM IN HOMOGENEOUS AND, HETEROGENEOUS SYSTEMS, 11.5.1 Homogeneous and Heterogeneous Systems, Homogeneous system is one which has one phase. It has the same chemical, composition and uniform properties throughout. It is formed by particles of, molecular size only. Pure solids, liquids, gases and solutions are the examples of, homogeneous systems., CHEMISTRY, , 297
Page 327 :
MODULE - 5, Chemical Dynamics, , Chemical Equilibrium, , A system consisting of only one phase is called a homogeneous system, Heterogeneous system, on the other hand has at least two phases – a mixture of, solids or immiscible liquids constitutes a heterogeneous system., Any system consisting of two or more phases is called heterogeneous system, , Notes, , 11.5.2 Homogeneous and Heterogeneous Equilibrium Systems, Equilibrium can be established in either type of systems. Since all physical, equilibria involve at least two phases, therefore these are all examples of, heterogeneous equilibrium. But chemical equilibrium can be homogeneous or, heterogeneous in nature. It is homogeneous if both the reactants and products, are present only in one phase gas or liquid and heterogeneous if present in more, than one phase. In the following sections we shall study such systems., 11.5.3 Homogeneous Chemical Equilibrium System, (a) Gas – Phase homogeneous systems, Such systems contain only gaseous reactants and products. Since all gaseous, mixtures are homogeneous in nature they constitute only one phase. Following, are examples of this type of equilibrium:, (i) N2 (g) + 3H2 (g) U 2NH3 (g), (ii) 2N2O5 (g) U 4NO2 (g) + O2 (g), (b) Liquid – Phase homogeneous systems, These are the systems in which both the reactants and products are present in, only one liquid phase (as a solution) for example :, +, , H, ZZZ, X CH COOC H (l) + H O (l), (i) CH3 COOH (l) + C2H5OH (l) YZZZ, 3, 2 5, 2, , ZZX HCN (aq) + KOH (aq), (ii) KCN (aq) + H2O (l) YZZ, , 11.5.4 Heterogeneous Chemical Equilibrium Systems, The systems in which reactants and products are present in more than one phase, belong to this type. For example :, ZZX Fe3O4 (s) + 4H2 (g), (i) Fe (s) + 4H2O (g) YZZ, , ZZX CaO (s) + CO2 (g), (ii) CaCO3 (s) YZZ, , 298, , CHEMISTRY
Page 328 :
MODULE - 5, , Chemical Equilibrium, , Chemical Dynamics, , INTEXT QUESTIONS 11.1, 1. What is a reversible reaction? Give two examples., 2. When does a reaction reach equilibrium state?, 3. How would you know whether a system has reached the equilibrium state, or not?, , Notes, , 4. Give two examples of physical equilibrium., 5. Give two example each of chemical homogeneous and heterogeneous, equilibria., , 11.6 LAW OF MASS ACTION, Equilibrium state of a reversible reaction is characterized by its equilibrium, constant, which is related to the equilibrium concentrations of all the reactants, and products. The method of writing the expression for equilibrium constant, was given for the first time by Guldberg and Wage in 1863 in the form of the, law of mass action which states:, The rate at which a substance reacts is proportional to its active mass (molar, concentration) and the overall rate of the reaction is proportional to the product, of active masses (molar concentrations) of all the reacting substances., For a reaction, A + B ⎯→ Products, Rate of reaction, , ∝ [A][B], , and, , = k [A][B], , where [A] = molar concentration (active mass) of A, [B] = molar concentration of B., k = constant of proportionality and is called the specific rate constant, of the reaction., Consider a reaction, 2A ⎯→ Products, It may be written as, A + A ⎯→ Products., According to the law of mass action state, rate ∝ [A] [A], ∝ [A]2, = k[A]2, CHEMISTRY, , 299
Page 329 :
MODULE - 5, Chemical Dynamics, , Chemical Equilibrium, , For the reaction, aA ⎯→ Products, rate ∝ [A]a, = k[A]a, For a more general reaction, , Notes, , aA + bB ⎯→ Products, Rate = k[A]a [B]b, On applying the law of mass action to the reversible reaction, ZZX cC +dD, aA + bB YZZ, , the rate of the forward reaction rf is, rf = kf[A]a[B]b, and the rate of the reverse or backward reaction, rb is, rb = kb[C]c[D]d, where kf and kb are the rate constants of the forward and backward reactions, reversibly., At equilibrium, Rate of forward reaction = rate of backward reaction, rf = rb, or, , kf[A]a[B]b = kb[C]c[D]d, , Rearranging these, , Keqm =, , k f [C]c[D]d, =, k b [A]a [B]b, , Here Keqm = equilibrium constant of the reaction. It is also called the, concentration equilibrium constant and the symbol given is Kc., It may be noted here that the powers of concentration terms in the expressions, for rates rf and rb are each equal to the stoichiometric coefficient of the, respective reactant which is not correct in all the cases as you will learn later, in unit 16. Therefore, this derivation of equilibrium constant is not correct. We, can obtain the same relation from thermodynamics or we simply accept the, relation as the law of equilibrium as explained in the next section., 300, , CHEMISTRY
Page 330 :
MODULE - 5, , Chemical Equilibrium, , Chemical Dynamics, , 11.7 QUANTITATIVE ASPECT OF EQUILIBRIUM STATE, 11.7.1 Law of Equilibrium and Concentration Equilibrium Constant, Consider the following equilibrium, ZZX 2HI (g), H2(g) + I2(g) YZZ, , At equilibrium the concentrations of H2, I2 and HI become constant. Also, it has, been found experimentally that irrespective of the starting concentrations of H2, and I2 the following ratio of concentration terms always remains constant., Kc =, , Notes, , [HI]2, [H 2 ][I 2 ], , Here [H2], [I2] and [HI] represent the equilibrium molar concentrations of H2, I2, and HI respectively and Ke is called the concentration equilibrium constant (some, times it is written simply as K). In general, for reversible reaction, ZZX cC + dD, aA + bB YZZ, at equilibrium, the following ratio of concentration terms always remains constant, at a given temperature., Kc =, , [C]c [D]d, [A]a [B]b, , The above relation is known as the law of equilibrium. It may be noted here, that all the concentrations values in the law of equilibrium are the equilibrium, concentrations of reactants and products. The numerator of the law of equilibrium, is the product of equilibrium molar concentrations of products, each term being, raised to the power equal to its stoichiometric coefficient in the chemical equation, and the denominator contains products of similar concentration terms of reactants., 11.7.2 Pressure Equilibrium Constant Kp, In case of gases their partial pressures can also be used in place of molar, concentrations (since the two are directly proportional to each other) in the law, of equilibrium. The new equilibrium constant, Kp, is called the pressure, equilibrium constant. For the reaction between H2 and I2, Kp is given by, Kp =, , p 2 HI, p H 2 × p I2, , Here p H , p I and pHI are the equilibrium partial pressures of H2, I2 and HI, 2, , 2, , respectively. For the general gas phase reaction :, a A (g) + b B (g) U c C (g) + d D (g), , CHEMISTRY, , 301
Page 331 :
MODULE - 5, Chemical Dynamics, , Chemical Equilibrium, , it is given by :, , Kp =, , pcC × pdD, paA × pbB, , 11.7.3 Relation between Kp and Kc, For a general gas phase reaction at equilibrium, Notes, , aA (g) + b B (g) U c C (g) + d D (g), The pressure and concentration equilibrium constants Kp and Kc are, , Kp =, , pcC × pdD, , and KC =, , b, paA × pB, , [C]c [ D]d, [ A]a [ B]b, , For a gaseous substance i, the ideal gas equation is, piV = niRT, where pi and ni are its partial pressure and amount in a gaseous mixture and V, and T are its volume and temperature and R is the gas constant. The relation may, be written as, pi =, , ni, RT = ci RT, V, , Where ci is the molar concentration or molarity of ‘i’ expressed in moles per, litre. This relation can be used for replacing the partial pressure terms in the, expression for Kp., (cC RT ) c (cD RT ) d, Kp = (c RT ) a (c RT )b, A, B, cCc cDd, = c a c b (RT) (c + d) – (a + b), A B, , Using the square bracket notation for molar concentration the relation can be, written as, Kp =, , [C ]c [D]d, (RT ), [A]a [B]b, , Δn, = K c ( RT ), , (nP – nR ), , g, , where Δng is the change in the moles of gaseous substances in the reaction and is, equal to the difference in the moles of gaseous products nP and the moles of, gaseous reactants, nR. Δng may be zero positive or negative., , 302, , CHEMISTRY
Page 333 :
MODULE - 5, Chemical Dynamics, , Chemical Equilibrium, , 11.8.1 Heterogeneous Equilibrium, Consider the following equilibrium, CaCO3 (s) U CaO (s) + CO2 (g), According to the law of equilibrium, , Notes, , Kc =, , [CaO][CO2 ], [CaCO3 ], , Here CaCO3 and CaO are pure solids. The concentration of any solid is constant, at a fixed temperature therefore these are not written in expression for equilibrium, constant for hetrogenous reactions. Equilibrium constants for the reaction can, be written as, Kc = [CO2] and Kp = Pco2, Following are some more examples of heterogenous equilibrium, (i) Reaction between iron and steam, 3 Fe (s) + 4H2O (g) U Fe3O4 (s) + 4H2 (g), p 4H2, [H 2 ]4, ; Kp = 4, Kc =, p H2O, [H 2 O]4, , (ii) Liquid – Vapour Equilibrium, H2O(I) U H2O (g), Kc = [H2O; g] ; Kp = p H2O ., , 11.9 CHARACTERISTICS OF EQUILIBRIUM CONSTANT, 11.9.1 Equilibrium Constant and Chemical Equation, The expression of equilibrium constant depends upon the manner in which the, chemical equation representing it is written. For the reaction, H2 (g) + I2 (g) U 2HI (g), The equilibrium constant K is given by K =, , [HI]2, [H 2 ][I 2 ], , When the same reaction is written as, (a), , 304, , 1, 1, H2 (g) + Ι2 (g) U HI (g), 2, 2, , CHEMISTRY
Page 334 :
MODULE - 5, , Chemical Equilibrium, , Chemical Dynamics, , the corresponding equilibrium constant K1 is given by, [HI], , K1 =, , 1, , 1, , [H 2 ] 2 [I 2 ] 2, , It may be noted that equilibrium constants K and K1 are related as K1 =, , K, , (b) When the reaction is written as reverse, , Notes, , 2HI (g) U H2 (g) + I2 (g), K2 =, , [H 2 ] [I 2 ], [HI]2, , Here it can be seen that, K2 =, , 1, K, , Similar relationship is also observed in the pressure equilibrium constant Kp., Thus the expression of equilibrium constant depends on how the reaction is, expressed in the form of a chemical equation., 11.9.2 Units of Equilibrium Constant, Units of equilibrium constant Kc or Kp depend upon the fact whether during the, reactions there is any change in the moles of substance or not., (a) The reactions in which there is no change in moles of substance i.e., Δn = 0., The equilibrium constant for such reaction has no units. For example in the, reaction between H2 and I2, H2 (g) + I2 (g) U 2HI (g), [HI]2, Kc = [H ][I ], 2, 2, , p 2HI, Kp = p . p, H2, I2, , (mol L−1 ) 2, Kc = (mol L−1 )(mol L−1 ), , bar 2, Kp = (bar)(bar), , ∴ Hence Kp and Kc have no units in such cases., (b) The reaction where there is change in the moles of substance i.e. Δn ≠ 0., , CHEMISTRY, , 305
Page 335 :
MODULE - 5, Chemical Dynamics, , Chemical Equilibrium, , The equilibrium constant for such reactions has units which depend upon the change in, moles of substances., For example :, , Notes, , N2 (g) + 3H2 (g) U 2NH3 (g), Δn = ΔnP − ΔnR, =2–4=–2, The units of Kc for this reaction would be (mol L–1)–2 or L2 mol–2 and those of Kp, would be bar–2 as shown below :, The equilibrium constant for such reactions are, [NH 3 ]2, Kc =, [N 2 ][H 2 ]3, , Kp =, , p 2NH3, p N2 × p3H2, , (mol L−1 ) 2, Kc =, (mol L−1 )(mol L−1 )3, , = (mol L–1)–2, = L2 mol–2, Kp =, , pressure 2, pressure. pressure3, , = pressure–2, = bar – 2, For the reaction PCl5 (g) U PCl3 (g) + Cl2 (g), Δn = 2 – 1 = 1. Therefore,, The units for Kc and Kp are, Kc = mol L–1 and Kp = bar, 11.9.3 Significance of the Magnitude of K, The equilibrium constant of a reaction has a constant and characteristic value at, a given temperature. The changes in starting concentration, pressure and the, presence of a catalyst do not change the value of the equilibrium constant., However if the temperature is changed. The value of the equilibrium constant, also changes., The magnitude of the equilibrium constant is a measure of the extent upto which, a reaction proceeds before the equilibrium is reached. The magnitude of K is, large when the products are present in larger amounts than the reactants in the, equilibrium mixture. For the reaction, 306, , CHEMISTRY
Page 336 :
MODULE - 5, , Chemical Equilibrium, , H2 (g) + I2 (g) U 2 HI (g), , Kc = 90 at 298 K, , Chemical Dynamics, , and for 2CO (g) + O2 (g) U 2 CO2 (g) Kc = 2.2 × 1022 at 1000 K., A large value of Kc for the second reaction indicates that amount of products is, much more than the reactants present at the time of equilibrium. Thus the, magnitude of equilibrium constant tells us about the position of the equilibrium., 11.9.4 Calculation of Equilibrium Constants, , Notes, , Equilibrium constants K c and K p can be calculated if the equilibrium, concentrations or partial pressures are known or can be obtained from the given, data. The following examples illustrate the calculations., Example 11.1 : Calculate the equilibrium constant for the reaction, A (g) + B (g) U C (g) + D (g), If at equilibrium 1 mol of A, 0.5 mole of B, 3.0 mole of C and 10 mol of D are, present in a one litre vessel., Solution : From the law of equilibrium, [C][D], , Kc = [A][B], Since the volume of the vessel is one litre, the number of moles of A, B, C and D, are equal to their concentrations. Thus, [A] = 1 mol L–1, [B] = 0.5 mol L–1, [C] = 3.0 mol L–1 and [D] = 10 mol L–1 and, Kc =, =, , (3.0 mol L−1 ) (10 mol L−1 ), (1 mol L−1 ) (0.5 mol L−1 ), , 3.0 × 10, = 60, 1 × 0.5, , Example 11.2 In an experiment carried out at 298 K, 4.0 mol of NOCl were, placed in a 2 litre flask and after the equilibrium was reached 1.32 mol of NO, were formed. Calculate Kc at 298 K for the reaction, 2NOCl (g) U 2 NO (g) + Cl2 (g), Solution Calculation of equilibrium concentrations, (i) [NO] =, , No. of moles of NO, 1.32 mol, =, = 0.66 mol L–1, Volume, 2L, , 1, (No. of moles of NO), No. of moles of Cl2, 2, (ii) [Cl2] =, =, Volume, Volume, , CHEMISTRY, , 307
Page 337 :
MODULE - 5, , Chemical Equilibrium, , Chemical Dynamics, , =, , 1.32 mol, = 0.33 mol L–1, 2 × 2L, , No. of moles of NOCl, (Initial moles - moles decomposed), =, Volume, Volume, , (iii) [NOCl] =, =, , Notes, , (4.0 – 1.32) mol, 2.68 mol, =, = 1.34 mol L–1, 2L, 2L, , For the reaction, 2NOCl (g) U 2NO (g) + Cl2 (g), Kc =, , (0.66) 2 × 0.33, [NO]2 [Cl2 ], (0.66 mol L−1 ) 2 (0.33 mol L−1 ), =, =, (1.34) 2, (1.34 mol L−1 ) 2, [NOCl]2, , = 0.080 mol L–1, Kc = 0.080 mol L–1, Example 11.3 : 2 moles of HI were heated in a vessel of one litre capacity at 713, K till the equilibrium was reached. At equilibrium HI was found to be 25%, dissociated. Calculated Kc and Kp for the reaction., Solution Initial moles of HI = 2, Moles of HI dissociated =, , 25 × 2, = 0.5 mol, 100, , Moles of HI at equilibrium = 2.0 – 0.5 = 1.5 mol, The dissociation of HI occurs as, 2HI (g), , U, , H2 (g), , +, , I2 (g), , Initial moles, , 2, , 0, , 0, , Equilibrium moles, , (2 – 0.5), , 0.25, , 0.25, , 1.5 mol, , 0.25 mol, , 0.25 mol, , Volume of reaction vessel, , 1L, , 1L, , 1L, , Equilibrium concentration, , 1.5 mol L–1, , 0.25 mol L–1, , 0.25 mol L–1, , For the reaction, (0.25 mol L−1 ) (0.25 mol L−1 ), [H 2 ] [I 2 ], Kc = [HI]2 =, (1.5 mol L−1 ) 2, (0.25) 2, =, = 0.028, (1.5) 2, , 308, , CHEMISTRY
Page 339 :
MODULE - 5, , Chemical Equilibrium, , Chemical Dynamics, , [CH3COOC2 H 5 ][H 2 O], , Kc = [CH COOH][C H OH], 3, 2 5, ⎛ 2n ⎞⎛ 2n ⎞, ⎜, ⎟⎜, ⎟, ⎝ 3V ⎠⎝ 3V ⎠, = n, =2×2=4, ⎛, ⎞⎛ n ⎞, ⎜, ⎟⎜, ⎟, ⎝ 3V ⎠⎝ 3V ⎠, , Notes, , Kc = 4, , INTEXT QUESTIONS 11.2, 1., , For a reversible reaction, 2A + B U 3C + 3D, Write the expression for the equilibrium constant, , 2., , What is the relation between Kp and Kc., , 3., , (i) Apply the law of equilibrium to the following and write the expression, for Kp and Kc., (a) CO2 (g) + H2 (g) U CO (g) + H2O (g), (b) I2 (s) U I2 (g), (ii) For the above reaction write equation for Kp and Kc., , 4., , The equilibrium constant for the reactions, (i) N2 (g) + 3H2 (g) U 2NH3 (g), (ii), , 1, 2, N2(g) + H2(g) U NH3, 3, 3, , are K1 and K2 respectively. What is the relation between them., 5., , What is the significance of the magnitude of equilibrium constant?, , 11.10 FACTORS AFFECTING EQUILIBRIUM STATE, Supposing a reaction has reached the equilibrium state and then some conditions, like concentrations, temperature, pressure etc. are changed, would it be affecting, the equilibrium state. If yes how?, In this section, we shall discuss these questions., The state of equilibrium is in a dynamic balance between forward and backward, reaction. This balance can be disturbed by changing concentration, temperature, or pressure. If done so a certain net change occurs in the system. The direction of, change can be predicted with the help of Le-Chatelier's principle., 310, , CHEMISTRY
Page 340 :
MODULE - 5, , Chemical Equilibrium, , 11.10.1 Le Chatelier's Principles, , Chemical Dynamics, , It states that when a system in equilibrium is disturbed by a change in, concentration, pressure or temperature, a 'net' change occurs in it in a, direction that tends to decrease the disturbing factor., The prinicple can be applied to various situations., 11.10.2 Change in Concentration, , Notes, , Consider the state of equilibrium for the formation of ammonia from nitrogen, and hydrogen., ZZX 2NH3(g), ΔH = –92.4 kJ/mol, N2(g) + 3H2(g) YZZ, , The concentration of nitrogen, hydrogen and ammonia become constant at the, point of equilibrium. Now if any amount of reactants or ammonia is added or, removed their concentration will change and the equilibrium will get disturbed., (i) Increase concentration of reactant : When the concentration of either, nitrogen or hydrogen is increased; a net forward reaction will take place which, consumes the added reactant., (ii) Increase in the concentration of any product : If the concentration of, product ammonia is increased, a net backward reaction would take place to utilise, the added ammonia., 11.10.3 Change in Pressure, Change in pressure affects equilibrium involving gaseous phase either in a, homogeneous or hetrogeneons system., Le Chatelier's prinicple for systems involving gases can be studied as follows :, (i) When the number of moles of products is more than the total number of, moles of reactants as in the following system, ZZX 2NO2(g), N2O4(g) YZZ, , Increase in total pressure keeping the temperature constant, will cause a, decrease in volume. This means that the number of moles per unit volume, will increase. A net change will take place in the equilibrium in the direction, where the number of moles decrease i.e. backward direction., (ii) When the number of moles of products is less than reactants. As in the, following case, ZZX 2NH3(g), N2(g) + 3H2(g) YZZ, CHEMISTRY, , 311
Page 341 :
MODULE - 5, Chemical Dynamics, , Notes, , Chemical Equilibrium, , According to Le Chatelier's principle increase in total pressure will bring a net, change to the equilibrium in the direction where the total number of moles is, decreasing i.e. to the product side as Δng = 2. Decrease in total pressure will, bring the net change to equilibrium in the direction where the total number of, moles is increasing i.e. backward direction., (iii) When there is no change in the total number of moles of reactant and product, as in the following state of equilibrium., H2(g) + I2(g) YZZ, ZZX 2HI, There is no net change in equilibrium state when pressure is changed., 11.10.4 Change of Temperature, According to Le Chatelier's prinicple when the temperature is changed (increased, or decreased) the equilibrium system reacts to nullify the change in heat content., However, the net change in equilibrium is directed by the exothermic or, endothermic nature of reaction., (i) Exothermic equilibrium : For the following system of equilibrium of, exothermic nature :, N2(g) + 3H2(g) YZZ, ZZX 2NH3(g);, , ΔH = – 92.4 kJ/mol, , according to Le Chatelier's prinicple increase in temperature brings a net change, in the equilibrium state in that direction where this extra heat is consumed. The, net change is in the backward direction and some ammonia will decompose, producing nitrogen and hydrogen. Similarly if the temperature is decreased the, equilibrium shifts to the forward direction., (ii) Endohermic equilibrium, N2(g) + O2(g) YZZ, ΔH = + 180.7 kJ/mol–1, ZZX 2NO(g);, If the temperature is increased the added heat will be absorbed by the reactant, and the net change takes place to the equilibrium in the forward direction. If the, temperature in decreased it will bring a 'net' change to equilibrium in the backward, direction i.e. direction in which it is exothermic., Addition of a Catalyst : It does not affect the equilibrium. However it helps to, achieve the equilibrium faster., 11.10.5 Applications of Le Chatelier’s Principle, It can be applied to physical as well as chemical equilibria, , 312, , CHEMISTRY
Page 342 :
MODULE - 5, , Chemical Equilibrium, , Chemical Dynamics, , (A) Physical Equilibria, (1) Melting of Ice, Ice U Water ;, , ΔH = + 6 kJ/mol–1, , The change of ice to water is endothermic process. According to Le Chatelier's, principle if the temperature is increased the net change will take place in the, forward direction some ice will melt into water., , Notes, , When the pressure is increased on the equilibrium system, then the volume should, decrease; according to Le Chatelier's principle the net change in equilibrium, takes place in the forward direction and ice melts. Therefore, ice melts on, increasing the pressure., (2) Vaporization of Water, Water(l) U Water vapour; ΔH = + ve, This process occurs with a large increase in volume since Δng = 1 – 0 = + 1, and, it occurs with absorption of heat., Increasing the temperature results in more vapour formation (endothermic, process). Since Δng = + 1, increase in pressure results in a net change in equilibrium, in the backward direction as the volume of water vapours is more than that of, liquid water for a given mass of water., (3) Solubility Equilibrium, The equilibrium is, Solute (s) U Solute (solution), The process of dissolution can be endothermic or exothermic. In case of solutes, like KCl, KNO3 and NH4Cl, ΔH is positive (endothermic) and more solute will, dissolve on heating. Thus, the solubility increases with rise in temperature. In, case of solutes like KOH and NaOH the ΔH is negative (exothermic) and their, solubility decreases on heating., (B) Chemical Equilibra, (1) Favourable Conditions for Synthesis of Ammonia : This reaction is of, great industrial importance. During the synthesis of ammonia such conditions, are maintained which favour the ‘net’ forward reaction namely low temperature, and high pressure. Addition of catalyst makes the reaction occur fast. Besides,, nitrogen and hydrogen gases are continuously fed into the reaction chamber and, ammonia is continuously removed. All this keeps the system under stress and, equilibrium is never permitted to be attained, so that the synthesis of ammonia, continues to occur., , CHEMISTRY, , 313
Page 343 :
MODULE - 5, Chemical Dynamics, , Chemical Equilibrium, , In industry the reaction is carried out at 450 0C and 200 atm pressure in the presence, of finely divided iron (catalyst) and molybdenum (promotor), (2) Formation of SO3, The reaction, 2SO2 (g) + O2 (g) U 2SO3 (g) ; ΔH = – ve, , Notes, , is extothermic and Δng = 2 – 3 = – 1. Formation of SO3 will be favoured by high, pressure and low temperature in the presence of a catalyst., (3) Formation of NO, The reaction, N2 (g) + O2 (g) U 2NO (g) ;, , ΔH = + ve, , is endothermic and Δng = 2 – 2 = 0. The reaction is not affected by pressure, changes and is favoured at high temperature. Presence of a suitable catalyst would, be helpful., , INTEXT QUESTIONS 11.3, 1. What is Le Chatelier’s principle?, 2. What are the factors that can affect a system at equilibrium?, 3. What will happen to solid-vapour equilibrium when the temperature and, pressure are decreased., 4. (a) Which of the following will result in ‘net’ forward reaction in case, of, A (g) + 2B (g) U C (s) + D (g) ; ΔH = + ve, (i) addition of C, (ii) addition of A, (iii) decrease in pressure, (iv) increase in temperature, (b) What are the most favourable conditions for the formation of C and, D?, , WHAT YOU HAVE LEARNT, z, , 314, , A chemical reaction is said to be reversible under certain conditions, if, along with the reactants forming the products, the products also react and, form back the reactants simultaneously., CHEMISTRY
Page 344 :
MODULE - 5, , Chemical Equilibrium, z, , Reversible reactions do not reach completion stage and result in a state of, equilibrium which is reached when two opposite processes occur at the, same rate., , z, , The macroscopic properties of the system do not change once the equilibrium, has been established., , z, , Irreversible reactions are in fact the reversible reactions in which the, equilibrium is reached only when a negligible amount of the reactants is, left unreacted., , z, , Chemical equilibrium is dynamic in nature. It can be attained by starting, the reaction from any side and only in a closed system., , z, , When equilibrium is reached as a result of two opposite physical changes,, it is called physical equilibrium and when as a result of two opposite chemical, changes it is called chemical equilibrium., , z, , A phase is a homogeneous system or a part of a system which has same, composition and uniform properties throughout. It is not same as physical, state., , z, , A system with only one phase is called a homogeneous system and the one, with more than one phases is called heterogeneous system., , z, , Chemical equilibrium can be homogeneous or heterogeneous while physical, equilibrium is always heterogeneous., , z, , For a general reaction aA + bB U cC + dD according to the law of, equilibrium, the equilibrium constant K is given by the expression, , Chemical Dynamics, , Notes, , [C]c [D]d, , K = [A]a [B]b, z, , Concentration equilibrium constant Kc is obtained when molar concentration, are used for calculating K. Concentrations of pure solids and liquids are, constant and are not included in the expression of Kc., , z, , In case of gaseous systems, the concentration of gases are expressed in, terms of their partial pressures. The equilibrium constant thus obtained is, called the pressure equilibrium constant, Kp., , z, , The relation between Kp and Kc is = Kc (RT ) where Δng is the change in, the number of moles of gaseous substances during the reaction., , z, , Expression of equilibrium constant depends upon how the chemical equation, is written for the reaction., , Δng, , CHEMISTRY, , 315
Page 345 :
MODULE - 5, Chemical Dynamics, , Chemical Equilibrium, z, , Magnitude of the equilibrium constant is a measure of how close the reaction is, to the completion stage., , z, , Units of K depends upon the change in the number of moles of the substances, during the reaction., , z, , Concentration, pressure and temperature can affect the equilibrium systems, and the affect can be qualitatively predicted by Le Chatelier’s principle, which states that when a system at equilibrium is disturbed by changing, concentration, pressure or temperature, a ‘net’ change occurs in the direction, that tends to neutralize the effect of the disturbing factor., , z, , Changes in concentration and pressure do result in some chemical reaction,, but the value of the equilibrium constant is not changed., , z, , A catalyst does not change the equilibrium constant. It only helps in reaching, the equilibrium state quicker., , z, , A change in temperature change the value of the equilibrium constant., , Notes, , TERMINAL EXERCISE, 1. What do you understand by reversible and irreversible reactions? Give one, example of each., 2. What is physical equilibrium? Give one example?, 3. Give characteristics of equilibrium state., 4. Is the phase same as physical state? Illustrate your answer with one example, of each., 5. How do homogeneous and heterogeneous systems differ from each other?, Which of the following are homogeneous systems?, (a) Liquid U Vapour, (b) N2O4 (g) U 2NO2 (g), (c) NH4Cl (s) U NH3 (g) + HCl (g), (d) CH3COOH (l) + C2H5OH (l) U CH3COOC2H5 (l) + H2O (l), 6. What are Kp and Kc? Derive a relation between them., 7. Write down the expression of Kc for the following. Also give units in each, case., (a) N2O5 (g) U 2NO2 (g) +, , 316, , 1, O (g), 2 2, , CHEMISTRY
Page 346 :
Chemical Equilibrium, , (b) CH4 (g) + H2O (l) U CO (g) + 3H2 (g), , MODULE - 5, Chemical Dynamics, , (c) FeCl3 (aq) + 3NH4SCN (aq) U Fe (SCN)3(aq) + 3NH4Cl (aq), 8. Write down the expression of Kp for the following and give its units (in, terms of atmosphere) in each case, (a) CO2 (g) + H2 (g) U CO (g) + H2O (l), (b) 3Fe(s) + 4H2O(l) U Fe3O4 (s) + 4H2 (g), , Notes, , (c) 2SO3 (g) U 2SO2 (g) + O2 (g), 9. Give the relation between Kc and Kp for the reaction., CaCO3 (s) U CaO (s) + CO2 (g), 10. Using the relaction between Kp and Kc write the expression of, (i) Kp for the reactions given in Q. No.7, (ii) Kc for the reactions given in Q. No.8, 11. List the factors that can affect, (i) a system at equilibrium and, (ii) equilibrium constant of a system, 12. State the Le Chatelier’s Principle., 13. What will be the effect of the following factors on the following systems at, equilibrium?, (i), (ii), (iii), (iv), (v), , 2 X (g) U 2Y (s) + Z (g); ΔH = + ve, Addition of X,, removal of Z, addition of a catalyst, increasing the pressure and, increasing the temperature., , 14. 5 moles of HI were produced by the reaction between 7.5 moles of H2 and, 2.6 moles of I2 vapours at 4440C. What is the equilibrium constant of the, reaction, H2 (g) + I2 (g) U 2HI (g), 15. The equilibrium constant Kp for the reaction, N2O4 (g) U 2NO2 (g), at 333 K is found to be 1.33 atm under a total pressure of 1 atm. Calculate, Kp for the reaction, 2NO2 (g) U N2O4 (g), at 333 K and under 1 atm pressure., CHEMISTRY, , 317
Page 347 :
MODULE - 5, Chemical Dynamics, , Chemical Equilibrium, , 16. At 444 0C, 0.30 mole of H2 and 0.30 mole of I2 were taken in a one litre flask., After some time the equilibrium H2 (g) + I2 (g), 2HI (g) was established and, it was found that the concentration of I2 decreased to 0.06 mol L–1. Calculate the, value of Kc for the reaction at this temperature., 17. The equilibrium constant for the reaction., CH3COOH(l) + C2H5OH (l) U CH3COOC2H5(l) + H2O(l) is 4.0., , Notes, , What will be the composition of the equilibrium mixture if 1 mole of acetic, acid is taken with 8 moles of ethanol?, 18. Kc for the reaction, N2 (g) + 3H2 (g) U 2NH3 (g), at 4000C was found to be 0.5 L2 mol–2. Calculate Kp of this reaction in atm., , ANSWERS TO INTEXT QUESTIONS, 11.1, 1., , A chemical reaction is said to be reversible, if under certain conditions its, products also react and form back the reactants., Examples :, H2 (g) + I2 (g) U 2HI (g), 2SO2 (g) + O2 (g) U 2SO3 (g), , 2., , A reaction reaches an equilibrium state when two opposing reactions occur, at the same rate and balance each other at a particular temperature., , 3., , When a system reaches the equilibrium state, its temperature, pressure and, concentrations of all the reactants and products do not change any further, with time., , 4., , (i) Water-vapour system in a closed container at a constant temperature., (ii) A saturated solution containing some undissolved solute at a constant, temperature., , 5., , (i) Homogeneous systems :, H2 (g) + I2 (g) U 2HI (g), 2SO2 (g) + O2 (g) U 2SO3 (g), , 318, , CHEMISTRY
Page 348 :
MODULE - 5, , Chemical Equilibrium, , Chemical Dynamics, , (ii) Heterogeneous systems :, CaCO3 (s) U CaO (s) + CO2(g), Zn (s) + CuSO4 (aq) U Cu (s) + ZnSO4 (aq), 11.2, 1., , [C]3 [D]3, K = [A]2 [B], , 2., , Kp = Kc (RT), , 3., , (i), , Notes, , Δn g, , (a) Kc =, , pCO × p H2O, [CO][H 2 O], ; Kp = p × p, CO2, H2, [CO 2 ][H 2 ], , (b) Kc = [I2]; Kp = PI2, (ii) For the first reaction Δng = (1 + 1) – (1 – 1) = 0, hence Kc = Kp while for, the second reaction Δng = 1 – 0 = + 1, ∴, , 4., , Kp, RT, , or Kc < Kp., , [NH 3 ]2, [NH 3 ]2 / 3, K1 = [N ][H ]3 and K2 = [N ]1/ 3 [H ], 2, 2, 2, 2, , ∴, 5., , Kp = Kc (RT) or Kc =, , K1 = [K2]3., , It is a measure of the extent up to which a reaction proceeds before the, equilibrium is reached., , 11.3, 1., , Le Chatelier’s principle states that when a system at equilibrium is disturbed, by changing concentration, pressure or temperature, a ‘net’ change occurs, in a direction that tends to neutralize the effect of the disturbing factor., , 2., , Changes in pressure, temperature and concentrations of reactants or products., , 3., , When the temperature is decreased some vapour will condense and when, the pressure is decreased some solid will sublime., , 4., , (a) (ii) and (iv), (b) High temperature, increase in pressure, presence of a catalyst and, continuous removal of D., , CHEMISTRY, , 319
Page 349 :
MODULE - 5, , Ionic Equilibrium, , Chemical Dynamics, , 12, Notes, , IONIC EQUILIBRIUM, , In the first lesson of this module you learnt about chemical equilibrium, its types, and the factors affecting the state of equilibrium. In this the lesson you will learn, about the equilibria involving ionic species. The equilibria involving acids and bases, are critically important for a wide variety of reactions. The use of buffer solutions, for pH control is of significance in living systems, agriculture and industrial processes., Similarly, the solubility equilibrium established in the solutions of sparingly soluble, salts is also very important. For example, our bones and teeth are mostly calcium, phosphate, Ca3(PO4)2, a slightly soluble salt. We would focus our attention primarily, on the acid- base and the solubility equilibria and some interrelated aspects concerning, these equilibria such as pH, buffer solutions and common ion effect. In this process, you would apply the concepts learnt in the first lesson., , OBJECTIVES, After reading this lesson, you will be able to:, , 320, , z, , Differentiate between strong and meak electrolyte ;, , z, , Correlate the degree of dissociation and dissociation constant of a weak, electrolyte, , z, , define and explain various concepts of acids and bases;, , z, , define conjugate acid-base pairs and identify them in an acid-base equilibrium;, , z, , derive the expressions for the ionisation constants of weak acids and bases;, , z, , correlate the ionisation constants and the strength of acids and bases;, , z, , explain self ionisation of water and derive an expression for its ionic product;, , z, , define pH and correlate it with the nature of aqueous solutions- neutral,, acidic or basic;, CHEMISTRY
Page 350 :
MODULE - 5, , Ionic Equilibrium, z, , define and explain common ion effect in ionisation of weak acids and bases;, , z, , define buffer solutions;, , z, , apply Henderson equation to calculate the pH of acidic and basic buffers;, , z, , explain hydrolysis of salts with the help of chemical equations;, , z, , express solubility equilibrium with the help of an equation;, , z, , identify the relationship between solubility and solubility product for salts, of AB, AB2 A2B and A2B3 types;, , z, , illustrate and explain the common ion effect on solubility equilibrium and, , z, , recall some applications of common ion effect ., , Chemical Dynamics, , Notes, , 12.1 STRONG AND WEAK ELECTROLYTIES, Electrolytes are the compounds which when dissolved in water produce ions, in their solutions. These ions are free to move about and can carry electric charge, through the solution when an electric field is applied. Such solutions are, therefore good conductors of electricity. Thus, electrolytes are the compounds, whose solutions can conduct electricity., Many electrolytes, when dissolved in water, are almost completely converted, into ions. Such electrolytes are called strong electrolytes. All ionic compounds, like NaCl, KCl, KNO3, NH4Cl and some colvent compounds like HCl. etc are, strong electrolytes. In their case, the process of formation of ions (ionization, or dissociation) is represented with the help of a single arrow., , NaCl(aq) → Na + (aq) + Cl− (aq), HCl(g) + H 2O(l) → H3O + (aq) + Cl− (aq), On the other hand, many electrolytes ionize only partially. Such electrolytes are, called weak electrolytes. Many covalent compounds like CH3COOH, NH4OH,, C6H5NH2 are weak electrolytes. In its solution, such a compound is present, partly in the unionized molecular form and partly in the form of ions. A dynamic, equilibrium is established between the two forms when the rate of ionization, of molecules to form ions and the rate of combination of ions to form molecules, become equal. An equilibrium involving ions is called ionic equilibrium. This, equilibrium between unionized molecules and the ions is represented by, ‘reversible arrows’. For example:, CH3COOH(l) + H 2O(l) U H 3O + (aq) + CH 3COO − (aq), The extent to which a weak electrolyte ionizes is expressed with the help of, its degree of ionization, α. It is defined as the fraction or percentage of the, total amount (or concentration) of the electrolyte that is present in the form of, ions., , CHEMISTRY, , 321
Page 351 :
MODULE - 5, Chemical Dynamics, , Ionic Equilibrium, , Ionization of Weak Electrolytes, Ionization of a weak electrolyte, AB, is represented as:, AB(aq) + H 2O(l) U An + (aq) + Bn − (aq), For this equilibrium, the law of equilibrium can be written as:, , Notes, , K=, , n+, n–, [A(aq), ][B(aq), ], , (1), , [AB(aq)], , K is called ionization constant or dissociation constant of the electrolyte. It, is a characteristic property of the electrolyte. It depends upon temperature., Since AB is a weak electrolyte, its ionization is not complete. Let α be its degree, of ionization. If the initial concentration of the electrolyte is c mol dm–3, the, amount of AB ionized at equilibrium would be cα mol dm–3. Hence, the, concentrations of An+(aq) and Bn–(aq) would be cα mol dm–3 each. The, concentration of unionized AB will be c − cα or c (1 − α ). These equilibrium, concentrations are generally represented along with the equilibrium, as shown, below., n+, n−, AB(aq) + H 2O(l) U A(aq), + B(aq), (3), , Initial concentrations (mol dm–3), c, –3, Equilibrium concentrations/(mol dm ) c(1 – α ), , O, cα, , O, cα, , Putting these concentrations in the expression for equilibrium constant (1), K=, , (cα)(cα) cα 2, =, c(1− α) 1 − α, , (2), , For weak electrolytes, generally α is very small and it can be neglected in, comparison to l, i.e., (1 − α ) � 1, , With this approximation, the expression of K simplifies to, K = cα 2, and, , 322, , α=, , K, c, , (3), , CHEMISTRY
Page 352 :
MODULE - 5, , Ionic Equilibrium, , This expression for α is known as Ostwald’s Dilution Law. According to it α ,, the degree of ionization of a weak electrolyte depends upon:, , Chemical Dynamics, , (i) temperature (since K depends upon T), (ii) concentration of the electrolyte, c., At a constant temperature, α is inversely proportion to the square root of the, concentration. Qualitatively, α increases with the dilution of the solution., , Notes, , Example: Calculate the degree of ionization of NH4OH in 0.001 M solution, at 25°C. The ionisation constant of NH4OH is 1.8 × 10–5 mol dm–3 at 25°C., Solution:, According to the Ostwald’s dilution law, , α=, , K, c, , 1.8 ×10−5, 0.001, = 0.134, =, , 12.2 GENERAL CONCEPTS OF ACIDS AND BASES, You are quite familiar with the terms acid, base, acidic and acidity etc. But how, do we define an acid or a base? There is no general definition of acids and bases., There are three different concepts of acids and bases ( proposed by Arrhenius,, Brønsted and Lowry and Lewis respectively) which are well known. Each of, these emphasize a different aspect of acid - base chemistry. Let us try to understand, these concepts., 12.2.1 Arrhenius Concept, The most commonly used concept of acids and bases was developed by Svante, Arrhenius (1884). According to this concept an acid is a substance that is capable, of producing hydrogen ion (H+ ) by dissociating in aqueous solution. The reaction, can be represented as, HA (aq) ⎯⎯, → H + (aq) + A − (aq), , (12.1), , Where HA represents the acid and A − refers to the acid molecule (ion) without, the hydrogen ion. Hydrochloric acid, HCl is an example of an Arrhenius acid, whose ionisation can be represented as, , CHEMISTRY, , 323
Page 353 :
MODULE - 5, , Ionic Equilibrium, , Chemical Dynamics, , HCl (aq) ⎯⎯, → H + (aq) + Cl − (aq), , (12.2), , The proton or hydrogen ion binds itself to a water molecule and form H3O+ ion, which is called hydronium ion., H + + H 2 O ⎯⎯, → H3O +, , Notes, , The hydronium ion is also known as oxonium ion or the hydroxonium ion., In the light of this fact the equation 12.1 can be rewritten as, HA (aq) + H 2O(l) ⎯⎯, → H3O+ (aq) + A − (aq), , (12.3), , A base on the other hand is defined as a substance capable of providing a hydroxyl, ion OH− on dissociation in aqueous solutions., MOH (aq) ⎯⎯, → M + (aq) + OH − (aq), , (12.4), , Where M+ refers to the base molecule (ion) without the hydroxyl ion. Sodium, hydroxide is an example of a Arrhenius base, dissociating as,, NaOH (aq) ⎯⎯, → Na + (aq) + OH − (aq), , (12.5), , Arrhenius concept is quite useful and explains the acid- base behaviour to a, good extent. However it has certain drawbacks like,, z, , It is limited to only aqueous solutions and require dissociation of the, substance., , z, , It does not explain the acidic behaviour of some substances which do not, contain hydrogen. for example, AlCl3. Similarly it does not explain the basic, character of substances like NH3 and Na2CO3 which do not have a hydroxide, groups., , 12.2.2 Brønsted and Lowry Concept, In 1923, Brønsted and Lowry pointed out independently that acid-base reactions, can be interpreted as proton-transfer reactions. According to them, an acid is, defined as a proton (H + ) donor, and a base is defined as a proton acceptor. The, definition is sufficiently broad and removes the first limitation of Arrhenius concept., Any hydrogen-containing molecule or ion capable of donating or transferring a, proton is an acid, while any molecule or ion that can accept a proton is a base., For example, in a reaction between ammonia (NH3 ) and hydrogen fluoride (HF);, ammonia acts as a base (accepts a proton) while HF behaves as an acid (donates, a proton)., NH 3 + HF ⎯⎯, → NH 4+ + F −, , 324, , (12.6), CHEMISTRY
Page 354 :
MODULE - 5, , Ionic Equilibrium, , According to Bronsted-theory an acid is a substance that can donate a, proton whereas a base is a substance that can accept a proton., , Chemical Dynamics, , You may note in this example that there is no role of a solvent. Let us consider, the reaction of hydrochloric acid with ammonia in a solvent like water. We can, write ionic equation as, H3O + (aq) + Cl − (aq) + NH3 (aq) ⎯⎯, → H 2 O(l ) + NH +4 (aq) + Cl −, , Notes, , The hydronium and chloride ions are obtained from the ionisation of HCl ., After cancelling Cl− on both sides, we get the following ionic equation as, NH3 (g) + H3O + (aq) ⎯⎯, → H 2 O(l ) + NH 4+ (aq), , (12.7), , Here, in aqueous solution, a proton is transferred from the hydronium ion, H3O+,, to the NH3 molecule, giving H2O and NH4+. In this case H3O+ acts as proton, donor or an acid and NH3 as proton acceptor or a base. We may visualise water, (the solvent) playing the role of mediator in the transfer of proton from, hydrochloric acid to ammonia. It should be noted that in the Brønsted- Lowry, concept, acids and bases can be either ions or molecular substances., In any acid-base equilibrium both forward and reverse reactions involve proton, transfers. Let us consider the reaction of NH3 with H2O., ZZX NH +4 (aq) + OH - (aq), H 2O(l) + NH3 (aq) YZZ, acid1, , base1, , acid 2, , base2, , (12.8), , In the forward reaction, NH3 accepts a proton from H2O. Thus, NH3 is a base, and H2O is an acid. In the reverse reaction, NH4+ donates a proton to OH–. Thus, NH4+ ion acts as an acid and OH– as a base. Here you find that NH3 and NH4+, differ by a proton. That is, NH3 becomes NH4+ ion by gaining a proton, whereas, NH4+ ion becomes NH3 molecule by losing a proton. The species NH4+ and NH3, are called conjugate acid-base pair. A conjugate acid-base pair consists of two, species in an acid-base reaction, one acid and one base, that differ by the gain or, loss of a proton. It can be represented as NH4+ / NH3 . The acid in such a pair is, called conjugate acid of the base, where as the base is the conjugate base of acid., Thus, NH4+ is the conjugate acid of NH3, and NH3 is the conjugate base of NH4+., The members of each conjugate pair are designated by the same numerical subscript, e.g., NH4+ / NH3 pair is marked as 2 while the H2O / OH– pair is marked as 1., Let us take another example say of ionization of hydrogen fluoride in water. It, can be represented as, ZZX H 3O + (aq) + F- (aq), HF (g) + H 2O YZZ, acid1, , base2, , acid 2, , base1, , (12.9), , The equilibrium sign indicates that the reaction does not go to completion. The, H+ ions obtained from the ionisation of HF molecule can be accepted by either of, CHEMISTRY, , 325
Page 355 :
MODULE - 5, Chemical Dynamics, , Ionic Equilibrium, , the two bases, F − and H2O. The fact that the equilibrium is only slightly towards, right tells us that it is the F − that accepts the proton and make the reaction go to, the left i.e., F − is a stronger base than H2O., Similarly when HCI is dissolved in water, the HCI molecules give up H+ ions, that can be accepted by either of two bases, Cl− and H2O., HCl + H 2 O ⎯⎯, → H3O + + Cl −, , Notes, , (12.10), , The fact that HCI is completely ionized in dilute aqueous solution ( indicated by, a single arrow) tells us that Cl− is a weaker base than H2O. We can conclude from, these two examples that, a stronger acid (HCl) has a weaker conjugate base, Cl−, and the weaker acid, (HF) has a stronger conjugate base, F −. We can generalize, that in a conjugate acid-base pair the weaker an acid is, stronger is its conjugate, base. Likewise, the weaker a base is, the stronger is its conjugate acid., Here you should understand that the terms ‘strong’ and ‘weak’ like many other, adjectives are used in a relative sense. Consider the following reaction, ZZX HF + OH F- + H 2O YZZ, , (12.11), , In this case F − is a weaker base as compared to OH–., Let us write a few reactions involving conjugate acid-base pairs., ZZX H 2CO3 (aq) + F- (aq), HF (aq) + HCO3- (aq) YZZ, acid1, , base2, , acid 2, , base1, , ZZX CO32- (aq) + H 2O(l), HCO3- (aq) + OH - (aq) YZZ, acid1, , base2, , base1, , acid 2, , ZZX HCO3- (aq) + HCN(aq), H 2CO3 (aq) + CN - (aq) YZZ, acid1, , base2, , base1, , acid 2, , (12.12), , If you examine the above reactions closely, you would find that some species, can act both as an acid and as a base. Such species are called amphiprotic species., In the above reactions HCO3–(aq) acts as a base in presence of HF but an acid in, presence of CN –. Similarly H2O behaves as an acid and a base., z, , Thus, we have seen that the Brønsted- Lowry concept of acids and bases has, greater scope than the Arrhenius concept., , 12.2.3 Lewis Concept, As discussed above the Brønsted- Lowry concept does not depend on the nature, of the solvent ( a short coming of the Arrhenius concept removed ). However,, like Arrhenius concept it does not explain the acidity of the substances which do, not have a hydrogen atom (e.g., AlCl3) and the basicity of the substances devoid, 326, , CHEMISTRY
Page 356 :
MODULE - 5, , Ionic Equilibrium, , of a OH group (e.g., Na2CO3). G.N.Lewis proposed (1923) a yet another concept, of acids and bases that includes such substances also. According to him, an acid, may be defined as, ‘ any atom, molecule or ion that can accept an electron pair, from any other atom , molecule or ion, A lewis base on the other hand can be, defined as, ‘ any atom , molecule or ion that can donate a pair of electrons, Let us, take an example, AlCl3 + NH3 ⎯⎯, → Cl3Al ←⎯, ⎯ NH3, , (12.13), , Chemical Dynamics, , Notes, , In the above example AlCl3 is an electron deficient species. It accepts an electron, pair from a molecule of NH3 which has a lone pair of electrons on N atom. Thus,, AlCl3 is a Lewis acid and NH3 is a Lewis base., , INTEXT QUESTIONS 12.1, 1. Define Arrhenius acid and give two examples., 2. What are the limitations of Arrhenius definition?, 3. How does a Brønsted- Lowry base differ from an Arrhenius base?, 4. Classify the following into Brønsted- Lowry acid or Brønsted- Lowry base., HCl, NH3, H3O+, CN −, 5. The degree of dissociation of two electrolytes X and Y are 1.0 × 10–4 and, 0.96. Comment on the nature of these electrolytes., , 12.3 RELATIVE STRENGTH OF ACIDS AND BASES, Different acids and bases have different strength depending on their nature. Since, there are different ways of defining acids and bases, there are different ways of, comparing their relative strengths also., 12.3.1 Relative strength according to Arrhenius concept, According to Arrhenius concept strong electrolytes (like HCl) which dissociate, completely in aqueous solutions and produce H+ ( or H3O+) ions are called strong, acids., HCl (g) + H 2O ⎯⎯, → H3O + (aq) + Cl − (aq), , (12.14), , Other examples of strong acids are H2SO4, HBr, HI, HNO3 and HClO4. On the, other hand weak electrolytes like CH3COOH whose ionisation is not complete,, ( because the process is reversible) and produce H+ ( or H3O+) ions are called, weak acids., ZZX H + (aq) +CH 3COO - (aq), CH 3COOH (aq) + H 2O YZZ, CHEMISTRY, , (12.15), 327
Page 357 :
MODULE - 5, Chemical Dynamics, , Notes, , Ionic Equilibrium, , Similarly strong bases may be defined as the electrolytes with complete ionisation, and weak bases as the electrolytes with incomplete ionisation. NaOH and NH3, are typical examples of strong and weak bases respectively. Their ionisation can, be represented as, NaOH (aq) ⎯⎯, → Na + (aq) + OH − (aq), , (12.16), , ZZX NH +4 (aq) + OH - (aq), NH 3 (aq) + H 2 O YZZ, , (12.17), , The principal strong bases are hydroxides of Groups 1 and 2 elements (except, Be). Most of the other acids and bases we come across are weak bases., 12.3.2 Relative strength according to Brønsted- Lowry concept, You have learnt that according to Brønsted- Lowry concept an acid is a species, that donates a protons while a base is a species that accepts a protons. The, tendency of a substance to donate a proton also depends on the species accepting, the proton. A given acid may have different strengths in different solvents ( of, varying basic strength or proton accepting tendencies) e.g.,, CH3COOH +, , +, , CH3COO – +, NH2 , , CH3, CH3COOH + H2SO4 , , NH3, , C, , OH + HSO–4, , O, , H, , +, , (12.18), , (12.19), , Acetic acid loses a proton to a basic solvent aniline but in sulphuric acid it infact, accepts a proton and acts as a base. Therefore the relative strengths of different, acids and bases are compared in the same solvent which normally is water., Therefore, in Brønsted- Lowry concept, we may define the relative strength of, an acid as its relative tendency to lose (or donate) a proton to water. According, to the Brønsted- Lowry concept strong acid are those which lose their protons, almost entirely to water. The reaction,, HCl (aq) + H 2 O (aq) ⎯⎯, → H3O + (aq) + Cl − (aq), acid, , base, , acid, , base, , (12.20), , goes completely to the right indicating that HCl is a strong acid. Acetic acid, donates its proton to water to the extent of only about 3% and the following, equilibrium exists., ZZX H 3O + + CH3COOCH 3COOH + H 2 O YZZ, , (12.21), , Acetic acid is, therefore, termed as a weak acid., 328, , CHEMISTRY
Page 358 :
MODULE - 5, , Ionic Equilibrium, , Chemical Dynamics, , 12.4 QUANTITATIVE ASPECTS OF STRENGTHS OF, ACIDS AND BASES, We have so far discussed the relative strengths of acids and bases only qualitatively, i.e., which is strong and which one is weak. Many a times we need to know the, quantitative aspects also i.e., how much?. That is if a acid is stronger than the, other we would like to know how many times stronger. Let us learn how do we, quantify it ?, , Notes, , 12.4.1 Ionisation of weak acids, The dissociation or ionisation of a weak acid, HA, can be represented as, ZZX H3O + (aq) + A - (aq), HA (aq) + H 2 O (l) YZZ, , (12.22), , As you know that in case of strong acids the ionisation is almost complete or, close to 100% or we may say that the equilibrium lies far to the right. In such, cases the sign of equilibrium may be replaced by a single arrow (→), HA (aq) ⎯⎯, → H + (aq) + A − (aq), , or, HA (aq) + H 2 O (l ) ⎯⎯, → H3O + (aq) + A − (aq), , ...12.23, , The reaction given above (eq 12.22) is referred to as ionisation equilibrium and is, characterized by an equilibrium constant, K eq =, , [H3O + ][A − ], [H 2O][HA], , ...12.24, , Since the concentration of a pure liquid or a solid is taken as 1, we can rewrite the, above expression can as, K eq =, , [H3O + ][A − ], = Ka, [HA], , ...12.25, , where Ka is a new constant called acid dissociation constant or ionisation constant, of the acid., The magnitude of the equilibrium constant is a measure of the strength of the, acid. Higher the value of the equilibrium constant the stronger is the acid. For all, strong acids the values of the equilibrium constants is quite high and does not, help much in suggesting their relative strengths. However, for a weak acid , this, constant is quite useful., , CHEMISTRY, , 329
Page 359 :
MODULE - 5, Chemical Dynamics, , Ionic Equilibrium, , Example 12.1 : Write down the expression for the dissociation constant for acetic, acid- a weak acid that ionizes as, ZZX H 3O + (aq) + CH 3COO - (aq), CH 3COOH (aq) + H 2 O YZZ, , Solution : Applying the law of chemical equilibrium, we can write equilibrium, constant K as, Notes, , K=, , [CH3COO − ][H3O + ], [CH3COOH][H 2O], , Rearranging, we can write ., K[H 2O] = K a =, , [H3O + ][CH3COO − ], [CH3COOH], , Dissociation constant of other weak acids also can be written in the same way., The values of Ka show the extent to which the acids are dissociated in water., Relative strength of acids is judged on the basis of dissociation constant. Like, other equilibrium constants the dissociated constant, Ka also depends on the, temperature. Therefore, the ionisation constants or dissociation constants are, compared at the same temperature. For example, ZZX H + (aq) + CH3COO- (aq) Ka = 1.8 × 10–5, CH 3COOH (aq) YZZ, , (12.26), , ZZX H + (aq) + CN - (aq), HCN (aq) YZZ, , (12.27), , Ka = 4.9 × 10–10, , On the basis of Ka values we can say that acetic acid is much more ionized than, hydrocyanic acid. In other words acetic acid is stronger acid than hydrocyanic, acid although both are weak; neither is completely ionized., 12.4.2 Ionisation of weak bases, The ionisation of weak bases (BOH) can be expressed as :, ZZX B+ (aq) + OH - (aq), BOH (aq) YZZ, , (12.28), , (The equilibrium sign may be replaced by → in case of a strong base.), The solution contains the base, B the protonated base, BH+, hydroxide ion, OH −, and water in equilibrium. The equilibrium constant expression for the, reaction is, Kb =, , 330, , [B+ ][OH − ], [BOH], , (12.29), , CHEMISTRY
Page 360 :
MODULE - 5, , Ionic Equilibrium, , Chemical Dynamics, , For example, the dissociation of NH4OH is represented as, ZZX NH 4+ (aq) + OH - (aq), NH 4 OH (aq) YZZ, , and is characterized by, Kb =, , [NH +4 ][OH − ], , (12.30), , [NH 4OH], , Notes, , The constant Kb is called dissociation constant of the base. Similar to values of, Ka, Kb values also give us the idea about the relative strengths of weak bases., Higher the value of Kb the stronger is the base., 12.4.3 Polyprotic Acids, Many acids have more than one ionizable protons. These are called polyprotic, acids. The acids are called diprotic if there are two ionizable protons per molecule., (e.g. H2SO3, H2CO3), and triprotic if there are three ionizable protons (e.g. H3PO4,, etc). Such acids dissociate in more than one steps or stages, each with its own, ionization constant. In the case of sulphurous acid, H2SO3, these steps are, ZZX HSO3- + H 3O +, H 2SO3 + H 2O YZZ, , (12.31), , [H3O + ][HSO3− ], K1 =, = 1.3 × 10–2, [H 2SO3 ], , ZZX SO32- + H 3O +, HSO3- + H 2 O YZZ, K2 =, , [H3O + ][SO32 − ], [HSO3− ], , (12.32), , = 6.3 × 10–8, , The values of the two ionisation constants ( K1 and K2) are quite different ; K1, being twenty million times K2. It suggests that the first ionisation of sulphurous, acid is much more than the second one. In other words the sulphurous acid behaves, as a much stronger acid than the bisulphite ion., 12.4.4 Degree of Ionisation or Degree of Dissociation, As you know that the weak acids / bases do not ionize completely and an, equilibrium exists between the ionized and unionized species. The degree of, ionisation may be defined as the fraction of total amount of a weak acid or a base, that exists in the ionized form. It is denoted by a Greek letter ‘á ‘. The equilibrium, constant can be used to calculate the degree of ionisation of a weak acid or a, base. An expression relating á and Ka or Kb can be derived as follows., , CHEMISTRY, , 331
Page 361 :
MODULE - 5, Chemical Dynamics, , Ionic Equilibrium, , Consider a weak acid HA which partially dissociates in its aqueous solutions and, the following equilibrium is established, ZZX H 3O + (aq) + A - (aq), HA (aq) + H 2O(l) YZZ, , Notes, , Initial concentrations, (in moles), , c, , ~ 55, , 0, , 0, , Equilibrium concentrations, , c(1–α), , ~ 55, , cα, , cα, , The equilibrium constant expression can be written as, Ka =, , [H3O + ][A − ] [cα ][cα ], =, [H 2 O][HA] c (1 − α ) 55, , rearranging we get,, ⇒, , 55K = K a =, , [cα ][cα ], c 2α 2, cα 2, =, =, c [1 − α ] c [1 – α ] (1 − α ), , (12.33), , Since the acid HA is very weak, α < < 1; we can neglect á in comparison to 1 in, the denominator to get, K a = cα 2, , or, , α2 =, , Ka, or α =, c, , Ka, c, , (12.34), , So if we know the value of the dissociation constant of the acid and the, concentration of the weak acids we can find its degree of dissociation or ionisation., Let us take up an example to see the application of this relationship., Example 12.2: Compute the degree of dissociation and percent dissociation of, acetic acid in its 0.1 M solution. Given Ka = 1.8 x 10–5., Solution: Using the formula α =, , Ka, and substituting the values of Ka and c,, c, , we get,, α=, , 1.8 × 10 −5, = 1.8 × 10 −4 = 1.34 × 10 −2 = 0.0134, 0.1, , The percent dissociation of a weak acid is defined as, Percent dissociation =, , The number of moles of acid in the dissocia, Total number of moles of the acid, , (12.35), = Degree of dissociation × 100%, ⇒, 332, , = α × 100% = 0.0134 × 100 = 1.34%, CHEMISTRY
Page 362 :
MODULE - 5, , Ionic Equilibrium, , Thus acetic acid is dissociated to the extent of only 1.34 % in a 0.1 M aqueous, solution., , Chemical Dynamics, , A similar expression can be obtained for the degree of dissociation of a weak, base. The desired expression is, α=, , Kb, c, , (12.36), , Notes, , 12.5 THE AUTO-IONISATION OR SELF-IONISATION OF, WATER, We have seen that water can act as a very weak acid and also as a very weak base., In a sample of water a small number of water molecules undergo auto ionisation., Half of them acting as an acid while the other half acting as a base. As a consequence, small concentration of H3O+ and OH − are formed in water. The self ionisation of, water can be represented as, ZZX H 3O + + OH H 2O + H 2O YZZ, , (12.37), , The corresponding equilibrium constant expression can be written as, K eq =, , [H3O+ ][OH − ], [H 2O]2, , (12.38), , Since the concentration of H2O is constant we can rearrange the expression and, define a new constant Kw , as, [H3O+] [OH−] = Keq × [H2O]2 = Kw (a new constant), , (12.39), , This constant, Kw, is called the dissociation constant or ionic product constant, of water. The value of Kw at 298 K has been determined from the measurement of, electrical conductivity of carefully purified water and has been found to be 1.0 ×, 10–14 mol2 dm-6., Since the concentrations of H3O+ and OH − ions is equal we may write, Kw = [H3O+] [OH−] = 1.0 × 10–14 mol2 dm–6, Kw = [H3O+]2 = 1.0 × 10–14 mol2 dm–6, ⇒, , [H3O+] = 1.0 × 10−7 mol dm−3, , and similarly,, , [OH−] = 1.0 × 10−7 mol dm−3, , Thus in pure water and in neutral solutions, [H3O+] = [OH−] = 1.0 × 10−7 mol dm−3 at 298 K, CHEMISTRY, , (12.40), 333
Page 363 :
MODULE - 5, Chemical Dynamics, , Ionic Equilibrium, , 12.5.1 Acidic, Basic and Neutral Solutions, An acidic solution is defined as one in which the hydrogen ion ( or hydronium, ion) concentration is greater than the hydroxide ion concentration. A basic, solution is one in which the reverse is true, that is, one in which [OH − ] exceeds, [H3O+] and a neutral solution is one in which [OH − ] equals [H3O+]., , Notes, , Neutral solution, , [H3O+] = [OH − ], , Acidic solution, , [H3O+] > [OH − ], , Basic solution, , −, [H3O+] < [OH ], , (12.41), , Since the product [H3O+] [OH − ] is constant, if the concentration of either of, these increases, the other one would decrease. In other words the concentrations, of [H3O+] and [OH − ] are not independent but are linked by the relationship, −, [H3O+] [OH ] = Kw, , (12.42), , This provides an easy way to calculate concentration of one of these if we know, that of the other., You must note that the self ionisation equilibrium discussed above applies not, only to pure water but also to the self-ionization of water in any aqueous solution., Hydronium ions and hydroxide ions are present in every aqueous solution, and, they are always in equilibrium with water molecules. Let us try to calculate the, concentration of these ions in some simple solutions., Example 12.3 : Calculate the concentrations of OH − and H3O+ ions in 0.01 M, solution of HCl., Solution: In an aqueous solution of HCl, the following two processes would, occur simultaneously, ZZX H 3O + + OH H 2O + H 2O YZZ, , ZZX H 3O + + ClHCl + H 2O YZZ, , The ionisation of HCl goes to completion and that of water to a very limited, extent. In addition according to Le- Chatlier principle, the H3O+ from the ionization, of HCl will shift the position of the equilibrium of the self-ionization reaction to, the left. As a consequence the concentration of the OH− ions would reduce further., Suppose concentration of OH− is ‘x’ mol dm−3, then concentration of H3O+ from, the self-ionization of water must also be x mol dm−3. The concentration of H3O+, from ionization of HCl is 0.010 mol dm−3. Therefore, total concentration of H3O+, (aq) = (0.010 + x) mol dm−3., Thus Equilibrium Concentrations of H3O+ and OH− ions would be (0.01 + x) and, x mol dm−3 respectively., 334, , CHEMISTRY
Page 364 :
MODULE - 5, , Ionic Equilibrium, , Substituting these values into the equilibrium constant for the self-ionization of, water, we get, , Chemical Dynamics, , Kw = [H3O+] [OH−] = (0.01 × x) (x) mol2 dm−6 = 1.0 × 10−14 mol2 dm−6, Since x must be very small, we can assume that x << 0.01 and therefore we may, assume that the equilibrium concentration of H3O+ is equal to 0.01 M, (0.01 + x) ≅ 0.01, so, , Notes, , 0.01x = 1.0 × 10−14, x = 1.0 × 10−14 / 0.01, , or, , x = 1.0 × 10−12, [OH−] = 1.0 × 10−12 mol dm−3 and, [H3O+] = 0.01 + 1.0 × 10−12 mol dm−3 = 0.01 mol dm−3, Since the value of x (1.0 × 10−12) the hydronium ions obtained from the self, ionisation of water) is far smaller than 0.01, our assumption is justified. Thus you, may note that in case of aqueous solutions of strong acids it is reasonable to, assume that the concentration of H3O+ ions equals the concentration of the acid, itself., 12.5.2 pH Scale, In aqueous solutions of acids and bases the concentration of H3O+ and OH −, ions may vary from about 10 M to 10−14 M. It is quite inconvenient to express, these concentrations by using powers of 10. in 1909 a Danish botanist S.P.L., Sorensen proposed a logarithmic scale ( called pH scale) for expressing the, concentrations of H+ ions. He defined pH as the negative logarithm of the molar, concentration of hydrogen ions. That is,, pH = − log10 [H + ], , (12.43), , We now a days it is represented as, pH = − log10 [H3O+ ], , (12.44), , For neutral solutions ( or pure water), [H3O+] = [OH−] = 1 × 10–7, ⇒, , pH = –log 1 × 10–7 = 7.0, , For acidic solutions, [H3O+] > [OH−], ⇒, , [H3O+] > 1 × 10–7, , CHEMISTRY, , 335
Page 365 :
MODULE - 5, Chemical Dynamics, , Ionic Equilibrium, , ⇒, , pH = –log (>1 × 10–7 ) = < 7.0, , For basic solutions, [H3O+] < [OH−], , Notes, , ⇒, , [H3O+] < 1 × 10–7, , ⇒, , pH = –log ( < 1 × 10–7 ), , ⇒, , > 7.0, , A strongly acidic solution can have a pH of less than zero ( i.e., negative) and a, strongly alkaline solution can have a pH value greater than 14. However the pH, range normally observed is between 0 to 14., The notation p is used in a number of places. It carries the meaning, ‘the negative, −, logarithm of’. It has been extended to OH (aq) and equilibrium constants like,, Ka, Kb and Kw, etc., pOH = − log10 [OH − ], pK a = − log10 K a, pK b = − log10 K b, pK w = − log10 K w, , (12.45), , This gives us an important relation. You would recall (eq 12.42), Kw = [H3O+] [OH−], Taking logs on both sides, we get, log Kw = log [H3O+] + log [OH−], Multiplying through out by –1, −log Kw = − log [H3O+] − log [OH−], pKw = pH + pOH, Since the value of Kw = 1.0 × 10–14 pKw = 14, i.e.,, , pH + pOH = 14, , (12.46), , If we know the value of pH of a given solution we can find its pOH and vice, versa., Let us take up some examples to understand the application of these important, formulae., Example 12.4: What is the pH of a 0.01 M aqueous solution of HCl?, Solution: Since HCl is a strong acid it would ionize completely., Therefore, [H3O+] in 0.01 M HCl = 0.01 M, pH = − log10 [H3O+ ] = − log 10−2, , = – (–2.0) = 2.0., 336, , CHEMISTRY
Page 366 :
MODULE - 5, , Ionic Equilibrium, , Example 12.5 : Calculate the pH of 0.010 M aqueous solution of NaOH., , Chemical Dynamics, , Solution: Since NaOH is a strong base, it is fully ionized to give Na+ and OH−, [OH−] = 1.0 × 10−2 mol L−1, Kw = [H3O+] [OH−] = 1.00 × 10−14 mol2 L−2, [H3O + ] =, , So,, , Kw, , [OH − ], , =, , 1.00 ×10 −14 mol2 dm −6, , Notes, , 1.00 × 10−2 mol dm −3, , = 1.00 × 10–12 mol dm–3, pH = –log (1.0 × 10–12) = 12, 10, , Example 12.6 : The pH of a sample of rain water at 25 °C is 5. What is the, concentration of hydronium ions ?, Solution: pH = − log [H3O+], or, , 5 = −log [H3O+] ⇒ log [H3O+] = –5, , Taking antilogs, we get, [H3O+] =10−5 mol dm−3., Example 12.7: Calculate the pH of 0.1 M aqueous solution of acetic acid. The, dissociation constant, Ka= 1.85 x 10−5, α = 0.0134., Solution: The following equilibrium exists in this solution, ZZX H 3O + (aq) + CH 3COO - (aq), CH 3COOH (aq) + H 2 O YZZ, , If α be the degree of dissociation of acetic acid in this solution, the equilibrium, concentrations of various species would be, ZZX H 3O + (aq) + CH 3COO - (aq), CH3COOH(aq) + H 2O YZZ, , c(1– á), , cá, , cá, , 0.1á, , 0.1á, , Since c = 0.1 M, 0.1(1– á), [H3O+] = c á, ⇒, , [H3O+], , = 0.1 × 0.0134 = 0.00134, , pH = − log[H3O+] = − log [ 0.00134] = − log [1.34 × 10−3] = −(−2.87 ) = 2.87, , CHEMISTRY, , 337
Page 368 :
MODULE - 5, , Ionic Equilibrium, , CH3COO– = 0.1 + 0.1á = 0.1 (1 + á), , Chemical Dynamics, , H3O+ = 0.1á, Ka =, , [H3O + ][CH3COO − ], [CH3COO − ], , rearranging the expression we get,, [H3O + ] = K a =, , Notes, , [CH3COOH], [CH3COO − ], , Substituting the values, we get, [H3O+ ] = 1.85 × 10−5 ×, , 0.1 (1 − α ), 0.1 (1 + α ), , Since acetic acid is a weak acid, its degree of dissociation further decreases in, presence of acetate (ions the common ion). Therefore it is reasonable to assume, that, , α < < 1; and (1 – α) ~ 1; also (1 + α) ~ 1, This gives, [H3O+] =, , 1.85 × 10–5, , × 0.1 / 0.1 = 1.85 × 10–5, , and, , pH = –log (1.85 × 10–5) = 4.73, , Also, , since [H3O+] = 0.1 α, ∴ α = 1.85 × 10–5/ 0.1 = 1.85 × 10–4 = 0.000185, , The concentration of different species at equilibrium will be, CH3COOH = 0.1 ( 1 − 0.000185 ) = 0.1, CH3COO− = 0.1 ( 1 + 0.000185 ) = 0.1, H3O+ = 0.1 × á = 0.1 × 0.000185 = 1.85 × 10–5, ⇒, , the concentration of acid = initial concentration of acid, , ⇒, , the concentration of CH3COO− ions = initial concentration of the salt, , INTEXT QUESTIONS 12.2, 1., 2., , HF is a weak acid in water. Write down the expression for Ka for the, dissociation of HF., Consider a weak base BOH which partially dissociates in its aqueous solutions, as per the following equilibrium, , CHEMISTRY, , 339
Page 369 :
MODULE - 5, , Ionic Equilibrium, , Chemical Dynamics, , ZZX BH + + OH B + H 2 O YZZ, , 3., 4., Notes, , A sample of lime juice has a hydronium ion concentration of 6.3 × 10−2 M., Calculate its pH., Calculate the pH of 1.0 M aqueous solution of amino acid glycine – a weak, acid. The Ka = 1.67 ×10−10., , 12.6 BUFFER SOLUTIONS, The example discussed above leads us to a very important conclusion that the, extent of dissociation of a weak acid can be decreased by adding a salt containing, a common ion. Further, it can be shown that the change in the extent of dissociation, can also be varied by changing the concentration of the common ion. The mixture, of a weak base and a salt of common ion also behave in the same way. These, aqueous solutions containing weak acids / bases and a salt of common ion are, important in a yet another way. These act as buffer solutions., Buffer solutions are the ones that resist a change in its pH on adding a small, amount of an acid or a base., In laboratory reactions, in industrial processes and in the bodies of plants and, animals, it is often necessary to keep the pH nearly constant despite the addition, of acids and bases. The oxygen carrying capacity of haemglobin in our blood and, activity of the enzymes in our cells depends very strongly on the pH of our body, fluids. pH of the blood is very close to 7.4 and pH of saliva is close to 6.8., Fortunately, animals and plants are protected against sharp changes in pH by the, presence of buffers., There are two kinds of commonly used buffer-solutions, (i), , A weak acid and a soluble ionic salt of the weak acid e.g. acetic acid and, sodium acetate; CH3COOH + CH3COONa and,, , (ii) A weak base and a soluble ionic salt of the weak base e.g. ammonium, hydroxide and ammonium chloride ; NH4OH + NH4Cl., The buffers with pH less than 7 are called acidic buffers and those with pH above, 7 are called basic buffers. Acetic acid - sodium acetate buffer is an example of, acidic buffer while Ammonium hydroxide - ammonium chloride is a basic buffer., 12.6.1 Buffer Action, A buffer system contains a conjugate acid- base pair and the concentrations of, these two are quite high as compared to that of the hydronium ions. These are, called as the acid reserve and the base reserve respectively. The added acid or, base reacts with these reserves and gets consumed without significantly altering, 340, , CHEMISTRY
Page 370 :
Ionic Equilibrium, , the hydronium ion concentration and therefore the pH does not change, significantly. Let us consider a buffer solution containing acetic acid, CH3COOH, and sodium acetate CH3COONa to understand the buffer action., In acetic acid - sodium acetate buffer CH3COOH is the acid reserve while, CH3COONa ( or CH3COO− ions ) is the base reserve. In the solution mixture the, added components dissociate as follows. The weak acid dissociates partially while, the salt undergoes complete dissociation., , MODULE - 5, Chemical Dynamics, , Notes, , ZZX H 3O + (aq) + CH 3COO- (aq), CH 3COOH (aq) + H 2O(l) YZZ, CH 3COONa (aq) ⎯⎯, → Na + (aq) + CH 3COO- (aq), , If we add a strong acid such as HCI to this solution, it produces H3O+. These, added H3O+ (acid) react with an equivalent amount of the base reserve, [CH3COO−] to generate undissociated acetic acid. The reaction being, H 3O + (aq) + CH 3COO − (aq) ⎯⎯, → CH 3COOH(aq) + H 2 O(l ), , The net effect of this reaction is that there is a slight increase in the concentration, of the acid reserve and an equivalent decrease in the concentration of the base, reserve. The effective reaction being, HCl(aq) + CH3COONa(aq) ⎯⎯, → CH3COOH(aq) + NaCl(aq), , Similarly, when small amounts of a strong base like NaOH is added , it generates, OH − ions. These additional OH − neutralize some of the H3O+ ions present in the, solution,, ZZX H3O + (aq) + CH3COO- (aq), H 3O + (aq) + OH - (aq) YZZ, , Since one of the products of the acid dissociation equilibrium (eq) is used up,, there is some more ionisation of CH3COOH to re-establish the equilibrium., ZZX H 3O + (aq) + CH 3COO - (aq), CH 3COOH(aq) + H 2O YZZ, , The net result is the neutralization of OH − by CH3COOH. In other words we can, say that the added OH− ions ( base) react with the acid reserve to produce, CH3COO− ions, OH − (aq) + CH3COOH(aq) ⎯⎯, → CH3COONa − + H 2 O(l ), , The effective reaction being the reaction of the added base with acid reserve., NaOH(aq) + CH3COOH(aq) ⎯⎯, → CH3COONa(aq) + H 2O(l ), , The net effect of this reaction is that there is a slight increase in the concentration, of the base reserve and an equivalent decrease in the concentration of the acid, reserve., You may note here that the added acid or the base only cause minor changes in, the concentrations of the weak acid and the salt. The concentration of the, CHEMISTRY, , 341
Page 371 :
MODULE - 5, Chemical Dynamics, , Ionic Equilibrium, , hydronium ions and thereby the pH does not change significantly. Let us derive a, mathematical expression for determining the pH of a buffer solution., 12.6.2 Henderson-Hasselbalch Equation, , Notes, , This equation relates the pH of a given buffer solution to the concentrations of its, components viz. weak acid / salt or weak base / salt. Let us derive the expression, for an acidic buffer system that we have discussed above. In acetic acid – sodium, acetate buffer the central equilibrium is, ZZX H 3O + (aq) + CH 3COO - (aq), CH 3COOH(aq) + H 2O YZZ, , which is characterized by the acid dissociation constant,, Ka =, , [H3O + ][CH3COO − ], [CH3COOH], , rearranging, we get, [H3O + ] = K a ×, , [CH3COOH], [CH3COO − ], , The concentration of undissociated acetic acid can be taken as total acid, concentration [Acid] and that of sodium acetate as the total salt concentration, [Salt]. In the light of this the above equation may be re written as, [H3O + ] = K a, , [Acid], [Salt], , Taking logarithm and multiplying through out by (-1) we get, − log [H + ] = − log K a − log, , [Acid], [Salt], , Recall that pH = −log [H3O+] and pKa = −log Ka. This gives the desired equation., pH = pK a − log, , [Acid], [Salt], = pK a + log, [Salt], [Acid], , This equation is known as Henderson-Hasselbalch equation. A similar expression, can be derived for a basic buffer ( e.g., ammonium hydroxide and ammonium, chloride) . The expression is, pOH = pK b + log, , [Salt], [Base], , Let us take up some examples to see the application of this equation., , 342, , CHEMISTRY
Page 372 :
MODULE - 5, , Ionic Equilibrium, , Example 12.9 : Calculate the pH of acetic acid – sodium acetate buffer containing, 0.1 M acetic acid and 0.1 M sodium acetate. (Ka = 1.85 × 10–5 mol dm–3)., , Chemical Dynamics, , Solution: Here, [ Acid] = 0.1 M and [Salt] = 0.1 M, Since Ka = 1.85 × 10–5 mol dm–3 ;, pKa = −log Ka = −log 1.85 × 10–5, ⇒, , Notes, , pKa = 4.73, [Salt], , According to Henderson equation, pH = pKa + log [Acid], Substituting the values in Handerson equation, we get, pH = 4.73 + log ( 0.1 / 0.1 ) = 4.73 + log 1 = 4.73., Example 12.10 : Calculate the pH of ammonium hydroxide – ammonium chloride, buffer solution that is 0.1 M in ammonium hydroxide and 0.01 M in ammonium, chloride. ( pKb of NH4OH = 9.25)., Solution: Here, [ Base] = 0.1 M and [ Salt} = 0.01 M, Since pK = 9.25 ;, b, , [Salt], , According to Henderson equation, pOH = pKb + log [Base], Substituting the values in Handerson eqation, we get, pOH = 9.25 + log ( 0.01 / 0.1 ) = 9.25 + log 0.1 = 9.25−1.0 = 8.25, , 12.7 SALT HYDROLYSIS, The aqueous solutions of certain salts also behave as acids or bases. They do so, because of the hydrolysis of the cation or anion or both. As you know, hydrolysis, is a reaction with water. Depending on the behaviour towards hydrolysis there, are four different types of salts., Salt of strong acid + strong base (eg. HCl + NaOH), , NaCl, , Salt of strong acid + weak base ( e.g. HCl + NH4OH), , NH4Cl, , Salt of weak acid + strong base ( e.g. CH3COOH + NaOH), , CH3COONa, , Salt of weak Acid + weak Base (e.g. CH3COOH + NH4OH), , CH3COONH4, , Let us learn about the acid- base behaviour of the different types of salts., Salt of strong acid + strong base : the cations of the strong bases and the anions, of the strong acids do not get hydrolysed. Therefore the salts of this category do, not show any acid-base behaviour and are neutral., CHEMISTRY, , 343
Page 373 :
MODULE - 5, Chemical Dynamics, , Ionic Equilibrium, , Salt of strong acid + weak base: the salts of this type dissociate in aqueous, solutions to give a cation of a weak base and the anion belonging to strong acid, . For example, NH4Cl dissociates as :, NH 4Cl(aq) ⎯⎯, → NH +4 (aq) + Cl − (aq), , Notes, , As mentioned above, the anion does not get hydrolysed but the cation does get, hydrolysed as per the following equation., NH 4+ (aq) + H 2 O(l ) ⎯⎯, → NH 4 OH + H + (aq), , since it generates H+(aq) ions, the solution is acidic in nature., Salt of weak acid and strong base: the salts of this type dissociate in aqueous, solutions to give a anion of a weak acid and the cation belonging to strong base., for example, CH3COONa dissociates as :, CH 3COONa(aq) ⎯⎯, → Na + (aq) + CH 3COO − (aq), , in this case the cation does not get hydrolysed but the anion does get hydrolysed, as per the following equation., ZZX CH 3COOH (aq) + OH - (aq), CH 3COO - (aq) + H 2 O(l) YZZ, , Since it generates hydroxyl ions the solution is basic in nature., Salt of weak Acid and weak Base : the salts of this type dissociate in aqueous, solutions to give a anion of a weak acid and the cation belonging to a weak base., for example, ammonium acetate, CH3COONH4 dissociates as :, ZZX NH 4+ (aq) + CH 3COO- (aq), CH 3COONH 4 (aq) YZZ, , In this case both the cation as well as the anion would undergo hydrolysis and the, nature of the solution, whether acidic, basic or neutral would depend on the, relative strength of the weak acid and the weak base., , 12.8 THE SOLUBILITY EQUILIBRIUM, When we try to dissolve a solid into water, if it dissolves, there are three, possibilities:, 1., , The solid is a non-electrolyte and it dissolves as neutral molecules., , 2., , The solid is a highly soluble electrolyte; it dissolves almost completely., , 3., , The solid is a sparingly soluble electrolyte; it dissolves to a limited extent., , It is the third possibility that interests us here. Let us take the example of dissolution, of AgCl to understand the equilibria in such cases. When silver chloride is added, to water, the following equilibrium is established., ZZX Ag + (aq) + Cl- (aq), AgCl(s) YZZ, 344, , CHEMISTRY
Page 374 :
Ionic Equilibrium, , This is an example of a heterogeneous equilibrium because it involves both a, solid and a solution. This equilibrium is known as the solubility equilibrium for, which the equilibrium constant expression is, K=, , MODULE - 5, Chemical Dynamics, , [Ag + ][Cl− ], [AgCl(s)], , As a matter of convention the concentration of the undissolved solid is taken as, one. We can rewrite the equilibrium as, , Notes, , K sp = [Ag + ] [Cl − ], , The equilibrium constant now is the product of the concentrations of the ions. It, is called solubility product constant or simply solubility product. A new symbol,, Ksp, has been assigned to this constant. The mass expression on the right , is, called, ion product or ionic product. The solubility product constant of a given, salt is constant at a given temperature., 12.8.1 Relationship between Solubility and Solubility Product Constant, The solubility product constant for a substance is related to its solubility. The, nature of relationship depends on the nature of the salt., Salt of AB type: ( For example AgCl, CaSO4). In such cases the solubility, equilibrium can be represented as, ZZX A + (aq) + B- (aq), AB(s) YZZ, and K = [A+] [B–], sp, , If the solubility of salt is ‘s’ mol dm−3 then the concentrations of the cations and, the anions would be ‘s’ mol dm−3 each. Substituting the values in the expression, of Ksp we get,, Ksp = [‘s’ mol dm−3 ] × [‘s’ mol dm−3] = s2 mol2 dm−6, Salt of AB2 type: (For example CaF2). In such cases the solubility equilibrium, can be represented as, ZZX A 2+ (aq) + 2B- (aq), AB2 (s) YZZ, , and, , Ksp = [A2+] [B–]2, , If the solubility of salt is ‘s’ mol dm−3 then the concentration of the cations and, the anions would be ‘s’ mol dm−3 and ‘2s’ mol dm−3 respectively. Substituting the, values in the expression of Ksp we get,, Ksp = [‘s’ mol dm−3 ] × [‘2s’ mol dm−3]2 = 4s3 mol3 dm−9, , CHEMISTRY, , 345
Page 376 :
MODULE - 5, , Ionic Equilibrium, , Example 12.12: Solubility product of silver iodide, Agl is 8.5 × 10−17 at 25°C., What is the molar solubility of AgI in water at this temperature?, , Chemical Dynamics, , Solution: Silver iodide dissolves according to the equation, ZZX Ag + (aq) + I- (aq), Agl(s) YZZ, Let the solubility of AgI be is ‘s’ mol dm−3 the concentrations of silver and iodide, ions would be is ‘s’ mol dm−3 each., , Notes, , At equilibrium, Ksp = [Ag+] [I−] ; Substituting the values, we get, [‘s’ mol dm−3 ] [‘s’ mol dm−3 ] = s2 mol2 dm−6 = 8.5 × 10−17 mol2 dm−6, This gives , solubility (s) = [8.5 × 10−17 mol2 dm−6 ]1/2, = 9.2 × 10−9 mol dm−3., The solubility of AgI in water is therefore 9.2 × 10−9 mol dm−3 at 298 K., 12.8.2 Effect of Common Ion on Solubility Equilibria, What will happen if we add a soluble salt containing a common-ion to a solution, of a sparingly soluble salt ? You may reason out that according to Le Chatelier’s, principle, the common-ion will shift the equilibrium in backward direction which, would reduce its solubility still further. This actually is the case. Let us understand, it with the help of an example., Example 12.13: Calculate the molar solubility of AgI in a solution containing, 0.1 mol dm−3 AgNO3. The solubility product of silver iodide, Agl is 8.5 × 10−17 mol2, dm−6 at 298 K., Solution: Silver nitrate is a strong electrolyte ionsing as, AgNO3 (s) ⎯⎯, → Ag + (aq) + NO 3− (aq), , and for AgI the solubility equilibrium is, ZZX Ag + (aq) + I- (aq), Agl(s) YZZ, If we take the solubility of AgI to be ‘s’ mol dm−3, then the total concentration of, Ag+ ions in the solution would be [ 0.1 + s ] mol dm−3 ~ [0.1] mol dm−3 because, the value of ‘s’ is very small. And the concentration of I− ions would be ‘s’ mol, dm−3., Substituting in the expression for Ksp = [Ag+] [I−] ; we get, [0.1] mol dm−3 ] [‘s’ mol dm−3 ] = 0.1 s mol2 dm−6 = 8.5 × 10−17 mol2 dm−6, , CHEMISTRY, , 347
Page 377 :
MODULE - 5, Chemical Dynamics, , Ionic Equilibrium, , This gives , solubility (s) = [8.5 × 10−17] / [0.1] mol dm−3, = 8.5 × 10−16 mol dm−3., (The value of ‘s’ is clearly negligible in comparison with 0.10 and thus justifies, our assumption), , Notes, , The solubility of AgI in 0.1M AgNO3 is therefore 8.5 × 10−16 mol dm−3 at 298 K., Compare this value with the solubility of AgI in water as determined in the previous, example, Solvent, , Water, , 0.1M AgNO3, , Solubility, , 9.2 × 10−9 mol dm−3, , 8.5 × 10−16 mol dm−3., , Thus we see that the solubility of a sparingly soluble salt is decreased in the, presence of another salt that has common ion. This decrease in solubility is an, example of the Common Ion Effect., , INTEXT QUESTIONS 12.3, 1. Calculate the pH of a solution containing 0.05 M benzoic acid and 0.025, M sodium benzoate. Benzoic acid has a pKa of 4.2., 2. Calculate the solubility product for Ag2SO4 if [SO42-] = 2.5 ´ 10-2 M., 12.8.3 Application of Solubility Product in Qualitative Analysis, The qualitative analysis of cations is carried out by dividing them into several, groups. This group separation of cations is based upon selective precipitation, of some cations out of many that are present in a solution. This is achieved by, adjusting the solution conditions in such a way that the Ksp of specific salts of, some cations is exceeded and they precipitate out. The remaining cations remain, in the solution. A typical example is the use of H2S. The dissociation of H2S can, be written as, ZZX 2H + (aq) + S2- (aq), H 2S(aq) YZZ, , Since the equilibrium involves hydrogen ions, the acidity of the solution would, play an important role in controlling the concentration of sulphide ions., You are aware, that in the separation of group II sulphides, the medium of the, solution is kept acidic. In this medium the concentration of the S2− ions is very, small and only the sulphides of group II are precipitated. On the other hand in the, alkaline medium the concentration of sulphide ions is fairly high and the sulphides, of group IV cations precipitate out., , 348, , CHEMISTRY
Page 378 :
Ionic Equilibrium, , MODULE - 5, Chemical Dynamics, , WHAT YOU HAVE LEARNT, z, , Electrolytes are compounds, which, when dissolved in water, produce ions., , z, , Strong electrolytes are almost completely dissociated while weak electrolytes, are only partially dissociated in their solutions., , z, , There are three different concepts of acids and bases proposed by Arrhenius,, Brønsted and Lowry and Lewis respectively., , z, , According to Arrhenius Concept an acid is a substance capable of producing, hydrogen ions by dissociating in aqueous solution while a base is a substance, capable of providing a hydroxyl ion. The neutralization reaction is basically, the reaction between a proton and a hydroxyl ion to give a molecule of water., , z, , Since a hydrogen ion H+ is very small with high charge density it does not, exist free in a polar solvent like water. It binds itself to a water molecule and, form a hydronium ion (H3O+)., , z, , According to Brønsted and Lowry, an acid is defined as a proton (H+) donor,, and a base is defined as a proton acceptor. An acid-base reactions can be, thought of as a proton-transfer from an acid to a base. In this concept, acids, and bases can be either ions or molecular substances., , z, , According to Brønsted and Lowry definition the species in either side of, the acid-base equilibrium, differ by the gain or loss of a proton. These are, called a conjugate acid-base pair. In such a pair a strong acid has a weak, conjugate base while a weak acid has a strong conjugate base., , z, , Lewis definition is quite broad, according to him, an acid is defined as,‘any, atom, molecule or ion that can accept an electron pair from any other atom,, molecule or ion, while a base is ‘any atom, molecule or ion that can donate, a pair of electron’. The product of a reaction between an acid and a base is, called an adduct., , z, , Strong Arrhenius acids and bases dissociate completely in aqueous solutions, where as the weak acids and bases undergo partial ionisation. Higher the, extent of ionisation stronger the acid or the base., , z, , In Brønsted- Lowry concept, the relative strength of an acid is defined as, its relative tendency to lose/donate a proton to water., , z, , The ionisation equilibria of weak acids and bases are characterized by, equilibrium constants called ionisation constants. The values of these, constants is a measure of their relative strength., , z, , Water can act both as a weak acid as well a weak base. In a sample of water, a small number of water molecules undergo autoionisation, in which half the, ions act as an acid while the other half acts as a base., , CHEMISTRY, , Notes, , 349
Page 379 :
MODULE - 5, Chemical Dynamics, , Ionic Equilibrium, z, , In aqueous solutions the concentration of H3O+ can be expressed in terms, of a logarithmic scale called pH scale. The pH of a solution is defined as pH, = –log10[H+] or pH = –log10[H3O+]., , z, , A neutral solution has a pH of 7 ; any solution with a pH less than 7 is acidic, while the ones with a pH of greater than 7 are basic in nature., , z, , The presence of common ions in a solution of a weak acid or a weak base, suppress its dissociation. Such solutions act as buffer solutions which resist, a change in their pH on addition of small amount of an acid or a base. The, pH of buffer solutions depend on their composition and can be found by, using a simple equation called Henderson Hasselbalch equation., , z, , The aqueous solutions of certain salts also behave as acids or bases due to, the hydrolysis of their cation or anion or both., , z, , In an aqueous solution of a sparingly soluble salt an equilibrium exists between, the undissolved salt and the ions obtained from the dissolved salt. This is, called solubility equilibrium., , z, , The product of the concentration of the ions in the solubility equilibrium is, a constant called solubility product (Ksp) and is proportional to the solubility, of the sparingly soluble salt., , z, , The presence common ions decreases the solubility of a sparingly soluble, salt. This is called common ion effect and has widespread applications in, qualitative analysis., , Notes, , TERMINAL EXERCISE, 1., 2., , What is degree of dissociation? What are factors upon which it depends., Write the equilibrium constant expression for the following reaction., ZZX H 3O + (aq) + HCO3- (aq), H 2CO3 (aq) + H 2O(l) YZZ, , 3., , Explain why does a strong Bronsted – Lowry acid has a weak conjugate, base?, , 4., , What do you understand by the term ‘amphoteric’? Show with the help of, equations that water is amphoteric in nature., Calculate the pH of 1 × 10–3 M solution of NH4OH. The dissociation constant, of NH4OH is 1.85 × 10–5 mol dm–3., The pH of an aqueous solution of HCl is 2.301. Find out the concentration, of hydrogen ions in this solution., What is a buffer solution? What are its main constituents?, , 5., 6., 7., 8., , 350, , Solubility of lead iodide PbI2 is 1.20 × 10–3 mol dm–3 at 298K. Calculate its, solubility product constant., CHEMISTRY
Page 380 :
MODULE - 5, , Ionic Equilibrium, , Calculate the solubility of Bi2S3 in water at 298K if its Ksp = 1.0 × 10–97 mol5, dm–15., 10. Calculate the solubility of AgI in 0.10M Nal at 298 K. Ksp for AgI is 8.5 ×, 10−7 at this temperature., 9., , ANSWERS TO INTEXT QUESTIONS, , Chemical Dynamics, , Notes, , 12.1, 1., , 2., , 3., , 4., , 5., , According to Arrhenius concept an acid is defined as a substance that is, capable of producing hydrogen ion (H+) by ionisation in aqueous solution., For example, HCl and CH3COOH., Arrhenius definition has the following drawbacks:, z It is limited to only aqueous solutions and requires ionisation of the, substance., z It does not explain the acidic or basic behaviour of some substances, which lack a hydrogen (or a hydroxide) ion. For example, AlCl3 and, Na2CO3 which lack a hydroxide., In the Brnsted-Lowry concept, any molecule or ion that can accept a proton, is a base whereas in Arrhenius concept a base is the one which provides, hydroxide ions in solution., Acids, , HCl, H3O+, , Bases, , NH3, CN–, , X is a weak electrolyte and Y is a strong electrolyte., , 12.2, 1., , The ionisation of weak acid, HF, can be represented as, ZZX H 3O + (aq) + F- (aq), HF(aq) + H 2O YZZ, [H3O+ ][F− ], The expression for K a would be, K a =, [HF], , 2., , For a weak base BOH which partially dissociates in aqueous solutions, and, has a degree of dissociation as α we can write, ZZX BH + + OH B + H 2 O YZZ, , Initial concentrations, , c, , Equilibrium concentrations c(1 –α), , ~55, , 0, , 0, , ~55, , cα, , cα, , The equilibrium constant expression or base dissociation constant can be, written as, CHEMISTRY, , 351
Page 381 :
MODULE - 5, , Ionic Equilibrium, , Chemical Dynamics, , K=, , [BH + ][OH − ], [cα ][cα ], =, [H 2 O][B], c [1 – α ] 55, , rearranging we get,, ⇒, Notes, , 55K = K b =, , [cα ][cα ], c 2α 2, cα 2, =, =, c [1– α ] c [1 – α ] (1 – α ), , Since the acid B is very weak, α <<1; we can neglected in comparison to 1, in the denominator to get, K b ≈ cα 2, , 3., , or, , α2 =, , Kb, c, , or, , α=, , Kb, c, , Given hydronium ion concentration, [H3O+] = 6.3 × 10–2M, As per definition pH = –log [H3O+], ⇒ pH = – log 6.3 × 10–2, ⇒ pH = –(0.7993 – 2.0000), ⇒ pH = –(–1.2007) = 1.2007, , 4., , Given: Concentration of glycine = 1.0M, Ka = 1.67 × 10–10., For a weak acid α =, ⇒, , Ka, =α=, c, , –5, 1.67 × 10 −10 = 1.29 × 10, , [H3O+] = 1 × 1.29 × 10–5 = 1.29 × 10–5M, , pH = –log [H3O+] = –log [1.29 × 10–5] = – (–4.8894) = 4.8894, 12.3, 1., , Here, [Acid] = 0.05M and [Salt] = 0.025M; and pKa = 4.2, Substituting the values in Handerson equation, we get, pH = 4.2 + log (0.05/0.025) = 4.2 + log 2 = 4.2 + 0.3010 = 4.5010, , 2., , Let the solubility of Ag2SO4 be ‘s’ mol dm–3, The concentrations of the Ag+ and the SO4–2 would be ‘2s’ mol dm–3 and ‘s’, mol dm–3 respectively, and Ksp = [Ag+]2 [SO4–2], Given [SO4–] = 2.5 × 10–2M ⇒ [Ag+] = 2 × 2.5 × 10–2M = 5 × 10–2M, Substituting the values in the expression of Ksp we get,, Ksp = [5 × 10–2]2 × [2.5 × 10–2] = 6.25 × 10–5 mol3 dm–9, , 352, , CHEMISTRY
Page 382 :
MODULE - 5, , Electrochemistry, , Chemical Dynamics, , 13, Notes, , ELECTROCHEMISTRY, , Electrochemistry deals with the conversion of electrical energy into chemical, energy and vice versa. When electric current is passed through an aqueous solution, of certain substances or through molten salts, it causes a chemical reaction to, occur. On the other hand, in dry cells, button cells or lead acid batteries chemical, reactions occur which produce electrical energy. In this lesson you will study, some aspects of these processes., , OBJECTIVES, After reading this lesson you will be able to:, z understand oxidation and reduction in terms of electron transfer concept;, z calculate oxidation number (ON) of an atom in a molecule or ion;, z balance the chemical equation for redox reaction;, z explain electrolytic conduction, conductance and molar conductivity;, z describe the effect of dilution on conductivity and molar conductivity of an, electrolyte;, z differentiate between electrolytic and Galvanic cell;, z state Faraday's laws of electrolysis;, z predict and justify the products of electrolysis of some common electrolytes;, z state standard electrode potential and use it for calculation of standard, electrode potential of a cell;, z explain standard Hydrogen electrode;, z describe electrochemical series and its application;, z state effect of concentration on electrode potential (Nernst equation);, z solve numericals based on Nernst equation and, z find relationship between emf and Gibbs energy change., CHEMISTRY, , 353
Page 383 :
MODULE - 5, , Electrochemistry, , Chemical Dynamics, , 13.1 OXIDATION AND REDUCTION AS ELECTRON, TRANSFER PROCESS, , Notes, , Oxidation and reduction reactions constitute a very important class of chemical, reaction. The electronic concept looks at oxidation and reduction in terms of, electron transfer : process in which an atom or ion looses one or more electron to, the other is called oxidation and the process in which an atom or ion gains one or, more electron is termed as reduction. In the formation of NaCl from Na and Cl, Na → Na+ + e–, , (loss of e– by Na; oxidation), , Cl + e– → Cl–, , (gain of e– by Cl; reduction), , Sodium undergoes oxidation and chlorine undergoes reduction. Here, sodium, helps chlorine to undergo reduction and therefore it is called a reducing agent or, reductant., A reductant is a species in a chemical reaction which looses its electron to another, reactant. Chlorine, on the other hand accepts electron, therefore it is an oxidising, agent or oxidant. An oxidant is a species which accepts electrons in a chemical, reaction., It may be noted that oxidation and reduction processes do not take place, independently but occur simultaneously and are thus called oxidation-reduction, reaction or redox reactions. A redox reaction is a sum of oxidation and reduction, half reactions in a chemical reaction., , 13.2 OXIDATION NUMBER, It is easy to identify species undergoing oxidation or reduction in simple molecules., However, in polyatomic molecules, it is difficult to do the same. In the example, of NaCl taken earlier it was easy to identify as sodium undergoing oxidation and, chlorine undergoing reduction but in the reaction involving ferrous sulphate with, potassium permanganate (KMnO4) it is difficult. Therefore, a new term called, Oxidation number has been introduced, Oxidation number is the apparent, charge which an atom appears to have when each pair of electrons is counted, with more elecronegative atom. Oxidation number is always assigned to an atom., It is a number written with +ve or – ve sign. The number indicates the number of, electrons that has been shifted from an atom towards a more electro-negative, atom, in a hetronuclear covalent bond. The +ve sign for the atom shifting its, electron away from itself and –ve is given to more electro –ve atom. The concept, of Oxidation Number is based on the assumption that in a polyatomic covalent, bonding, shared pair of electrons belongs to more electro–ve atom. Oxidation, state (OS) is also used for Oxidation Number., 354, , CHEMISTRY
Page 384 :
MODULE - 5, , Electrochemistry, , 13.2.1 Rules for Assigning Oxidation Number, , Chemical Dynamics, , There are certain rules that are followed for computing the oxidation number of, an atom in a molecule or ion., 1., , Oxidation number is taken as zero if atoms are present in elemental form., For example, O2, Na, P4 are elemental forms. They have oxidation number, zero., , 2., , The oxidation number of a monatomic ion is the same as the charge present, on it. For example, Na+, Mg2+, Al3+, Cl–, S2– will have oxidation no +1, +2,, +3, –1, –2 respectively., , 3., , The oxidation number of oxygen is –2 in almost all the compounds except, (a) in peroxides e.g. Na2O2, H2O2 where oxidation number is –1 and (b), , Notes, , 1, 2, , super oxides (KO2) where it is – ., 4., , The oxidation number of H is +1 when combined with non-metal and is –1, when combined with metal e.g. in HCl the O.N. of H is +1 but in Ca H2 it is, –1., , 5., , The Oxidation Number of alkali metal is +1 in its compounds., , 6., , In a compound made up of different elements the more electro negative, element will have negative oxidation number and less electro negative atoms, will have positive oxidation number e.g. in N Cl3 , N has +3 oxidation number, and Cl has –1 oxidation number., , 7., , The sum of the oxidation numbers of all the atoms in a neutral compound is, zero., , 8., , In a polyatomic ion, the sum of the oxidation numbers of all the atoms is, equal to the charge on the ion. e.g. in CO32− , the sum of oxidation Number, of carbon and oxygen is –2., , Let us illustrate the above rules taking few examples. The oxidation number of S,, N and Cl atoms in : (a) H2SO4 (b)NO–3 (c) ClO–4 respectively will be calculated as, (a), , 1. Let the oxidation number of sulphur be x., 2. Since the oxidation number of O is –2. Therefore the sum of four O, atoms is equal to –8., 3. The oxidation number of each H is +1 as bonded to a non-metal so two, H atoms have total oxidation number of +2., 4. H2SO4 is a neutral molecule. Therefore the sum of all the oxidation, numbers is equal to zero. Thus, +2 + x – 8 = 0, x=+6, Therefore oxidation number of sulphur in H2SO4 is + 6., , CHEMISTRY, , 355
Page 385 :
MODULE - 5, Chemical Dynamics, , Electrochemistry, , (b), , NO3–, , first assign –2 oxidation number to each O atom. Here the, sum of the oxidation number of all the atoms will equal to, charge present on the ion., , ∴, , x – 6 = –1, x = +5, oxidation number of N is +5., , Notes, (c), , In ClO 4–, , x – 8 = –1, x = +7, , 13.3 BALANCING REDOX REACTION, The redox reaction can be balanced by any of the following methods :, (a) Oxidation number method., (b) Ion electron method., 13.3.1 Balancing by Oxidation Number method, The steps involved in balancing redox reactions by this method are as follows:, , 356, , 1., , Write the skeletal equation of reaction i.e. chemical equation without the, stoichiometric coefficient., , 2., , Write the oxidation number of each atom above its symbol in the equation., , 3., , Identify the atoms undergoing change in oxidation number., , 4., , Calculate the increase or decrease in oxidation number per atom for the, atom undergoing a change in oxidation number. If more than one atom is, involved, multiply the increase or decrease in number with the number of, atoms undergoing the change to determine the total change in oxidation, number., , 5., , Equate the increase and decrease in oxidation number on the reactant side, by multiplying the formulae of the oxidising and reducing agents suitably., , 6., , Balance the equation with respect to all the atoms except hydrogen and, oxygen., , 7., , Finally balance H and O also., , 8., , If the reaction is taking place in acidic medium balance the O atoms by, adding required number of H2O molecule on the side where O atoms are, less in number. Balance the H atoms by adding H+ to the side deficient in H, atoms., , CHEMISTRY
Page 386 :
MODULE - 5, , Electrochemistry, , 9., , In the basic medium by add required number of negative charges by adding, required number of OH– ions to the side deficient in the magnitude of charges,, then add H2O molecules to balance OH– ions., , Chemical Dynamics, , For example : When Phosphorus is treated with nitric acid, nitric oxide is formed., 1., , The skeletal equation is, P + HNO3, , 2., , HPO3 + NO + H2O, , Notes, , Write the oxidation number of each atom on the given skeletal equation, 0, , +1 + 5 –2, , +1 + 5 –2, , +2 – 2, , +1 – 2, , P + HNO3 ⎯⎯, → HPO3 + NO + H 2 O, , 3., , P and N are undergoing change in Oxidation Number., , 0, , +5, , P + HNO 3, , increase in ON = 5, , +5, , +2, , HPO3 + NO + H2O, , decrease in ON = 3, , 4., , Equating the increase and decrease in Oxidation Number of P and N on the, reactant side, 3P + 5HNO3, , 5., , Balance the P and N atoms on both sides of the equation, 3P + 5HNO3, , 6., , HPO3 + NO + H2O, , 3HPO3 + 5NO + H2O, , O and H are already balanced in the equation., , 13.3.2 Balancing by Ion Electron Method, This method is based on the principle that electrons lost during oxidation half, reaction is equal to the electrons gained in the reduction half reaction. The steps, involved are, 1., , Write the skeleton equation., , 2., , Write the oxidation number of all the atoms above their symbols in the skeletal, equation., , 3., , Find the atoms undergoing change in Oxidation Number. Thus find out the, species getting oxidised and reduced respectively., , 4., , Split the whole (net) equation into two half reactions i.e. oxidation half, reaction and reduction half reaction., , CHEMISTRY, , 357
Page 387 :
MODULE - 5, Chemical Dynamics, , Electrochemistry, , 5., , Balance the atoms, undergoing change in oxidation number in each half, reaction., , 6., , Calculate the total change in oxidation number in each half reaction which is, equal to total number of electron transfer., , 7., , Add total number of electron transfer as calculated above on the reactant, side in reduction half and on the right hand side on the oxidation half reaction., , 8., , Balance the charges by adding H+ (for reactions in acidic medium) or OH–, (reactions basic medium) either on left or right of equation., , 9., , Finally balance H and O by adding H2O on the required side of the reaction., , Notes, , 10. Add the two half reactions such that total number of electrons cancel out on, both sides. To do so half reactions may be required to multiplied by some, numbers to make the number of electrons equal on both sides., 13.3.3 Example of Balancing, Example 13.1 : Balance the following skeletal reaction by ion electron method, Cr2 O 72– + Fe2+, , Cr3+ + Fe3+ in acid medium, , Refering to the rules given :, Step I and II, Write the oxidation number of the atoms above their symbol in the skeletal equation, +2, , +6, , +3, , +3, , Cr2 O 72 − + Fe 2+ ⎯⎯, → Cr 3+ + Fe3+, , Step III : Oxidation number of Fe2+ is increasing; therefore it is undergoing, oxidation and oxidation number of Cr is decreasing so it is undergoing reduction., Step IV : Split the reactions in two half reactions, (a) Reduction half reaction, Cr2 O 72–, , Cr3+, , (b) Oxidation half reaction, Fe2+, , Fe3+, , Balance the first reduction half reaction, Cr2 O 72–, , Cr3+, , Step V : Balance the atoms undergoing change in Oxidation Number., Cr2 O 72–, , 358, , 2Cr3+, , CHEMISTRY
Page 389 :
MODULE - 5, Chemical Dynamics, , Electrochemistry, , 4. Write the half reaction for the following, , 2I(−aq ) + 2Fe(3aq+ ) ⎯⎯, → I2 ( s) + 2Fe2+ (aq), Mg ( s) + CI2 ( g ) ⎯⎯, → MgCI 2 ( s), , → HIO3 + NO2 + H2O, I2 + HNO3 ⎯⎯, Notes, , 5. Balance the equation by oxidation number method, → Cu + N2 + H2O, CuO + NH3 ⎯⎯, → MnCl2 + C2 + H2O, MnO4 + HCl ⎯⎯, 6. Balance the following by ion eelctro half reaction method, → Bi3+ + NO2, N O 3− + Bi ⎯⎯, , acidic medium, , → Mn2+ + Fe3+, MnO4 + Fe2+ ⎯⎯, , acidic medium, , → Fe3 + Cr3+, Cr2 O 72 − + Fe2+ ⎯⎯, , acidic medium, , Al + NO3− ⎯⎯, → Al (OH) 4− + NH 3, , basic medium, , 13.4 ELECTROLYTIC CONDUCTION, When electricity is passed through an aqueous solution, it may or may not conduct, current. The chemical substances whose aqueous solutions conduct electricity, are called electrolytes and those which do not conduct current are called as nonelectrolytes. This phenomenon of conduction of current through a solution is, called electrolytic conduction., Electrolytic conduction takes place due to the movement of cations and anions in, a solution. The electrical conductance of a solution, depends upon (a) nature of, solute (b) valency of its ion, (c) the concentration in solution and (d) the, temperature. In this section we will learn about various ways of expressing the, conductance of electrolytes and the factors affecting them., 13.4.1 Conductance and Conductivity, Like solid conductors, electrolytic solutions also obey Ohm’s Law. When a current, of I amperes flows through a solution which offers a resistance of R ohms and a, potential difference of V volts is applied, then according to ohm’s law, V=I.R, , 360, , CHEMISTRY
Page 390 :
MODULE - 5, , Electrochemistry, , If the solution is taken in a conductivity cell which has two parallel electrodes l, cm apart and each having an area of cross section A cm2, the resistance R of the, electrolyte is found to be directly proportional to l and inversely proportional to, A i.e., R∝, , l, A, , R=ρ., , or, , Chemical Dynamics, , l, A, , Notes, ....(i), , Where ρ “rho” is a constant of proportionality and is called specific resistance or, resistivity. It is characteristic of the nature of electrolyte, its concentration and, temperature., In case of solutions, it is preferred to discuss their conductance and conductivity, rather than their resistance and specific resistance. The conductance is reciprocal, of resistance and the conductivity is reciprocal of specific resistance., Conductance is denoted by L and is measured in the unit of ohm–1 which has now, been named as siemens, S. The conductivity is denoted by k “kappa”. Thus by, definition, L=, , 1, 1, and k = ρ, R, , ...(ii), , The units of k can be worked out from relation (i) as under :, The inverse of (i) is,, 1 A, 1, = ρ ., R, l, , or, , L=k, , A, l, , and, , k=L, , l, A, , =S, , cm, cm 2, , = S cm–1, The conductivity (K) is expressed in S cm–1 or 100 S m–1., 13.4.2 Measurement of Conductance, The conductance of an electolyte is measured with the help of a conductivity cell., Conductivity cell is a device which has two parallel platinum electrodes coated, with platinum black., , CHEMISTRY, , 361
Page 391 :
MODULE - 5, Chemical Dynamics, , Electrochemistry, , is Scm–1. In the expression for conductivity,, , Notes, , Connecting, wires, , The SI unit of length is metre, hence SI unit of, conductivity (K) is Sm–1, but the commonly used unit, l, is a, A, , constant. Here l represents the distance between the, two parallel electrodes and A represents the area of, cross section of the electrodes. Thus for a given, Platinized Pt, Electrodes, , l, is a constant called cell constant., conductivity cell,, A, , K (conductivity) = conductance × cell constant, The conductivities of some substances are given in the, table., , Fig. 13.1 : Condivity Cell, , Table 13.1 : The values of conductivity of some selected substances at 298 K, Substance, , k/S cm–1, , Substance, , k/S cm–1, , Pure water, , 6.0 × 10–8, , Silver metal, , 6.1 × 105, , 0.1 M HCl, , 3.5 × 10–2, , Mercury metal, , 1.0 × 104, , 0.1M NaCl, , 9.2 × 10–3, , Glass, , 1.0 × 10–14, , 0.1M CH3COOH, , 4.7 × 10–4, , 0.1M NH4OH, , 3.4 × 10–4, , We find from the table that the conductivities of metals are very high and that of, pure water and glass very low., 13.4.3 Molar Conductivity, The electrolytic conductivity of a solution depends on the concentration of the, electrolyte in the solution. Therefore, the conductivity of an electrolyte is normally, expressed as molar conductivity., Molar conductivity is the conducting power of all the ions furnished by one, mole of an electrolyte in a solution of specified concentration., It is denoted by λm and is related to K by the relation., λm =, , 1000 K, M, , .....(vi), , Where M is the molarity of the solution. Its units are S cm2 mol–1., , 13.5 FACTORS AFFECTING CONDUCTIVITY, As mentioned the conductivity of an electrolyte depends upon the following aspects, of the electrolyte., 362, , CHEMISTRY
Page 392 :
MODULE - 5, , Electrochemistry, , (a) Nature of Electrolyte : Conductivity of an electrolyte depends upon the, nature of electrolyte on the following points :, , Chemical Dynamics, , (i) Weak or strong electrolyte : A weak electrolyte furnishes fewer ions, therefore it has lower conductivity than a strong electrolyte of same, concentration., (ii) Valency of the ions : The ions with higher valency carry more charge, and therefore they conduct more charge than the ion of lower valency., Thus higher the valency of the ion greater is the conducting power., , Notes, , (iii) Speed of the ion : The ion which can move faster will carry the charge, also faster and therefore has more conducting power., (b) Temperature : Conductivity of an electrolyte generally increases by 2–3, percent for each degree rise in temperature. With increase in temperature, the viscosity of the solvent decreases and thus ion can move faster. In case, of weak electrolyte, when the temperature is increased its degree of, dissociation increases, thus conductivity increases., (c) Concentration :, (i) Variation of conductvity (k) with concentration. When the solution, is diluted its conductivity also decreases. It is because k is the conducting, power of all the ions present per cm3 of the solution. When the solution, is diluted the number of ions per cm3 also decreases, hence k decreases., , KOH, k, CH3COOH, C, Fig. 13.2 : Variation of conductivity with concentration, , (ii) Variation of Molar and Equivalent conductivity with, concentration: As the solution is diluted its molar conductivity, increases. Λm is given as, λm =, , 1000k, M, , where k is conductivity and M is molar concentration., , CHEMISTRY, , 363
Page 393 :
MODULE - 5, Chemical Dynamics, , Electrochemistry, , This increase in λm is a resultant of two factors. On decreasing the concentration, both k and M decreases. Of the two (k) tries to decrease λm while the other, factor (M) tries to increase it. Since the decrease in M is much more, the net, result is that λm increases. However, strong and weak electrolyte as show different, type of behaviour on dilution (Fig. 13.3), , Notes, KCl (strong electrolyte), lm, CH3COOH (weak electrolyte), C, , Fig. 13.3 : Variation of molar conductivity with concentration, , From the Fig. 13.3 we find that the increase in molar conductivity for a strong, electrolyte like KCl is very gradual on dilution and also the value is high at all, concentrations. Whereas for a weak electrolyte like CH3COOH, there is a gradual, increase in λm on dilution which is followed by a sharper increase on further, dilution. These observations can be explained as : since KCl is a strong electrolyte,, it is fully dissociated at all concentrations. In concentrated solution, attraction, between opposite ions is large and their conducting ability is less. On dilution, inter-ionic forces decrease and ions can move faster leading to the increase in, molar conductivity., On the other hand, in weak electrolytes, molar conductivity is low in concentrated, solution. This is due to partial dissociation (ionisation) of weak electrolytes. On, diluting the solution the degree of ionisation increases, which increases the number, of ions. This leads to a sharp increase in molar conductivity in weak electrolytes., 13.5.1 Kohlrausch’s Law, Kohlrausch determined the molar conductivity at infinite dilution for a large number, of strong electrolytes. On the basis of his observations he concluded that at infinite, dilution, each ion makes a definite contribution to the total molar conductivity of, an electrolyte. This individual contribution is called molar ionic conductivity. He, generalised his observations as, “At infinite dilution each ion of the electrolyte makes a definite contribution, towards conductivity of the electrolyte and it is independent of the presence of, other ions of the electrolyte.” This is called Kohlrausch’s Law of independent, migration of ions., 364, , CHEMISTRY
Page 394 :
MODULE - 5, , Electrochemistry, , For a salt like KCl, molar conductivity at infinite dilution can be written as, , Chemical Dynamics, , λ m∞ KCl = λ m∞ K+ + λ m∞ Cl–, In general for a salt of formula AxBy the molar conductivity at infinite dilution is, written as, , λ m∞ (AxBy) = x λ m∞ (Ay+) + y λ m∞ (Bx–), ∞ indicates molar conductivity at infinite dilution. This law is used to, where λ m, calculate the molar conductivity at infinite dilution for weak electrolytes whose, , Notes, , λ m∞ can not be obtained graphically., Example 13.2 : Λ 0 for NaCl, HCl and CH3COONa are 126.0, 426.0 and 91.0 S, cm2 mol–1 respectively. Calculate Λ 0 for CH3COOH., Solution Λ 0 CH3COOH = λ 0 (H + ) + λ 0 (CH3COO − ), = λ 0 (H + ) + λ 0 (Cl − ) + λ 0 (Na + ) + λ 0 (CH3COO − ) − λ 0 (Na + ) − λ 0, = 426.0 + 91.0 – 126.0 = 391.0 S cm2 mol–1., , INTEXT QUESTION 13.2, 1. How does the solution of electrolytes conduct electricity?, 2. Define conductivity and molar conductivity., 3. Give the units of conductance and conductivity., 4. List factors affecting the conductance of an electrolyte., 5. Draw a graph showing variation in molar conductivity of weak and strong, electrolytes., 6. Write the expression for molar conductivity at infinite dilution for Al2(SO4)3., , 13.6 ELECTRO CHEMICAL CELLS, An electrochemical cell is a device used for the interconversion of electrical and, chemical energy. An electrochemical cell contains two electrodes (cathode and, anode) and an electrolyte., These are of two types; based on the nature of conversion of energies, (a) Electrolytic cell (Faradaic cell), In electrochemical cells, a battery is used to carry out redox reactions on, the electrodes of the cell. Thus, these cells convert electrical energy into, chemical energy., , CHEMISTRY, , 365
Page 395 :
MODULE - 5, Chemical Dynamics, , Electrochemistry, , (b) Galvanic cell (Voltaic cell), In galvanic cells an emf is developed as a result of radox reaction occuring, on the electrodes. These cells convert chemical energy into electrical energy., , 13.7 ELECTROLYTIC CELLS OR RARADAIC CELLS, Notes, , An electrolytic cell consists of two electrodes connected to a battery as shown in, Fig 13.4., Anode, , Cathode, –, , +, , –, , +, , –, , +, , fused NaCl, , Fig. 13.4 : Electrolytic cell, , In an electrolytic cell electrical energy is converted into chemical energy. The, process of decomposition of an electrolyte into its ions when an electric current, is passed through it, is called electrolysis., When electricity is passed through an electrolyte, a chemical change i.e., decomposition of the electrolyte into ions takes place at the electrode. Oxidation, and reduction reactions occur in the cell., In the electrical field Cl– ions migrate to the +ve electrode (anode) and undergo, oxidation by loosing electrons. Na+ ions will go to –ve electrode (cathode) and, undergo reduction., The process can be represented as :, oxidation at anode, , and reduction at cathode,, , Cl–, , Cl + e–, , Cl + Cl, , Cl2 (g), , Na+ + e–, , Na., , 13.7.1 Faraday’s Laws of Electrolysis, Michael Faraday studied the quantitative aspects of electrolysis and expressed, the results of his study in the form of the following two laws which are known, as the Faraday’s laws of electrolysis., , 366, , CHEMISTRY
Page 396 :
MODULE - 5, , Electrochemistry, , Chemical Dynamics, , (I) Faraday’s First Law of Electrolysis, It states that the mass of a substance liberated during electrolysis is directly, proportional to the quantity of electricity passed through the electrolyte taken, as a solution or as melt., Mathematically, if w is the mass of a substance liberated or discharged on passing, Q coulomb of electricity, , Notes, , w∝Q, w = zQ, , and, , (1), , where z, the constant of proportionality is called electrochemical equivalent, of the substance, The quantity of electricity passed, Q, is related to the strength of the current, passed in amperes I, and the time in seconds, t., Q = I.t, ∴, , w = zIt, , (2), , Electrochemical equivalent, z, of a substance is its mass liberated or deposited, on passing 1 coulomb (Q = 1) charge or one ampere (I = 1) current for one, second (t = 1)., (II) Faraday’s Second Law of Electrolysis, This law relates the masses of different substances liberated or discharged by, passing the same amount of electricity. It states that when the same quantity, of electricity is passed through different electrolytes (taken in different electrolytic, cells which are connected in series) the masses of different substances liberated, are proportional to their chemical equivalent masses. Equivalent mass of any, substance undergoing a redox reaction (oxidation or reduction) is related to its, molar mass as., Equivalent mass =, , Molar mass, Number of electrons last or gained, , Let wA and wB be the masses of two substances liberated in two electrolytic, cells connected in series, then:, wA Equivalent mass of A, =, ., wB Equivalent mass of B, , CHEMISTRY, , 367
Page 397 :
MODULE - 5, Chemical Dynamics, , Electrochemistry, , Example 13.3: What is the mass of silver deposited when 500 coulomb, electricity is passed through a solution of AgNO3? (Atomic mass of Ag =, 108 u), Solution:, Silver is deposited on cathode according the reaction, , Notes, , Ag + (aq) + e− → Ag(s), 1 mol, , 1F, , 1 mol, , 96500 C, , 108 g, , Thus, 1 mole of electrons (1 Faraday) or 96500 coulombs of electricity are, required to produce 108 g of silver, 96500 C produce 108 g of Ag, 500 C would produce, , 108 × 500, = 0.56g of Ag, 96500, , Example 13.4: On passing electric current for some time, it was found that 32.4, g Ag had deposited on cathode in a cell filled with AgNO3. How much would, a copper cathode of another electrolytic cell containg CuSO4 solution weigh, after the electrolysis if it was connected in series to the AgNO3 cell? The mass, of the copper cathode before electrolysis was 27.33 g, Equivalent masses:, Ag = 108 g; Cu = 31.75 g, Solution:, According to the Faraday’s second law of electrolysis, Mass of copper deposited Equivalent mass of copper, =, ., Mass of silver deposited, Equivalent mass of silver, Mass of copper deposited 31.75 g, =, 32.4 g, 108 g, Mass of copper deposited =, , 31.75 g × 32.4 g, = 9.53 g, 108 g, , Mass of copper cathode before electrolysis = 27.33g, Mass of copper cathode after electrolysis = 27.33 + 9.53, = 36.86 g, 368, , CHEMISTRY
Page 398 :
MODULE - 5, , Electrochemistry, , Chemical Dynamics, , 13.7.2 Products of Electrolysis, During electrolysis, cations are reduced at cathode and anions are oxidized at, anode and a chemical reaction occurs in a non-spontaneous direction. The, minimum potential required for any electrode process to occur is called its, discharge potential or decomposition potential. Discharge potential is equal, to the electrode potential for cathodic (reduction) process and equal in, magnitude but opposite in sign to the electrode potential for anodic (oxidation), process. At any electrode that process (oxidation or reduction) would occur, which is the easiest. The reduction of that ion would occur which has highest, reduction potential and the oxidation of that ion would occur which has the, smallest reduction potentital in highest oxidation potential., , Notes, , In many cases, the actual discharge potential exceeds the expected value. The, difference between the two is known as over potential. Over potential is, commonly observed when gaseous products are formed and depends upon the, nature of cathode. Over potential for the discharge of H2 gas is zero on a, platinum cathode but it is 1.5 V on mercury cathode. Due to over potential the, process which occur may not be the one which otherwise is the easiest. Let us, take some, examples now., 1. Electrolysis of molten NaCl (Pt electrodes)., Molten NaCl furnishes its own cations and anions, , NaCl(l) → Na + + Cl−, So only one process is possible at anode and cathode, Anodic process (oxidation): 2Cl− → Cl2 (g) + 2e −, Cathodic process (reduction): 2Na + + 2e− → 2Na(l), 2. Electrolysis of aqueous NaCl (Pt electrodes), Dissolved NaCl ionizes in its solution as, , NaCl(aq) → Na + (aq) + Cl− (aq), At Cathode, At cathode it is not only Na+ ions that can be reduced, but H2O also can be, reduced. The two possible reduction processes are:, (i) Na + + e− → Na, , E ° = −2.71 V, , (ii) 2H 2O + 2e− → H 2 (g) + 2OH −, , E ° = −0.83 V, , Out of these, the reduction potential of water is much greater than that of Na+., Therefore only H2 gas is evolved at cathode. (However, if mercury cathode, , CHEMISTRY, , 369
Page 399 :
MODULE - 5, Chemical Dynamics, , Electrochemistry, , is used, due to large over potential of discharge of H2, Na+ ions are reduced, and Na is produced which reacts with water to produce NaOH and H2. It is, used for manufacture of NaOH), At anode, At anode, Cl– ions and H2O both can get oxidized:, , Notes, , (iii) 2Cl − → Cl 2 (g) + 2e −, , E° = −1.36 V, , (iv) 2H 2O → O 2 (g) + 4H + + 4e −, , E° = −1.23 V, , Oxidation potential of water is slightly greater than that of Cl– so its oxidation, should be easier. But due to over potential for discharge of O2, it becomes more, difficult and Cl2 gas is evolved at anode. Thus the electrolysis of aqueous NaCl, occurs as:, Anodic process (oxidation): 2Cl− → Cl2 (g) + 2e −, Cathodic process (reduction): 2H 2O + 2e − → H 2 (g) + 2OH− (aq), H2 is evolved at cathode and Cl2 at anode., 3. Electrolysis of aqueous CuSO4 (Pt electrodes), CuSO4 ionizes in its aqueous solution as, CuSO4 (aq) → Cu 2+ (aq) + SO 42− (aq), At cathode, copper is deposited because reduction of Cu2+ is easier than that, of water and at anode O2 is evolved as oxidation of water is easier than that, of SO 24− ions., , +, + 4e−, Anodic Process (oxidation): 2H 2O → O2 (g) + 4H (aq), , Cathodic process (reduction): Cu 2+ + 2e− → Cu(s), Thus copper is discharged at cathode and O2(g) at anode., 4. Electrolysis of aqueous CuSO4 (Cu electrodes), When copper electrodes are used, oxidation of Cu (from electrode) is easier, than oxidation of H2O or SO 24− ions. However at cathode, reduction of Cu2+, ions occurs as the reduction of water is more difficult even on copper electrode, Anodic process (oxidation): Cu(s) → Cu 2+ (aq) + 2e−, Cathodic process (reduction): Cu 2+ (aq) + 2e− → Cu(s), This process is used for electrolytic refining of copper in its metallurgy., 370, , CHEMISTRY
Page 400 :
MODULE - 5, , Electrochemistry, , Chemical Dynamics, , 13.8 GALVANIC CELLS OR VOLTAIC CELLS, In such a cell chemical energy is converted into electrical energy. Dry cells, car, batteries and button cells used in wrist watches are all examples of this type of, cell. They are energy producing devices., 13.8.1 Redox Reaction and Galvanic Cell, , Notes, , You have already learnt that when electricity is passed through a solution, redox, reaction takes place. Now we shall learn how redox reaction can be used to, produce electricity., When a Zinc rod is dipped in CuSO4 solution, a reaction starts in the solution., Zn(s) + CuSO4(aq), , Zn SO4 (aq) + Cu(s), Zinc rod, , CuSO4 solution, , Fig. 13.5 : Redox reaction, , It is an example of redox reaction. The two half reactions are, Zn2+(aq) + 2e–, , Zn(s), Cu2+ (aq) + 2e–, , Cu(s), , oxidation, reduction, , In this redox reaction the electrons given by zinc rod have been directly consumed, by Cu2+ ion. But, if somehow we make the electrons given by Zinc rod to flow, through a wire to reach Cu2+ ions, we shall be producing electric current. To do, so, the reaction is carried out in the electrochemical cell as shown Fig. 13.6 :, e–, , Ammeter, , Anode (–), zinc rod, , e–, cathode (+), copper rod, , Salt Bridge, , 2–, , So4, , CuSO4, ZnSO4, , 2+, , Zn, , 2+, , Cu, , 2–, , So4, , Fig. 13.6 : Daniell cell having zinc and copper electrodes, CHEMISTRY, , 371
Page 401 :
MODULE - 5, Chemical Dynamics, , Notes, , Electrochemistry, , The redox reaction in the electro chemical cell has been modified Zinc rod is, dipped in zinc sulphate solution in one beaker while copper rod is dipped in, another beaker containing CuSO4 solution. The two solutions are connected, through a salt bridge and the two metals are connected to an ammeter with the, help of wire. We find electrons move through the wire from zinc to copper rod., A metal dipped in its own salt solution is called as half cell. Zinc rod dipped inzinc sulphate solution is oxidation half cell because oxidation takes place., Zn(s), , Zn2+(aq) + 2e–, , (oxidation), , The released electrons are taken up by zinc rod and it becomes negatively charged., Copper in copper sulphate is reduction half cell. Copper acts as cathode and, reduction take place here. Copper rod becomes positively charged. Copper gains, electrons and in this process, beomes positively charged., Cu2+(aq) + 2e, , Cu(s), , Here the electrons will move from negatively charged electrode to positively, charged copper electrode., Flow of electrons in the external circuit, The electrons released at the anode during oxidation flow through the external, circuit and reach the cathode where they are taken up for reduction. Thus in a, galvanic cell the electrons always flow from anode to cathode while the, conventional positive current flows in the opposite direction i.e. from cathode to, anode. Since the electric current always move in a closed circuit salt bridge is, used to makeelectrical contact between the two half cells., 13.8.2 Salt Bridge, A salt bridge is a inverted U tube filled with a concentrated solution of an inert, electrolyte like KCl or NH4NO3 which does not take part in the cell reaction. The, electrolyte is taken in the form of solution and mixed with agar-agar. The mixture, is heated and filled in the U tube when hot. On cooling it sets into a jelly like mass, and does not flow out, during its use. Salt bridge has two functions., (i) It completes the inner circuit. It acts as a contact between the two half cells, without any mixing of electrolytes., (ii) It prevents accumulation of charges in two half cells and maintains electrical, neutrality., Cations and anions of the salt bridge move into two half cells and neutralise the, excess charge. The anions move into oxidation half cell and neutralise the excess, charge. The cations move into the reduction half cell and neutralise the charge., In a Daniell cell a salt bridge is replaced by a porous pot, to make the cell more, handy to use., 372, , CHEMISTRY
Page 402 :
MODULE - 5, , Electrochemistry, , Chemical Dynamics, , 13.8.3 Symbolic Representation of Galvanic Cells, In the previous section the cell was a Zn-Cu cell. But any two suitable metals can, be used to make the cell and everytime we do not always draw the diagrams, showing the cell. It is represented in the symbolic form with the help of standard, notation. The rules of notations are as follows :, 1., , Anode is written on the left hand side and cathode on the right hand side., , 2., , The metal electrode in the anode half cell is written by its symbol and this is, followed by the cation (metal ion) along with its concentration in a small, bracket. The metal and cations are separated by vertical line or a semicolon, (:), , Notes, , Zn (s) | Zn2+(aq) (1M), 3., , In the reduction half cell the anion along with its concentration is written, first, then vertical line and then the metal, Cu2+(aq) (1M) | Cu(s), , 4., , A salt bridge is represented by two vertical lines., Thus the Galvanic cell described above is written as, Zn(s) | Zn2+(aq)(1M) ||Cu2+(aq)(IM) | Cu(s), or, Zn | Zn2+ (1M) || Cu2+(1M) | Cu, , 13.9 ELECTRODE POTENTIAL, Metal atoms have tendency to lose electrons and go into solution as metal ions., Electrode potential is a measure of the tendency of metal atoms to gain or loose, electrons when in contact with a solution of its own ions., When a metal strip M is immersed in a solution of its salt containing ions (Mn+),, one of the processes as showin in Fig. 13.7 (a) or (b) can occur., Metal M, , n+, , M, , n+, , M, , M, , n+, , ne, , ne, n+, , M, , M, , ne, Solution, , M, , Reduction, , M, , M, , ne, , n+, , n+, , –, , ne, , n+, , M, , M+ ne, , solution, , (a), , n+, , M+ ne, , (b), , Fig. 13.7 : Metal placed in a solution of its ions, CHEMISTRY, , 373
Page 403 :
MODULE - 5, Chemical Dynamics, , Electrochemistry, , (i) The dissolution process where atoms of metal electrode M may loose some, electrons to the electrode and enter the solution as Mn+, M → Mn+ + ne (metal is oxidised), The metal electrode gets negative charge and the solution gets extra positive, charge., , Notes, , (ii) The deposition process where metal cations Mn+ from the solution may come, in contact with the metal strip, gain some electrons and get converted into, metal atoms M, which get deposited on the surfance of metal strip. Seperation, of charges take place and a potential is developed called electrode potential., Mn+ + ne– → M (the ion is reduced), The electrode reaction reaches an equilibrium as represented below, oxidation, , n+, ZZZZZX, Z, M(S) YZZZZZ, Z M (aq) + ne, reduction, , Electrode potential is the potential developed at the interface between metal, and its salt solution; when a metal is dipped in its own salt solution., 13.9.1 Standard Electrode Potential, An electrode is said to be in the standard state if the concentration of the electrolyte, is one molar and the temperature is 298K. Then its electrode potential is called, standard electrode potential and denoted by Eº. If any gas is used to make the, electrode then the pressure of the gas should be 1 bar., , 13.10 MEASUREMENT OF ELECTRODE POTENTIAL, It is not possible to measure single electrode potential. It is because the reaction, taking place at the electrodes is oxidation or reduction and these reactions do not, take place in isolation. It can be measured with respect to a reference electrode., The electrode used as reference electrode is standard hydrogen electrode (SHE)., 13.10.1 Standard Hydrogen Electode, Standard Hydrogen Electrode (SHE) consists of a container, containing 1M HCl, solution kept at 298K. A wire containing Platinum electrode coated with platinum, black is immersed in the solution. Pure hydrogen gas is bubbled in the solution at, 1bar pressure., The potential of SHE (Eº) is taken as zero volt at all temperatures., Standard hydrogen electrode may act as anode or cathode depending upon the, nature of the other electrode. If its acts as anode, the oxidation reaction taking, place is, , 374, , CHEMISTRY
Page 404 :
MODULE - 5, , Electrochemistry, , Chemical Dynamics, , 2H+(aq) + 2e–, , H2(g), , If it acts as cathode then the reduction half reaction occuring is, 2H+(aq) + 2e–, , H2(g), , Notes, H2, (At 1 bar), , HCl (1M), Platinum coated, with Pt black, , Fig. 13.8 : Standard Hydrogen electode, , 13.10.2 Measurement of Standard Electrode Potential, (i) Determination of magnitude : The standard electrode potential of an electrode, can be measured by combining it with standard hydrogen electrode. To illustrate,, let us take the example for the measurement of standard electrode potential of, zinc electrode. A zinc strip is dipped in 1M ZnSO4 solution and it is connected to, Standard Hydrogen electrode. The cell emf is found to be 0.76 V., e, (–), , –, , e, voltmeter, , –, , (+), , Zinc Rod, , H2 (1 bar pressure), , Standard, Hydrogen, Electrode, , one molar solution, 2+, of Zn ions at 298 K, , one molar solution, +, of H at 298 K, , Fig. 13.9 : Measurement of standard electrode potential of Zn/Zn2+ electrode, , When copper electrode i.e. copper dipped in 1M CuSO4 solution is connected to, standard hydrogen electrode then the cell emf is 0.34 ., , CHEMISTRY, , 375
Page 405 :
MODULE - 5, Chemical Dynamics, , Notes, , Electrochemistry, , (ii) Sign of electrode potential, The galvanic cell formed by the combination of SHE and electrode under study,, the polarity of the electrode is determined with the help of a voltmeter. In case, the given electrode is found to be positive electrode, its electrode potential is, given the positive sign and if it is negative then it is given the negative sign. In the, case of zinc connected to SHE the polarity is negative but in case of copper it is, positive., , 13.11, , ELECTROCHEMICAL SERIES AND ITS, APPLICATIONS, , 13.11.1 Cell emf and Potential difference, The difference in potential of the two electrodes (or half cells) of a galvanic cell,, when measured in the open circuit is called the cell electromotive force or cell, emf. When it is measured in a closed circuit with some external load it is called, potential difference., Cell emf can be measured by using a potentiometer. It depends on the nature of, electrodes, concentration of electrolyte and the temperature., 13.11.2 Standard cell emf, The emf of a cell has a standard value if both its half cells are in their standard, states. It is denoted by Eº cell., 13.11.3 Cell emf and electrode potential, The standard cell emf is related to the standard electrode potentials of its anode, and cathode., E0 cell = E0 cathode – E0 anode., = EºRight – Eºleft, Cell emf is related to the electrode potentials of its anode and cathode, Ecell = Ecathode – Eanode, = Eright – Eleft, 13.11.4 Electrochemical Series, Standard potential of a large number of electrodes have been measured and they, have been listed in the increasing order of electrode potential in a series called, electro chemical series. The table 13.2 gives the standard reduction potentials of, some electrodes., , 376, , CHEMISTRY
Page 407 :
MODULE - 5, Chemical Dynamics, , Electrochemistry, , Example 13.5 : Predict the redox reaction between zinc and iron. Given E0 of, Zn2+ / Zn is – 0.763 and E0 for Fe2+ / Fe is –0.44 V., The E0 value of Zn2+/Zn is lower than Fe2+/Fe. It means Zn has a greater reducing, power than Fe or zinc can undergo oxidation more quickly than Fe. Zinc will, reduce Fe2+ ions and itself undergoes oxidation. The given reaction between Zn, and Fe will take place as shown., , Notes, , Zn + Fe2+, , Fe + Zn2+, , (ii) It helps to calculate the emf of a galvanic cell., E0cell = E0cathode – E0anode., E0 cell should always be positive. If E0 cell comes as –ve it means the cell, cannot work and electrodes should be interchanged., Example : Predict the E° for the cell, Mg | Mg2+(1M) || Ag+(1M) | Ag, From the table, 0, Ecell, = E0cathode – E0anode, , E0Mg2+/Mg = –2.365V and, E0Ag+/Ag = 0.80V, E0cell = 0.80 – (–2.365)V, = 0.80 + 2.365, = 3.165 V, (iii) It helps to predict the feasibility of a redox reaction, The feasibility of a redox reaction can be predicted by calculating E0 cell for the, redox reaction. The redox reaction is broken in two half reactions : oxidation half, reaction acts as anode and reduction half acts as cathode. The positive E0 cell, indicates the redox reaction is possible., Example 13.3 : Predict whether the following reaction is feasible or not?, Cu2+(aq) + 2Ag(s), Given, , Cu(s) + 2Ag+(aq), , E0Ag+/Ag = 0.80V and E0Cu2+/cu = 0.34V, , The given redox reaction can be written as two half reactions, Anode (Oxidation), , 2Ag(s), , Cathode (Reduction) Cu2+(aq) + 2e–, , 378, , 2Ag+(aq) + 2e–., Cu(s), , CHEMISTRY
Page 408 :
MODULE - 5, , Electrochemistry, , Chemical Dynamics, , E0cell = E0 cathode — E0 anode, = E0 Cu2+/Cu — E0Ag+/Ag, = 0.34 V – 0.80 V, = –0.46V, The –ve E0 value indicates that the above reaction will never take place and silver, cannot displace Copper from a solution of Cu2+ ion. Instead the reverse reaction, would be feasible., , Notes, , (iv) It helps to predict whether a metal can liberate hydrogen from acids., Any metal which is above hydrogen in the electro chemical series can liberate, hydrogen from acid since it is a better reducing agent than hydrogen. Thus metals, like, Zinc, Magnesium, Calcium etc can displace hydrogen from HCl or H2SO4, but metals like Copper, silver etc cannot displace hydrogen from acid., , 13.12 NERNST EQUATION FOR ELECTRODE POTENTIAL, Nernst equation relates electrode potential to the concentration of ions in the, electrolyle. An electrode reaction is always written as reduction reaction. Let us, take a general example for a metal M., Mn+(aq) + ne, , M(s), , The Nernst equation for its electrode potentials is :, E = E0 –, where, , [M], 2.303 RT, log, [M n + ], nF, , (i), , E = Electrode potential, E0 = Standard electrode Potential (Reduction), R = gas constant in JK–1 mol–1, T = Temperature in Kelvin, F = Faraday constant, n = number of electrons involved in the electrode, , reaction, [Mn+] = molar concentration of Mn+ ion, [M] = concentration of pure solid metal taken as unity, Therefore,, , CHEMISTRY, , E, , = E0 –, , 1, 2.303 RT, log, [M n + ], nF, 379
Page 409 :
MODULE - 5, Chemical Dynamics, , Electrochemistry, , If we put the values of R, T and F in equation (i), R = 8.314 J K–1 mol–1, , F = 96500 Coulomb, T = 298 K, E = E0 –, , we have, Notes, , 1, 0.0591, log, [M n + ], n, , (ii), , For example : For copper electrode as half cell, Cu2+(aq) + 2e, here n = 2., , Cu(s), , E 0Cu 2+ / Cu = + 0.34V, E Cu 2+ / Cu = E 0Cu 2+ / Cu –, , 1, 0.0591, log, [Cu 2+ ], 2, , Since E 0Cu 2+ / Cu is 0.34 V the equation beccomes, = 0.34 – 0.0295 log, , 1, [Cu 2+ ], , Example 13.7 : Calculate the reduction potential for the following half cell at, 298 K, Ag+ (0.1M) + e–, , Ag(s), , E0 = 0.80V, E = E0 –, , 1, 0.0591, log, [Ag + ], n, 0.0591, 1, log, 1, 0.1, , = 0.80 –, , = 0.80 – 0.0591 log 10, = 0.80 – 0.0591 = 0.741V, 13.12.1 Nernst Equation for Cell emf, For a general cell reaction :, aA + bB, , xX + yY, , [X]x [Y] y, 2.303 RT, E cell = E cell –, log, [A]a [B]b, nF, 0, , 380, , CHEMISTRY
Page 410 :
MODULE - 5, , Electrochemistry, , Chemical Dynamics, , Thus, for the cell reaction :, Ni(s) + 2Ag+(aq), , Ni2+(aq) + 2Ag, , [Ni 2+ ], 2.303 RT, log, E cell = E cell –, [Ag + ]2, nF, 0, , Note: Only the ions are written in the fraction as concentration of pure solid or, liquid is taken as unity., , Notes, , To determine the value of n the reaction is written in two half reactions., Anode reaction (oxidation), Ni(s), , Ni2+(aq) + 2e–, , Cathode reaction (reduction), 2Ag+(aq) + 2e–, , 2Ag(s), , This can be represented in the form of a cell as, Ni | Ni2+ || Ag+ | Ag, The value of n = 2 as 2e– are exchange between anode and cathode, Example 13.8 : Calculate the EMF of the following cell at 298 K, Ni(s)|Ni2+ (0.001M) || Ag+ (0.1M) | Ag(s), E 0Ni2 + | Ni = 0.25V, , E 0Ag + | Ag = 0.80V, , From the given values first find, E0 cell = E0 cathode – E0 anode = 0.80 – (–0.25) V = 1.05V, E cell = E0cell –, , [Ni 2+ ], 0.0591, log, [Ag + ]2, 2, , 0.001, 10–3, 0.0591, log, =, 1.05, –, 0.0295, log, (0.1) 2, 2, 10 –2, = 1.05 + 0.0295 log 10–1 = 1.0795 V, = 1.05 –, , INTEXT QUESTION 13.2, 1. What type of process-spontaneous or non-spontaneous, occurs in, (i) electrolytz cell (ii) halvanic cell., 2. Atomic mass of setner is 108 u. What is its electrochemical equivalent?, 3. Why is salt bridge used for setting up of galvanic cells., 4. A galvanic cell is made in which the following reactioin occurs., Fe(s) + CuSOu(aq) → FeSou(aq) + Cu(s), Write down the cell notation, for this cell., CHEMISTRY, , 381
Page 411 :
MODULE - 5, Chemical Dynamics, , Electrochemistry, , 5. Prodict whether the following reaction is feasible or not?, Cu(s) + Zn2+(aq) → Cu2+(aq) + 2n(s), Given E°Cu2+ | Cu = +0.34 V and E°2n2+ | Zn = –0.76 V, , 13.13 BATTERIES AND FUEL CELLS, Notes, , The cells and batteries (combination of 2 or more cells) that we use for various, purposes from torch lights, cameras, watches to cars and bikes, are basically, of three types: dry cells or primary cells, storage cells or secondary cells and, fuel cells. A primary cell is the one that is consumed chemically when current, is drawn from it. A secondary cell is the one which after discharge can be, recharged by passing electricity through it and carrying out electrolysis. In fuel, cells the oxidizing and reducing agents are fed continuously to the cell from, outside., (i) Dry cell (Primary cell), It is the cell commonly used in torches transistor radios, portable music players,, clock etc. It is also known as Leclanche cell after the name of its inventer. It, consists of a zinc container which also acts as the anode and a carbon (graphite), rod which acts as the cathode. Around carbon rod, a moist paste of graphite,, ammonium chloride and manganese dioxide is placed which acts as the electrolyte, (Fig 13.10). The electrode reactions that occur are complex but can be written, in simplified way as:, Carbon rod, (cathode), , Zinc cup, (anode), , MnO2 +, carbon black, + NH4Cl paste, , Fig. 13.10: Dry cell, , 382, , CHEMISTRY
Page 412 :
MODULE - 5, , Electrochemistry, , Anodic process (oxidation): Zn(s) → Zn 2+ (aq) + 2e−, , Chemical Dynamics, , Cathodic process (reduction):, 2MnO 2 (s) +NH +4 (aq) e− → MnO(OH)(aq) + NH 3 (g), The ammonia gas evolved at cathode combines with Zn2+ ions produced at, anode to give complex ion [Zn(NH3)4]2+. A single dry cell has a potential of, nearly 1.5V. It can be used only once and cannot be recharged., , Notes, , (ii) Lead Storage Battery (Secondary cell), Lead storage batteries are used in automobiles (cars, bikes, trucks, buses etc), and in inverters., A lead storage battery consists of alternate plates of anodes and cathodes. The, anode consists of lead plate and the cathode of a grid of lead packed with lead, dioxide (PbO2). The electrolyte is an aqueous solution of sulphuric acid which, is 38% or 3.7 M. (See Fig. 13.11) The reactions that occur at the electrodes, during the discharge of the battery when it is used to draw current are:, Anodic process (oxidation): Pb(s) + SO 24− (aq) → PbSO 4 (s) + 2e−, Cathodic process (reduction):, PbO2 (s) + SO24− (aq) + 4H + (aq) + 2e − → PbSO4 (s) + 2H 2O(l), Net reaction: Pb(s) + PbO 2 (s) + 2H 2SO4 (aq) → 2PbSO4 (s) + 2H 2O(l), (Lead plates), Anode, , Cathode (lead grids packed, with PbO2 ), , 38% sulphuric, acid solution, , Fig. 13.11: Lead storage battery, , The battery can be recharged by passing current through it. Now it acts like, an electrolytic cell and the electrode reactions are reversed and the original, reactants are regenerated. The battery can be charged and discharged a large, number of times., , CHEMISTRY, , 383
Page 413 :
MODULE - 5, Chemical Dynamics, , Notes, , Electrochemistry, , (iii) Fuel Cells, The maximum amount of electricity is produced by thermal power plants which, use fossil fuel like coal or natural gas. Their efficiency is very low but these, plants can be run continuously by constant feeding of the fuel. Galvanic cells, have high efficiency but can be used only once and then have to be discarded,, because reactants can not be fed into them continuously. Fuel cells combine the, advantages of the two. They are efficient and can be used continuously. Most, of the fuel cells are based on combustion reactions of fuels like hydrogen,, methane, methanol etc which are fed continuously into fuel cells. One of the, most successful fuel cells uses the combustion reaction of hydrogen (Fig. 13.12)., Anode –, , H2O, , + Cathode, , Aqueous electrolyte, , H2, , O2, , Figure 13.12: Hydrogen-oxygen fuel cell, , The electrodes used in it are made of porus carbon impregnated with catalyst, (finely divided Pt or Pd). The electrolyte used is an aqueous solution of KOH., Hydrogen gas is bubbled around the anode and oxygen gas around the cathode., The electrode process are:, Anodic process (oxidation): 2H 2 (g) + 4OH − (aq) → 4H 2O + 4e −, Cathodic process (reduction): O 2 (g) + 2H 2O(l) + 4e − → 4OH − (aq), Overall process: 2H 2 (g) + O 2 (g) → 2H 2O(l), The cell potential of this cell is 0.9V. It was used as a power source in Apollo, space programme and water produced was used for drinking purpose by the, crew of the spacecraft., , 13.14 CELL EMF AND GIBBS ENERGY, The maximum amount of work that a cell in its standard state can perform is, given by, Wmax = –n FE0, , 384, , CHEMISTRY
Page 414 :
MODULE - 5, , Electrochemistry, , The significance of –ve sign is that the work is done by the cell. Since the maximum, amount of useful work which a system can perform is equal to decrease in Gibbs, energy thus, , Chemical Dynamics, , Wmax = ΔG0 = –nFE0, If ΔG0 calculated is negative, then the cell reaction is spontaneons otherwise not., Example 13.9 : Calculate the standard Gibb’s energy ΔG0 for the reaction, occurring in Daniell cell, Zn(s) + Cu2+(aq), , Notes, , Zn2+(aq) + Cu(s), , at 298 K. The E0 cell at this temperature is 1.1V. Is the reaction spontaneous., For Daniell cell, n = 2., ΔG0 = – nFE0, , Solution :, , ΔG0 = –2 × 96500 × 1.1, = –212,300J, = –212.3kJ, Since ΔE0 is –ve, the cell reaction is spontaneous, , INTEXT QUESTIONS 13.4, 1. Differentiate between electrolytic cell and galvanic cell., 2. What is a salt bridge? What is the role of the salt bridge?, 3. What is electrochemical series? List any two applications of the series., 4. With reference to the electrochemical series arrange the following metals, in the order in which they displace each other from their salt solutions., Al, Cu, Ge, Mg, Zn, Ag, , 13.15 CORROSION, Corrosion is the process of deterioration of metals due to their exposure to, environment. It slowly forms a layer of oxides, basic carbonates or other salts, on the surface of metals. Rusting of iron and tarnishing of silver objects are, common examples of corrosion. Corrosion results in huge material loss resulting, in damage to buildings, ships, bridges, machinery etc., Corrosion is essentially an electrochemical process involving anodic oxidation, of metals resulting in the formation of their ions and a cathodic process that, , CHEMISTRY, , 385
Page 415 :
MODULE - 5, Chemical Dynamics, , Electrochemistry, , consumes the electrons. Though rusting is a complex process. The following, reactions can be considered to be taking place. One spot on the surface of iron, becomes anode and undergoes oxidation., , Notes, , Fig. 13.13: Corrosion of iron, , Anodic process:, , 2Fe(s) → 2Fe2+ (aq) + 4e−, , E ° = −0.44V, , The electrons released here move through the metal to another place which acts, like a cathode. Here the reduction of oxygen takes place in presence of H+ ions., Cathodic proess: O 2 (g) + 4H + (aq) + 4e − → 2H 2O, , E ° = +1.23 V, , The overall process is, 2Fe(s) + O 2 (g) + 4H + (aq) → 2Fe 2+ (aq) + 2H 2O E °cell = 1.67, ° indicates that this reaction occurs spontaneously. Fe2+, The positive sign of Ecell, ions formed heroe are further oxidized to Fe3+ ions by atmospheric oxygen to, form rust, , 4Fe 2+ (aq) + O 2 (g) + 4H 2O(l) → 2Fe 2O3 (s) + 8H + (aq), Fe2 O3 (s) + xH 2O(l) → Fe2 O3 ⋅ xH 2O(s) (rust), Rusting requires the combined effect of oxygen and water. It is accelerated by, CO2 and SO2 (acidic conditions) and retarded by alkalis. It has been observed, that completely homogeneous iron does not rust., Protection against corrosion, There are two general methods used for protection of metallic objects against, corrosion., (a) application of protective layer to inhibit anodic process, (b) cathodic protection, 386, , CHEMISTRY
Page 416 :
MODULE - 5, , Electrochemistry, , Chemical Dynamics, , (a) Use of Protective Layer, Many substances are used for applying a protective layer to prevent or limit, corrosion:, (i) Machinery, tools and automobile engine parts are often protected by a thin, film of oil or grease., (ii) Metalic doors, windows, coolers are painted with a suitable paint to prevent, corrosion., (iii) In automobile industry, the chassis of vehicles is painted with phosphoric, acid which reacts with iron to form an insoluble film of iron (III) phosphate., (iv) Iron containers are made from tin plated sheets. Tin is less reactive than, iron and forms a protective layer. However, if tin layer is broken, rusting, °, starts because iron is more electropositive than tin (E 2+ = −0.44V, Fe, , and, , E ° 2+, Sn /Sn, , Notes, , /Fe, , = −0.14V). In contact with each other and with atmosphere,, , iron becomes anode and tin becomes cathode and rusting of iron begins., (b) Cathodic Protection, Cathodic protection of a metal can be done by coating it with a more reactive, or more electropositive metal with smaller electrode potential., (i) Galvanisation:, Iron sheets or other objects are coated with a layer of zinc by dipping them, in molten zinc or using electrolysis. Zinc layer is protected against corrosion, by a firmly sticking layer of ZnO. Even when the zinc layer is broken, zinc being, more electropestive than iron becomes the anode and iron, (E°, , Zn 2+ /Zn, , = −0.76 V; E°, , Fe2+ /Fe, , = −0.44 V) becomes the cathode, the cell, , reaction that occurs is, , Zn(s) + Fe2+ (aq) → Zn 2+ (aq) + Fe(s), Thus, any Fe2+ irons formed as a result of atmospheric oxidation are reduced, back to iron at the cost of zinc. Therefore, zinc coating becomes sacrificial, anode., (ii) Chromium plating (electroplating) is not only decorative, but also protects, iron against rusting. Like zinc, chromium is also more electropositive than iron, (E°, , Cr3+ /Cr, , = −0.74 V; E°, , Fe2+ /Fe, , = −0.44 V). Chromium itself is not affected by, , atmospheric oxygen. Its layer protects iron. Once this layer is broken, chromium, becomes the anode and iron the cathode and the following reaction occurs:, , 2Cr(s) + 3Fe2+ (aq) → 2Cr 3+ (aq) + 3Fe(s), , CHEMISTRY, , 387
Page 417 :
MODULE - 5, Chemical Dynamics, , Electrochemistry, , (iii) Cathodic protection can also be provided by attaching, rather than coating,, a piece of more reactive metal to the object to be protected. Ships, water pipes,, iron tanks etc. are often protected against corrosion by connecting a piece of, magnesium either directly or through a wire. Magnesium being more, °, °, electropositive than iron (E Mg 2+ /Mg = −2.36V; E Fe2+ /Fe = −0.44V) becomes, , Notes, , sacrificial anode. The following reaction occurs in the cell formed., , Mg(s) + Fe2+ (aq) → Mg 2+ (a) + Fe(s), , WHAT YOU HAVE LEARNT, , 388, , z, , Oxidation is a process in which electrons are lost by an atom or ion., , z, , Reduction is a process in which electrons are gained by an atom or ion., , z, , Atoms or ions undergoing oxidation are reducing agents and atoms or ions, undergoing reduction are oxidising agents., , z, , Oxidation number is the state of oxidation of an element in a compound,, which is calculated by a set of rules. It is based on the concept that electrons, in a covalent bond belong to the more electro negative element., , z, , Oxidation number of an atom in elemental form is zero. Otherwise the number, is always written with positive or negative sign., , z, , A substance, if in its molten state or aqueous solution conducts current is, called electrolyte and if it doesnot conduct electric current then it is called, non-electrolyte., , z, , Ions carry charge in an electrolyte from one point to other. The conduction, of electricity follows Ohm’s law., , z, , Reciprocal of resistance and resistivity are called conductance and, conductivity respectively., , z, , On dilution of a solution, its conductivity decreases while molar conductivity, increases., , z, , Electrolysis is a process in which electrical energy is used to decompose an, electrolyte into its ions and it is done in an electrolytic cell, , z, , Electrochemical cell or Galvanic cell produce electricity due to oxidation, and reduction reactions occurring in their half cells. Oxidation occurs at the, anode (negative electrode) and reduction at the cathode (positive electrode)., , CHEMISTRY
Page 418 :
MODULE - 5, , Electrochemistry, z, , A galvanic cell can be written in symbolic form as Anode |Electrolyte|, |Electrolyte| Cathode., , z, , The emf of a cell is the potential difference between two electrodes in the, open circuit., , z, , When a metal is dipped in its own salt solution then the potential of metal, with respect to solution is called electrode potential. This potential is, measured with respect to a reference electrode called Standard Hydrogen, electrode., , z, , Electrochemical series is the arrangement of electrodes in the order of their, increasing electrode potential., , z, , The cell emf is related to the electrode potential (reduction), Ecell = Ecathode – Eanode, The Nernst equation is, , z, , Chemical Dynamics, , Notes, , [Re d], 2.303RT, log, [Oxi], nF, The standard Gibbs energy of the cell reaction ΔG0 is related to the standard, cell emf as ΔG0 = –nFE0., E = E0 –, , z, , TERMINAL EXERCISE, 1., , Calculate the Oxidation number of the elements written in bold letters, [Cr(H2O)6]3+,, , 2., , [Fe (CN)6]3–,, , Balance the following reactions by oxidation number method, (a), , Fe2O3 + C, , (b) C6H6 + O2, 3., , Fe + CO, CO2 + H2O, , Balance the following reactions by ion-electron method:, (i) Zn + HNO3, (ii), , ClO3– + Mn2+, , (iii) Fe(OH)2 + H2O2, 4., , HC O 3– , Pb3O4, , Zn (NO3)2 + NO2 + H2O, MnO2 + Cl– in acidic medium, Fe(OH)3 + H2O in basic medium, , Define the following and give its units:, (i) Conductivity, (ii) Molar conductivity, , CHEMISTRY, , 389
Page 419 :
MODULE - 5, Chemical Dynamics, , Electrochemistry, , 5., , Draw a graph showing the variation in molar conductivity in a weak and a, strong electrolytes with concentration., , 6., , Explain why the molar conductivity of an electrolyte increases with dilution, while conductivity decreases on dilution., , 7., , The measured resistance of a conductance cell containing 7.5 × 10–3M, solution of KCl at 25ºC was 1005 ohms. Calculate (a) Conductivity (b), Molar conductivity of the solution. Given the cell constant 1.25 cm–1., , 8., , The conductivity of 0.05M solution of an electrolyte at 298 K is 0.0025, cm–1. Calculate the molar conductivity., , 9., , How much aluminium will be deposited on passing a current of 25 A for 10, hr by electrolysis of malten Al2O3?, , Notes, , 10. Equivalent mass of an element is 94 g. What is its electrochemical equivalent?, 11. Two electrolytic cells A and B containing ZnSO4 and CuSO4 solutions, respectively are connected in series on passing certain amount of electricity,, 15.2 g of zinc was deposited in cell A. How much copper would be deposited, in cell B?, 12. Explain the term standard electrode potential. How is it determined, experimentally., 13. Draw the diagram of the cell whose cell reaction is, Zn(s) + 2Ag+(aq), , Zn2+(aq) + 2Ag(s), , 14. For the cell, Mg | Mg2+ || Zn2+ | Zn, (i) Draw the cell diagram, (ii) Identify anode and cathode, (iii) Write cell reaction, (iv) Write Nernst equation for the cell, (v) Calculate E0 use the data given the table 13.2, 15. What are the functions of a salt bridge?, 16. Using electro chemical series predict whether the following reaction is feasible, or not, Ni(s) + Cu2+ (aq), , 390, , Cu(s) + Ni2+(aq), , CHEMISTRY
Page 422 :
MODULE - 5, , Electrochemistry, , (iv), , Al + 4 0H–, , NH3 + 9 OH– ] × 8, , Chemical Dynamics, , NH3 + 9 OH– ] × 3, , N O 3– + 6H20 + 8e–, 8Al + 3N O 3– + 18H2O + 5OH–, , 8Al (OH) 4– + NH3, , 13.2, 1., , Electrolytes give ions in the solution and these ions conduct electricity by, moving to their respective electrodes i.e. cations towards cathode and anions, towards anode., , 2., , Specific conductance is the conductance of a solution taken in a cell in which, two electrodes are 1cm apart and surface area of each one of them is 1cm2., , Notes, , Equivalent conductance is the conductance of all the ions furnished by an, equivalent of the electrolyte in a solution of given concentration., 3., , Conductance S; specific conductance Scm–1, , 4., , Nature of electrolyte (strong or weak), valency of its ions, speed of ions,, concentration and temperature., , 5., , See Fig. 13.2., , 6., , ∞ Al3+ + 3 ∞ S 2–, λ ∞m Al2 (SO4)3 = 2 λ m, λm O4, , 13.3, 1., , (i) non-spontaneous, , (ii) spontaneous, , 2., , 1.12 × 10–3 g c–1, , 3., , See section 13.8.2, , 4., , Fe(s) | Fe SO4(aq) || CuSO4(aq) | Cu(s), , 5., , In feasible since E° = –1.10 V, , 13.4, 1., , In electrolytic cell electrical energy is used for carrying out redox reaction, while in an electrochemical cell a redox reaction is used for producing, electrical energy., , 2., , See sec. 13.6.2, , 3., , See sec. 13.8, , 4., , Mg > Al > Zn > Fe > Cu > Ag., , CHEMISTRY, , 393
Page 423 :
MODULE - 5, , Chemical Kinetics, , Chemical Dynamics, , 14, Notes, , CHEMICAL KINETICS, , You know that a knowledge of Gibbs energy change in a given process can tell, you whether the process is feasible or not. However, the Gibbs energy decrease, does not indicate anything about the speed of a reaction. For example, the reaction, between hydrogen and oxygen to give water is accompanied by a large decrease, in Gibbs energy. However, you would not be able to observe the formation of, water, even if hydrogen and oxygen are kept in contact with each other for ever., On the other hand, there are reactions which take place instantaneously. For, example, when HCl is added to AgNO3, white precipitate of AgCl is formed, instantaneously. In this lesson we shall study the speed or rate of reactions which, are neither very slow nor very fast. We shall also study the factors that control the, rate of a reaction., , OBJECTIVES, After reading this lesson you will be able to :, , 394, , z, , explain the rate of a chemical reaction;, , z, , differentiate between average rate and instantaneous rate;, , z, , correlate the average and instantaneous rates with changes in concentrations, of various reactants and products;, , z, , explain the factors that affect the rate of a reaction;, , z, , define rate law and rate constant;, , z, , define order and molecularity of a reaction;, , z, , distinguish between order and molecularity;, , z, , derive rate law for first order reaction and define half life period for first, order reaction;, CHEMISTRY
Page 424 :
MODULE - 5, , Chemical Kinetics, z, , derive a relationship between half life and rate constant for first order reaction;, , z, , solve numericals on the relationships;, , z, , explain collision theory of reaction rates., , z, , explain the effect of temperature on reaction rate, and, , z, , explain Arrhenius equation and activation energy., , Chemical Dynamics, , Notes, , 14.1 RATE OF A CHEMICAL REACTION, When the reactants are mixed to perform a reaction, there are no products present, initially. As time passes, the concentration of the products increases and that of, the reactants decreases. The rate of any chemical reaction can be expressed as, the rate of the change in concentration of a reactant (or a product)., Rate of a chemical reaction = Change in concentration of a reactant or a product, Time taken, , mol litre −1, =, second, , Let us consider the following chemical reaction :, → 2NOBr(g), 2NO(g) + Br2(g) ⎯⎯, , The rate for this reaction can be determined by measuring the increase in the, molar concentration of NOBr at different time intervals., Let us see how we can express the rate of this reaction. You know that molar, concentration of a substance is expressed by enclosing the formula of the substance, in square bracket., For example, [NOBr] represents the molar concentration of NOBr., Let us suppose that [NOBr]1 is the molar concentration at time t1 and [NOBr]2 is, the molar concentration at time t2., Then, change in molar concentration = [NOBr]2 – [NOBr]1 = Δ[NOBr], Time required for the change = t2 – t1 = Δt, Here, Δ means change in the respective quantity., Therefore, the rate of formation of NOBr =, , Δ[NOBr], Δt, , This expression gives the rate of reaction in terms of NOBr., If the decrease in the molar concentration of NO or Br2 is measured we can write, the rate of the reaction with respect to NO as, =, CHEMISTRY, , −Δ[NO], Δt, , 395
Page 425 :
MODULE - 5, , Chemical Kinetics, , Chemical Dynamics, , and w.r.t. Br2 as =, , Notes, , −Δ[Br2 ], Δt, , Thus, the rate of a reaction can be expressed either in terms of reactants or, products. We find in the reaction mentioned above that two moles of NO react, with one mole of Br2. Therefore, the change in concentration of NO in a given, time Δt will be double than that for Br2. Thus, in order to make the rates with, respect to different reactants or products equal, the rate expression is divided by, the storichrometric coefficient in the balanced chemical equation., For example, in the equation,, → 2NOBr(g), 2NO(g) + Br2(g) ⎯⎯, , The rate of reaction with respect to reactants and products is expressed as, rate of reaction = +, , −Δ[Br2 ], 1 Δ[NOBr], 1 Δ[NOBr], =–, =, 2, Δt, 2, Δt, Δt, , 14.2 AVERAGE RATE AND INSTANTANEOUS RATE, The rate of a reaction depends on the concentration of reactants. As the reaction, proceeds the reactants get consumed and their concentration decreases with time., Therefore, the rate of reaction does not remain constant during the entire reaction., The rate of a reaction given as, , For example,, , Δ[concentration], gives an average rate., Δt, , Δ[NOBr], gives the average rate of reaction. Instantaneous rate of, Δt, , a reaction is the rate of reaction at any particular instant of time, we express, instantaneous rate by making Δt very small φ, d[NOBr], lim [NOBr], =, Δt → 0, Δt, dt, , When concentration of any of the reactants or products is plotted against time,, the graph obtained is as given below :, , Concentration, , Product, , Reactant, Time, , 396, , CHEMISTRY
Page 426 :
MODULE - 5, , Chemical Kinetics, , Chemical Dynamics, , For the reaction,, 2N2O5(g) ⎯⎯, → 2NO2(g) + O2(g), Average rate of reaction, =–, , 1 Δ[N 2O5 ], 1 Δ[NO 2 ], Δ[O2 ], =, =, 2, 2, Δt, Δt, Δt, , and instantaneous rate = −, , Notes, , 1 d [N 2 O5 ] 1 d [NO2 ] d [O 2 ], =, =, 2, dt, 2, dt, dt, , 14.3 FACTORS AFFECTING RATE OF A REACTION, The rate of a chemical reaction is affected by the following factors :, 1., , The concentration of reactants : Generally the rate of a reaction increases, as the concentration of the reactants is increased., , 2., , Temperature – A reaction is faster when the reaction temperature is, increased., , 3., , Presence of a catalyst – A catalyst alters the reaction rate without being, consumed by the reaction., , For example :, (i) Reaction between hydrogen and oxygen to form water is extremely slow. It, occurs very fast in the presence of platinum as catalyst., You would study these effects in more detail in the following sections of this, lesson., Example 14.1 : Express the average and instantaneous rate of the following, reaction, N2(g) + 3H2(g), in terms of, , 2NH3(g), , (i) rate of formation of NH3,, (ii) rate of disappearance N2, and, (iii) rate of disappearance of H2., , Write the different expressions for the rate of reactions., Solution : The expression for the three rates are :, Average rate of formation of NH3 =, , Δ[NH 3 ], Δt, , Average rate of disappearance of N2 = –, CHEMISTRY, , Δ[N 2 ], Δt, , 397
Page 427 :
MODULE - 5, , Chemical Kinetics, , Chemical Dynamics, , Notes, , Average rate of disappearance of H2 =, , Δ[H 2 ], Δt, , Instantaneous rate of formation of NH3 =, , d[NH3 ], dt, , Instantaneous rate of disappearance of N2 = –, Instantaneous rate of disappearance of H2 =, , d[N 2 ], dt, , d[H 2 ], dt, , To equate the three rates, divide each rate by the coefficient of the corresponding, substances in the balanced equation., Average rate = +, Instantaneous rate =, , Δ[N 2 ], 1 Δ[H 2 ], 1 Δ[NH3 ], =–, =–, 3 Δt, Δt, Δt, 2, , d[N 2 ], 1 d[H 2 ], 1 d[NH3 ], =–, =–, 3 dt, dt, dt, 2, , INTEXT QUESTIONS 14.1, 1. Which of the following units could be used to express the rate of reaction, between magnesium and hydrochloric acid?, (a) cm–3 s, (b) cm3 min–1, (c) cm3 s–1, (d) mol dm–3 min–1, 2. For the following reaction,, 2NO2(g) + F2(g) ⎯⎯, → 2NO2F(g), Write the expression for the average, rate of reaction in terms of :, (a) rate of formation of NO2F, (b) rate of disappearance of NO2, (c) rate of disappearance of F2, (d) equivalent rate of formation of product and disappearance of reactants., 3. Express the instantaneous rates of formation of product and disappearance, of reactants for the above reaction., 4. Explain why the reaction CO(g) + NO2(g) → CO2(g) + NO(g) occurs more, slowly when the volume of the system is increased., 398, , CHEMISTRY
Page 428 :
MODULE - 5, , Chemical Kinetics, , Chemical Dynamics, , 14.4 DEPENDENCE OF REACTION RATE UPON, CONCENTRATION, Rate law : If we follow a chemical reaction over a period of time, we find that its, rate slowly decreases as the reactants are consumed. We can say that the rate is, related to the concentration of the reactants. Rate is directly proportional to the, concentration of the reactants raised to some power., , Notes, , For example, consider the reaction, xA (g) + yB(g) → Products, , ...(i), , where a and b are coefficients of A and B respectively in the balanced equation., We can express the rate as, Rate α [A]x [B]y, or Rate = k [A]x [B]y, where k is the constant of proportionality., The above equation is known as the rate law for the reaction., Rate law is defined as the mathematical relationship between rate of a, reaction and the concentration of the reactants., It is important to note that x and y are not necessarily equal to the stoichiometric, coefficient of A and B in the reaction. The constant k in the rate law is called the, rate constant. It is numerically equal to the rate of the reaction if all the, concentrations were set equal to unity., rate = k [1]x [1]y, ∴, , rate = k, , It means that large values of k indicate fast reaction and small values of k indicate, slow reactions. Each reaction is characterised by its own rate constant, whose, value is dependent on the temperature, but independent of concentration of the, reactants., , 14.5 ORDER AND MOLECULARITY OF A REACTION, Order and molecularity are two properties of a chemical reaction. In this section, we shall learn about these., 14.5.1 Order of a Reaction, The powers to which the concentration terms are raised in the rate expression, describes the order of the reaction with respect to that particular reactant, In the rate law,, rate = k [A]x [B]y, CHEMISTRY, , 399
Page 429 :
MODULE - 5, Chemical Dynamics, , Chemical Kinetics, , the values of x and y are order of the reaction with respect to the reactants A and, B respectively. The sum of the powers x + y represents the overall order of the, reaction., For example in the reaction 2NO(g) + O2(g), , 2NO2(g), , The rate law as found by experiment is, Notes, , rate = k [NO]2 [O2]1, Here the order of reaction with respect to NO is 2 and with respect to O2 is 1. The, sum of the powers is termed as overall order of reaction. In the above example it, is 2 + 1 = 3., It must be remembered that order of a reaction must be determined experimentally, and cannot be deduced from the coefficients in the balanced equation, 2N2O5(g), , 4NO2(g) + O2(g), , The overall order is 1 and it is termed as first order reaction., The order of reaction can be 0, 1, 2, 3 called as zero order, first order, second, order and third order respectively. The order of a reaction can be a fraction as, well; for example the decomposition of ethanal to methane and carbon monoxide,, CH3 CHO (g), , 723 K, , CH4(g) + CO(g), , is experimentally found to the following rate law :, rate = k [CH3CHO]3/2, 14.5.2 Difference between Rate of Reaction and Rate Constant, Rate of reaction, 1. It is the speed at which the reactants, are converted into products. It is, measured as the rate of decrease, of concentration of reactant or, rate of increrase in the concentration, of the products., 2. It depends upon the initial, concentration of the reactant., , Rate constant, It is the proportionality constant, in the rate law and is defined as, the rate of the reaction when, the concentration of each of the, reactant is unity., It is independent of the initial, concentration of reactant., , 14.5.3 Molecularity of a Reaction, A reaction occurs when its reactants are mixed. At molecular level, this ‘mixing’, occurs through molecular collisions. The balanced chemical equation does not, always gives the correct picture of how a reaction is occurring at molecular level., For the following reaction to occur, MnO −4 + 5Fe 2+ + 8H + → Mn 2+ + 5Fe3+ + 4H 2O, 400, , CHEMISTRY
Page 430 :
MODULE - 5, , Chemical Kinetics, , apparently 14 ionic species must collide. However, the probability of a collision, involving more than 3 particles is practically zero. Therefore, this reaction must, be occurring in many steps, each step involving collision of not more than 3, species. Such reactions are called complex reactions and each step is called an, elementary reaction step. Some reactions occur in a single step and such, reactions are called simple reactions., Molecularity is the number of molecules, atoms or ions taking part in an, elementary reaction step, which must collide simultaneously in order to, bring about the chemical reaction., , Chemical Dynamics, , Notes, , Molecularity of a reactions is its characteristic property and reactions are, classified according the their molecularity., (i) Unimolecular reactions, These are reactions whose molecularity is one. For example, NH 4 NO 2 → N 2 + 2H 2O, 2N 2O5 → 2N 2O4 + O 2, (ii) Bimolecular reactions, These are the reactions with molecularity equal to two, for example:, 2HI → H 2 + I 2, [H + ], , CH 3COOC 2 H 5 + H 2O ⎯⎯⎯, → CH 3COOH + C 2 H 5OH., , (iii) Termolecular or trimolecular reactions, These reactions have molecularity equal to three, for example, 2NO + O 2 → 2NO 2, 2NO + Cl2 → 2NOCl, Termolecular reactions are rare because the probability of three molecules, colliding simultaneously is very small. Most of the reactions are unimolecular, or bimolecular., Molecularity of a reaction can only be a whole number, 1, 2 or 3. It is never, zero, fractional or more than 3., , 14.6 UNITS OF RATE CONSTANT, For zero order, Rate = k [A]º, Rate = k, , CHEMISTRY, , 401
Page 431 :
MODULE - 5, Chemical Dynamics, , Chemical Kinetics, , The unit for rate of a reaction is mol L–1 s–1. The unit of k for zero order is same, as that for rate., For a first order reaction, Rate = k [A]1, mol L−1, = k mol L–1, s, , Notes, , k = s–1, For a first order reaction, the unit for rate constant is time–1., For second order reaction, Rate = k [A]2, mol L−1, = k (mol L–1)2, s, , Therefore, k = mol–1 L s–1, In general for any order n the unit for rate constant k is given as, k = (mol L–1)1-n s–1, 14.6.1 Zero Order Reactions, Zero order reactions are those whose rate is proportional to zeroth power of, concentration, that is, the rate is independent of concentration of the reactant., Table: Compession of Order and molecularity, , Order, , 402, , Molecularity, , 1. It is the sum of powers of concentration, terms in the experimentally determined, rate equation, , It is the number of molecules, atoms, or ions which must collide simultaneously in order to bring about the chemcal reaction., , 2. Order of a reaction can be 1, 2, 3, zero, or fractional., , Molecularity can be 1, 2 or 3 only. It, cannot be zero or fractional., , 3. It is an experimental quantity., , It is a theoretical quantity and depends on the mechanism of the reaction., , 4. It is applicable to elementary as well, as complex reaction., , It is applicable to only elementary, reaction., , 5. For complex reactions, order is determined by the slovest step and the order, and molecularity of the slowest step, are the same., , For complex reactions, molecularity, has no meaning., , CHEMISTRY
Page 432 :
MODULE - 5, , Chemical Kinetics, , Chemical Dynamics, , Examples of Zero Order Reactions, Some reactions show zero order under certain conditions., (1) Decomposition of HI on the surface of gold catalyst when the pressure of, HI is high., (2) Photochemical combination of H2 and Cl2 to form HCl when carried out, over water., , Notes, , (3) Enzyme reactions when the substrate concentration is high in comparison, to the enzyme concentration., Consider the reaction:, , A → Products., Since the order of the reaction is zero, its differential rate equation is, Rate = −, , d[A], = k [A]0, dt, , Since anything raised to the power zero is equal to 1,, Rate = −, , d[A], =k, dt, , (1), , Separating the variables, we get, d [A] = − kdt, , Integrating both sides, , ∫ d [A] = −k ∫ dt, [A] = − kt + I, , (2), , Where [A] is the concentration of A at time t and I is the constant of integration., It can be evaluated from the initial conditions. When t = 0, [A] = [A]0, where, [A]0 is the initial concentration of the reactant. Substituting in equation (2), [A]0 = − k × 0 + I = 0 + I, ∴, , [A]0 = I, , (3), , Substituting for I in equation (2), [A] = –kt + [A]0, , (4), , This equation is the integrated rate equation for a zero order reaction. This, equation is an equation of a straight line and is of the form, y = mx + c, CHEMISTRY, , 403
Page 433 :
MODULE - 5, Chemical Dynamics, , Chemical Kinetics, , It [A] is plotted as a function of t, the plot is a straight line graph with intercept, equal to [A]0 and slope equal to –k., [A]o, , Notes, , Slope = –k, , [A], , t®, , Fig. Concentration versus time plot for a zero order reaction., , Units of k, Equation (4) can be rearranged as, kt = [A]0 – [A], or, , k=, , [A]0 − [A] concentration, =, t, time, , (5), , = Concentration time–1 = mol L–1s–1, Half-Life Period, t1/2, It is the time during which one-half of the initial amount of the reactant reacts., It is denoted by t1/2 or t0.5. It is also called half-change period. It can be, obtained from equation (4). Rearranging it, we get, t=, , [A]0 − [A], k, , (6), , 1, when t = t1/2, [A] = [A]0, 2, , that is after half-life period, one-half of the reactant is consumed. Also, one1, half reactant is left unreacted or [A] = [A]0 ., 2, , Putting in equation (6), , t1/2, 404, , 1, [A]0 − [A]0, 2, =, k, CHEMISTRY
Page 434 :
MODULE - 5, , Chemical Kinetics, , Chemical Dynamics, , t1/2, , [A]0, =, 2k, , (7), , Thus, the half-life period of a zero order reaction depends upon the initial, concentration of the reactant [A]0 and from the above equation, t1/2 ∝ [A]0, , Notes, , the half-life period is directly proportional to the initial concentration of the, reactant., Example: The rate constant of a zero order reaction is 2.4 × 10–3 mol L–1s–1., What would be the half-life of the reaction if the initial concentration of the, reactant is 1.2 × 10–2 mol L–1?, Solution, For a zero order reaction, t1/2 =, , [A]0, 2k, , Given: initial concentration of the reactant, [A]0 = 1.2 × 10–2 mol L–1 and, k = 2.4 × 10−3 mol L−1s −1., ∴, , t1/2 =, , 1.2 ×10−2 mol L−1, 2 × 2.4 ×10−3 mol L−1s −1, , ., , = 2.5 s., 14.6.2 First Order Reaction, We will now discuss how to determine the rate constant for a first order reaction., For the first order reaction, this equation would tell us how does the concentration, vary with time. The predicted variations can then be compared with the, experimental data to obtain the order of the reaction., Let us consider for the reaction A, , Product., , For first order reaction., rate =, , −d[A], = k1[A], dt, , where k1 is the rate constant,, , CHEMISTRY, , 405
Page 435 :
MODULE - 5, Chemical Dynamics, , Chemical Kinetics, , Rearranging the rate expression, we have :, − d[A], = k1dt, [A], , Integrating the two sides we get :, –1n [A] = k1t + constant, Notes, , ...(i), , where the constant can be determined from the initial conditions., Let [A]0 be concentration of [A] when t = 0 i.e., at the beginning of the reaction,, then –1n [A] = –1n [A]0, when t = 0, or, , constant = –1n [A]0, , Putting the value of the constant in equation, –1n [A] = k1t –1n[A]0, or, , –1n [A] + 1n [A]0 = k1t, , or, , 1n[A]0, = k1t, [A], [A], , 1, , 0, Rate constant, k1 = t .1n [A], , We can convert it in to log to the base 10, k1 =, , [A]0, 2.303, log, t, [A], , k1t, = log [A]0 – log [A], 2.303, −, , k1t, = log [A] – log [A]0, 2.303, , ⎛ k1 ⎞, ⎟ t + log [A]0, 2.303 ⎠, , log [A] = – ⎜⎝, The unit of k1 = time–1, , This equation has the same form as the equation of a straight line., y = mx + c, where m is the slope and c is the intercept. If we plot log10 [A] vs t, it would give, a straight line with a slope equal to –, , k1, . The rate constant, k1 can then be, 2.303, , calculated from the slope as shown in Fig. 14.1., , 406, , CHEMISTRY
Page 436 :
MODULE - 5, , Chemical Kinetics, , log10 (concentration), , Chemical Dynamics, , k1, , Slope = –, , 2.303, , Slope, , Notes, Time, , Fig. 14.1 : A graph of log10 (concentration) against time for a first-order reaction., , 14.6.3 Half-Life Period, The time taken for a reaction to reach the half-way stage i.e. when half of the, starting material has reacted is called half-life period of the reaction. It is denoted, by t1/2 or t0.5. Let us now see how the half-life period of a first order reaction is, calculated., You know that, ln[A] = 1n[A]0 – k1t, when [A] =, , 1, [A]0 (reactant reduces to half of the initial concentration), 2, , then, , t = t1/2 (half life period), , Therefore, we have, ⎧[A]0 ⎫, ⎬ = 1n[A]0 – k1t1/2, ⎩ 2 ⎭, , 1n ⎨, or, or, or, , ⎧[A]0 ⎫, ⎬ – 1n [A]0 = –k1t1/2, ⎩ 2 ⎭, , 1n ⎨, , 1n, , 1, = – k1t1/2, 2, , 1n2, , 0.693, , t1/2 = k = k, 1, 1, , You would notice that half-life period is independent of the initial concentration., Example 14.2 : The decomposition of hydrogen peroxide to water and oxygen., 2H2O2(l) ⎯⎯, → 2H2O (l) + O2(g), is a first order reaction with a rate constant of 0.0410 min–1. If we start with 0.20, M solution of H2O2, what will be concentration after 10 minutes?, CHEMISTRY, , 407
Page 437 :
MODULE - 5, Chemical Dynamics, , Chemical Kinetics, , Solution : We have equation for first order reaction as, [A]0, , 1, , k = t In [A], converting it into log10 base, Notes, , k =, , [A]0, 2.303, log, t, [A], , If [A] = x, after 10 minutes then subsituting the values of k and t, we have, 2.303, , 0.20, , 0.0410 (min–1) = 10(min) log x, or, , log, , 0.20, 10(min) (0.0410 min −1 ), =, = 0.178, x, 2.303, , Taking antilog, 0.20, = antilog 0.178 = 1.51, x, , Solving, , x =, , 0.20, = 0.132 mol litre–1., 1.51, , Example 14.3 : In example 14.2 if we start with initial concentration of 0.50 how, long will it take for this concentration to drop to 0.10 M., Solution : We have to find the time taken by the reaction starting from, concentration of H2O2 of 0.5M to concentration of 0.1 M., We have the equation,, k =, , [A]0, 2.303, log [A], t, , Substituting the values of k, [A]0 and [A] we have, 0.0410 min–1 =, , 2.303, 0.50, log, t, 0.10, , t = log 5 ×, or, , 2.303, 0.699 × 2.303, =, min, −1, 0.041, 0.0410 min, , t = 39 minutes, , Example 14.4 : In example 14.2, how long will it take for the sample to decompose, to 50%., Solution : When half the sample has decomposed, we have, 408, , CHEMISTRY
Page 438 :
MODULE - 5, , Chemical Kinetics, , Chemical Dynamics, , 0.693, t1/2 =, k, , Putting the value of k = 0.0414 min–1 we have, t=, , 0.693, min = 16.9 minutes, 0.0414, , Notes, , INTEXT QUESTIONS 14.2, 1. The rate of a certain reaction, A ⎯⎯→ Product is 1.0 × 10–3 mol/litre., When conc. A = 0.020M, what is rate constant k, if the reaction is :, (a) zero order, (b) first order, 2. For the following reaction, C2H4 (g) + I2(g), , C2H4I2(g), , the rate equation is, rate = k [C2H4(g)] [I2(g)]3/2, (a) What is the order of reaction with respect to each reactant?, (b) What is the overall order of reaction?, (c) What is the unit of k, if concentrations are measured in mol dm–3?, 3. The first order rate constant for the decomposition of C2H5Cl at 700K is 2.5, × 10–3 min–1. If the initial concentration is 0.01 mol L–1, calculate the time, required for the concentration of C2H5Cl to drop to half of its original value., , 14.7 COLLISION THEORY OF REACTION RATES, Collision theory was proposed to explain the rates of reactions occurring in gas, phase. It is largely based on kinetic molecular theory of gases. According to, this theory:, 1. A reaction would occur only when the reactant molecules collide with one, another. Thus, the rate of a reaction would depend upon the collision, frequency, ZAB which is the number of collisions involving one molecule each, of two reactants A and B occurring in unit volume of the gaseous reaction, mixture and in unit time., 2. Not all molecular collisions result in chemical reaction. Only effective, collisions can bring about chemical reaction. An effective collision is the one, CHEMISTRY, , 409
Page 439 :
MODULE - 5, Chemical Dynamics, , Chemical Kinetics, , in which the combined kinetic energy of colliding moleculas is equal to or, more than the activation energy of the reaction Ea. The fraction of molecules, possessing a minimum of activation energy Ea at a particular temperature, E, − a, , Notes, , T is given by e RT ., 3. For a reaction to occur not only the collision should be energetically, effective, the orientation of the colliding molecules should be such which, would permit the formation of new bonds. Consider, for example, the, decomposition reaction of HI, 2HI(g) → H 2 (g) + I 2 (g), The product would be formed only if during the collision the two H atoms are, near each other and so are the two I atoms. (Fig 14.3(a)). This would permit, the formation of bond between two H atoms and another bond between two, I atoms. If orientation is not proper (Fig 14.3(b), it is not possible as two I atoms, are too far apart for the bond formation. After the collision, the two HI molecules, rebound back without any reaction. Only a certain fraction of energetically, effective collisions would have proper orientation and would result in a chemical, reaction. This fraction is represented in terms of probability P, that molecules, during a given collision would have proper orientation. P is called probability, factor or steric factor., H—I, , H—I, , H—I, , I—H, , (a), , (b), , Fig. 14.3 (a) Proper orientation of two HI molecules (b) Improper orientation., , Taking these three factors into account, the rate of the reaction is given by the, relation, Rate = Z AB, , E, − a, RT, ⋅e, , ⋅P, , 14.7 DEPENDENCE OF REACTION RATE ON, TEMPERATURE, You have learnt earlier that an increase in temperature causes an increase in the, rate of reaction. The rate is about doubled, for many reactions, by a 10 degree, rise in temperature. How can we explain this behaviour?, In order for a chemical reaction to occur, the reacting molecules must collide, with each other. Only fast moving molecules i.e. the molecules having high energy, are able to react. There must be some minimum energy possessed by the reacting, molecules during the collision. This is called the threshold energy. All molecules, 410, , CHEMISTRY
Page 440 :
MODULE - 5, , Chemical Kinetics, , having energy higher than the threshold energy are therefore, capable of reacting., What would happen if we increase the number of molecules having higher energy?, More molecules will react. In other words rate of reaction would increase. Thus,, if we increase the temperature, we increase the rate of reaction. Let us see if we, can express it quantitatively., The change in energy that takes place during the course of a reaction is shown in, Fig. 14.2. The horizontal axis denotes the progress of the reaction and it indicates, the extent to which the reaction has progressed towards completion. The graph, indicates that the reactant molecules A and B must possess enough energy. This is, known as Activation energy, to climb the potential energy hill to form the activated, complex. All the reacting molecules do not possess energy equal to threshold, energy in their normal energy state. Therefore a certain extra energy needs to be, supplied so that their energy equals the threshold energy. The potential energy of, activated complex is maximum. The reaction thereafter proceeds to completion, (i.e. formation AB). Ea indicates the activation energy of forward reaction and, Ea′ is the activation energy of the backward reaction., , Chemical Dynamics, , Notes, , Energy, , energy of the Activated complex, , Ea, , A+B, , Ea¢, A + B, , Reactants, , AB Products, Progress of reaction, Fig. 14.2 Energy diagram for a reaction, , You know that rate of reaction = k [concentration]. If we have value of, concentration as unity, then rate of reaction is equal to the rate constant, k. The, rate constant k depends on the magnitude of the activation energy, Ea, and also, on the absolute temperature (T), k is small when Ea is very large or the temperature, is low., We can express this relationship by a mathematical equation known as Arrhenius, equation after its discoverer, S. Arrhenius as follows :, k = Ae–Ea/RT, Where A is a proportionality constant and is also known as frequency factor, R is, the gas constant. How can we utilise this relationship between k, Ea and T? We, , CHEMISTRY, , 411
Page 441 :
MODULE - 5, Chemical Dynamics, , Chemical Kinetics, , can calculate activation energy, if we measure the rate constant at two different, temperatures., Taking natural logarithm of equation, we get, ln k = lnA –, , Notes, , Ea, RT, , We can compare this equation with that of a straight line y = mx + c as follow :, ln k =, , –E a ⎛ 1 ⎞, ⎜ ⎟ + lnA, R ⎝T⎠, , The plot of in k vs 1/T gives a straight line whose slope is equal to –Ea/R and, whose intercept is In A (in Fig. 14.3)., , lnk, , 1, T, Fig. 14.3 Graphical determination of Ea., , We can also obtain Ea from k at two temperatures by direct computation., At T1, the equation becomes, k1 = Ae − Ea / RT1, At T2, we can write, k2 = Ae − Ea / RT2, k1, Ae – Ea / RT1, k2 = Ae – Ea / RT2, , Dividing k1 by k2 we get, , ⎛1, , k, , 1 ⎞, , –E a, 1, ⎜ – ⎟, ln k =, R ⎝ T1 T2 ⎠, 2, , taking natural logarithm, Converting into logarithm (base 10), , k1, , –E, , ⎛ T2 – T1 ⎞, , k2, , E, , ⎛ T2 – T1 ⎞, , a, ⎜, ⎟, log k =, 2.303R ⎝ T1 . T2 ⎠, 2, Multiply by –1 on both sides and inverting the fraction, , a, ⎜, ⎟, log k =, 2.303R ⎝ T1 . T2 ⎠, 1, , 412, , CHEMISTRY
Page 442 :
MODULE - 5, , Chemical Kinetics, , This equation can also be used to calculate the rate constant at some specific, temperature if Ea and k at some other temperature are known., , Chemical Dynamics, , Example 14.5 : What must be the value of Ea if the rate constant for a reaction is, doubled when the temperature increases from 300K to 310K?, k2, , Solution : Given k = 2, R = 8.31 JK–1, 1, T2 = 310 K, , Notes, , T1 = 300 K, Ea = ?, We have equation :, k2, , ⎛ T2 – T1 ⎞, , Ea, , log k = 2.303 R ⎜ T . T ⎟, ⎝ 1 2 ⎠, 1, substituting the values we have, Ea, , (310 – 300)K, , log 2 = 2.303 × 8.31 JK –1 (310K × 300K), solving, we have, Ea = 53.5 kJ, , INTEXT QUESTIONS 14.3, 1. As a rough rule of thumb, the rates of many reactions double for every 10ºC, rise in temperature? Explain., 2. The rate constant of a reaction at 288 K is 1.3 × 10–5 litre/mol. While at 323K, its rate constant is 8.0 × 10–3 lit./mol. What is the Ea for the reaction., 3. The rate of the reaction triples when temperature changes from 293 K to 323, K. Calculate the energy of activation for such a reaction., 4. H2(g) and O2(g) combine to form H2O(l) by an exothermic reaction. Why do, they not combine when kept together for any period of time., 5. What is an effective collision?, , WHAT YOU HAVE LEARNT, z, , The rate of a chemical reaction can be expressed as the rate of change in, concentration of a reactant or product., , CHEMISTRY, , 413
Page 443 :
MODULE - 5, Chemical Dynamics, , Chemical Kinetics, z, , The factors that affect the rate of a chemical reaction are : the concentration, of the reactants, temperature and catalyst., , z, , Rate law is defined as the mathematical relationship between rate of a reaction, with the concentration of the reactant., , z, , The constant k in the rate law is called the rate constant. It is numerically, equal to the rate of the reaction if all the concentrations were set equal to, unity., , z, , The sum of the powers to which the concentration terms are raised in the rate, expression describe the order of the reaction., , z, , The rate constant of a first order reaction, k1, is given by, , Notes, , k1 =, , [A]0, 1, In [A], t, , z, , The time taken for a reaction to reach the half way stage, i.e., when half of the, starting material has reacted is called half-life period of a reaction., , z, , According to the collision theory, a reaction occurs only when the reactant, molecules collide provided they have sufficient energy and proper orientation., , z, , The dependence of the rate constant on the magnitude of the activation energy,, Ea, and the absolute temperature, T, is given by the Arrhenius equation, k =, Ae–Ea/RT., , z, , The activation energy for the decomposition of a compound corresponds to, the difference between the energy of the reactants and the maximum on the, energy curve., , TERMINAL EXERCISE, 1. Sketch an energy vs progress of reaction diagram for a reaction. Indicate the, activation energy for both forward (Ea) and reverse reaction (E′a)., 2. For the reaction 2N2O5(g) → 4NO2(g) + O2(g), it is found that N2O5 is, decomposing at the rate of 0.02 mol/litre sec. Calculate to rate of reaction, defined as, , Δ [NO 2 ], with Δt in seconds., Δt, , 3. The rate constant for a certain first order decomposition reaction is 0.23 s–1, at 673K. Calculate the half-change period for this reaction., , 414, , CHEMISTRY
Page 444 :
MODULE - 5, , Chemical Kinetics, , 4. The rate constant for a certain first order reaction is 1.00 × 10–3 s–1 at 298K., The rate constant for the reaction is 1.4 × 10–2 s–1 at 323K. Calculate the, activation energy for this reaction., , Chemical Dynamics, , 5. The rate of a reaction was found to be 3.0 × 10–4 mol/litre second. What will, be the rate if it were expressed in the units mol/litre minute., 6. List the three factors that affect the rate of a chemical reaction., , Notes, , 7. For a certain first order reaction the concentration of the reactant decreases, from 2.00 mol/litre to 1.50 mol/litre in 64 minutes. Calculate the rate constant, for this reaction., 8. The rate constant for a certain first order reaction is 1.0 × 10–3 min–1 at 298K., If the activation energy is 10.0 k cal, calculate the rate constant at 323K., 9. The reaction, A2 + B ⎯⎯, → C+D, Occurs in the following steps, → 2A, (i) A2 ⎯⎯, → X, (ii) A + B ⎯⎯, → C+D, (iii) A + X ⎯⎯, , The order of this reaction is one. What is its molecularity. What one of the, three steps is the slowest or rate determining step?, , ANSWERS TO INTEXT QUESTIONS, 14.1, 1. d, 2. (i), , Δ [NO2 F], Δt, , (ii), , – Δ [NO2 ], Δt, , – Δ [F2 ], Δt, , (iv), , – Δ [F2 ], 1 Δ [NO2 F], 1 Δ [NO2 ], =–, =, 2, 2, Δt, Δt, Δt, , (iii), 3., , d [F2 ], 1 d [NO 2 F], 1 d [NO2 ], =–, =, 2, 2, dt, dt, dt, , 4. Increase in volume would decrease the pressure which would decrease the, rate of reaction., CHEMISTRY, , 415
Page 445 :
MODULE - 5, Chemical Dynamics, , Chemical Kinetics, , 14.2, 1. (a) 1.0 × 10–3 mol litre–1 s–1, (b) 5.0 × 10–2 s–1, 2. (a) First order with respect to C2H4 and 1.5 with respect to I2., (b) The over all order of reaction is 2.5, , Notes, , sec –1, (c) k = (mol dm –3 )3 / 2, , = mol–3/2 dm9/2 s–1., 2.303, 0.01 mol L–1, 3. (a) 2.5 × 10 (min ) = 10 min log10, x, –3, , –1, , (b) When half of the sample is decomposed, t1/2 =, , 0.693, min = 0.277 × 103 min = 2.77 × 102 min, 2.5 × 10 –3, , 14.3, 1. 10, 2. 34.0 K cal mol–1, 3. 28.82 kJ, 4. Molecules do not have enough energy to be equal to threshold energy., , 416, , CHEMISTRY
Page 446 :
MODULE - 5, , Adsorption and Catalysis, , Chemical Dynamics, , 15, Notes, , ADSORPTION AND CATALYSIS, , Surface of solids plays a crucial role in many physical and chemical phenomena., There are two main reasons for this special role. Firstly, the surface of a substance, interacts first with its surroundings. Secondly, the surface molecules are in a, different state as compared to the molecules in the interior of the solid. The, surface molecules interact more readily with other substances which come close, by and are responsible for many special properties. In this lesson we shall study, about two such properties – adsorption and catalysis., , OBJECTIVES, After reading this lesson you will be able to :, z, , define adsorption;, , z, , distinguish between physical adsorption and chemisorption;, , z, , list and explain the various factors that affect adsorption;, , z, , state Freundlich adsorption isotherm mathematically and explain it;, , z, , explain Langmuir isotherm;, , z, , define catalysis;, , z, , distinguish between homogeneous and heterogeneous catalysis, and, , z, , explain the role of activation energy in catalysis., , 15.1 ADSORPTION, The surface of a solid attracts and retains molecules of a gas or a dissolved, substance which comes in its contact. These molecules remain only at the surface, and do not go deeper into the bulk Fig. 15.2(a)., , CHEMISTRY, , 417
Page 447 :
MODULE - 5, Chemical Dynamics, , Adsorption and Catalysis, , The phenomenon of attracting and retaining the molecules of a gas or a, dissolved substance by the surface of a solid, resulting in their higher, concentration on the surface is called adsorption., The substance which gets adsorbed is called the adsorbate and the solid substance, which adsorbs is called the adsorbent., , Notes, , A molecule in the interior of a solid is surrounded by other molecules in all, directions (Fig. 15.1). However, a molecule at the surface is surrounded by other, molecules within the solid phase but not from the outside.Therefore, these surface, molecules have some unbalanced or residual forces., , –x–x–, –x–x–, x– x – x –, x– x – xx–– x – x – x –, –x– x – x – x – x – x –, x –x – x – x – x –, Fig. 15.1 : Molecules in the interior and at the surface of a solid, , Solid, Solid, Adsorbent, , Adsorbed, substance, , Adsorbed, substance, , (Adsorbate), , (a), , (b), Fig. 15.2 : (a) Adsorption (b) Absorption, , 15.1.1 Adsorption and Absorption, The phenomenon of adsorption is different from that of absorption. The latter, term implies that a substance is uniformly distributed throughout the body of a, solid, Fig. 15.2(b). If we leave a small lump of calcium chloride in open, it absorbs, water vapour (moisture) from air and after some time even starts dissolving in it., On the other hand if we keep a sample of silica gel in open, it adsorbs water, vapour on its surface as shown in Fig. 15.2 (a)., , 418, , CHEMISTRY
Page 448 :
MODULE - 5, , Adsorption and Catalysis, , Chemical Dynamics, , 15.1.2 Factors Affecting Adsorption, Adsorption occurs on the surface of almost all solids. However, the extent of, adsorption of a gas on the surface of a solid depends upon the following factors:, (i) Nature and surface area of the adsorbent, (ii) Nature of the adsorbed gas, , Notes, , (iii) Temperature, (iv) Pressure of the gas, Let us now discuss these factors briefly., (i) Nature and Surface Area of the Adsorbent, Different solids would adsorb different amounts of the same gas even under similar, conditions. Substances like charcoal and silica gel are excellent adsorbents. The, substances that are porous in nature and have rough surfaces are better adsorbents., The extent of adsorption also depends upon the surface area of the solid. Greater, the surface area, more is the surface available for adsorption and greater is the, adsorption. The surface area depends upon the particle size of the substance. A, cube of each side equal to 1cm has six faces. Each of them is a square with, surface area of 1cm2. Thus, the total surface area of this cube is 6 cm2 Fig. 15.3, (a). If its each side is divided into two equal halves, ½ cm long, and the cube is, divided into two equal halves, ½ cm long, and the cube is cut along the lines, indicated in the Fig (b), the cube would be divided into 8 smaller cubes with each, side 0.5 cm long [Fig. 15.3 (b)]. Surface area of each small cube would be (6 ×, 0.5 × 0.5) = 1.5 cm2 and the total surface area of all the 8 smaller cubes would be, 12 cm2 which is double the surface area of the original cube. If it is subdivided, into smaller cubes, each of side equal to 1 × 10–6 cm the surface area will increase, to 6 × 106 cm2 or 600 m2. The increase in surface area would result in greater, adsorption., 1 cm, , 1 cm, , 1 cm, (a), , (b), Fig. 15.3 : Subdivision of a cube, , CHEMISTRY, , 419
Page 449 :
MODULE - 5, Chemical Dynamics, , Adsorption and Catalysis, , Now we can explain why the solids that are porous in nature and have rough, surfaces are better adsorbents. It is so because each of these features increases, the surface area., (ii) The Nature of the Adsorbed Gas, , Notes, , The extent of adsorption also depends upon the nature of the gas. The gases, which are more easily liquifiable or are more soluble in water are more readily, adsorbed than others. For example, under similar conditions, the amount of SO2, or NH3 adsorbed by charcoal is much more than that of H2 or O2 gases. It is, because the intermolecular forces are stronger in more easily liquifiable gases,, therefore, they get adsorbed more strongly., (iii) Temperature, The extent of adsorption decreases with rise in temperature. For example, under, one atmosphere pressure, one gram of charcoal adsorbs about 10 cm3 of N2 gas, at 272 K, 20 cm3 at 248 K and 45 cm3 at 195 K., Adsorption is an exothermic process. The change in enthalpy when one mole of, a substance is adsorbed, is called enthalpy of adsorption. The adsorption process, is similar to the condensation process. The reverse process is called desorption, and is endothermic in nature. It is similar to the evaporation process. When a gas, is kept in contact with a solid adsorbent in a closed container, a dynamic equilibrium, is established in due course of time., gas, , +, , adsorbate, , solid, , ZZX gas adsorbed on the solid, YZZ, , +, , heat, , adsorbent, , Since the forward process (adsorption) is exothermic in nature, according to the, Le Chatelier's principle, it would be favoured at low temperature. Therefore, the, extent of adsorption would increase on decreasing the temperature and would, decrease on increasing the temperature., (iv) Pressure of the gas, At a constant temperature the extent of adsorption increases with increase in the, pressure of the gas (adsorbate). We shall study the relation between the two in, detail a little later., 15.1.3 Physical and Chemical Adsorption, Adsorption can be divided into two main categories – physical and chemical, adsorption., (i) Physical Adsorption, It is the common type of adsorption. The basic feature of physical adsorption is, that the adsorbate molecules are held at the surface of the adsorbent by weak van, 420, , CHEMISTRY
Page 450 :
MODULE - 5, , Adsorption and Catalysis, , der Waals forces. These are the forces that exist between particles of all matter., Because of their universal nature, these forces would operate between any, adsorbent and adsorbate pair. Therefore, the physical adsorption is observed on, surface of any solid. Only, the extent of adsorption varies according to the nature, of the adsorbent and adsorbate as discussed earlier., Physical adsorption is characterized by low enthalpy of adsorption, that is about, 10 – 40 kJ mol–1., , Chemical Dynamics, , Notes, , Another feature of the physical adsorption of a gas by a solid is that it is reversible, in nature and an equilibrium is established between the adsorbent and the adsorbate, as discussed earlier. Increase of pressure increases the adsorption and the release, of pressure desorbs the gas. When temperature is increased, the physical adsorption, decreases and when it is lowered, the adsorption increases. In physical adsorption,, several layers of adsorbate are adsorbed one over the other., (ii) Chemisorption or Chemical Adsorption, We have seen earlier that some unsaturated valancies exist on the surface of a, solid. Whenever a chemical combination takes place between the adsorbent and, the adsorbate the adsorption becomes very strong. This type of adsorption caused, by forces similar to chemical bonds between the adsorbent and the adsorbate is, called chemisorption or chemical adsorption., The enthalpy of chemisorption is as high as that of chemical bonds (bond enthalpies), and is in the range of 40 – 400 kJ mol–1. Chemisorption is highly specific and is, possible between a specific adsorbent – adsorbate pair. Like most of the chemical, changes it is irreversible. Attempts to release the adsorbed gas gives the gas and, some amount of a definite compound. For example, oxygen gas is chemisorbed, on tungsten. It is released from the surface of tungsten as a mixture of oxygen, and tungsten oxide. Unlike physical adsorption, chemisorption first increases and, then decreases with rise in temperature [Fig. 15.4 (b)]. This shows that, chemisorption has an energy of activation*. During chemisorption, only one layer, of adsorbate molecules is adsorbed. The main distinctions between physical, adsorption and chemisorption are summarized in Table 15.1., Chemisorption, Physical, adsorption, , Extent, of, adsorption, , Extent, of, adsorption, , Temperature ®, , (a), , Temperature ®, , (b), , Fig. 15.4 : Effect of temperature on (a) physical adsorption and (b) chemisorption., * You will learn more about energy of activation later in this lesson., CHEMISTRY, , 421
Page 451 :
MODULE - 5, Chemical Dynamics, , Adsorption and Catalysis, Table 15.1 : Physical Adsorption and Chemisorption, Physical Adsorption, , Notes, , Chemisorption, , 1. The forces operating between adsorbate, and adsorbent are the weak van der, Waals forces., , 1. The forces operating between adsorbate, and adsorbent are strong and similar to, chemical bonds., , 2. The enthalpy of adsorption is low and, ranges from 10 to 40 kJ mol–1., , 2. The enthalpy of adsorption is high, and ranges from 40 to 400 kJ mol–1., , 3. No activation energy is involved., , 3. Significant activation energy is involved., , 4. Adsorption occurs more readily at low, temperature and high pressure., , 4. Chemisorption occurs at relatively high, temperature and high pressure., , 5. It is not specific in nature. All gases are, adsorbed on all solids and no compounds, are formed., , 5. It is highly specific in nature and occurs, between those adsorbents and, adsorbates which have a possibility of, compound formation between them., , 6. It is reversible in nature. The gas is, desorbed on increasing the temperature, or decreasing the pressure., , 6. It is irreversible in nature. Desorption, also separates some amount of the, compound formed., , 7. Multilayer formation is common., , 7. Monolayer formation occurs., , 15.1.4 Adsorption Isotherms, x, , The extent of adsorption is measured in terms of the quantity, where, x is the, m, mass of the gas (adsorbate) adsorbed at equilibrium on mass m of the adsorbent., x, is the mass of the adsorbate adsorbed per unit mass of the adsorbent. The, m, x, graph showing variation in, with pressure(p) at a constant temperature is called, m, adsorption isotherm. Let us see the variation in extent of adsorption in case of, gases and of solutes from their solutions., (i) Adsorption of Gases, , 422, , T1< T <, 2 T, , 3, , T1, T2, , ®, , The adsorption isotherm of a gas, which is adsorbed on a solid is shown, in Fig. 15.5. It shows that the extent, of adsorption of a gas on a solid, increases with the increase in the, pressure of the gas, p at three, different constant temperatures. The, curves also show that the extent of, adsorption, decreases at a fixed, pressure as the temperature is, increased (see the dotted line)., , x, m, , T3, , p®, Fig. 17.5 : Adsorption isotherm of a gas, , CHEMISTRY
Page 452 :
MODULE - 5, , Adsorption and Catalysis, , Chemical Dynamics, , Freundlich Adsorption Isotherm, Freundlich gave an empirical mathematical relationship between the extent of, ⎛x⎞, , adsorption ⎜ ⎟ and the equilibrium pressure (p) of the gas as :, ⎝m⎠, 1, x, = k p n where n > 1, m, , Notes, , In this relation k is a constant at a given temperature and depends upon the nature, of the adsorbate and adsorbent. The value of n changes with pressure. It is 1 at, low pressures and increases with pressure. The relationship is valid at a constant, temperature. Therefore, it is called Freundlich Adsorption Isotherm. On taking, logarithm of the above equation, we get, log, , x, 1, = log k + log p, m, n, , This is an equation of a straight line and a plot of log, a straight line with slope, , x, against log p should be, m, , 1, as depicted in Fig. 15.6. In actual practice, a straight, n, , ®, , line is obtained provided the data at very low and very high pressures is neglected., , log, , x, m, , Intercept = log k, log p ®, , Fig. 15.6 : Plot of log, , x, against log p., m, , 15.1.5 Langmuir Adsortion Isotherm, Adsorption Isotherm : One of the drawbacks of the Freundlich adsorption, isotherm is that it fails at high pressure of the gas. Langmuir derived an adsorption, isotherm on theoretical considerations based on kinetic theory of gases. This is, , CHEMISTRY, , 423
Page 453 :
MODULE - 5, Chemical Dynamics, , Adsorption and Catalysis, , named as the Langmuir adosrption isotherm. This isotherm is based on the, assumption that every adsorption site is equivalent and that the ability of a particle, to bind there is independent of whether or not nearby sites are occupied. In his, derivation, Langmuir considered adsorption to consist of the following two, opposing processes :, Asorption of the gas molecules on the surface of the solid., , Notes, Desorption of the adsorbed molecules from the surface of the solid., Langmuir believed that eventually a dynamic equilibrium is established between, the above two opposing processes. He also assumed that the layer of the adsorbed, gas is only one molecule thick i.e., unimolecular. Since such type of adsorption is, obtained in the case of chemisorption. Langmuir adsorption isotherm works, particularly well for chemisorption., The Langmuir adsorption isotherm is represented by the relation., x, ap, =, m 1 + bp, , ...(15.1), , where a and b are two Langmuir parameters. At very high pressure, the above, isotherm acquires the limiting form., x a, =, m b, , (at very high pressure), , ...(15.2), , At very low pressure, Eq. (15.1) is reduced to x/m = ap (at very low pressure), ...(15.3), In order to determine the parameters a and b, Eq. (15.1) may be written in its, inverse form:, m 1 + bp, b 1, +, =, =, x, ap, a ap, , ...(15.4), , A plot of m/x against 1/p gives a straight line the slope and intercept equal to 1/a, and b/a, respectively. Thus, both parameters can be determined., The Langmuir isotherm, in the form of Eq. (15.1) is generally more successful in, interpreting the data than the Freundlich isotherm when a monolayer is formed. A, plot of x/m versus p is shown in (Fig15.7). At low pressures, according to Eq., (15.3), pressure x/m increases linearly with p. At high pressure according to Eq., (15.2), x/m becomes constant i.e. the surface is fully covered and change in pressure, has no effect and no further adsorption takes place, as is evident from Fig. 15.7., , 424, , CHEMISTRY
Page 454 :
MODULE - 5, , Adsorption and Catalysis, , Chemical Dynamics, , Notes, , Fig. 15.7 : Langmuir Adsorption isotherm., , (ii) Adsorption from Solutions., Adsorption occurs from solutions also. The solute gets adsorbed on the surface, of a solid adsorbent. Charcoal, a good adsorbent, is often used to adsorb acetic, acid, oxalic acid and organic dyestuffs from their aqueous solutions., The extent of adsorption,, , x, depends upon the concentration c of the solute., m, , Freundlich isotherm is applicable to adsorption from solutions when concentration, is used in place of pressure as shown below., 1, x, = k cn, m, , and in the logarithmic form as, log, The plot of log, , x, 1, = log k + log c, m, n, , x, against c is also a straight line, provided very low and very, m, , high concentrations are avoided., 15.1.6 Applications of Adsorption, The phenomenon of adsorption finds many applications, some of which are given, below:, 1. Activated charcoal is used in gas masks in which toxic gases are adsorbed, and air passes through it., 2. Silica gel packed in small cloth bags is used for adsorbing moisture in bottles, of medicine and in small electronic instruments., 3. Animal charcoal is used for decolourizing many compounds during their, manufacture., CHEMISTRY, , 425
Page 455 :
MODULE - 5, Chemical Dynamics, , Adsorption and Catalysis, , 4. In chromatography, the selective adsorption of different solutes on the surface, of solid adsorbent helps in their separation., 5. Mordants are used during dyeing process with certain dyes. In such cases,, the mordants fix the dye on the fabric by adsorption., , Notes, , INTEXT QUESTIONS 15.1, 1. Indicate which of the following statements are true or false. (T/F), (i) More easily liquifiable gases are adsorbed more strongly., (ii) Non-porous adsorbents would adsorb more quantity of a gas than, porous adsorbents under similar conditions., (iii) The extent of adsorption increases with rise in temperature., (iv) Chemisorption is highly specific in nature., (v) Adsorption can occur from solutions also., , 15.2 CATALYSIS, When hydrogen and oxygen gases are kept in contact with each other, no, observable reaction occurs. If we add a small piece of platinum gauge in the, mixture of these gases, the reaction occurs readily. Here platinum gauge speeds, up the reaction and is called a catalyst., A catalyst is a substance which changes the rate of a reaction but remains, chemically unchanged at the end of the reaction., The phenomenon of change of reaction rate by addition of a substance which, itself remains unchanged chemically is called catalysis. The following are some, more examples of catalysis:, (i) Decomposition of potassium chlorate occurs at high temperature. If a small, amount of the manganese dioxide is added, the decomposition occurs at, much lower temperature. Here, manganese dioxide acts as catalyst., 2 KClO3(s), , MnO 2 ( s ), 2KCl(s) + 3O2(g), ⎯⎯⎯⎯→, , (ii) The evolution of hydrogen by the reaction between zinc and hydrochloric, acid is catalysed by Cu2+(aq) ions., Cu 2+ ( aq ), Zn(s) + 2HCl(aq) ⎯⎯⎯⎯⎯, → ZnCl2(aq), , + H2(g), , (iii) The oxidation of hydrogen chloride gas by oxygen occurs more quickly if, the gases are passed over cupric chloride., CuCl2 ( s ), 4HCl(g) + O (g) ⎯⎯⎯⎯→, 2H O(g) + Cl (g), 2, , 426, , 2, , 2, , CHEMISTRY
Page 456 :
Adsorption and Catalysis, , Auto-catalysis, , MODULE - 5, Chemical Dynamics, , In certain reactions, one of the products of the reaction acts as the catalyst. For, example, the oxidation of oxalic acid by acidified potassium permanganate occurs, as, 2KMnO4(aq) + 3H2SO4(aq) + 5(COOH)2(aq), K2SO4(aq) + 2MnSO4(aq) + 8H2O(l) + 10 CO2(g), , At room temperature, the reaction is quite slow in the beginning. Gradually it, becomes fast due to the catalytic action of Mn2+ ions which are one of the products, as MnSO4 in the reaction., , Notes, , The phenomenon in which one of the products of a reaction acts as a catalyst, is known as auto-catalysis., Negative Catalysis, Some catalysts retard a reaction rather than speed it up. They are known as, negative catalysts. For example :, (i) Glycerol retards the decomposition of hydrogen peroxide., (ii) Phenol retards the oxidation of sulphurous acid., Promoters and Poisons, Certain substances increase or decrease the activity of the catalyst, although, by, themselves they do not show any catalytic activity., The substances which increase the activity of a catalyst are called promoters, and those which decrease the activity of a catalyst are called poisons. For example:, (i) In Haber’s process for the manufacture of ammonia, the catalytic activity of, iron is enhanced by molybdenum which acts as promoter., Fe( s ), ZZZZX, N2(g) + 3H2(g) YZZZZ, 2NH3(g), Mo( s ), , (ii) Copper promotes the catalytic activity of nickel during hydrogenation of oils., (iii)In Haber’s process the catalyst iron is poisoned by hydrogen sulphide H2S., (iv)In contact process for the manufacture of sulphuric acid, the catalyst platinum, is poisoned by even the traces of arsenious oxide As2O3., 15.2.1 General Characteristics of a Catalyst, The following are the general characteristics of a catalyst :, (i) A catalyst remains unchanged at the end of the reaction., , CHEMISTRY, , 427
Page 457 :
MODULE - 5, Chemical Dynamics, , Adsorption and Catalysis, , The amount and the chemical composition of a catalyst remain unchanged in a, catalytic reaction. However, the catalyst may undergo a physical change. For, example, manganese dioxide, which is used as a catalyst in thermal decomposition, of potassium chlorate becomes powder during the course of the reaction., (ii) A small quantity of the catalyst is generally enough., , Notes, , In most of the reactions, only a minute amount of catalyst is required. Only one, gram of Cu2+ ions is sufficient to catalyse the oxidation of 109 litres of sodium, sulphite solution. In some cases, the rate of reaction is directly proportional to the, concentration of catalyst present. Catalysis by acids or bases is usually of this type., (iii) A catalyst does not alter the position of equilibrium state of a reversible, reaction., A catalyst allows the equilibrium to be reached faster. However, it does not alter, the equilibrium composition of the reaction mixture. It is because, a catalyst, increases the rates of forward and backward reaction equally., (iv) Catalysts are generally specific in their action., Generally, one catalyst will change the rate of only one reaction. For example,, manganese dioxide catalyses the decomposition of potassium chlorate but not of, potassium perchlorate., (v) A catalyst cannot initiate a reaction., A catalyst can change the rate of a reaction which occurs even in the absence of, catalyst. It cannot start a reaction., (vi) The activity of a catalyst can be increased by the presence of promoters, and decreased by the presence of poisons., Presence of a promoter increases the activity of a catalyst, while the presence of, a poison decreases it., 15.2.2 Homogeneous and Heterogeneous Catalysis, The phenomenon of catalysis can be divided into two main types – homogeneous, and heterogeneous catalysis, on the bases of the number of phases present in the, reaction mixture (A phase is a homogeneous part of a system)., (a) Homogeneous Catalysis, When the catalyst is present in the same phase as the reactants, the phenomenon, is called homogeneous catalysis. For example :, (i) Nitric oxide catalyses the oxidation of sulphur dioxide to sulphur trioxide in, the lead chamber process., , 428, , CHEMISTRY
Page 458 :
MODULE - 5, , Adsorption and Catalysis, , Chemical Dynamics, , 2SO2(g), , + O2(g), , NO(g), , 2SO3(g), , (ii) Hydrogen ions catalyse the inversion of cane sugar, C12H22O11(aq) + H2O(aq), , H+(aq), , C6H12O6(aq) + C6H12O6(aq), Glucose, , Fructose, , Notes, , (b) Heterogeneous Catalysis, When the catalyst is present in a phase other than that of reactants the, phenomenon is called heterogeneous catalysis. For example :, (i) Iron (s) catalyses the formation of NH3 gas., Fe(s), N2 + 3H2 YZZZ, ZZZZ, X, Z 2NH3, , (ii) In contact process for the manufacture of sulphuric acid, platinized asbestos, is used as the catalyst, 2 SO2(g), , Pt(s), + O2(g) YZZZ, ZZZZ, X, Z 2SO3(g), , 15.2.3 Catalysis and Activation Energy, , Potential Energy, , We have seen that a catalyst increases the rate of a reaction. We explain it by, considering the Fig 15.8., , Ea, , Uncatalyzed, , E'a, Reactants, , Catalyzed, , Products, , Reaction Coordinate, Fig. 15.8 : Graphical representation of the effect of catalyst on a reaction., , In this figure Ea is the activation energy of uncatalysed reaction and E′a is the, activation energy of the catalysed reaction. A catalyst lowers the activation energy, as you can see in the figure (E′a < Ea). The reduction in activation energy is, achieved by providing an alternative pathway of lower energy for the reaction., You can also see in this figure that the relative energies of reactants and products, are not changed. The enthalpy change is the same for the catalysed and uncatalysed, reactions., CHEMISTRY, , 429
Page 459 :
MODULE - 5, , Adsorption and Catalysis, , Chemical Dynamics, , INTEXT QUESTIONS 15.2, 1. List any two characteristics of a catalyst., , Notes, , 2. A small amount of alcohol when added to a solution of sodium sulphite slows, down its oxidation to sodium sulphate. What type of catalyst is alcohol?, 3. How would the activation energy be affected in the above reaction (given in, Q.No.2) on adding the alcohol?, 4. Addition of molybdenum enhances the catalytic activity of iron in the Haber’s, process for the manufacture of ammonia. What are the substances like, molybdenum called?, , WHAT YOU HAVE LEARNT, z, , The phenomenon of attracting and retaining the molecules of a gas or of a, dissolved substance on the surface of a solid is called adsorption., , z, , The substance which gets adsorbed is called the adsorbate and the solid, substance which adsorbs is called the adsorbent., , z, , The substances that are porous in nature and have rough surfaces are better, adsorbent., , z, , Easily liquifiable gases are more readily adsorbed., , z, , Extent of adsorption decreases with rise in temperature and increases with, the increase in pressure of the gas., , z, , Physical adsorption is due to van der Waal forces and chemisorption is due to, forces similar to chemical bonds., , z, , Pressure dependence of adsorption of a gas at a constant temperature is given, by Freundich Adsorption Isotherm, 1, x, = k pn, m, , 430, , z, , A catalyst is the substance which changes the rate of a reaction, but itself, remains chemically unchanged during the reaction., , z, , The catalysts which increase the rate of a reaction are called the positive, catalysts while those which decrease the rate are called the negative catalysts., , z, , Auto catalysed reactions are those in which one of the products acts as the, catalyst., CHEMISTRY
Page 460 :
Adsorption and Catalysis, z, , A promoter enhances the activity of a catalyst while a poison hampers it., , z, , A catalyst can’t initiate a reaction, nor can it alter the position of equilibrium, state of a reversible reaction., , z, , When the catalyst is present in the same phase as the reactants it is called a, homogeneous catalyst., , z, , When the catalyst is present in a phase other than that of reactants it is called, a heterogenous catalyst., , z, , A catalyst changes the rate of a reaction by changing its path and the activation, energy., , MODULE - 5, Chemical Dynamics, , Notes, , TERMINAL EXERCISE, 1. What is the difference between adsorption and absorption?, 2. Distinguish between physical and chemical adsorption., 3. List the factors that affect adsorption., 4. What type of solids make better adsorbents?, 5. Easily liquifiable gases are adsorbed more readily. Explain., 6. What is ‘extent of adsorption’?, 7. How does extent of adsorption vary with temperature in case of (i) physical, adsorption and (ii) chemisorption? Depict graphically., 8. What is enthalpy of adsorption?, 9. Explain the effect of temperature on extent of physical adsorption with the, help of Le Chatelier’s Principle., 10. What is an adsorption isotherm?, 11. State mathematically Freundlich Adsorption Isotherm and depict it graphically., Under what conditions is it applicable., 12. Give the mathematical equation of Frundlich Isotherm for adsorption of solutes, from solutions., 13. Give any three applications of adsorption., 14. What is a (i) catalyst and (ii) negative catalyst?, 15. What are promoters and poisons? Give one example of each., CHEMISTRY, , 431
Page 461 :
MODULE - 5, Chemical Dynamics, , Adsorption and Catalysis, , 16. What is auto catalysis. Give one example., 17. Give any five characteristics of catalysis., 18. Distinguish between homogeneous and heterogeneous catalysis., 19. Give two examples each of homogeneous and heterogenous catalysis., , Notes, , 20. How does a catalyst change the rate of reaction. Explain with the help of, appropriate example., , ANSWERS TO THE INTEXT QUESTIONS, 15.1, (i) T,, , (ii) F,, , (iii) F,, , (iv) T,, , (v) T, , 15.2, 1. See text section 15.2.1, 2. Negative catalyst, 3. Increase, 4. Promoters, , 432, , CHEMISTRY
Page 462 :
Feed back on Lessons, Lesson Name, , Content, Difficult, , Interesting, , Language, Confusing, , Simple, , Complex, , Illustrations, Useful, , Not useful, , What You Have Learnt, Very helpful, , Not helpful, , Dear Learners,, You must have enjoyed going through your course books., It was our endeavor to make the study material relevant,, interactive and interesting. Production of material is a, two way process. Your feedback would help us improve, the study material. Do take a few minutes of your time, and fill-up the feedback form so that an interesting and, useful study material can be made., Thank you, Coordinators, (Chemistry), , Feed back on Questions, Lesson No., , Lesson Name, , ---Third fold----, , 1., 2., 3., 4., 5., 6., 7., 8., 9., 10., 11., 12., 13., 14., 15., ---Fourth fold----, , Final fold and seal, , Complete and Post the feedback form today, , Lesson, No., , Intext Questions, Useful, Not useful, , Terminal Questions, Easy, Diff., V. diff., , 1., 2., 3., 4., 5., 6., 7., 8., 9., 10., 11., 12., 13., 13., 14., 15., , Second Fold, , �, , �, , �