Page 1 :
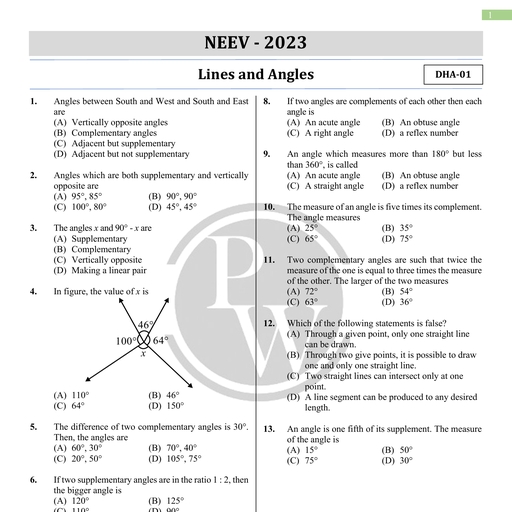
Chapter 10, , Wave Optics, , Chapter Contents, , e Introduction Introduction, , © Models of Light By now, you are well versed with laws that govern the formation of, H ‘ le images through lens, mirrors and various optical instruments. All, , © Huygens’ Princip what you have read was based on the fact that light travels in a, , © The Doppler’s Effect straight line. But from this chapter you will get to know that light, , does not always travel in straight line, indeed light is a wave. It, interferes, it diffracts and it even undergo polarization. This new, branch of Physics that deals with the wave nature of light is called, ¢ Interference of Light Wave “Wave Optics”., Reaa We will learn, how we reached the fact that light is a wave, its, interference experiments that confirmed that light indeed behaves, e Shape of Fringes on Screen _ like a wave. While reading this chapter you will also get to know, © Diffraction those queries which still puzzle you, why soapfilms and DVDs look, coloured when viewed at different angles. How shadows are not, ¢ Resolving Power of Optical —_ completely dark as they should be? Why polaroid sunglasses reduce, Instruments glare when ordinary cannot? So let us begin our discussion that led, © The Validity of Ray Optics _ the world to convincingly believe that light is a wave., , © Coherent and Incoherent, Sources of Light, , © ivlanteation MODELS OF LIGHT, , ¢ Some Important Definitions 1. Corpuscular model : In 1637, Descartes gave the corpuscular mode!, Formulae Chart of light. According to this model, a luminous body emits a stream of, particles in all directions. The particles are assumed to be very-very, © Quick Recap tiny. It explained the laws of reflection and refraction of light at an, interface using concepts of elastic collisions and momentum, conservation. Newton further developed this model and explained, sensation of colours at the retina of the eye and spectrum formed due, to prism. This model predicted that if the ray of light on refraction bends, towards the normal, then the speed of light would be greater in the, second medium. This prediction however proved wrong when Fermat, formulated his famous principle of least time. Although this law could, explain reflection and refraction, this law could not satisfactority explain phenomenon like interference, polarization and diffraction., , , , Aakash Educational Services Pvt. Ltd. - Regd. Office : Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph. 011-47623456
Page 2 :
110 Wave Optics Board & Competitive Exams., , 2. Wave model : The wave theory of light was first put forward by Christian Huygen in 1678. During that period,, everyone believed in Newton's corpuscular theory, which had satisfactorily explained the phenomenon of, reflection, refraction, the rectilinear propagation of light and the fact that light could propagate through vacuum., So empowering was Newton's authority that scientist fraternity highly believed in Newton's theory. When, Huygens put forward his wave theory, no one readily accepted it. Light as a wave model was not accepted, as it was believed that a wave necessarily require a medium for propagation. On the basis of his wave theory., Huygen explained satisfactorily the phenomenon of reflection, refraction and total internal reflection. This model, actually got acceptance when Thomas Young performed his famous interference experiments in 1801. Following, Young's experiments many experiments were carried out involving the interference and diffraction of light waves,, these experiments could only be satisfactorily explained by assuming a wave model of light. Thus around the, middle of nineteenth century, the wave theory gained well acceptance., , The only phenomenon that wave theory failed to explain at that time was propagation of light in vacuum, as, it was a firm belief that no wave could travel without a medium, it was only in the later part of the nineteenth, century, when Maxwell propounded his famous electromagnetic theory, could the nature of light waves be, understood properly. Maxwell had developed a set of equations describing the laws of electricity and magnetism, and using these equations he derived what is known as the wave equation of electromagnetic waves. From, , these equations Maxwell calculated theoretically the speed of electromagnetic waves as c = Ten which, HoFo, was later found quite close to the measured value of speed of light. According to Maxwell, light consists of, varying electric and magnetic field given E = E,cos(wt — kx), B = B,cos(wt — kx), These electric and magnetic fields are normal to each other and also normal to the propagation of light waves., , He advocated that changing electric field produces a time varying magnetic field, which in turn induces a, changing electric field, , , , HUYGENS’ PRINCIPLE, , Huygens’ theory is essentially based on a geometrical construction which allows us to determine the shape, of the wavefront at any time, if the shape of the wavefront at an earlier time is known. A wavefront is the locus, of the points which are in same phase; for example, if we drop a small stone in a calm pool of water, circular, ripples spread out from the point of impact, each point on the circumference of the circle (whose centre is, at the point of impact) oscillates with the same amplitude and same phase and thus we have a circular, wavefront. On the other hand, if we have a point source emanating waves in a uniform isotropic medium, the, locus of points which have the same amplitude and are in same phase are spheres. In this case we have, spherical wavefronts., , , , , , Spherical wavefronts of a point source, , The speed with which the wavefronts moves outwards from the source is called the speed of the wave. The, energy is carried in the direction perpendicular to the wavefront. At large distances from the source, a small, portion of the sphere can be considered as a plane and we have, what is known as a plane wave., , Aakash Educational Services Pvt. Ltd. - Regd. Office : Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph. 011-47623456
Page 3 :
Board & Competitive Exams., , , , Wave Optics 111, , Light Rays, , Plane wavefronts from distant source, If we know the shape of the wavefront at f = 0, then Huygens’ principle allows us to determine the shape of, the wavefront at a later time t. Thus, in a way Huygens’ principle is based on a geometrical construction, which allows us to determine the shape of the wavefront at any time, if the shape of the wavefront, , at an earlier time is known., , , , , , Knowledge Cloud, , , , 1. Reflection from plane mirror, , Incident, , Shapes of wavefronts In different situations, , wavefront (plane), , , , Reflected, wavefront (plane), , , , , , , , , , , , , , , , , , Incident, , , , 2. Reflection from curved mirror, |, Incident wavefront Reflected wavefront wavetror, (plane) (spherical) ee) nm, Reflected wavefront, Reflection from concave mirror pheccal), Reflection from convex mirror, , 3. Refraction from plane surface, , wavefront (plane), , , , , , , , Medium-1, , Medium-2, , , , Refracted, wavefront (plane), , , , Aakash Educational Services Pvt. Ltd. - Regd. Office : Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph. 011-47623456
Page 4 :
112 Wave Optics Board & Competitive Exams., , , , , , 4. Refraction through prism (Monochromatic beam), , Incident Emergent, wavefront wavefront, (plane) (plane), , 5. Refraction through lens, , a cpr (f, , , , , , , , wavefront, Incident wavefront (spherical) enn Emergent, (plane) (plane) wavefront, (spherical), Refraction through convex lens Refraction through concave lens, , , , , , According to Huygens’ principle, each point of a wavefront is a source of secondary disturbance and the, wavelets emanating from these points spread out in all directions with the speed of wave. The envelope of, these wavelets gives the shape of the new wavefront. Let us suppose we want to determine the shape of the, wavefront after a time interval of say Af. Then, with each point on the wavefront as centre, we draw spheres, of radius vAt, where v is the speed of the wave in that medium. If we draw a common tangent to all these, spheres, then we obtain an envelope which is again a sphere centered at point source., , Ss;, Ss,, % vAt, ay, f, s, SS, , , , S,S, = Shape of the wavefront at time t = ¢, SjS; = Shape of the wavefront at time t= t + At, , Drawback with Huygens’ Principle : Though Huygens’ Principle could very well explain the various, phenomenon unexplainable from corpuscular model, but it had one drawback. As we draw the wavefronts as, suggested by Huygen we also obtain a back wavefront which is not actually present. Huygen argued that, presence of backwave is avoided by assuming that the amplitude of the secondary wavelets is not uniform in, all directions, indeed it is maximum in the forward direction and zero in the backward direction. However this, assumption was later explained by rigrous wave theory., , Aakash Educational Services Pvt. Ltd. - Regd. Office : Aakash Tower, 8, Pusa Road, New Dethi-110005 Ph. 011-47623456
Page 5 :
Board & Competitive Exams. Wave Optics 113, , , , 1. | What is the shape of the wavefront in each of the following cases?, , (@) Light diverging from the point source., (©) Light emerging out of a convex lens when a point source Is placed at its focus., (c) The portion of the wavefront of light from a distant star., , , , , , Application of Huygens’ Principle to Study Refraction and Reflection, 1. Refraction of a Plane Wave: With the help of Huygens’ Principle we can derive Snell's law., (a) Refraction from Rarer to Denser Medium : Let v, and v, represents the speed of light in medium-1 and, , medium-2 respectively. Consider a plane wavefront PQ propagating in the direction P’P, incident on the, medium boundary at point P at an angle of incidence /. Let t be the time taken to travel from Q to B., , , , , P’ Incident ray incident, wavefront, , , , , , * QB=v,t, From the point P, draw a sphere of radius v2, let BR represent the forward tangent plane. It is refracted, wavefront at ¢., , PR = vet, , in = 08 vt _ vt, From APQB, sini PB” PB = PB tin ---(i), a PR Vat vat, , Also from APRB, sinr = PB PB => PB ane ---(ii), , Equating (i) and (ii), we have, , vit _ vot - Sint _ vy, , sini sinr sinr v2 .+ (iii), Now, if r <j (/.e., ray bends towards the normal), , sinr < sini, , ‘sini, , La], —>1 => +31 > y>v2, sinr v2, , i.e., speed of light in medium 1 is greater than that in medium 2. This is exactly what we studied in, Geometrical (Ray) optics., , Aakash Educational Services Pvt. Ltd. - Regd. Office : Aakash Tower, 8, Pusa Road, New Delhi-110005 Ph. 011-47623456