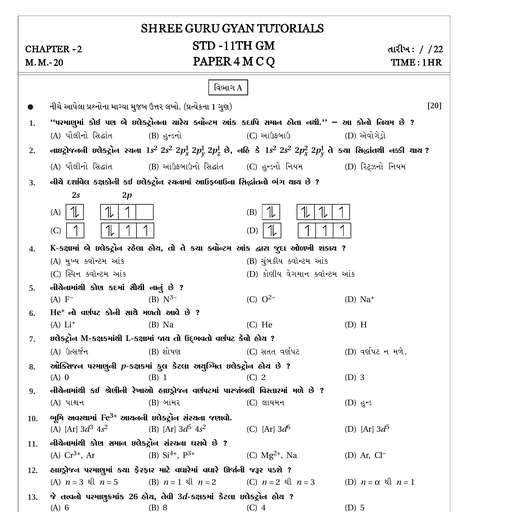
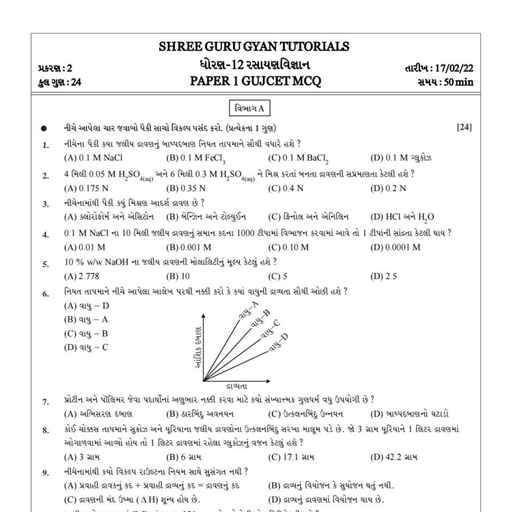
Page 1 :
SHREE GURU GYAN TUTORIALS, PH - 8980322383/9898553283, , Real Number, A collection of all rational and irrational numbers together is called a set of real numbers., , Euclid's Division Lemma, It states that, for any two positive integers a and b, we can find, two whole numbers q and r, such that, a = bq + r, where 0 £ r < b, Here, a = dividend, b = divisor, q = quotient, and r = remainder, i.e. Dividend = (Divisor ´ Quotient) + Remainder, , Fundamental Theorem of Arithmetic, Every composite number can be written as the product of, primes and this factorisation is unique, apart from the order in, which the prime factors occur. It is also called unique, factorisation theorem., , Prime Number, Euclid's Division Algorithm, , A number is called a prime number, if it has no factor other, than 1 and the number itself., , It is a technique to compute the highest common factor (HCF), of two given positive integers., , Composite Number, Highest Common Factor (HCF), A largest positive integer that divides given two positive integers, is called the highest common factor of these positive integers., , If a number has atleast one factor other than 1 and the, number itself, then this number is called a composite number., , Factor Tree, , Steps for Finding the HCF of Two Numbers, Step I Apply Euclid’s division lemma to a and b, for finding, whole numbers q and r such that, a = bq + r, 0 £ r < b, Step II If r = 0, then b is HCF of a and b. If r ¹ 0, then again, apply Euclid’s division lemma to b and r., Step III Continue this process till the remainder is zero. The, divisor at the last stage will be the required HCF., , Rational Numbers, , Irrational Numbers, , A number that can be, expressed as p/q, where p and, q are integers and q ¹ 0 is, called a rational number., , A number is called irrational, if, it cannot be expressed in the, form p/q, where p and q are, integers and q ¹ 0., , Decimal Expansion, The decimal expansion of every, rational number is either, terminating or non-terminating., , Non-terminating Decimal, Expansion, The rational number, which, does not terminate in the, process of division, is called, rational number having nonterminating decimal expansion., , Terminating Decimal Expansion, The rational number, which terminates after a finite number of steps, in process of division, is called rational number having terminating, decimal expansion., , SGGTC, , SHAILESHPRAJAPATI, , Factor tree of any composite number can be obtained by, following steps, Step I Divide the given composite number by a minimum, possible prime factor exactly and get the quotient., Step II Divide this quotient by a prime factor exactly and get, new quotient., Step III Repeat this process until the quotient become a prime, number., , Prime Factorisation Method, In this method, firstly expressed the given two or more, numbers into the product of prime numbers separately. Then,, , HCF of two or more numbers =, , LCM of two or more numbers =, , Product of the smallest power, of each common prime factor, involved in the numbers., , Product of the greatest power of, each prime factor involved in the, numbers., , Non-terminating Repeating, Decimal Expansion, The rational number, which does, not terminate but repeat the same, number again and again in the, process of division, is called, rational number having nonterminating repeating decimal, expansion or recurring decimal, expansion., , Non-terminating, Non-repeating Decimal, Expansion, The rational number, which, neither terminate nor repeat, the same number in process, of division, is called rational, number having nonterminating non-repeating, decimal expansion.