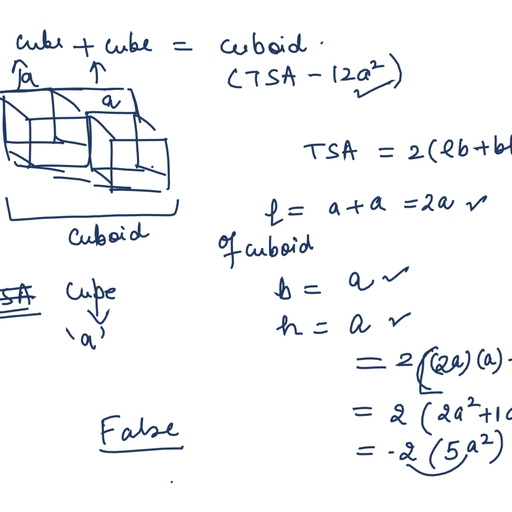
Page 1 :
Ic) Section B consists of 20 questions of 1 mark each. Any 16 questions are to be attempted., (a) Section C consists of 10 questions based on two Case Studies. Attempt any 8 questions., (b) Section A consists of 20 questions of 1 mark each. Any 16 questions are to be attempted., CBSE LATEST SAMPLE Paper., Paper, [Maximum Marks : 40], General Instructions:, lel There is no negative marking., SECTION - A, Section A consists of 20 questions. Any 16 questions are to be attempted., 1. The ratio of LCM and HCF of the least composite and the least prime numbers is, (b) 2:1, (a) 1:2, 2. The value of k for which the lines 5x + 7y 3 and 15x + 21y k coincide is, (c) 1:1, (d) 1:3, (b) 5, (a) 9, 3. A girl walks 200 m towards East and then 150 m towards North. The distance of the girl, (c) 7, (d) 18, from the starting point is, (a) 350 m, (b) 250 m, (c) 300 m, (d) 225 m, 4. The lengths of the diagonals of a rhombus are 24 cm and 32 cm, then the length of the, altitude of the rhombus is, (a) 12 cm, (b) 12.8 cm, (с) 19 сm, (d) 19.2 cm, 5. Two fair coins are tossed. What is the probability of getting at the most one head?, (a) 3/4, (b) 1/4, (c) 1/2, (d) 3/8, A. AABC-APQR. If AM and PN are altitudes of AABC and APQR respectively and AB? : PQ?, = 4:9, then AM:PN =, (a) 16:81, (d) 2:3, (b) 4:9, 1. If 2sin*B – cos²ß = 2, then ß is, (c) 3:2, (a) 0°, (c) 45°, * Pime factors of the denominator of a rational number with the decimal expansion 44.123, (b) 90°, (d) 30°, are, (a) 2,3, (b) 2,3,5, (c) 2,5, (d) 3,5, 9. The lines x = a and y = b, are, (a) intersecting, (b) parallel, (c) overlapping (d) None of these, Mathematics 125

Page 2 :
10. The distance of point A(-5, 6) from the origin is, (c) V11 units, (d) /61 units, (a) 11 units, (b) 61 units, 23, 11 If a =, then a is, 25, (a) rational, (c) whole number, (b) irrational, (d) integer, 12. If LCM (x, 18) = 36 and HCF (x, 18) = 2, then x is, (c) 4, (a) 2, (b) 3, (d) 5, 13. In AABC right angled at B, if tan A = 3, then cos A cos C- sin A sin C =, (a) -1, (b) 0, (c) 1, (d), (4), 2., 14. If the angles of AABC are in ratio 1:1:2, respectively (the largest angle being angle C), then, sec A, tan A, is, cot B, the value of, cosec B, (b) 을, (a) 0, (c) 1, (d), 2, 15. The number of revolutions made by a circular wheel of radius 0.7 m in rolling a distance of, 176 m is, (a) 22, 16. AABC is such that AB = 3 cm, BC = 2 cm, CA = 2.5 cm. If AABC ~ ADEF and EF =4 cm., then perimeter of ADEF is, (a) 7.5 cm, 17. In the figure, if DE || BC, AD = 3 cm, BD = 4 cm and BC = 14 cm, then DE equals, (b) 24, (c) 75, (d) 40, (b) 15 cm, (c) 22.5 cm (d) 30 cm, %3D, B, (a) 7 cm, (b) 6 cm, (c) 4 cm, (d) 3 cm, 4 sin B-3 cos B, 4 sin B+3 cos B, 18. If 4 tan B= 3, then, (a) 0, 3, (d), 4., (b), (c) 을, 19. One equation of a pair of dependent linear equations is -5x + 7y 2. The second equation, can be, (a) 10x + 14y +4 0, (c) -10x + 14y + 4 0, 20. A letter of English alphabets is chosen at random. What is the probability that it is a leier, of the word 'MATHEMATICS'?, (b) -10x -14y + 4 = 0, (d) 10x- 14y -4, 4, (a), 13, (b), 26, (c), 13, 11, (d), 26, 126 Together with Competency Based and Objective Type Questions-10

Page 3 :
22. Given below is the graph representing two linear equations by lines AB and CD respectively., Section B consists of 20 questions. Any 16 questions are to be attempted., What is the area of the triangle formed by these two lines and the line x = 0?, 21. If sum of two numbers is 1215 and their HCF is 81, then the possible number of pairs o, SECTION- B, such numbers are, (b) 3, (a) 2, (c) 4, (d) 5, 4 A, 3-, 2-, X, 4-3, O., -1-, 2., 3., 4., -2D, 4., Y', (a) 3 sq. units, (b) 4 sq. units, (c) 6 sq. units, (d) 8 sq. units, 23. If tan a + cot a = 2, then tan20a + cot20a =, %3D, (c) 20, 24. If 217x+ 131ly = 913, 131x + 217y = 827, then x +y is, (a) 0, (b) 2, (d) 220, %3D, (a) 5, (b) 6, (c) 7, (d) 8, 25. The LCM of two prime numbers p and q(p > q) is 221. Find the value of 3p - q., (a) 4, (b) 28, (c) 38, (d) 48, 20. A card is drawn from a well shuffled deck of cards. What is the probability that the card, drawn is neither a king nor a queen?, 11, (a), 12, (b), 13, 11, (c), 26, (d), 52, wo fair dice are rolled simultaneously. The probability that 5 will come up at least once is, 12, (c), 5, (a), 23, (d), 36, 11, 36, (b), 36, 36, Mathematics 127

Page 4 :
respectively. The length of the side of largest square FDGB that can be inscribed in the, 29. The vertices of a parallelogram in order are A(1, 2), B(4, y), C(x, 6) and D(3, 5). Then, 28. If 1 + sin'a = 3 sin a cos a, then values of cot a are, (c) 1, 2, (a) -1, 1, (b) 0, 1, (d) -1,-1, a, (x, y) is, (a) (6, 3), (b) (3, 6), (c) (5, 6), (d) (1, 4), 30. In the given figure, ZACB ZCDA, AC = 8 cm, AD = 3 cm, then BD is, %3D, C, 8 cm, 3 cm, (b), 22, cm, (c), 55, cm, 3, 64, cm, cm, 31. The equation of the perpendicular bisector of line segment joining points A (4,5) and B(-2,3), is, (a) 2x-y +7= 0, (b) 3x+ 2y-7 = 0, (c) 3x-y-7 = 0, (d) 3x +y-7=0, 32. In the given figure, D is the mid-point of BC, then the value of, cot y, is, cot x°, C, D., (a) 2, (b), (c), (d) 4, 33. The smallest number by which-, 13, after two decimal places is, should be multiplied so that its decimal expansion terminates, 13, (a), 100, 13, (b), 10, 10, 100, (d), 13, 13, 34. Sides AB and BE of a right triangle, right angled at B are of lengths 16 cm and o c, triangle ABE is, 128 7ogether with® Competency Based and Objective Type Questions-10, 113

Page 5 :
35. Point P divides the line segment joining R (-1, 3) and S (9, 8) in ratio k : 1. If P lies on, 16 cm, B, 8 cm, 16, (b), 8., (a), cm, (c), cm, 3, cm, (d), cm, the line x-y+ 2 = 0, then value of k is, 1., (a), (b) =, (c) -, (d), 3, le the figure given below, ABCD is a square of side 14 cm with E, F, G and H as the mid, ints of sides AB, BC, CD and DA respectively. The area of the shaded portion is, F, C, (a) 44 cm2, (d), 49, (b) 49 cm2, (c) 98 cm?, cm?, 31. Given below is the picture of the Olympic rings made by taking five congruent circles of, radius I cm each, intersecting in such a way that the chord formed by joining the point of, Intersection of two circles is also of length 1 cm. Total area of all the dotted regions assuming, the thickness of the rings to be negligible is, B, Mathematics 129